Creating Mathematical Experience in the Classroom - The Constant Sum Region: Regular \(n\)-gons

A follow-up question in Rethinking Proof (de Villiers, 1999) asks students to extend the triangle result to polygons with "a similar property." Since the proof of the initial theorem depends on the congruency of the sides of the triangle, the students tended to extend the results directly to equilateral polygons. However, the result does not generalize in this way, since there are points on the interior of most equilateral polygons for which the perpendicular from the point does not intersect the side, but rather extends outside the polygon, as in Figure 2.

Figure 2: Distance to the Side of a Hexagon

To set the stage for the first open question, I pointed out that the conjecture could be modified to read "the sum of the distances from a point on the interior of an equilateral polygon to the lines containing the sides is constant." This then could be proven in the same manner as the original case. However, the following question remained:

For a given equilateral polygon, what is the region for which the sum of the distances to the sides of the polygon from a point on the interior is constant?

One of the results of posing the question was the apparent awkwardness of referring to "the sum of the distances to the sides of the polygon from a point on the interior is constant" each time we wanted to discuss the question. As a result, the class came up with the following definitions.

Definition: The constant sum region (CSR) of a polygon is the region interior to the polygon for which the sum of the distances to the sides is constant.

Definition: The distance sum of a point is the sum of the distances from the point to the sides of a given polygon.

The distance sum of a point was defined after the class discussion on the CSR, and thus was not incorporated into its definition. The role definition plays in mathematics was not well understood by most students in the class. This was illustrated by earlier conversations in which Euclid's definition of a parallelogram was characterized as "wrong" since it was not the same as the one the teachers were familiar with. Having the participants in the class experience first-hand the creation of definitions out of need appeared to be a valuable lesson.

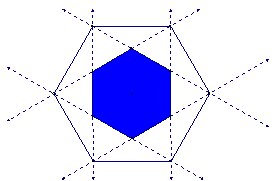
The first conjecture was quick in coming. Before the end of the class in which the question was posed, one student had constructed the constant sum region for a regular hexagon and made a conjecture. It is interesting to note that this student was one who had a minimal mathematics background. Following some suggestions I gave, the student made this conjecture.

Conjecture: The CSR for a regular hexagon is a smaller regular hexagon that has been rotated 30 degrees with respect to the original hexagon. The area of the CSR is one third of the area of the original hexagon, and the length of a side is approximately .577 the length of the side of the original. (L. Miller)

Figure 3: The CSR for a Regular Hexagon

The ease with which the conjecture was made is due in large part to the construction and measurement tools available in GSP. The fact that the conjecture contains an approximation is to be expected, as the conjecture was based on GSP measurements. In proving this conjecture, the students discovered that 0.577 is an approximation for.

Note: The students whose work is cited in this article have all given permission for their names to be used.

At this point some students decided to investigate other regular polygons. In addition, proofs of parts of this conjecture were made by several different students using a variety of arguments. They all assumed the CSR of a regular polygon is a hexagon, a point that was only informally argued. Given the time limits of the class, it seemed reasonable to assume this result. The results at this point in the project are summarized in the following conjectures and theorems. In proving parts of his original conjecture, L. Miller's understanding of the relationships deepened, as exemplified by his recognition of the actual ratio of the side of a regular hexagon to the side of its CSR.

Theorem: Assuming the CSR region for a regular polygon is a hexagon, it is an equiangular hexagon. (F. Rocha)

Theorem: The ratio of the area of a regular hexagon and its CSR is 3:1 and the ratio of the side of the regular hexagon to the side of the CSR is . (L. Miller, L. Cooke, R. Rocha)

Conjecture: The CSR for a regular 2n-gon is a regular 2n-gon, while the CSR for a regular (2n  + 1)-gon is a regular (4n  + 2)-gon. (M. Castro, L. Cooke)

In the course of the next few weeks, several students actively worked on the question of the CSR for equilateral polygons. F. Rocha proved that the CSR for a regular hexagon is not only equiangular, but is in fact regular. L. Cooke extended his analysis to regular 2n-gons and (2n  + 1)-gons. This work can be summarized by the following conjectures. L. Cooke proved the last three conjectures only for a number of specific values of n , but his methods would lend themselves to a proof of the general case stated in each conjecture.

Conjecture: The sides of the CSR for a 4n-gon are parallel to the sides of the 4n -gon.

Conjecture: Let  be the central angle (the angle formed by joining two adjacent vertices to the center of the regular n-gon) of a regular 2n-gon, n  > 2. Then the ratio of the length of the sides of the 2n -gon to its CSR is . The ratio of the areas is .

Conjecture: The length of the apothem (the segment measuring the distance from the center to a side) of the CSR for any regular n-gon is half the length of the side of the n -gon.

Conjecture: For any (2n+1)-gon, n  > 1, and its CSR, the ratio of the lengths of the sides is and the ratio of the areas is .