We owe many familiar area and volume formulas to Archimedes. For example, he proved that the area \(A\) of a circle equals half its circumference \(C\) multiplied by the radius \(r,\) i.e. \(A=\frac{1}{2}Cr.\)

This formula is often "explained" by the following picture, which shows that if you cut up the circle into an even number of equal sectors, the sectors can be reassembled into a figure that looks approximately like a parallelogram whose base is half the circumference of the circle and whose height is the radius. Click on the image below to see an animation.

Archimedes derived many formulas that are familiar to us today for computing relationships among volumes of spheres, cylinders, and paraboloids. How was he able to discover these formulas? About one hundred years ago, an old Greek manuscript containing works by Archimedes was found which explained his Method, based on the Law of the Lever. The exciting story of this manuscript can be found here.

Editor's note: This article was originally published in Convergence in 2005. We are pleased to "reprint" it in June 2016 with GeoGebra applets hosted by GeoGebraTube. In addition to viewing these applets in the webpages of this article, you may view and download all of the applets that appear in the article directly from GeoGebraTube at http://tube.geogebra.org/m/uYeV3NC8?doneurl=%2F.

Suppose two weights, W and w, are placed on a horizontal weightless stick that rests on a support, called the fulcrum. If W is at a distance D from the fulcrum and w is at a distance d from the fulcrum, then the system will balance if and only if WD=wd.

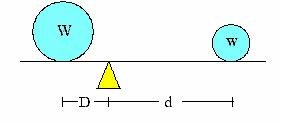
In order to determine the volume of a particular solid, Archimedes would try to find some other solid whose volume he knew, that could be placed in equilibrium with the unknown solid. Then by using the Law of the Lever he could solve for the volume of the unknown solid.

Example:

Suppose we have an orange that we know weighs 4 ounces. By trial and error, we find that when the orange is 2 cm from the fulcrum and a potato is 3 cm from the fulcrum on the opposite side, the system balances. By the Law of the Lever, 4×2 = p×3 (where p is the weight of the potato). Hence p = 8/3 = 2 2/3 oz.

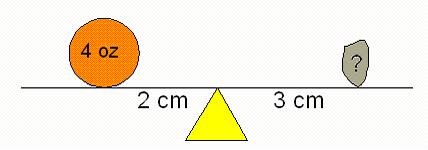
This is precisely the idea that Archimedes used to "discover" the volumes of certain solids. For example, he used his method to find the formula for the volume of a sphere.

Recall the following information about cylinders and cones with radius r and height h:

Suppose a sphere with radius r is placed inside a cylinder whose height and radius both equal the diameter of the sphere. Also suppose that a cone with the same radius and height also fits inside the cylinder, as shown below.

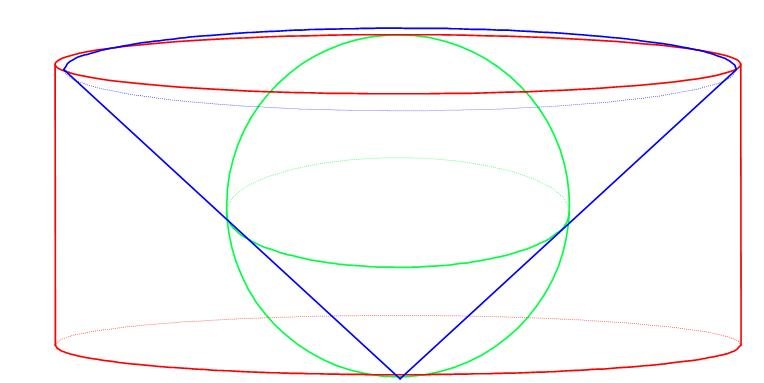
We place the solids on an axis as follows:

For any point S on the diameter AC of the sphere, suppose we look at a cross section of the three solids obtained by slicing the three solids with a plane containing point S and parallel to the base of the cylinder. The cross-sections are all circles with radii SR, SP, and SN, respectively. What Archimedes discovered was that if the cross-sections of the cone and sphere are moved to H (where |HA| = |AC|), then they will exactly balance the cross section of the cylinder, where HC is the line of balance and the fulcrum is placed at A.

This is not hard to show. If the radius of the sphere is \(r\), the origin is at \(A\), and the \(x\) coordinate of \(S\) is \(x\), then the cross-section of the sphere has area \(\pi(r^2-(x-r)^2)=\pi(2r x-x^2)\), the cross-section of the cone has area \(\pi x^2\), and the cross-section of the cylinder has area \(4\pi r^2\). So according to the law of the lever, in order for the above balancing relationship to hold we need to following equation to be true: \[2r\left[\pi x^2+\pi(2r x-x^2)\right]=4\pi r^2 x\] which can easily be verified.

The applet below illustrates the balancing relationship in Proposition 2 of Archimedes' The Method.

Since this balance holds for every cross section, it follows that if the entire sphere and cone are moved so that their centers of gravity are at H, they will exactly balance the cylinder, left where it is. By symmetry, the center of gravity of the cylinder is at K, so by the law of the lever,

\[\left(\mbox{Volume of Sphere}+\mbox{Volume of Cone}\right)\times|AH|=(\mbox{Volume of Cylinder})\times|AK|.\]

Since \(|AH| = 2|AK|,\) it follows that

\[2\left(\mbox{Volume of Sphere}+\mbox{Volume of Cone}\right)=\mbox{Volume of Cylinder}\] or \[\mbox{Volume of Sphere}=\frac12\left(\mbox{Volume of Cylinder}\right)-\mbox{Volume of Cone}.\] Finally, using the fact that the volume of the cone is 1/3 the volume of the cylinder, we find that the volume of the sphere is 1/6 the volume of the cylinder. Now since the cylinder's height and radius are both \(2r\) (where \(r\) is the radius of the sphere), the volume of the cylinder is \[\pi(\mbox{radius})^2\cdot (\mbox{height})=\pi(2r)^2\cdot(2r)=8\pi r^3\] so the volume of the sphere is \[V_{\mbox{sphere}}=\frac16\cdot 8\pi r^3=\frac43\pi r^3.\]

Investigate Archimedes' Proposition 4 below, which concerns a paraboloid (the solid obtained by rotating the curve \(x=y^2\) about the \(x\)-axis) inscribed inside a cylinder (with radius \(CD\) and height \(AD\)). 

a) Describe the balancing relationship that is illustrated in the sketch.

b) Use this relationship to determine a relationship between the volume of the paraboloid and the volume of the cylinder.

c) Find a formula for the volume of the paraboloid in terms of \(r\), the radius of the cylinder.

Click here to get the solutions for this exercise.

We can use Archimedes' method to determine the center of gravity of the paraboloid. By symmetry, we know that the center of gravity lies at some point \(X\) on its axis (not indicated on the applet). Archimedes' Proposition 5, illustrated below, shows a paraboloid and a cone inscribed inside a cylinder. 

The sketch illustrates that the paraboloid, left where it is, balances the cone, moved to \(H\), where \(|AH|=|AD|\). So, by the law of the lever,

\[(\mbox{Volume of paraboloid})\times |AX|=(\mbox{Volume of cone})\times |AH|.\]

Since the volume of the paraboloid is half the volume of the cylinder (by the previous exercise), and the volume of the cone is one-third the volume of the cylinder, this implies that \[\frac12|AX|=\frac13|AH|,\,\,\,\,{\rm{hence}}\,\,\,{\rm{that}}\,\,\,\, |AX|=\frac23|AH|.\] So the center of gravity of the paraboloid lies on its axis, twice as far from the vertex as from the base.

Archimedes' Proposition 6, illustrated below, concerns a cone inscribed in a hemisphere.

Exercise on Proposition 4:

a) Each cross-section of the cylinder, left where it is, balances the cross-section of the paraboloid, moved to H.

b) Thus the cylinder, left where it is, will balance the paraboloid moved so that its center of gravity is H. By the Law of the Lever we have

\[(\mbox{volume of paraboloid}) \times |AH|=(\mbox{volume of cylinder})\times\frac12|AD|\]

Since \(AD=AH\),

\[\mbox{volume of paraboloid} =\frac12(\mbox{volume of cylinder})\]

c) If \(r\) is the radius of the cylinder, then the height is \(h = r^2\) (since the paraboloid is generated by the curve \(x= y^2\)). So the volume of the cylinder is \(\pi r^2 h= \pi r^4\), and so the volume of the paraboloid is \(\frac12\pi r^4\).

Exercise on Proposition 6:

a) Each cross-section of the hemisphere together with the cross-section of the cone, left where they are, balance the cross-section of the cone moved to H.

b) Thus the hemisphere and cone, left where they are, balance a cone placed so that its center of gravity is at H. Let X be the location of the center of gravity of the hemisphere. By the Law of the Lever,

\[(\mbox{Volume of hemisphere})\times |AX|+(\mbox{Volume of cone})\times\frac34|AG|=(\mbox{Volume of cone})\times |AH|\]

Now we know that the volume of the hemisphere is \(\frac23\pi r^3\), the volume of the cone is \(\frac13\pi r^3\), and \(|AH|=2|AG|\).

Putting this information into the above equation and solving for \(|AX|\) we find that \(|AX|=\frac58|AG|\).

References

For more information, consult:

[1] T. L. Heath, The Works of Archimedes, Dover, New York, 1953.

[2] Sherman Stein, Archimedes: What Did He Do Besides Cry Eureka?, Mathematical Association of America, 1999.