The Great Art or the Rules of Algebra [1] by Gerolamo Cardano (1501-1576), published in 1545, is one of the great books in the history of mathematics. Most of it deals with cubic equations, whose algebraic solution had never appeared in print before Cardano wrote his masterpiece. Almost at the end of the book (chapter 39) we can find, also for the first time, a systematic approach to the solution of all quartics -- a method developed by Lodovico Ferrari (1522-1565), a disciple of Cardano.

Cardano was a remarkable physician and a first rate mathematician. Besides, as anyone who reads The Great Art (Ars Magna in Latin) will agree, he was an excellent writer. For instance, he wisely discusses quartics only after having analyzed biquadratic equations in chapters 1 and 24, and some special quartics in chapter 26. That is to say, Cardano starts with numerical examples of equations of the type $$x^{4}+ax^{2}+b=0$$ before dealing with numerical examples of the general case $$x^{4}+ax^{3}+bx^{2}+cx+d=0.$$ Indeed, one can understand Ferrari's method better after getting acquainted with the solution of biquadratics.

In Cardano's lifetime complex numbers were not considered bona fide numbers, neither were decimal approximations commonly used; thus, it is interesting to analyze parts of Ars Magna from a modern perspective. We have chosen all of Cardano's examples in chapter 39 related to quartics, dissected them in detail, and examined them through the lens of contemporary mathematics. We have not kept the original order, rather we have chosen to analyze problems I and X first (both lead to biquadratics); thereafter we discuss eight problems about quartics, starting from the ones that require less work.

We have benefited from T.R. Witmer's translation from Latin into English, and from Witmer's use of modern symbolism. Ars Magna was written using many idiosyncratic symbols, a notation somehow at the middle between the rhetorical algebra of Islamic mathematicians and modern day symbolic algebra.

At the very beginning of Ars Magna, five polynomial equations of the fourth order appear ([1], pp. 10-11), namely x⁴ = 81, x⁴ + 3x² = 28, x⁴ + 12 = 7x², x⁴ + 12 = 6x², and x⁴ = 2x² + 8. Cardano ascertains that 3 and -3 are solutions of x⁴ = 81, 2 and -2 are solutions of x⁴ + 3x² = 28, while 2, -2, √3, -√3 are solutions of x⁴ + 12 = 7x². Moreover, Cardano writes that x⁴ + 12 = 6x² has no solutions and x⁴ = 2x² + 8 has 2, -2 as solutions. It is to be noted that Cardano calls positive solutions "true" while negative solutions are called "fictitious". No mention is made of complex solutions of equations since complex numbers were not accepted during Cardano's time.

We can check that Cardano has found all the solutions in the real field. For instance, by long division we get x⁴ + 3x² - 28 = (x-2)(x+2)(x²+7), so 2 and -2 are the only real solutions of x⁴ + 3x² = 28 . Later, in chapter 24 of Ars Magna, we find the method used by Cardano. He starts with the biquadratic equation x⁴ + 2x² = 10 and claims that it is enough to find the solutions of x² + 2x = 10 and then take the square roots of them. The corresponding quadratic has only one positive solution, namely \( \sqrt{11} - 1 \); thus, \( \pm \sqrt{ \sqrt{11} - 1} \) are the only "real" solutions of the biquadratic. Although \( -\sqrt{11} - 1 \) is also a real solution of x² + 2x = 10, Cardano does not consider \( \pm i\sqrt{ \sqrt{11} + 1} \) as solutions of the given biquadratic since they are complex numbers.

From a modern perspective we can see that Cardano noticed that to solve x⁴ + 2x²=10 it is enough to define the transformation y = x² and then analyze the equation y² + 2y = 10. However, we run into difficulties when we try to solve x⁴ + 12 = 6x², one of the equations that appear at the beginning of Ars Magna, because the transformation y = x² leads to y² - 6y + 12 = 0, whose solutions are 3 ± i√3. Thus, we would have to solve x² = 3 ± i√3. This is a quadratic equation with complex coefficients; it is no wonder that Cardano claims that x⁴ + 12 = 6x² has no solutions - obviously he is thinking about solutions in the real field. In section 8 we will analyze this equation in detail.

Problem I, chapter 39 of Ars Magna, leads to a biquadratic: "Find three proportional quantities of which the square of the first is equal to the second and third, and the square of the third is equal to the squares of the second and first." Let the quantities be y, yx, yx², where x is the constant of proportionality. The problem demands that y² = yx + yx² and (yx²)² = y² + (yx)². The first equation leads to y = x + x² while the second equation leads to x⁴ = x² + 1. Defining z = x² we get z² = z + 1, whose solutions are $$z={1\over 2} \pm {\sqrt{5}\over 2}.$$ We are not interested in the negative solution since it will lead to the complex solutions $$x=\pm i\sqrt{{\sqrt{5}\over 2}-{1\over 2}}.$$ Rather we deal with x² = z = 1/2+√5/2, whose positive solution is $$x=\sqrt{{1\over 2}+{\sqrt{5}\over 2}}.$$ Then $$y=\sqrt{{1\over 2}+{\sqrt{5}\over 2}} + {1\over 2} +{\sqrt{5}\over 2},$$ and we can conclude that the three quantities are $$\sqrt{{1\over 2}+{\sqrt{5}\over 2}} + {1\over 2}+{\sqrt{5}\over 2},\quad \left(\sqrt{{1\over 2}+{\sqrt{5}\over 2}} + {1\over 2}+{\sqrt{5}\over 2}\right)\sqrt{{1\over 2}+{\sqrt{5}\over 2}}\quad {\rm and} \quad \left(\sqrt{{1\over 2}+{\sqrt{5}\over 2}} + {1\over 2}+{\sqrt{5}\over 2}\right)\left({1\over 2}+{\sqrt{5}\over 2}\right).$$

Of a similar nature is problem X, chapter 39 of Ars Magna: "Find three proportional numbers the sum of which is 8 and the square of the third of which is equal to the sum of the squares of the first and second". Let the numbers be y, yx, and yx², where x is the constant of proportionality. Then y²x⁴ = y² + y²x², which leads to x⁴ = x²+1. The transformation z = x² provides us with only one positive solution, namely $$x=\sqrt{1+\sqrt{5}\over 2}.$$ But y + yx + yx² = 8, so $$y={8\over \left(1+\sqrt{1+\sqrt{5}\over 2}+ {1+\sqrt{5}\over 2}\right)}.$$ The problem has come to an end because we have been able to find the values of y and x.

It is to be noted that Cardano reached the solution to problem X following a somewhat different procedure. He denotes the three proportional numbers 1, x, x². Since(x²)² = x² + 1 we will have x⁴ = x²+1, whose only positive solution is $$x=\sqrt{1+\sqrt{5}\over 2}.$$ But $$r =1+\sqrt{1+\sqrt{5}\over 2}+ {1+\sqrt{5}\over 2}$$ would have to be 8, which it is not. To remedy this situation Cardano uses the rule of three and concludes that $$y={8\over \left(1+\sqrt{1+\sqrt{5}\over 2}+ {1+\sqrt{5}\over 2}\right)}$$ is the first quantity, \( y\sqrt{\frac{1+\sqrt{5}}{2}} \) is the second quantity, and \( y\left(\frac{1+\sqrt{5}}{2}\right) \) is the third quantity.

Chapter 26 of Ars Magna starts with a rule: "If the fourth and first powers are equal to the square and constant and if, having divided the coefficient of x and the constant by the coefficient of x², half the result of dividing the coefficient of x is [equal to] the square root of that which came out of the division of the constant of the equation, then take the square root of the constant of the original equation, add to it one-fourth the coefficient of x², take the square root of the sum, from this subtract the square root of the same one fourth the coefficient of x², and the remainder is the value of x." Translated into modern symbols by T.R. Witmer ([1], p. 171), the rule establishes that if x⁴ + ax = bx² + N, and if a/2b = √(N/b), then $$x=\sqrt{\sqrt{N}+{b\over 4}} - \sqrt{b\over 4}.$$ We must stress that when an equation to be solved appears in the statement of a problem in chapter 26 of Ars Magna, without exception the coefficients are positive.

Cardano does not present a proof, so we might as well provide one. Since N = a²/4b, we will have $$x^4=bx^2-ax+N=bx^2-ax + {a^2\over 4b} = b\left(x^2-{a\over b}x+{a^2\over 4b^2}\right) = b\left(x-{a\over 2b}\right)^2,$$ hence $$x^2=\pm\sqrt{b}\left(x-{a\over 2b}\right).$$ The solutions of these two quadratic equations are $$x=\sqrt{b\over 4} \pm \sqrt{{b\over 4}-\sqrt{N}} \qquad {\rm and} \qquad x = -\sqrt{b\over 4}\pm \sqrt{{b\over 4} +\sqrt{N}}.$$ Cardano's solution, namely $$x = -\sqrt{b\over 4} + \sqrt{{b\over 4}+\sqrt{N}},$$ is one of them (obviously it is positive). The solutions of the first quadratic might be complex if b/4 - √N < 0.

It should come as no surprise that algebraic proofs, as we understand them nowadays, are absent in Ars Magna; in particular in chapter 26. Modern symbolic algebra came into being in the 17th century after the pioneering works of François Viète (1540-1603).

The first problem of chapter 26 has to do with money deposits, which leads to the equation x⁴ + 10x = 5x² + 5. We observe that 5 = 10²/(4 x 5), thus the equation is of the type just discussed. Rather than applying the rule, as Cardano does, it might be advisable to follow the procedure that led to a proof: x⁴ = -10x + 5x² + 5 = 5(x² - 2x + 1) = 5(x - 1)², whence x² = ±√5(x - 1). The equation x² = -√5(x - 1) has the solutions $$x= -\sqrt{5\over 4} \pm \sqrt{{5\over 4}+\sqrt{5}},$$ while the equation x² = √5(x - 1) has the complex solutions $$x=\sqrt{5\over 4} \pm i\sqrt{\sqrt{5}-{5\over 4}}.$$ As expected, Cardano considers only the positive solution $$x= -\sqrt{5\over 4} + \sqrt{{5\over 4}+\sqrt{5}}.$$

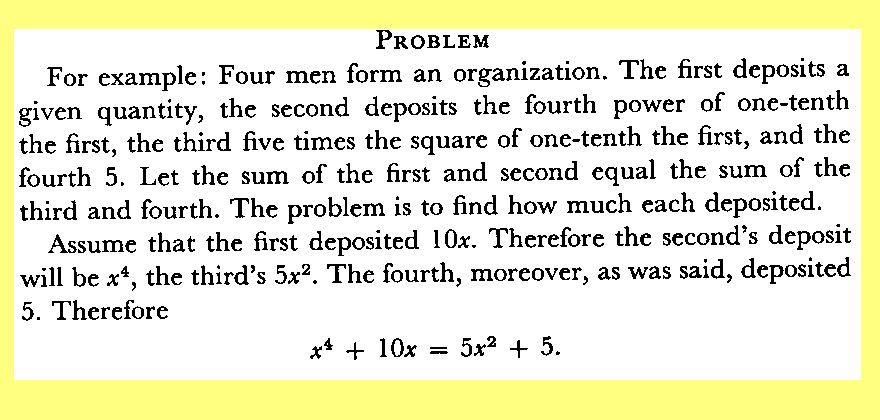
Figure 2: First problem from chapter 26

The second rule of chapter 26 is very similar to the first. In modern symbols it establishes that if x⁴ = bx² + ax + N and a/2b = √(N/b) then $$x=\sqrt{{b\over 4}+\sqrt{N}} + \sqrt{b\over 4}.$$ It can be proven, in an almost identical way as the first rule. Indeed, the equation has the solutions $$x=\sqrt{b\over 4} \pm \sqrt{{b\over 4}+\sqrt{N}}, \quad {\rm and} \quad x=-\sqrt{b\over 4} \pm \sqrt{{b\over 4}-\sqrt{N}}$$ and the latter will be complex if b/4 - √N < 0. For instance, x⁴ = 5x² + 10x + 5 ([1], p. 172) has the solutions $$x = \sqrt{5\over 4} \pm \sqrt{{5\over 4}+\sqrt{5}} \quad {\rm and} \quad x = -\sqrt{5\over 4} \pm i\sqrt{\sqrt{5}-{5\over 4}}.$$ Cardano writes only the positive solution $$x = \sqrt{5\over 4} + \sqrt{{5\over 4}+\sqrt{5}}.$$

The third rule, in Witmer's translation into modern symbols ([1], p.173), reads as follows: "If x⁴ + bx² + ax = cx³ + N, and if N = b+2, and if a = c, and if a/2 = √N, then $$x = {a\over 4} \pm \sqrt{\left({a\over 4}\right)^2+1-\sqrt{\left({a\over 2}\right)^2+1}}."$$ That is to say, we have to solve the equation x⁴+(a²/4 - 2)x² + ax = ax³ + a²/4. This problem looks more challenging since there is a cubic term in the equation. Can we provide a proof?

We start by observing that the given equation is equivalent to x⁴ - ax³+(a²/4)x² = 2x² - ax + a²/4. Thus x²(x² - ax + a²/4) = 2x(x - a/2) + a²/4, which in turn leads to x²(x - a/2)² - 2x(x - a/2) + 1 = a²/4 + 1. Therefore, $$\left(x\left(x-{a\over 2}\right) - 1\right)^2 = \left(\sqrt{{a^2\over 4}+1}\right)^2 \quad {\rm or} \quad x\left(x-{a\over 2}\right)-1 = \pm\sqrt{{a^2\over 4}+1}.$$ The equation $$x^2-{a\over 2}x - \left(1+\sqrt{1+{a^2\over 4}}\right) = 0$$ has the two solutions $$x = {a\over 4} \pm \sqrt{\left({a\over 4}\right)^2+1+\sqrt{\left({a\over 2}\right)^2+1}},$$ while the equation $$x^2-{a\over 2}x - \left(1-\sqrt{1+{a^2\over 4}}\right) = 0$$ has the two solutions $$x = {a\over 4} \pm \sqrt{\left({a\over 4}\right)^2+1-\sqrt{\left({a\over 2}\right)^2+1}},$$ Only the latter appear in the third rule.

As an example, Cardano puts forward the equation x⁴ + 34x² + 12x = 12x³ + 36. We will then have the solutions 3 ± √(10-√37) (both positive) and the solutions 3 ± √(10+√37) (one positive and the other negative). Cardano does not consider the solutions 3 ± √(10+√37), although one of them is positive.

Let us try to solve the biquadratic x⁴ + x² + 1 = 0, an equation that cannot be analyzed with ease if we were to use the transformation y = x² because we would have to calculate the square root of a complex number. Instead, "completing squares" we get (x²+ 1)² = x²; thus, x² + 1 = x or x² + 1 = -x. The first quadratic has the solutions 1/2 ± i√3/2 while the second has the solutions -1/2 ± i√3/2; these four numbers are precisely the solutions of the given biquadratic. The crux of the matter was to reach the equation (x² + 1)² = x², wherein two perfect squares appear at each side of the equality sign. Precisely this idea will help us to better understand what comes next.

Lodovico Ferrari invented a systematic procedure to solve all quartics and he is duly recognized in Ars Magna for this important achievement ([1] p. 237). As we will see, the procedure leads to the solution of a cubic, called the "Resolvent Cubic". A solution of the latter can be found by inspection - if we are lucky - or we have to use the Cardano-Tartaglia technique for solving cubics (Niccolo Tartaglia (1500-1557) gave Cardano, in 1539, the method to solve y³+ py = q, where p,q > 0, and two other particular cases of the cubic). To illustrate Ferrari's method let us discuss in detail two problems from chapter 39 of Ars Magna.

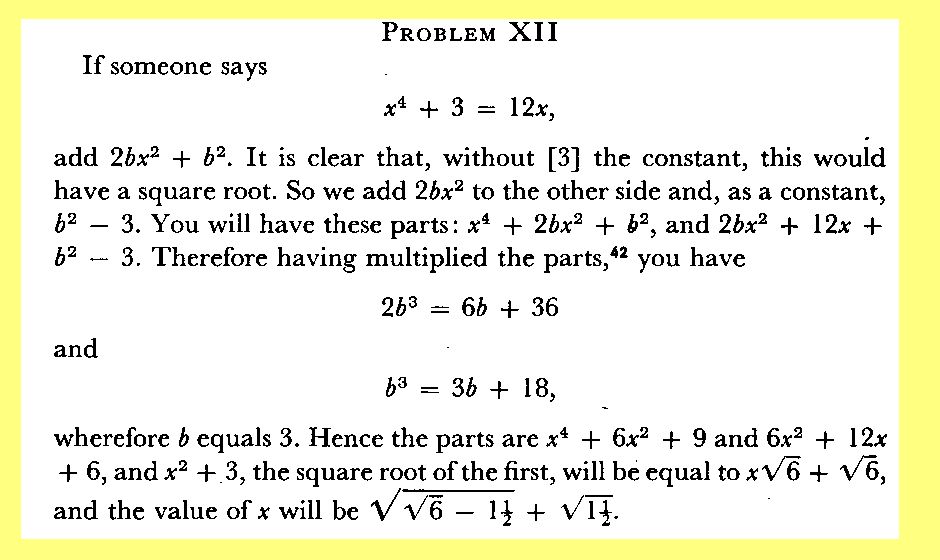
Figure 3: Problem XII from chapter 39

Let us start with problem XII: Solve the equation x⁴ + 3 = 12x, which is equivalent to (x²)² = 12x - 3. For every b, this implies (x² + b)² = 2bx² + 12x + (b² - 3). Now, in general, a quadratic polynomial is the square of another polynomial if the discriminant is equal to 0. Thus, we must find b such that the discriminant 12² - 4(2b)(b² - 3) of the polynomial 2bx² + 12x + b² - 3 equals 0. Expanding, we see that this is the same as asking that b³ = 3b + 18. By inspection we find that b = 3 is a solution. Using this value of b leads us to (x² + 3)² = 2*3x² + 12x + (3² - 3), i.e. (x² + 3)² = 6(x² + 2x + 1), which is equivalent to (x² + 3)² = (√6(x + 1))². Therefore x²+ 3 = √6(x + 1) or x² + 3 = -√6(x + 1). The first quadratic equation provides the positive real solutions √6/2 ± (1/2)√(-6 + 4√6) and the second quadratic equation provides the conjugate pair -√6/2 ± (i/2)√(6-4√6). These are precisely the solutions of the original quartic, although Cardano considers only √6/2 + (1/2)√(-6 + 4√6).

It should be noted that Cardano does not use the term "discriminant"; given any quadratic polynomial he realizes that it will have a double root provided that "the square of half the middle quantity, x, equals the product of the extremes" ([1], p. 244). In modern symbolism we would say that any quadratic polynomial px² + qx + r will have a double root if (q/2)² = pr, which is equivalent to demand that q² - 4pr = 0; thus, the discriminant of the quadratic polynomial has to be zero.

If we apply the preceding method to x⁴ + 4x + 8 = 10x² (problem IX) we would get, for any z, the equality (x² + z)² = 10x² - 4x - 8 + 2zx² + z², that is to say (x² + z)² = (10 + 2z)x² - 4x + (z² - 8). We wish to find a real value of z that will make the expression to the right of the equal sign a perfect square. This can be accomplished by solving the equation 0 = 16 - 4(2z + 10)(z² - 8), which is equivalent to z³ + 5z² - 8z - 42 = 0. By simple inspection we find a solution z = -3, therefore (x² - 3)² = 4x² - 4x + 1; that is to say (x² - 3)² = (2x - 1)². Hence x² - 3 = 2x - 1 or (x² - 3) = -(2x - 1). The solutions of these quadratics are 1 ± √3 and -1 ± √5. These are, then, the four solutions of the given quartic.

In problem IX Cardano takes a slightly different path: the given equation is equivalent to x⁴ - 2x² + 1 = 8x² - 4x - 7. Adding -2bx² + b² + 2b to both sides of the equality we get x⁴ - (2 + 2b)x² + (b + 1)² = 8x² - 2bx² - 4x + b² + 2b -7, hence (x² - (b + 1))² = (8 - 2b)x² - 4x + (b² + 2b -7). The right hand side will be a perfect square if 4 = (8 - 2b)(b² + 2b - 7), thus b³ + 30 = 2b² + 15b. It is not hard to see that b = 2 is a solution to the cubic, therefore (x² - 3)² = 4x² - 4x + 1 or, what is the same, (x² - 3)² = (2x - 1)². Finally we get x² - 3 = 2x - 1 or x² - 3 = -(2x - 1), the same quadratic equations we found in the previous paragraph. Why did Cardano choose a more elaborate path? Probably he preferred to reach a resolvent cubic with a positive solution b = 2 instead of the negative solution z = -3 obtained when we followed the first path. Let us recall that Cardano does accept negative solutions to equations, but he calls them "fictitious".

At the end of Problem IX Cardano asks whether in choosing another solution of the resolvent cubic we would reach the same solutions of the original quartic equation. He leaves this task to the reader asserting: "If this operation delights you, you may go ahead and inquire into this for yourself." Following Cardano's advice, let us first find another solution of the resolvent cubic z³ + 5z² - 8z - 42 = 0. Since z = -3 is a solution we can apply the method of long division and reach the equivalent equation (z + 3)(z² + 2z - 14) = 0. The solutions of z² + 2z - 14 = 0 are -1 ± √15, thus we have the three solutions of the cubic under discussion.

Let us choose z = √15 - 1. Replacing this value of z in (x² + z)² = (10 + 2z)x² - 4x + (z² - 8), an equation that we introduced at the beginning of the discussion of Problem IX, we obtain (x² + √15-1)² = (8 + 2√15)x² - 4x + 8 - 2√15. However, any quadratic polynomial px² + qx + r, with a double root, can be expressed as p(x + q/2p)². This is an elementary result of symbolic algebra that we will apply often in order to simplify Cardano's approach to quartics. Thus $$(8+2\sqrt{15})x^{2}-4x+8-2\sqrt{15}=(8+2\sqrt{15})\left(x-\frac{4}{2(8+2\sqrt{15})}\right)^{2},$$ consequently $$(x^{2}+\sqrt{15}-1)^{2}=\left(\sqrt{8+2\sqrt{15}}\left(x-\frac{1}{4+\sqrt{15}}\right)\right)^{2}.$$

We can then conclude that $$x^{2}+\sqrt{15}-1=\sqrt{8+2\sqrt{15}}\left(x-\frac{1}{4+\sqrt{15}}\right) \quad {\rm or} \quad
x^{2}+\sqrt{15}-1=-\sqrt{8+2\sqrt{15}}\left(x-\frac{1}{4+\sqrt{15}}\right).$$ The first quadratic equation has solutions √3 + 1 and √5 - 1, while the second quadratic equation has solutions – √3 + 1 and – √5 - 1. These are the four solutions of x⁴ + 4x + 8 = 10x² found before, thus giving an affirmative answer to Cardano's question. Note that Cardano has wisely chosen problems XII and IX in such a way that the corresponding cubic has an integer solution.

The first paragraph of Problem V states that "For example, divide 10 into three proportional parts, the product of the first and second of which is 6. This was proposed by Zuanne de Tonini da Coi, who said it would not be solved. I said it could, though I did not yet know the method [for doing so]. This was discovered by Ferrari." Cardano claims that the three numbers are 6/x, x, and x³/6. This makes sense because (6/x)x = 6, while the constant of proportionality is x/(6/x), that is to say x²/6. The third term will be x(x²/6). Since the sum of the three terms has to be 10, it follows that 6/x + x + x³/6 = 10, which in turn leads to x⁴ + 6x² + 36 = 60x.

Problem V is the first instance in chapter 39 of Ars Magna where Cardano has to solve a quartic. Before doing so, he presents an elaborate geometrical proof that justifies the algebraic identity (s + a + b)²=(s + a)² + 2bs + 2ab + b² ([1], p. 238; [8], p. 57). Making s = x² and a = 6, we get (x² + 6 + b)² = (x² + 6)² + 2bx² + 12b + b². But problem V is equivalent to x⁴ + 12x² + 36= 6x² + 60x, thus (x² + 6)² = 6x² + 60x; therefore (x² + 6 + b)² = (2b + 6)x² + 60x + (b² + 12b). The right side will be a perfect square provided that the discriminant is zero, consequently 0 = 3600- 4(2b + 6)(b² + 12b), i.e. b³ + 15b² + 36b = 450. The usual method for solving cubics leads to a solution $$b_1= oot 3of {190+sqrt{33,903}}+ oot 3of {190-sqrt{33,903}} - 5;$$ thus, (x² + 6 + b₁)² = (2b₁ + 6)(x + 60/(2(2b₁+6)))². Finally $$(x^2+b_1+6)^2 = left(sqrt{2b_1+6}left(x + {30over 2b_1+6} ight) ight)^2,$$ which in turn leads to $$x^2+b_1+6 = pm left(xsqrt{2b_1+6} = {30oversqrt{2b_1+6}} ight).$$ The problem has been reduced to the task of solving two quadratic equations. We could write the equations in full once we note that $$b_1+6 = oot 3of{190+sqrt{33,903}} + oot 3of{190-sqrt{33,903}} + 1 quad { m and} quad 2b_1+6 = oot 3of {1,520 + sqrt{2,169,792}} + oot 3of {1,520 - sqrt{2,169,792}} - 4.$$

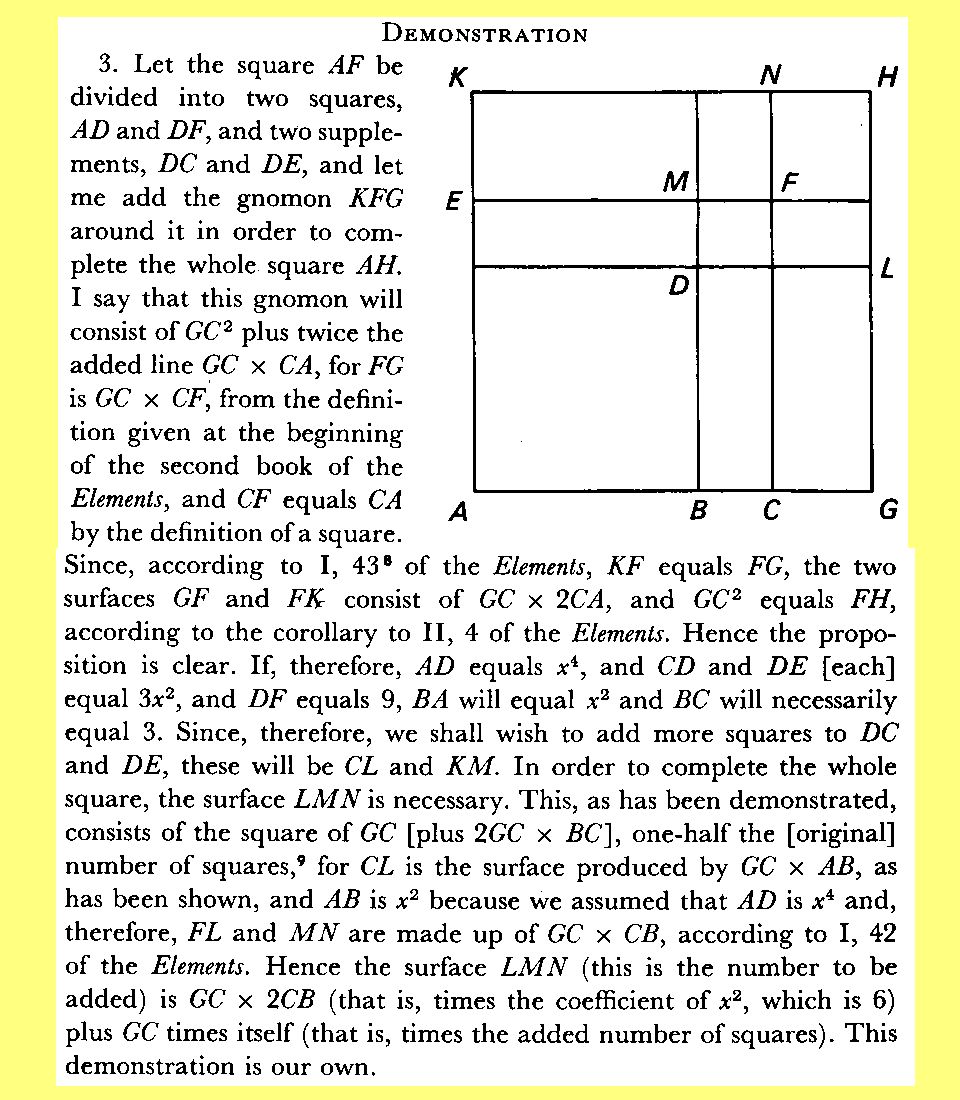
Figure 4: Cardano's geometrical proof related to problem V of chapter 39

Although as early as the 12th century Islamic mathematicians used decimals for the purpose of approximation ([7], p. 270), decimal approximations of irrational numbers were not used in Europe when Ars Magna was published for the first time. No wonder that decimals are nowhere to be found in Cardano's masterpiece; througout chapter 39 exact solutions are sought.

Decimals are very handy when discussing Problem V: Using the decimal approximation 4.00979 to b₁ we would have to solve, for instance, the quadratic equation $$x^2+4.00979 +6 = xsqrt{2cdot 4.00979+6} + {30over sqrt{2cdot 4.00979+6}}.$$ The solutions of it are, approximately, 3.09987 and 0.644398.

An alternative approach to the solution of x⁴ + 6x² + 36 = 60x is to introduce a new variable z and note that (x² + z)² = (2z - 6)x² + 60x + z² - 36. In order to have a perfect square we need to solve the equation 60² - 4(2z - 6)(z² - 36) = 0, i.e. z³ - 3z² - 36z - 342 = 0. The transformation y = z - 1 leads to y³ = 39y + 380, whose solution is $$y = oot 3of{190+3sqrt{3,767}} + oot 3of{190-3sqrt{3,767}}.$$ Therefore z » 10.0098. The problem is then reduced to the solution of the equation $$ (x^2+10.0098)^2 = (2cdot 10.098-6)left(x+{60over 2(2cdot 10.098-6)} ight)^2.$$ Thus, $$x^2+10.098 = sqrt{28.0392}left(x+{60over 28.0392} ight) quad { m or} quad x^2+10.0098= -sqrt{28.0392}left(x+{60over 28.0392} ight).$$ The first quadratic has the real solutions 3.09988 and 0.644399 while the second provides two complex solutions. The whole problem looks quite simple, but let us keep in mind that we are using decimal approximations! They have helped us to focus on the method by allowing us to avoid complicated expressions.

Problem VI asks to "find a number which is equal to its square root plus twice its cube root." Denoting the positive number by x⁶, we have to solve the equation x⁶= x³ + 2x². Dividing by x² we get the equivalent equation x⁴= x + 2, a quartic indeed. Cardano subtracts 1 from each side and reaches x⁴ - 1= x + 1, thus (x⁴ - 1)/(x + 1)= 1. Consequently x³ - x²+ x - 1= 1, that is to say x³ + x = x² + 2. He ascertains that $$x=\root 3\of{\sqrt{83\over 108}+{47\over 54}}-\root 3\of{\sqrt{83\over 108}-{47\over 54}}+{1\over 3}$$is a solution, without providing the details. Let us check whether this is a solution, using for that purpose the Cardano-Tartaglia method.

Defining y = x-1/3 we reach the depressed cubic y³ = -(2/3)y+ 47/27. Keeping in mind that (u - v)³ = -3uv(u - v) + (u³ - v³), a solution of the form y = u - v will be found provided that the algebraic system -3uv= -2/3, u³ - v³= 47/27 has a solution. Indeed u³- (2/9u)³= 47/27, that is to say u⁶ - (47/27)u³ - 8/729 = 0. One solution of this equation is $$u^3 = {47\over 54} + {1\over 2}\sqrt{\left({47\over 27}\right)^2 + {32\over 729}},$$ thus $$u = \root 3\of {{47\over 54}+\sqrt{83\over 108}}.$$ Replacing this value of u in u³ - v³= 47/27 we get $$v=\root 3\of{-{47\over 54}+\sqrt{83\over 108}}.$$ Then $$y=u-v=\root 3\of{{47\over 54}+\sqrt{83\over 108}} - \root 3\of{-{47\over 54}+\sqrt{83\over 108}},$$ which in turn leads to $$x=\root 3\of{\sqrt{83\over 108} + {47\over 54}}-\root 3\of{\sqrt{83\over 108}-{47\over 54}}+{1\over 3}.$$The other two solutions of the cubic are complex numbers, no wonder that Cardano ignores them.

A natural question, addressed in Ars Magna, is what happens if we follow Ferrari's procedure: We start with (x²)² = x + 2 and introduce a new variable b. We observe that (x² + b)² = 2bx² + x + b² + 2. In order to have a perfect square we need to impose the condition 1/4 = 2b(b² + 2), equivalent to demanding that the discriminant of 2bx² + x + b² + 2 is zero. Thus b³ + 2b= 1/8. Cardano ascertains that $$b=\root 3\of{{1\over 16}+\sqrt{2,075\over 6,912}}-\root 3\of{-{1\over 16}+\sqrt{2,075\over 6,912}}$$is a solution. We would have to replace this value of b, call it b₁, in (x² + b)²= 2bx² + x + b² + 2 knowing beforehand that the expression to the right is also a perfect square. In other words, it is necessary to solve (x² + b₁)² = 2b₁(x+ 1/4b₁)² or, equivalently, the pair of quadratic equations $$x^2+b_1=\sqrt{2b_1}\left(x+{1\over 4b_1}\right), \qquad x^2+b_1=-\sqrt{2b_1}\left(x+{1\over 4b_1}\right).$$The solutions of the first quadratic are $$x=\sqrt{b_1\over 2} \pm\sqrt{{1\over 2\sqrt{2b_1}}-{b_1\over 2}}.$$

Using the decimal approximation 0.062379 to b₁ we get x = 1.35321 and x = -0.999998. The first is a decimal approximation to the solution found before, while the other root is a decimal approximation to -1. But we are not interested in the latter because we are seeking only positive solutions to the problem (from the very beginning we ruled out the trivial solutions of the equation x⁶ = x³ + 2x², namely 0 and -1). It should be noted that the quadratic equation \( x^2 +b_1 = -\sqrt{2b_1} \left( x+\frac{1}{4b_1} \right) \) has complex roots.

Problem VIII asks to "divide 6 into three proportional quantities the sum of the squares of the first and second of which is 4." Let x, y, z denote the quantities. Since x² + y²= 4 we will have \( y = \sqrt{4 - x^2} \). But x + y + z = 6, consequently \( z = 6 - x - \sqrt{4 - x^2} \). Next Cardano ascertains that \( \left(6 - x - \sqrt{4 - x^2}\right) x = {4 - x^2} \), which is correct since x, y, z are proportional quantities. Then \( 6x - x^2 - x\sqrt{4 - x^2} = {4 - x^2} \), that is to say \( 6x - 4 = x\sqrt{4 - x^2} \). Squaring both sides we get x⁴ + 32x² + 16 = 48x, which is equivalent to (x² + 16)² = 48x + 240.

For any b we have (x² + 16 + b)² = 2bx² + 48x + b² + 32b + 240. We need to have the discriminant equal to zero in order to make sure that the right hand side is a perfect square, thus 0= 48² - 8b(b² + 32b + 240). This expression is equivalent to b³ + 32b² + 240b = 288. Then Cardano uses the transformation c= b + 32/3 and reaches the equation c³ = (101 1/3)c + 420 20/27, with a real solution c. Therefore b = c - 32/3 is a real solution of the resolvent cubic. Actually, Cardano does not introduce a new variable explicitly. Finally we have (x² + 16+ b)² = 2b(x + 48/4b)², which in turn leads to (x² + 16+ b)= ±√(2b)(x+ 12/b). Cardano only considers the equation with positive sign and writes it as x² + 16 + b = √(2b)x+ √(b² + 32b + 240), which is in agreement with x² + 16 + b = √(2b)x+ √(2b)(12/b) because √(2b)(12/b) = √(288/b)= √[(b³ + 32b² + 240b)/b] = √(b² + 32b + 240). He leaves the task of finding b, and solving the above-mentioned quadratic equation, to the readers of Ars Magna.

An alternative path is to start with x⁴ = -32x² + 48x - 16 and introduce a new variable z so that the expression on the right of (x²+ z)² = (2z - 32)x² + 48x + z² - 16 is a perfect square. This can be accomplished by solving 0 = 48² - 4(2z - 32)(z² -16), i.e. z³ - 16z² - 16z - 32= 0. The usual approach to cubics leads to \( z \approx 17.0486 \), therefore $$(x^2+17.0486)^2 = 2.0972x^2+48x+274.655 = 2.0972\left(x+{48\over 2\cdot 2.0972}\right)^2, \quad {\rm or}\quad (x^2+17.0486)^2 = \left(\sqrt{2.0972}\left(x+{48\over 4.1944}\right)\right)^2.$$ Hence $$x^2+17.0486 = \sqrt{2.0972}\left(x+{48\over 4.1944}\right) \quad {\rm or} \quad x^2+ 17.0486 = -\sqrt{2.0972}\left(x+{48\over 4.1944}\right).$$ The first quadratic has the real solutions 0.943916 and 0.504855 while the second quadratic has complex solutions. Obviously, both real solutions are approximations.

The five problems about quartics that we have discussed so far (problems XII, IX, V, VI, and VIII) have no cubic terms; thus, Ferrari's method can be applied readily. In problem VII, namely x⁴ + 6x³ = 64, Cardano realizes that a transformation is needed to eliminate the cubic term. Indeed, the seventh rule of chapter 7 suggests defining the transformation y = 4/x, which leads to y⁴ = 6y + 4. This is a quartic without cubic term, hence we can apply Ferrari's method to it; for each solution y = b we will get a root x = 4/b of the original equation. In problem VII Cardano does not look for the solutions, rather he is mainly interested in stressing the need to apply a transformation to eliminate the cubic term.

We may add that by inspection it is possible to conclude that x = 2 is a root of x⁴ + 6x³ = 64, then by long division we get the equivalent equation (x - 2)(x³ + 8x² + 16x + 32) = 0. The problem has been reduced to the solution of a cubic.

Figure 5: Problem XI from chapter 39

Cardano solves problem XI, namely x⁴ - 3x³ = 64, in a rather novel way. Essentially, he does the following: A new variable b is introduced and the expression 2bx² + (b²/64)x⁴ is added to both sides of the aforementioned equation, leading to (b²/64 + 1)x⁴ - 3x³ + 2bx² = 64 + 2bx² + (b²/64)x⁴. Consequently $$x^2left[left({b^2over 64} + 1 ight)x^2-3x+2b ight] = left(8+{bover 8}x^2 ight)^2 (1)$$

The expression in brackets will be a perfect square provided (b²/64+1)(2b) = (-3/2)², i.e. b³ + 64b = 72. The real (positive) solution of this cubic is $$b= oot 3 of{sqrt{11,005{1over 27}}+36} - oot 3of{sqrt{11,005{1over 27}}-36}.$$ Therefore $$left({b^2over 64} + 1 ight)x^2-3x+2b = left({b^2over 64}+1 ight)left(x - {3over 2(b^2/64 + 1)} ight)^2 = left(xsqrt{{b^2over 64}+1} - {3over 2sqrt{b^2/64 + 1}} ight)^2.$$ But 3/2=√(b²/64+1)√(2b), thus $$left({b^2over 64}+1 ight)x^2-3x+2b = left(xsqrt{{b^2over 64}+1}-sqrt{2b} ight)^2.$$ Replacing this expression in (1) we obtain $$left(x^2sqrt{{b^2over 64}+1} - xsqrt{2b} ight)^2 = left(8+{bover 8}x^2 ight)^2.$$ Therefore $$x^2sqrt{{b^2over 64}+1}-xsqrt{2b} = 8+{bover 8}x^2.$$ Cardano is not interested in going any further because his stated purpose is to show a methodology that does not require a transformation.

Another possible quadratic is $$x^2sqrt{{b^2over 64}+1} - xsqrt{2b} = -left(8+{bover 8}x^2 ight),$$ which does not appear in Ars Magna. Of course, we can provide the solutions of both quadratics using the decimal approximation 1.10398 for b; the first quadratic has the solutions 4 and -2.29495 while the second has complex solutions. It should come as no surprise that 4 is a solution because by simple inspection we could have noticed, right at the beginning, that x = 4 is indeed a solution of x⁴ - 3x³ = 64. Then long division leads to (x - 4)(x³ + x² + 4x + 16) = x⁴ - 3x³ - 64 and we can ascertain that the only real solution of x³ + x² + 4x + 16 = 0 is approximately -2.29495.

Cardano does mention that Problem XI can be solved through the same transformation used in Problem VII, though he does not pursue this path. Indeed, defining y = 4/x we get the quartic equation y⁴ = -3y + 4 and we realize that for any z we have (y² + z)² = 2zy² - 3y + (z² + 4). The expression to the right of the equal sign will be a perfect square if 0 = 9 - 8z(z² + 4), that is to say z³ = - 4z + 9/8. The usual procedure for cubics leads to the real solution $$z= oot 3of{{9over 16}+{1over 2}sqrt{{81over 64}+{256over 27}}}- oot 3of {-{9over 16}+{1over 2}sqrt{{81over 64}+{256over 27}}} approx 0.275994.$$ Then $$(y^2+0.275994)^2 = 2cdot 0.275994y^2-3y + (0.275994^2+4) = 0.551988left(y-{3over 2cdot 0.551988} ight)^2.$$ Therefore $$y^2+0.2759994 = pmsqrt{0.551988}left(y-{3over 1.10398} ight).$$ The solutions of the first quadratic are complex, but the solutions of the second quadratic are approximately 0.999996 and -1.74295. Then x = 4/0.999996 = 4.00002 and x = 4/(-1.74295) = -2.29496. The first solution is, as expected, practically 4.

The last problem of chapter 39 is Problem XIII; after that there are no more references to quartics in Ars Magna. Problem XIII states: " Find a number the fourth power of which plus twice its cube is one more than the number." Thus, it is necessary to solve the equation x⁴ + 2x³ = x + 1. Evidently, a transformation of the type y = c/x will not eliminate the cubic term. Cardano chooses an ad-hoc method, which can be interpreted as follows: the given equation is equivalent to x⁴ + 2x³ + x² = x² + x + 1, thus (x² + x)² = 1 + x + x². Letting y = x² + x we get y² = y + 1. One solution of this equation is y=1/2+√5/2. In turn, the solutions of x² + x = 1/2 + √5/2 are -1/2 ± √(3/4 + √5/2) (only the positive solution is mentioned in Ars Magna). It is worth mentioning that the other solution of y² = y + 1, namely y=1/2 - √5/2, leads to the two complex solutions -1/2 ± i√(√5/2 - 3/4) of the original problem.

As an alternative path, not pursued by Cardano, we can use the transformation z = x + 2/4, i.e. x = z - 1/2, which eliminates the cubic term of the equation x⁴ + 2x³ = x + 1 and leads to the biquadratic equation z⁴ - (3/2)z² - 11/16 = 0, whose four solutions are $$pmsqrt{{3over 4}+{sqrt{5}over 2}}, quad pm isqrt{{sqrt{5}over 2}-{3over 4}}.$$ Then the four solutions of the original equation are $$-{1over 2}pm sqrt{{3over 4}+{sqrt{5}over 2}}, quad -{1over 2}pm isqrt{{sqrt{5}over 2}-{3over 4}}.$$

Having exhausted the contributions of Cardano and Ferrari to the problem of quartics in chapter 39, in the next section we will analyze an approach to biquadratics that was developed almost 200 years after the publication of the first edition of Ars Magna at a time when symbolic algebra had already reached its present form.

Can we find the solutions of x⁴ + x² - 1 = 0? Defining y = x², we get y² + y - 1 = 0, whose solutions are -1/2 ± √ 5/2. Then the four solutions of the original equation stem from x² = -1/2 ± √ 5/2; they are $$\pm\sqrt{-1+\sqrt{5}\over 2}, \quad \pm i\sqrt{1+\sqrt{5}\over 2}.$$ Problems of this sort were discussed in section 2. It is clear that, given the equation x⁴ + ax² + b = 0, with real coefficients, the transformation y = x² works well whenever a² - 4b > 0. We use the phrase "works well" to mean that we do not have to worry about finding the square root of a complex number. From a slightly different perspective we might observe that x⁴ + ax² = -b implies (x²+a/2)² = a²/4 - b; thus, (x² + a/2)² = (√(a²-4b)/2)², an equality that allows us to conclude that x² + a/2=(1/2)√(a² - 4b) or x² + a/2 = -(1/2)√(a²-4b). From these quadratic equations the four solutions (real or complex) do follow.

At the beginning of section 4 we analyzed the equation x⁴ + x² + 1 = 0 after writing x⁴ + 1 = -x² and "completing squares" in the sense that (x² + 1)² = -x² + 2x². Is it possible to extend this idea to all biquadratics whenever the transformation cannot be applied with ease? In his quest of a proof of the Fundamental Theorem of Algebra, Leonhard Euler (1707-1783) considered the equation x⁴ + ax² + b = 0, where a² - 4b < 0 ([5], p. 364). The inequality a² < 4b implies a <= |a| < 2√b, hence 2√b - a > 0. We have x⁴ + b = -ax², which is equivalent to (x² + √b)² = -ax² + 2√bx². Therefore (x² + √b)² = (x√(2√b-a)², which in turn leads to x² + √b = x√(2√b-a) or x² + √b = -x√(2√b-a). The two quadratics provide the four solutions, real or complex, of x⁴ + ax² + b = 0. We note that the expression a² - 4b, called the "discriminant" of the biquadratic, determines the path to be followed. We have succeeded in solving the most general biquadratic with real coefficients.

To illustrate the procedure let us analyze with care the equation x⁴ + 12 = 6x², one of Cardano's original equations that we mentioned in section 2. We note that its discriminant is negative, thus we write (x²+√12)² = 6x² + 2(√12)x², that is to say (x² + √12)² = (√(6 + 2√12)x)². Hence x² + √12 = √(6 + 2√12)x or x² + √12 = -√(6+2√12)x. The first equation has the two complex solutions $${1\over 2}\sqrt{6+2\sqrt{12}}\pm i{1\over 2}\sqrt{2\sqrt{12}-6}$$ while the second equation has the two complex solutions $$-{1\over 2}\sqrt{6+2\sqrt{12}} \pm i{1\over 2}\sqrt{2\sqrt{12}-6}.$$ These are the four solutions of the given biquadratic in the complex field. Cardano was right in claiming that x⁴ + 12 = 6x² has no solutions in the "real" realm, the only type acceptable to him and his contemporaries.

After having discussed several examples of quartics, and the most general biquadratic, it is time to look at Ferrari's method from a modern perspective. Let us consider the equation x⁴ + d₁x³ + d₂x² + d₃x + d₄ = 0, where the coefficients are real numbers. Using the transformation x=y - d₁/4 we can convert it into an equation of the type y⁴ + ay² + by + c = 0. We may assume that b ¹ 0 because b = 0 leads to a biquadratic, whose method of solution was analyzed in the previous section.

Next we will apply Ferrari's technique. Indeed y⁴ = -ay² - by - c. So (y² + z)² = 2y²z + z² - ay² - by - c for any z. Our goal is to find a real number z such that the right hand side of the last equality becomes a perfect square. Rearranging terms we get $$(y^2+z)^2 = (2z-a)y^2-by+(z^2-c)\ \ \ \ (2).$$

The expression (2z - a)y² - by + (z² - c) will be a perfect square provided its discriminant is zero, i.e. b² - 4(2z - a)(z² - c) = 0 or, what is the same, 8z³ - 4az² - 8cz + (4ac - b²) = 0. This is a cubic equation, customarily called a "resolvent cubic". Let z₁ be a real solution of it. Evidently 2z₁ - a ¹ 0 because otherwise the resolvent cubic implies b² = 0, which in turn leads to b = 0. Then $$(2z_1-a)y^2-by+(z_1^2-c) = (2z_1-a)\left(y-{b\over 2(2z_1-a)}\right)^2.$$ Thus, thanks to (2) we get $$(y^2+z_1)^2 = (2z_1-a)\left(y-{b\over 2(2z_1-a)}\right)^2.$$ Consequently, $$y^2+z_1 = \sqrt{2z_1-a}\left(y-{b\over 2(2z_1-a)}\right) \quad {\rm or} \quad y^2+z_1 = -\sqrt{2z_1-a}\left(y-{b\over 2(2z_1-a)}\right).$$

Solving these quadratics we get the four solutions of y⁴ + ay² + by + c = 0, which in turn lead to the four solutions of the original quartic through the use of the transformation x = y - d₁/4. We have to keep in mind that if 2z₁ - a < 0 we will have to deal with a quadratic that happens to have complex coefficients.

An alternative way to solve y⁴ + ay² + by + c = 0, through Ferrari's technique, starts by noticing that (y² + a/2)² = -by -c + a²/4 (recall Cardano's solution of problem VIII, discussed in detail in section 6). For any z we will have (y² + a/2 + z)² = -by - c + a²/4 + z² + 2z(y² + a/2), that is to say (y² + a/2 + z)² = 2zy² - by + (z²- c + a²/4 + az). In order to have a perfect square to the right of this expression we need to make the discriminant equal to zero, thus b² - 8z(z² - c + a²/4 + az) = 0. In other words, we need to solve the equation 8z³ + 8az² - 8cz + 2a²z - b² = 0, a resolvent cubic different from the one we found before.

Let z₁ be a real solution of it, which has to be different from zero because otherwise b = 0; therefore (y² + a/2 + z₁)² = 2z₁(y - b/4z₁)². The four solutions of y⁴ + ay² + by + c = 0 will stem from the quadratics (y² + a/2 + z₁) = √(2z₁)(y - b/4z₁), (y² + a/2 + z₁) = -√(2z₁₎(y - b/4z₁). Explicitly, the four solutions (real or complex) are $$y={1\over 2}\left(\sqrt{2z_1} \pm \sqrt{-2z_1-2a-b\sqrt{2/z_1}}\right),\quad y = {1\over 2}\left(-\sqrt{2z_1}\pm \sqrt{-2z_1-2a-b\sqrt{2/z_1}}\right).$$

Ferrari's technique works for all quartics, although it is not of practical use in the case of biquadratics. For instance, let us analyze the biquadratic equation x⁴ + 12 = 6x², whose complex solutions were found at the end of the previous section. We have (x²+z)² = 6x² - 12 + z² + 2zx² for any z. That is to say $$(x^2+z)^2 = (2z+6)x^2+(z^2-12)\ \ \ \ (3).$$

We wish to find z such that the right hand side of (3) is a perfect square. This will happen if its discriminant is zero; thus, -4(2z + 6)(z² - 12)=0. We can choose the solution √12. Replacing this value in (3) we get (x² + 2√3)² = (x√(4√3+6))². The four solutions follow from this equation; a lot of work taking into consideration that there is a straightforward alternative for biquadratics, which we analyzed in sections 2 and 8.

Solving the corresponding cubic is usually the only time-consuming task in the whole process. A natural question to ask is whether there is a simpler method to solve quartics. Rene Descartes (1596-1650) developed a novel approach, which he published in 1637 in The Geometry ([2], p. 180), almost 90 years after the first edition of Ars Magna. Let us succintly present Descartes' method from a modern perspective. For this purpose we will analyze Problem IX, chapter 39, of Cardano's book: x⁴ + 4x + 8 = 10x². First we try to express x⁴ - 10x² + 4x + 8 as a product of two quadratic factors for certain undetermined coefficients a,b,c,d, say:

Let r₁, r₂ be the solutions of x² + ax + b = 0 and r₃, r₄ the solutions of x² + dx + c = 0. Then r₁ + r₂ = -a and r₃ + r₄ = -d. But r₁, r₂, r₃, r₄ are solutions of a quartic without cubic term, hence r₁ + r₂ + r₃ + r₄ = 0. Therefore d = -a. The original problem is thus reduced to finding a, b, c such that

Expanding terms we get c + b = a² -1 0, c - b = 4/a, and bc = 8. Adding and subtracting the first two equalities we obtain b = (1/2)(a² - 10 - 4/a), c=(1/2)(a² - 10 + 4/a). Then 32 = 4bc = (a² - 10)² - 16/a², which is equivalent to u³ - 20u² + 68u - 16 = 0 with u = a². By simple inspection we note that u = 4 is a solution, hence a = 2, c = -2, and b = -4. That is to say, x⁴ - 10x² + 4x + 8 = (x² + 2x - 4)(x² - 2x - 2). As expected, the solutions of these quadratics are 1 ± √3, -1 ± √5 respectively, which happen to be the solutions of the original quartic.

Ferrari's approach compares favorably with Descartes' method, as was illustrated in the solution of the preceding problem. Both require the solution of a corresponding cubic; as a matter of fact, Galois theory implies that any algebraic method for quartics using radicals will have to go through the solution of a cubic, except for some particular cases like biquadratics. For instance, Euler's method ([5], pp. 282-288) requires, in general, the solution of a cubic too.

There is a particular case that has all biquadratics as a special subcase: namely, quasisymmetric equations, meaning equations of the form a₀x⁴ + a₁x³ + a₂x² + a₁mx + a₀m² = 0. To solve such an equation, divide by x² and then define z = x + m/x. We obtain a₀z² + a₁z + (a₂ - 2a₀m) = 0, a quadratic equation in z. For each solution z we will have to solve the equation x² - zx + m = 0. Actually, two equations of the above-mentioned type appear in chapter 34 of Cardano's book (problems II and III); he solves them through a rather convoluted "Rule for a Mean". It is worth noting that the transformation z = x + m/x does not appear in Ars Magna; it belongs to a later period in the history of algebra.

From a pedagogical perspective one might ask: Which method is easier to learn and apply? Apparently it is a matter of taste. Among 20th century authors, Dickson ([3], pp. 51-52) chose a variation of Ferrari's method, and Feferman ([6], pp. 349-350) preferred Descartes' method, while Weisner ([9], pp. 140-143) presented Euler's method. In any case, probably it is best to acquaint students with at least two methods. Each corresponds to a different period in the development of algebra.

One of the authors (MH) regularly discusses Ferrari's and Descartes' method in a course on teaching of secondary mathematics intended for seniors planning to become high school mathematics teachers (syllabus). One class is devoted to cubics and another to quartics (80-minute classes). Both topics could very well be chosen as enrichment units for high school students taking either Algebra II or Pre-Calculus; not only will they learn new algebraic techniques, but their understanding of quadratics might be enhanced. At the college level, students of Abstract Algebra would surely benefit from a historical approach to the solution of cubics and quartics; the topic is interesting on its own and lies at the foundation of several mathematical developments of the 19th and 20th century.

Chapter 39 of Ars Magna can be seen as exemplifying three principles that should still hold in the classroom today; namely, the need to learn more than one approach to the solution of a problem whenever this is possible, the advisability to travel from the particular to the general, and the reliance on methods rather than rules.

One might marvel that as early as the 1540's Lodovico Ferrari, who started as a servant in the household of Cardano, could have solved an outstanding open problem in algebra, namely finding the real roots of any quartic equation. Our admiration goes also to Cardano for having written an epoch-making mathematical book.

1. Cardano, G ., The Great Art or The Rules of Algebra, translated and edited by T. R. Witmer, The MIT Press, 1968.

2. Descartes, R ., The Geometry, translated by D.E. Smith and M.L. Latham, The Open Court Publishing Company, 1925.

3. Dickson, L.E., New First Course in the Theory of Equations, John Wiley & Sons, 1939.

4. Dunham, W., Euler and the Fundamental Theorem of Algebra, College Mathematics Journal, Vol. 22, 1991, 361-368.

5. Euler, L., Elements of Algebra, Fifth Edition, translated by J. Hewlett, 1840 (Springer Verlag reprint)

6. Feferman, S., The Number Systems, Addison-Wesley, 1964.

7. Katz, V., A History of Mathematics (3rd edition), Addison Wesley, 2009.

8. van der Waerden, B.L., A History of Algebra, Springer Verlag, 1985.

9. Weisner, L., Introduction to the Theory of Equations, The MacMillan Company, 1938