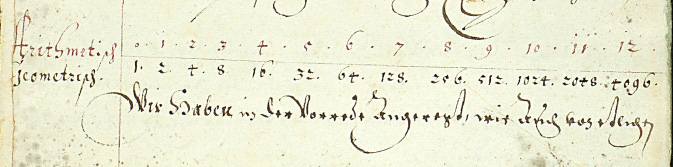Logarithms: The Early History of a Familiar Function
It may come as a surprise to many that often times mathematical concepts don't end up like they started! For those of you who think mathematics is timeless, fixed, and full of unchanging truths, such a proposition may seem unbelievable. But there are many instances in the history of mathematics of the development of a mathematical concept way beyond the purposes and potentialities that its original inventors intended. An example that will be familiar to you all is the logarithm.
What is a logarithm? Ask a modern mathematician nowadays and you will get a very different answer from the one you might have got from a mathematician several centuries ago. Indeed, even the very first mathematicians who worked with the logarithmic relation would have given an explanation that would seem quite foreign to a modern mathematician. So how did the logarithmic relation come about, and how is it that the concept underwent so much change? We will address these questions by looking at the emergence of this concept, and examining some of the issues surrounding its origins.
In fact, the question of the origins of the logarithmic relation does not have a simple answer. At least two scholars, the Scottish baron John Napier (1550-1617) and Swiss craftsman Joost Bürgi (1552-1632), produced independently systems that embodied the logarithmic relation and, within years of one another, produced tables for its use. This parallel insight is fascinating and rich in historical detail, and it reveals some methodological challenges for historians of mathematics. In light of all this, we will examine the ideas of these two scholars, as well as explore how historians have portrayed this intricate situation and the questions it raises about mathematics.
In large part, we intend to re-introduce teachers to a concept that is often taught without any reference to its original appearance on the mathematical scene. We hope that a close examination of Napier's and Bürgi's conceptions will enable teachers to consider alternative placement for introducing the idea of logarithms – as part of or after a unit on sequences. Furthermore, we provide in what follows mathematical and historical content, as well as student exercises, to promote the teaching of the logarithmic relation from its historical roots, which are firmly situated in simultaneous consideration of arithmetic and geometric sequences.
Logarithms: The Early History of a Familiar Function - Introduction
It may come as a surprise to many that often times mathematical concepts don't end up like they started! For those of you who think mathematics is timeless, fixed, and full of unchanging truths, such a proposition may seem unbelievable. But there are many instances in the history of mathematics of the development of a mathematical concept way beyond the purposes and potentialities that its original inventors intended. An example that will be familiar to you all is the logarithm.
What is a logarithm? Ask a modern mathematician nowadays and you will get a very different answer from the one you might have got from a mathematician several centuries ago. Indeed, even the very first mathematicians who worked with the logarithmic relation would have given an explanation that would seem quite foreign to a modern mathematician. So how did the logarithmic relation come about, and how is it that the concept underwent so much change? We will address these questions by looking at the emergence of this concept, and examining some of the issues surrounding its origins.
In fact, the question of the origins of the logarithmic relation does not have a simple answer. At least two scholars, the Scottish baron John Napier (1550-1617) and Swiss craftsman Joost Bürgi (1552-1632), produced independently systems that embodied the logarithmic relation and, within years of one another, produced tables for its use. This parallel insight is fascinating and rich in historical detail, and it reveals some methodological challenges for historians of mathematics. In light of all this, we will examine the ideas of these two scholars, as well as explore how historians have portrayed this intricate situation and the questions it raises about mathematics.
In large part, we intend to re-introduce teachers to a concept that is often taught without any reference to its original appearance on the mathematical scene. We hope that a close examination of Napier's and Bürgi's conceptions will enable teachers to consider alternative placement for introducing the idea of logarithms – as part of or after a unit on sequences. Furthermore, we provide in what follows mathematical and historical content, as well as student exercises, to promote the teaching of the logarithmic relation from its historical roots, which are firmly situated in simultaneous consideration of arithmetic and geometric sequences.
Logarithms: The Early History of a Familiar Function - Logarithms: A 'Great Tale' for Use in the Classroom
The logarithmic relation, captured in modern symbolic notation as \[ \log(a\cdot b) = \log(a) + \log(b),\] is useful primarily because of its power to reduce multiplication and division to the less involved operations of addition and subtraction. When this relation hit the scene in the early seventeenth century, its impact was substantial and immediate. Modern historians of mathematics, John Fauvel and Jan van Maanen (2000), illustrate this vividly:
When the English mathematician Henry Briggs learned in 1616 of the invention of logarithms by John Napier, he determined to travel the four hundred miles north to Edinburgh to meet the discoverer and talk to him in person. (p. xi)
Indeed, Fauvel and van Maanen assert that “the meeting of Briggs and Napier is one of the great tales in the history of mathematics” (p. xi). Unfortunately, it seems that many teachers (and their students) are not aware of this particular “great tale” – or, at most, superficially associate the names Briggs and Napier with the invention of the logarithm. Typically, these groups know little about the original conceptions of the logarithmic relation.
An anecdote concerning a conversation between Fauvel (1995) and a colleague reinforces this. Fauvel recounted that when he inquired of his colleague how to teach logarithms, the colleague responded, “Whatever for? Surely no one needs to learn about those any more, now that we have calculators and computers” (p. 39). Many teachers, when approached about the possibility of teaching logarithms using a historical context, may express the same opinion. Fauvel's counter-argument to his colleague was that “logarithms are a good and accessible example of something fundamentally changing its conceptual role within mathematics” (p. 45). Indeed, examining the historical development of the logarithm with students by exploring arithmetic and geometric progressions allows students “a more deeply rooted understanding of what is going on” (p. 42).
The modern concept of the logarithm typically appears late in a second algebra or precalculus course (grades 10 or 11 in the US), situated after a study of polynomial and rational functions, but before sequences and series and conic sections. This organization often leaves students with an impression of disconnectedness between mathematical topics. Complicating matters further, the logarithm is often presented only briefly in such an algebra or precalculus course, in order to lead to a broader study of logarithmic functions to match students' previous study of other functions (e.g., linear, polynomial, rational). Lastly, in order to focus on the study of logarithmic functions, instruction and curricular organization dictate that this function exist as the inverse of the exponential function. This, in particular, contrasts starkly with the historical circumstances: in fact, the trajectory of modern mathematical pedagogy does not imitate history, as exponentials arrived on the mathematical scene well after the introduction of the logarithm!
Victor Katz (1995; 1997) provided a succinct argument for examining the development of logarithms from a historical perspective. He observed that Napier developed logarithms “for use in the extensive plane and spherical trigonometrical calculations necessary for astronomy” (Katz, 1995, p. 49). Although the motivation for developing logarithms is significant, Katz noted that, in general, students today often know very little about astronomy and about the magnitude of both the numbers and the calculations involving such numbers that were necessary to advance the science of astronomy. Astronomical advances have remained critical throughout civilization, however, and Katz (1997) indicated that, “it is well for us to introduce it [astronomy] whenever possible” (p. 63).
Although there is a strong temptation simply to present the definition and several properties of the logarithm and exercises to practice each, we propose that incorporating original and parallel insights of the logarithm can enrich instruction and learning of the topic, both for this concept and more broadly for a student's understanding of mathematics and its relations and development.
Logarithms: The Early History of a Familiar Function - Before Logarithms: The Computational Demands of the Late Sixteenth Century
The late sixteenth century saw unprecedented development in many scientific fields; notably, observational astronomy, long-distance navigation, and geodesy science, or efforts to measure and represent the earth. These endeavors required much from mathematics. For the most part, their foundation was trigonometry, and trigonometric tables, identities, and related calculation were the subject of intensive enterprise. Typically, trigonometric functions were based on non-unity radii, such as \(R=10,000,000,\) to ensure precise integer output.* Reducing the calculation burden that resulted from dealing with such large numbers for practitioners in these applied disciplines, and with it, the errors that inevitably crept into the results, became a prime objective for mathematicians. As a result, much energy and scholarly effort were directed towards the art of computation.
Accordingly, techniques that could bypass lengthy processes, such as long multiplications or divisions, were explored. Of particular interest were those that replaced these processes with equivalent additions and subtractions. One method originating in the late sixteenth century that was used extensively to save computation was the technique called prosthaphaeresis, a compound constructed from the Greek terms prosthesis (addition) and aphaeresis (subtraction). This relation transformed long multiplications and divisions into additions and subtractions via trigonometric identities, such as: \[2\cos(A)\cos(B)=\cos(A+B)+\cos(A-B).\]
When one needed the product of two numbers \(x\) and \(y,\) for example, trigonometric tables would be consulted to find \(A\) and \(B\) such that: \[x=\cos(A)\;\;{\rm and}\;\;y=\cos(B).\] With \(A\) and \(B\) determined, \(\cos(A+B)\) and \(\cos(A-B)\) could be read from the table and half of the sum taken to find the original product in question. Thus the long multiplication of two numbers could be replaced by table look-up, addition, and halving. Such rules were recognized as early as the beginning of the sixteenth century by Johannes Werner in 1510, but their application specifically for multiplication first appeared in print in 1588 in a work by Nicolai Reymers Ursus (Thoren, 1988). Christopher Clavius extended the methods of prosthaphaeresis, of which examples can be found in his 1593 Astrolabium (Smith, 1959, p. 455).
Finally, with the scientific community focused on developing more powerful computational methods, the desire to capture symbolically essential mathematical ideas behind these developments was also growing. In the fifteenth and sixteenth centuries, mathematicians such as Nicolas Chuquet (c. 1430–1487) and Michael Stifel (c. 1487–1567) turned their attention to the relationship between arithmetic and geometric sequences while working to construct notation to express an exponential relationship. The focus on mathematical symbolism in centuries prior and the growing attention to notation–particularly the experimentation with different versions of exponent notation–played a critical role in the recognition and clarification of such a relationship. Now the mathematical connection between a geometric and an arithmetic sequence could be made all the more apparent by symbolically capturing these sequences as successive exponential powers of a given number and the exponents themselves, respectively (see Figure 6). The work on the relationships between sequences was mathematically important per se, but was equally significant for providing the inspiration for the development of the logarithmic relation.
________________________
*Note: Modern trigonometry is essentially based on triangles inscribed in a unit circle; that is, a circle with radius \(R = 1\). Early practitioners used circles with various values for the radius. The relationship between the modern sine function and a sine or half-chord in a circle of radius \(R\) is given by \({\rm Sin}\,{\theta}=R\sin \theta\), where the modern sine function has a lower case '\(\rm{s}\)' and the pre-modern sine an upper case '\(\rm{S}\)'.
Logarithms: The Early History of a Familiar Function - John Napier Introduces Logarithms
In such conditions, it is hardly surprising that many mathematicians were acutely aware of the issues of computation and were dedicated to relieving practitioners of the calculation burden. In particular, the Scottish mathematician John Napier was famous for his devices to assist with computation. He invented a well-known mathematical artifact, the ingenious numbering rods more quaintly known as “Napier's bones,” that offered mechanical means for facilitating computation. (For additional information on “Napier's bones,” see the article, “John Napier: His Life, His Logs, and His Bones” (2006).) In addition, Napier recognized the potential of the recent developments in mathematics, particularly those of prosthaphaeresis, decimal fractions, and symbolic index arithmetic, to tackle the issue of reducing computation. He appreciated that, for the most part, practitioners who had laborious computations generally did them in the context of trigonometry. Therefore, as well as developing the logarithmic relation, Napier set it in a trigonometric context so it would be even more relevant.

Figure 1. John Napier (1550-1617)
(from MacTutor History of Mathematics Archive)
Napier first published his work on logarithms in 1614 under the title Mirifici logarithmorum canonis descriptio, which translates literally as A Description of the Wonderful Table of Logarithms. Indeed, the very title Napier selected reveals his high ambitions for this technique---the provision of tables based on a relation that would be nothing short of “wonder-working” for practitioners. As well as providing a short overview of the mathematical details, Napier gave technical expression to his concept. He coined a term from the two ancient Greek terms logos, meaning proportion, and arithmos, meaning number; compounding them to produce the word “logarithm.” Napier used this word as well as the designations “natural” and “artificial” for numbers and their logarithms, respectively, in his text.
Despite the obvious connection with the existing techniques of prosthaphaeresis and sequences, Napier grounded his conception of the logarithm in a kinematic framework. The motivation behind this approach is still not well understood by historians of mathematics. Napier imagined two particles traveling along two parallel lines. The first line was of infinite length and the second of a fixed length (see Figures 2 and 3). Napier imagined the two particles to start from the same (horizontal) position at the same time with the same velocity. The first particle he set in uniform motion on the line of infinite length so that it covered equal distances in equal times. The second particle he set in motion on the finite line segment so that its velocity was proportional to the distance remaining from the particle to the fixed terminal point of the line segment.
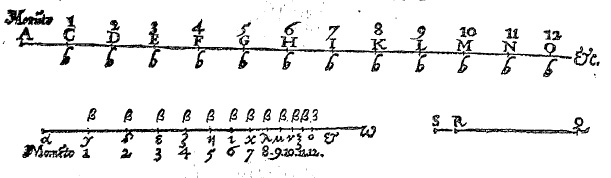
Figure 2. Napier's two parallel lines with moving particles
(Image used courtesy of Landmarks of Science Series, NewsBank-Readex)
More specifically, at any moment the distance not yet covered on the second (finite) line was the sine and the traversed distance on the first (infinite) line was the logarithm of the sine. This had the result that as the sines decreased, Napier's logarithms increased. Furthermore, the sines decreased in geometric proportion, and the logarithms increased in arithmetic proportion. We can summarize Napier's explanation as follows (Descriptio I, 1 (p. 4); see Figure 3): \[AC = \log_{nap}(\gamma\omega)\;\;{\rm where}\;\;\gamma\omega = {\rm Sin}\,\theta_{1}\]
\[AD = \log_{nap}(\delta\omega)\;\;{\rm where}\;\;\delta\omega = {\rm Sin}\,\theta_{2}\]
\[AE = \log_{nap}(\epsilon\omega)\;\;{\rm where}\;\;\epsilon\omega = {\rm Sin}\,\theta_{3}\]
and so on, so that, more generally: \[x={\rm Sin}\,(\theta)\]
\[y={\log_{nap}}(x)\] where \( \log_{nap}\) has been used to distinguish Napier's particular understanding of the logarithm concept from the modern one.
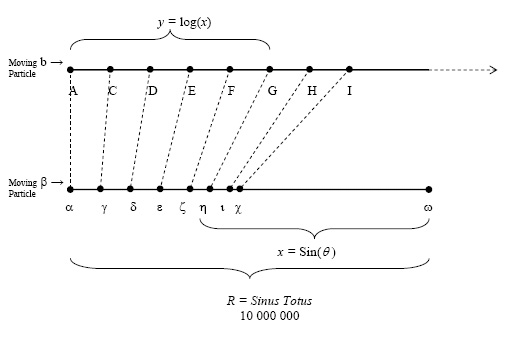
Figure 3. The relation between the two lines and the logs and sines
Napier generated numerical entries for a table embodying this relationship. He arranged his table by taking increments of arc \(\theta\) minute by minute, then listing the sine of each minute of arc, and then its corresponding logarithm. However in terms of the way he actually computed these entries, he would have in fact worked in the opposite manner, generating the logarithms first and then choosing those that corresponded to a sine of an arc, which accordingly formed the argument. For example, he would have computed values that appear in the first column of Table 1 via the relation: \[p_{n+1}=p_n\left(1-{\frac{1}{10^7}}\right)\;\;{\rm where}\;\;{p_0=10^7}.\]
| \(p_{n}\) | \(n=\log_{nap}(p_n)\) | Corresponding angle (\(\theta\)) |
| \({\bf{10000000.0000000}}\) | \(0\) | \(90^{\circ}00^{\prime}\) |
| \({\bf{9999999.0000000}}\) | \(1\) | \(89^{\circ}59^{\prime}\) |
| \({\bf{9999998.0000001}}\) | \(2\) | \(89^{\circ}58^{\prime}\) |
| \(9999997.0000003\) | \(3\) | |
| \({\bf{9999996.0000006}}\) | \(4\) | \(89^{\circ}57^{\prime}\) |
| \(9999995.0000010\) | \(5\) | |
| \(9999994.0000015\) | \(6\) | |
| \({\bf{9999993.0000021}}\) | \(7\) | \(89^{\circ}56^{\prime}\) |
| \(9999992.0000028\) | \(8\) | |
| \(9999991.0000036\) | \(9\) | |
| \(9999990.0000045\) | \(10\) | |
| \({\bf{9999989.0000055}}\) | \(11\) | \(89^{\circ}55^{\prime}\) |
Table 1. Napier's logarithms
The values in the first column (in bold) that corresponded to the Sines of the minutes of arcs (third column) were extracted, along with their accompanying logarithms (column 2) and arranged in the table. The appropriate values from Table 1 can be seen in rows one to six of the last three columns in Figure 4. Napier tabulated his logarithms from \(0^{\circ}\) to \(45^{\circ}\) in minutes of arc, and by symmetry provided values for the entire first quadrant. The excerpt in Figure 4 gives the first half of the first degree and, by symmetry, on the right the last half of the eighty-ninth degree.
To complete the tables, Napier computed almost ten million entries from which he selected the appropriate values. Napier himself reckoned that computing this many entries had taken him twenty years, which would put the beginning of his endeavors as far back as 1594.
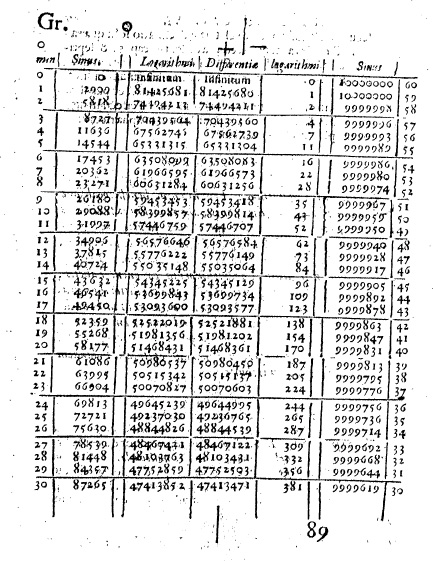
Figure 4. The first page of Napier's tables
(Image used courtesy of Landmarks of Science Series, NewsBank-Readex)
Napier frequently demonstrated the benefits of his method. For example, he worked through a problem involving the computation of mean proportionals, sometimes known as the geometric mean. He reviewed the usual way in which this would have been computed, and pointed out that his technique using logarithms not only finds the answer “earlier” (that is, faster!), but also uses only one addition and one division by two! He stated:
Let the extremes 1000000 and 500000 bee given, and let the meane proportionall be sought: that commonly is found by multiplying the extreames given, one by another, and extracting the square root of the product. But we finde it earlier thus; We adde the Logarithme of the extreames 0 and 693147, the summe whereof is 693147 which we divide by 2 and the quotient 346573 shall be the Logar. of the middle proportionall desired. By which the middle proportionall 707107, and his arch 45 degrees are found as before.... found by addition onely, and division by two. (Book I, 5 (p. 25), as translated by Edward Wright)
In order to find the mean proportional by traditional methods, Napier observed that one has to compute the product and then take the square root; that is: \[\sqrt{1 000 000 \times 500 000} = \sqrt{500 000 000 000} \approx 707106.78\] This method involves the multiplication of two large numbers and a lengthy square-root extraction. As an alternative, Napier proposed (with computations to 6 significant figures): \[\log_{nap}(1 000 000) + \log_{nap}(500 000) = 0 + 693147 = 693147\]
\[693147 \div 2 = 346573\;\;\;{\rm to}\;\;6\;\;{\rm significant}\;\;{\rm figures}\]
\[\Rightarrow {\rm mean}\;\;{\rm proportional} = 707107,\;\;\;{\rm as}\;\;{\rm required,}\] which he rightly deemed was much simpler to compute.
Logarithms: The Early History of a Familiar Function - Joost Bürgi Introduces Logarithms
At about the same time in Switzerland, Joost Bürgi, a court clock maker by profession, grappled with the same issues of computation. Bürgi's key motivation was not only to facilitate computation, but also to produce a single table that could be applied to all arithmetical operations, rather than needing various tables to perform them all. In his work, Arithmetische und Geometrische Progress Tabulen (Arithmetic and Geometric Progression Tables), published in 1620, Bürgi noted that having separate tables for multiplication, division, square roots, and cube roots is “not alone irksome, but also laborious and cumbersome” (Preface, 1, xi-xii).

Figure 5. Joost Bürgi (1552-1632)
(from MacTutor History of Mathematics Archive)
Furthermore, Bürgi grounded his conception directly in the relation between two progressions. He stated that he was able to create one table for a multiplicity of calculations by considering two “self-producing and corresponding progressions” (Preface, 1, xv-xvi): one arithmetic and the other geometric. To illustrate the underlying principle by means of “nice” numbers, he gave corresponding progressions based on the powers of two, as shown in Figure 6.
Figure 6. Bürgi presents an arithmetic progression (top numbers in red) \(0,1,2,3,\) ... and beneath it a corresponding geometric sequence, \(1,2,4,8,\) ...; that is \(2^0,2^1,2^2,2^3\),...
(Image used by permission of University Library - Graz)
Bürgi gave the relation of powers of two as an example, but in fact different parameters underpinned his logarithmic relation. As he noted, successive powers of two increase too quickly to be useful to interpolate between values so instead he used a common ratio of \(1.0001\), and the successive values were tabulated as follows: \[b_{n+1} = b_{n}(1.0001)\;\;{\rm where}\;\;b_{0} = 10^8\]
Thus, each successive value in the table can be generated by multiplying the previous one by \(1.0001\). Bürgi used the factor of \(10^8\) to allow for greater integer precision.
For example, the logarithm of \(101907877\) (a black number) can be found using the tables as follows (we must note that his logarithm values increased by \(10\) and were also multiplied by a factor of \(10\)).
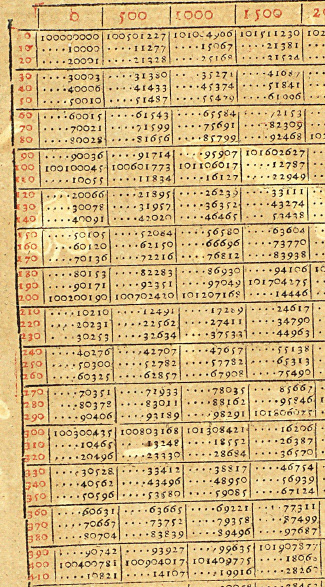
Figure 7. Locating a logarithm value
(Image used by permission of University Library - Graz)
One takes the black number \(101907877\) from the table (see Figure 7) and finds the corresponding red number or logarithm; in this case it is \(189\). To check the value \(101907877\), we see: \[101907877 = (10^8)(1.0001)^{189}.\]
One of the interesting aspects of Bürgi's system was his use of color to emphasize the relationship between the arithmetic and geometric progressions. Unlike Napier, Bürgi did not develop technical terminology to capture the relationship, but simply referred to numbers and their logarithmic counterparts as black numbers and red numbers, respectively, and used black and red ink to maintain this distinction. This was done consistently throughout the text as well as in the tables: the arguments of tabulation, the logarithms, are printed in red, and the tabular entries, the ordinary numbers or antilogarithms, are printed in black.
Bürgi stated that his tables could be used for all manner of calculations, including multiplication, division, extraction of roots, and computing mean proportionals. He provided some 26 examples of the various calculations and the corresponding use of the tables. Here we examine two of his examples. To multiply two numbers using the tables, Bürgi simply listed the black numbers (antilogarithms), identified their corresponding red numbers (logarithms) and added them, and finally determined the black number corresponding to the sum. (Bürgi described linear interpolation methods whenever corresponding black or red numbers were not found in the tables.) For example, Bürgi set out \(154030185\) to be multiplied by \(205518112\) (given as black numbers). To do this he found their corresponding logarithms (red numbers) in the tables, then added them together, then converted the resulting red number back to its original (antilogarithmic) black number, as follows:
| \(154030185\times 205518112\) | \(\leftrightarrow\) | \(\log_{burgi}(154030185)\)+\(\log_{burgi}(205518112)\) |
| =\(43200\)+\(72040\) | ||
| =\(115240\) |
This sum, \(115240\), is then located in the tables and the corresponding black number (the product) is \(316559928\).
The cube root of a very large number is also easily computed using Bürgi's tables. For example, to extract the cube root of \(561203700\) (a given black number), Bürgi located the corresponding logarithm (red number) and simply took a third of it, as follows: \[\sqrt[3]{561203700}\Rightarrow \log_{burgi}(561203700) = 172500\]
\[172500 \div 3 = 57500\]
\[57500 = \log_{burgi}(X) \Rightarrow X = 177.707944 \;{\rm as}\;{\rm required}\] so that the desired cube root is \(177.707944\). This process bypasses an involved cube root extraction algorithm.
Bürgi computed logarithms for \(100 000 000\) to \(1 000 000 000\). This filled fifty-eight pages of tables for a total of 23,030 entries (23,027, plus an additional 3 entries) to be computed to eight significant digits. In addition to the tables, Bürgi wrote an accompanying text, which gave his general motivations as well as many worked examples to show exactly how the tables could be used to lessen the labors of computation. These were published in 1620, although there are reasons to suppose that the tables were in existence prior to this time.
Logarithms: The Early History of a Familiar Function - The Challenges of Parallel Insights in the History of Mathematics
When French mathematician Pierre-Simon Laplace noted that logarithms “by shortening the labors, doubled the life of an astronomer,”** he hardly exaggerated. Where previously practitioners had been slowed down by long and tedious calculations required in their disciplines, now that logarithms had been introduced, they literally had to spend only half as much time on these necessary computations. Therefore, right from its inception, there has never been any doubt as to the importance of the logarithmic relation. Furthermore, its usefulness has persisted in different areas of mathematics right to the present day.
As a result, the logarithm has always formed an important part of historical scholarship, with many historians reflecting on various aspects of its development. In particular, the introduction of the logarithm by (at least) two individuals seemingly simultaneously has led historians of mathematics to wonder to whom to attribute the “discovery.” This issue of priority is a compelling one. When a mathematical idea or concept is introduced independently at roughly the same time by different scholars, historians are faced with an interesting dilemma. They can identify a single individual as ultimately responsible for the idea. Or, they can resist this inclination to focus on a single person as preeminent over all others, and instead consider on equal footing the multiple proposals given by the various scholars and what these proposals reveal more broadly about the mathematical scene in which these ideas flourished. The former approach often has the consequence of selecting that individual whose proposal most resembles how we understand the concept today, which can often be considered anachronistic and even ahistorical. The latter approach tends to result in a broader contextual historical account and for this reason, many historians would argue, is preferable.
One particular historian of mathematics, Florian Cajori, concluded at the very beginning of his work on the history of logarithms, “Few inventors have a clearer title to priority than has Napier to the invention of logarithms” (Cajori, 1915, p. 93; for other historians who hold a similar position, see Amy Shell-Gellasch (“Napier’s e,” 2008) and David E. Smith (1959, p. 149)). From the outset then, Cajori's focus was on Napier's claim to preeminence with respect to the logarithm concept, and his evaluation consisted of a systematic examination of Napier's contemporaries to reject any claims they might have had to this priority.
Indeed, Cajori brings to mind the challenges related to the retrospective appraisal of ideas in history. To find traces of an idea in the work of mathematicians past is too easily done from a future vantage point, and it risks anachronism or ignoring the context in which these ideas were understood. Accordingly, to speak of Napier’s “clear title to priority” to logarithms obscures the fact that his proposal remained always within a particular context for Napier. His logarithm concept was not expansive enough to include the general notion of a function under which we understand logarithms today.
Another approach for historians is rather to examine more roundly the various proposals and to refrain from attaching a single historical figure to the emergence of the logarithm. Focusing on the question of priority in mathematics causes scholars to look at the similarities between different manifestations of the same concept. This is very valuable for the history of mathematics, but emphasizing these similarities can distract from the more insightful issue of how mathematical inventors experimented with the concept, both imperfectly and successfully, and articulated it. Parallel insights reveal an abundant legacy of the motivations of these inventors and of challenges they faced and the ways in which they responded. They reveal the various individual and creative ways in which mathematicians reacted to the intellectual environment around then, and the unique methods or products they developed as a result of these stimuli. Parallel insights are in fact immensely rich for the historian; that legacy is too significant to be obscured by the determination to single out one individual as ultimately preeminent over the others. The parallel insights of Napier and Bürgi epitomize this. As we have seen, both presentations were richly and meaningfully different in many respects. Their conceptions were characterized by distinctive motivations, general designs, presentations, theoretical backdrops, and were also different in many of the details.
________________________
**Note: This quote is frequently invoked in modern scholarship, but its origin remains a mystery to the authors of this paper, who have been unable to track it down in primary source material. Whether or not Laplace actually made the statement remains an outstanding question.
Logarithms: The Early History of a Familiar Function - Parallel Insights and the Reception of Mathematical Ideas
All of this leads us to the question: what causes some mathematical ideas to get a better reception than others? History shows us that it is not simply a question of mathematical merit, nor the precise instant at which they officially entered the public domain, but that many other factors can be influential. Napier was keen to spread his ideas. We can see this clearly in his endorsement of the translation into English of his work by his contemporary Edward Wright (1616). Napier expressed his delight in “this secret invention ... so much the better as it shall be the more common.” Evidently, Napier was concerned with disseminating his ideas as widely as possible.
In contrast, even Bürgi's contemporaries noted that Bürgi made little effort in promoting his work. Johann Kepler, who was most likely aware of Bürgi's endeavors while the two served in the court of Duke Wilhelm II, the Langrave of Hesse-Kassel (d. 1592), portrayed Bürgi as being neglectful of his ideas and failing to promote them more widely. Kepler noted:
These logistic indices ... showed Iustus Byrgi many years before the edition of Napier the way to these very same logarithms. Even so, this man, a procrastinator and guardian of his secrets, abandoned his baby in childbirth and did not nurture it for public use. (As cited in Kepler, Tabulae Rudolphinae, 1627, p. 48 (Gronau, 1996, p. 6))
In addition, Napier had two works on logarithms published. Bürgi had only one published, though another was promised. One can only guess at the difference in reception had this second, more “theoretical” work made its way to public consumption. Napier's first work was published in 1614 and was typeset and printed. Bürgi's was published in 1620, and although his tables were printed, his original manuscript remains to the present day only in handwritten form. Napier was mindful of his audience, tailoring his system to those who used arithmetical operations in a trigonometric context. His table entries, as we have seen, were essentially logarithms of sines of uniformly increasing angles. Bürgi's tables were not compiled in this way and needed (at least) an additional step to be useful in a trigonometric context.
Napier was part of the busier academic community, and had his work translated into the vernacular by Wright almost immediately. Bürgi wrote in German, which was arguably less accessible to the wider community. Furthermore, Napier seems to have responded more quickly to the reaction of his contemporaries and to suggestions they had for improving the system. Napier updated his system and made various accommodations so that it would be easier to use. His contemporaries, chief among them Briggs, helped figure out and initiate these changes, and published the results (Jagger, 2003). A primary improvement of Napier's logarithms was the modification that (log 1 = 0), usually credited to Henry Briggs. Most likely this important change was not solely Briggs' idea. However, the very quick meeting and collaboration between the two men enabled “the idea [to come] about in discussions between Napier and Briggs” (O'Connor & Robertson, 1999). Napier was an established scholar; Bürgi was an employee of the court with (presumably) limited resources and connections, despite the presence of Kepler.
Logarithms: The Early History of a Familiar Function - Conclusion
Logarithms represented at this time in so many ways both what was old and what was new. This relation looked back to reflect concerns of computation, but looked forward to nascent notions about mathematical functions. Although logarithms were primarily a tool for facilitating computation, they were but another of the crucial insights that directed the attention of mathematical scholars towards more abstract organizing notions. But one thing is very clear: the concept of logarithm as we understand it today as a function is quite different in many respects from how it was originally conceived. But eventually, through the work, consideration, and development of many mathematicians, the logarithm became far more than a useful way to compute with large unwieldy numbers. It became a mathematical relation and function in its own right.
In time, the logarithm evolved from a labor saving device to become one of the core functions in mathematics. Today, it has been extended to negative and complex numbers and it is vital in many modern branches of mathematics. It has an important role in group theory and is key to calculus, with its straightforward derivatives and its appearance in the solutions to various integrals. Logarithms form the basis of the Richter scale and the measure of pH, and they characterize the music intervals in the octave, to name but a few applications. Ironically, the logarithm still serves as a labor saving device of sorts, but not for the benefit of human effort! It is often used by computers to approximate certain operations that would be too costly, in terms of computer power, to evaluate directly, particularly those of the form \(x^n\).
Logarithms: The Early History of a Familiar Function - Appendix: Student Tasks
The Logarithms of Napier and Bürgi
1. Laplace: “Logarithms, by shortening the labors, doubled the life of an astronomer.” What does this quote mean? Give an example of another “device” that can be thought of as having the same effect on mathematicians, scientists, or other professionals.
2. As discussed, Napier's tables were not tabulated in terms of logarithms themselves but rather by the minutes of arc and their sines. But that is not all he included! Ingeniously he organized his data into seven columns, as seen in Figure 8, including the angle, its corresponding sine, and the corresponding logarithm, as well as the logarithm of its tangent, the logarithm of its cosine, its cosine, and the complement of the angle. From your knowledge of trigonometry and especially the relations between sine, cosine, and tangent, identify each of these columns and explain how they are related (hint: the fourth column is called “Differences”).
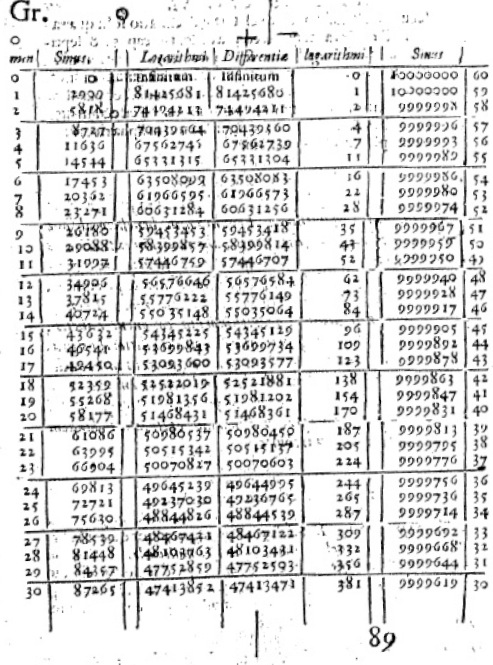
Figure 8. An excerpt from the first page of Napier’s tables
(Image used courtesy of Landmarks of Science Series, NewsBank-Readex)
3. Using the excerpt in Figure 9 from page 3 of Bürgi's Arithmetische und Geometrische Progress Tabulen (1620), explain the calculations (on the red numbers) that he used to determine the fourth value in the following “rule of three” computation:
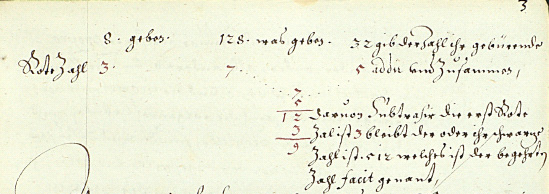
Figure 9. An excerpt from Bürgi's Progress Tabulen (Image used by permission of University Library - Graz)
Translation:
8 gives 128 which gives 32. Into their appropriate red numbers:
| 3 | 7 | 5 add together: | |||||
| 7 | |||||||
| 5 | |||||||
| 12 | Subtract from this the first red number, 3, | ||||||
| 3 | |||||||
| 9 | its black number is 512 which is the desired numer. |
4. Solve the proportion in Problem 3 using a modern method and verify the results.
5. Using the relationship, \[b_{n} = (10^8)(1.0001)^{R/10},\] where \(b_{n}\) is a black number and \( R\) is its corresponding red number, verify the table entries for \(R = 221500,\) \(221510,\) and \( 221520\), as given in the upper right portion of Figure 10.
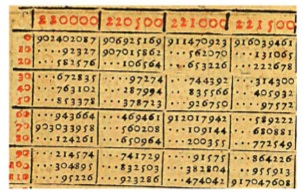
Figure 10. Another excerpt from Burgi’s tables
(Image used by permission of University Library - Graz)
6. The “Temple of Astronomy” (below, in Figure 11) appears as the frontispiece in Kepler's Rudolphine tables, a work commissioned in 1601 (and completed in 1627) by the emperor Rudolf II. The temple shows six muses of astronomy, the goddesses Physica (physics), Optica (optics), Geometrica (geometry), Stathmica (weights), Magnetica (magnetics), and Logarithma (logarithms). Logarithma, placed third from the left, holds in her hands two unequal measuring sticks, rods of the ratio of one to two. Around her head is haloed the number “\(6931472.\)”
(a) What does this number represent and why do you think it appears in Logarithma's halo?
(b) Match the remaining five goddesses with their correct names.

Figure 11. Temple of Astronomy from Kepler’s Rudolphine Tables
(Image used by permission of the Columbia University Library. For two more images from Kepler's Rudolphine Tables, see "Mathematical Treasures" in Convergence.)
Logarithms: The Early History of a Familiar Function - Bibliography
Bürgi, J. (1620). Arithmetische und geometrische progress tabulen/sambt gründlichem unterricht/wie solche nützlich in allerley rechnungen zu gebrauchen/und verstanden werden sol. Prague: Paul Sessen.
Cajori, F. (1915). Algebra in Napier's day and alleged prior inventions of logarithms. In C. G. Knott (Ed.), Napier tercentenary memorial volume (pp. 93–109). London: Longmans, Green and Company.
Caulfield, M.J. (2006). John Napier: His Life, His Logs, and His Bones. Retrieved on October 16, 2009 from http://mathdl.maa.org/mathDL/46/?pa=content&sa=viewDocument&nodeId=2331
Fauvel, J., & J. van Maanen (Eds.) (2000). History in mathematics education: The ICMI study. Dordrecht, The Netherlands: Kluwer.
Fauvel, J. (1995). Revisiting the history of logarithms. In F. Swetz, J. Fauvel, O. Bekken, B. Johansson, & V. Katz (Eds.), Learn from the masters! (pp. 39–48). Washington, DC: Mathematical Association of America.
Gronau, D. (1996). The logarithm: From calculation to functional equations. Notices of the South African Mathematical Society 28(1), 60–66.
Jagger, G. (2003). The making of logarithm tables. In M. Campbell-Kelly, M. Croarken, R. Flood, & E. Robson (Eds.), The history of mathematical tables: From Sumer to spreadsheets (pp. 48–77). Oxford: Oxford University Press.
Katz, V.J. (1995). Napier's logarithms adapted for today's classroom. In F. Swetz, J. Fauvel, O. Bekken, B. Johansson, & V. Katz (Eds.), Learn from the masters! (pp. 49–55). Washington, DC: Mathematical Association of America.
Katz, V. (1997). Some ideas on the use of history in the teaching of mathematics, For the Learning of Mathematics 17(1), 62–63.
Napier, J. (1614). Mirifici Logarithmorum Canonis descriptio, Eiusque usus, in utraque Trigonometria; ut etiam in omni Logistica Mathematica, Amplissimi, Facillimi, and expeditissimi explicatis. Edinburgi: Ex officima Andreae Hart Biblipoloae.
O'Connor, J. J., and E.F. Robertson, (1999). Henry Briggs. Retrieved April 20, 2010 from http://www-history.mcs.st-andrews.ac.uk/Biographies/Briggs.html
Shell-Gellasch, A. (2008). Napier's e. Retrieved on October 16, 2009 from http://mathdl.maa.org/mathDL/46/?pa=content&sa=viewDocument&nodeId=3209
Smith, D.E. (1958). History of mathematics. New York: Dover Publications.
Smith, D.E. (1959). A source book in mathematics. New York: Dover Publications. Originally published in 1929.
Thoren, V. (1988). Prosthaphaeresis revisited. Historia Mathematica 15, 32–39.
Wright, E. (1616). A description of the admirable table of logarithms. Translated from J. Napier's Latin to English. London: Nicholas Oaks. Partial transcription: http://www.johnnapier.com/table_of_logarithms_001.htm
Logarithms: The Early History of a Familiar Function - About the Authors and More Information on Sources
About the Authors
Kathleen M. Clark is an Assistant Professor of Mathematics Education and a core-faculty member in the FSU-Teach program at Florida State University. Her research interests include: the impact of the study of history of mathematics on teachers' mathematical knowledge for teaching, the ways in which teachers use history in the teaching of mathematics, and the practices and development of mathematics teacher educators.
Clemency Montelle is a Lecturer in the Department of Mathematics and Statistics at the University of Christchurch, New Zealand. Her research interests include history and philosophy of mathematics, with an emphasis on the preparation, translation, and commentary of ancient mathematical texts in Greek, Latin, Sanskrit, Arabic and Akkadian and ancient mathematical astronomy and modeling.
More Information on Sources
The portraits of Napier and Bürgi in Figures 1 and 5 are from the MacTutor History of Mathematics Archive (http://www-history.mcs.st-and.ac.uk/). The portrait of Bürgi in Figure 5 also is in the Convergence Portrait Gallery.
A transcription of Booke I of Wright’s 1616 translation of Napier's 1614 Mirifici logarithmorum canonis descriptio, titled A description of the admirable table of logarithms, is available from http://www.johnnapier.com/ The transcription itself begins at http://www.johnnapier.com/table_of_logarithms_001.htm
Translations into English of very brief passages from Napier's 1614 Descriptio also appear in the following sources.
Fauvel, John, and Jeremy Gray (1987). The history of mathematics: a reader. London: Macmillan and Milton Keynes: The Open University, pp. 296-297.
Stedall, Jacqueline (2008). Mathematics emerging: a sourcebook, 1540-1900. Oxford University Press, pp. 39-42.
Translations into English of parts of Napier's 1619 Mirifici logarithmorum canonis constructio (Construction of the wonderful table of logarithms) appear in the following sources.
Smith, D.E. (1959). A source book in mathematics. New York: Dover Publications, pp. 149-155.
Struik, Dirk J. (1969). A source book in mathematics, 1200-1800. Cambridge, Mass.: Harvard University Press, pp. 12-21.
The following book contains a translation into English of the entire Mirifici logarithmorum canonis constructio.
Macdonald, William R. (1889). The construction of the wonderful canon of logarithms by John Napier, Edinburgh and London: W. Blackwood and Sons.
A copy of Napier's Mirifici logarithmorum canonis constructio from 1620 is available from Google Books.
Finally, modern translations of both the Descriptio and the Constructio by Ian Bruce are available from Bruce's "Some Mathematical Works of the 17th and 18th Centuries" (http://www.17centurymaths.com/) website.