In 1882, Thomas Muir wrote the following passage in his Treatise on the theory of determinants ([TM, p. 224]), giving the definition of the Wronskian with which we are familiar today.

This passage was actually the first time that someone called these matrices Wronskians, after Jozef Maria Hoene-Wronski ([PP]). Muir continued ([TM, p. 234]):

Obviously, mathematicians accepted Muir’s suggestion with zeal, as evidenced by the fact that we continue associating Wronski’s name with these determinants today. Most students see the Wronskian in a differential equations class during the discussion of fundamental sets of solutions. 

We learn in basic linear algebra that if functions \( y_1,y_2,\dots,y_n \) are linearly dependent, then the determinant of the Wronskian will be zero. However, as noted by Bostan and Dumas in [BD], if one were to open any modern text in differential equations, one would find the following warning: “linearly independent functions may have an identically zero Wronskian!” 

The story behind this warning is quite interesting. For years, respected mathematicians took it for granted, and even provided proofs, that a zero Wronskian would imply linear dependence. The first person to realize that this was not true appears to have been Giuseppe Peano, but even after he provided an elementary counterexample, people had difficulty understanding the subtlety of the situation – just as our students often do!

Mark Krusemeyer explained, “This warning was first given by Peano ...; he actually had to give it twice, because an editor added a mistaken footnote that contradicted the main point of Peano’s first warning” [K]. The two papers to which Krusemeyer referred are short notes (each is less than two pages) published in 1889 in the French journal Mathesis. The articles appeared in the exact same issue, 25 pages apart. They can be seen in the original French in [P1] and [P2] and translated in their entirety in Appendix 2. In this paper we present a translation and analysis of these two notes by Peano, the mistaken footnote added by the editor, as well as the other papers Peano cites.

We will begin by translating Peano’s first note, Sur le déterminant Wronskien ([P1]), which contains his original warning, an example showing that linearly independent functions may have a zero Wronskian, and the footnote that contradicts his main point. We next translate this footnote, added by editor Paul Mansion, which makes reference to Mansion’s textbook on analysis. This textbook contains an incorrect proof that a zero Wronskian implies linear dependence.

We continue by translating Peano’s second note, Sur les Wronskiens ([P2]), which is an excerpt of a letter from Peano to the editors. It explains in no uncertain terms the problem with stating that a zero Wronskian provides a sufficient condition for equations to be linearly dependent. He also gave the example we typically see in an ordinary differential equations course today. At the end of this letter he implied that he had never seen this fact accurately expressed, and gave several citations of published incorrect propositions. Our last translations are of these cited works.

Our paper finishes by looking at ways in which this project could be used in a standard ordinary differential equations class. It is our hope to show how it can be a helpful addition to a differential equations course—either as a short point of interest, or as a starting point for homework, projects, or exam questions.

Peano opened his first article, Sur le déterminant Wronskien, by citing a proposition that most of our students assume to be true, and apparently most mathematicians did as well until 1889.

1. On the Wronskian determinant (by G. Peano, professor at the University of Turin). In nearly all papers, one finds the proposition: If the determinant formed with n functions of the same variable and their derivatives of orders \( 1,\dots,(n-1)\) is identically zero, there is between these functions a homogeneous linear relationship with constant coefficients.

Passage 1

Today, we would say that these functions are linearly dependent.

It is true that if the functions are linearly dependent, their Wronskian is zero. To see this, consider \( y_1 = c_2 y_2 +\cdots + c_n y_n\). By the linearity of the differential, all derivatives of \( y_1,y_2,\dots,y_n\) must obey the exact same relation. This means that the entire first column of the Wronskian can be written as a linear combination of other columns, implying a zero determinant.

The proposition in question, then, asserts the converse of this self-evident theorem, namely that the vanishing of the Wronskian is sufficient to demonstrate linear dependence. To disprove this proposition, Peano gave a simple counterexample. He presented two linearly independent continuous functions \( x(t)\) and \( y(t),\) each with continuous derivatives, that have a zero Wronskian at every real number.

This wording is too general. We offer, in fact, \[ x = t^2 [1+\phi(t)],\quad\quad y = t^2 [1-\phi(t)],\] where \( \phi(t)\) designates a function equal to zero for \( t = 0\), equal to \( 1\) for positive \( t \), to \( -1\) for negative \( t \) (*). One has for \[ t < 0,\quad \phi(t)=-1,\quad x=0,\quad {y=2t^2};\] for \[ t= 0,\quad \phi(t)=0,\quad x=0,\quad {y=0};\] for \[ t > 0,\quad \phi(t)=1,\quad {x=2t^2},\quad {y=0}.\] The functions \( x\) and \( y,\) and their derivatives \[ {x^\prime} = 2t [1+\phi(t)],\quad\quad {y^\prime} = 2t [1-\phi(t)],\] are continuous, and one has, for all values of \( t ,\) \[ \left| \begin{array}{cc} x&y\\x^\prime&y^\prime\\ \end{array} \right| =0 \] But between \( x\) and \( y,\) there is no relation of the expressed form, because the coordinate point \( (x, y) \) does not describe a straight line passing through the origin, but the two half-axes \( ox\) and \( oy.\)

Passage 2

Notice that the argument for linear dependence is very geometric. Peano was using the fact that two functions are linearly dependent if and only if they are constant multiples of each other, since rearrangement of \( c_1 f_1 + c_2 f_2 = 0 \) will give \( f_1 = c\,f_2 \). Hence, if one were to parametrically plot two linearly dependent functions in the \( xy \) plane, one would get a line through the origin of slope \( c\). As Peano stated, his example instead gives the positive \( x\) and positive \( y\) axes when plotted parametrically, as we see here.

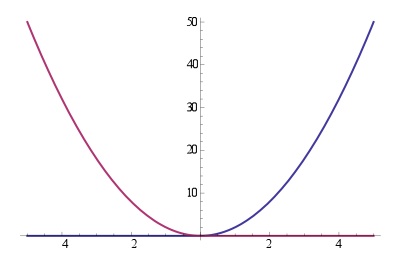
The plots of \( x\) and \( y\) as functions of \( t\).

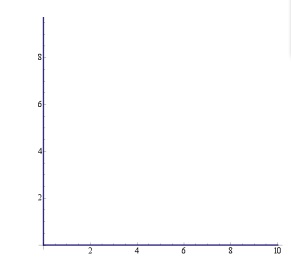
Parametric plot of \( x\) and \( y\).

We see that Peano provided a piecewise-defined formula rather than a single formula for a function \( \phi(t)\) that satisfies the conditions he proposed. Evidently, Mansion must have noted this as he added the following footnote to the above passage, which clearly references a conversation with Peano on the subject:

(*) For example: \[ \phi (t) = \frac{2}{\pi} {\int_0^\infty} \frac{\sin tx}{x}\,dx \] says Mr. Peano. If one wants a more elementary example, we offer \[ \psi (t) = E\left({\frac{1}{1+t^2}}\right),\quad\quad \phi (t) = [1 - \psi (t)]\,{\frac{t+\psi(t)}{{\rm mod.}t+\psi(t)}},\] where \( E(u)\) designates the largest integer less than \( u,\) \( {\rm mod.}t,\) the absolute value of \( t\). (P.M.).

Peano then concluded his note by considering conditions that would make the originally stated proposition true (Passage 1). He gave one such condition, and mused that it would be nice to find others:

The proposal is true if one supposes that there exists no value of \( t\) which cancels all the minors of the last line of the proposed determinant, and perhaps in other cases which would be interesting to investigate.

The phrasing of Peano’s proposed condition is somewhat confusing due to the use of the word “line” (linge). It is unclear whether he was referring to the last row or to the last column.

In 1900, Maxime Bocher indicated that Peano was referring to columns. Bocher added a note to the end of a short article in which he explained his interpretation of Peano’s condition ([B1, p. 121]).

Image used with permission from the American Mathematical Society.

A year later, Bocher changed his mind and stated that Peano was referring to the \( (n-1)\times (n-1) \) matrices formed by eliminating the last row and a column of the Wronskian. Notice that these minor determinants are actually just the Wronskians of the functions taken \( (n-1)\) at a time, which might provide a basis for induction. He called this Peano’s Second Theorem ([B3, p. 146]).

Passage 3. Image used with permission from the American Mathematical Society.

Indeed, mathematicians such as Vivanti ([V]), Bocher ([B1], [B2], [B3], [B4]), and Curtiss ([C]) later offered additional conditions that make the proposition true. The most famous theorem is attributed to Bocher, and states that if the Wronskian of \( n\) analytic functions is zero, then the functions are linearly dependent ([B2], [BD]). 

In response to Peano’s request for other interesting conditions that would make the proposal true, Paul Mansion added a second footnote to [P1]:

(*) One can correct the wording of the expressed theorem at the beginning of this note, as we have indicated in our Résume d’analyse, by adding the words: or one of the functions is identically zero. (P.M.).

Passage 4

The passage to which he was referring is from his textbook Résumé du Cours d’Analyse Infinitésimale de l’Université de Gand ([PM]). It is unclear how such an addition would fix the proposition, as neither of Peano’s example functions is identically zero, though if we consider only positive or negative \( t\) values, one of the functions will be identically zero. To see exactly what Mansion means, we turn to the relevant section of his textbook.

The original French passage of Résumé du Cours d’Analyse Infinitésimale de l’Université de Gand (Survey of Infinitesimal Analysis, Ghent University) can be found at [PM], while our English translation can be found in Appendix 2. Mansion began his passage by stating:

III. A Wronskian \( W(r,s,t,u) \) is identically zero if one of the functions \( r,s,t,u \) is identically zero, or there exists between them a homogeneous linear relationship; and VICE VERSA.

This statement is only partially valid, since Peano’s counterexample disproves this statement as well (Passage 2). We have already noted that the Wronskian of a set of functions will be zero if the functions are linearly dependent, and Mansion gave the same argument we provided earlier.

If \( r, \) for example, is identically zero, the same is true of the derivatives \( {r^{\prime}}, {r^{\prime\prime}}, {r^{\prime\prime\prime}}, \) and \( W, \) having a column of zeros, is also identically zero. If one has \( u=ar+bs, \) and as a result, \( {{u^{\prime}}=a{r^{\prime}}+b{s^{\prime}}},\) \({{u^{\prime\prime}}=a{r^{\prime\prime}}+b{s^{\prime\prime}}},\) \({{u^{\prime\prime\prime}}=a{r^{\prime\prime\prime}}+b{s^{\prime\prime\prime}}}, \) \( W\) will have a column of zeros, when one takes out the fourth column, the first multiplied by \( a \) and the second multiplied by \( b\).

The problem, then, must be in the proof of the “vice versa.” Mansion proceeded by induction, and while the induction step is (essentially) valid, there is a problem with the base case. 

SIMILARLY, if \( W\) is identically zero, one of the functions \( r,s,t,u \) is equal to zero, or there exists between \( r,s,t,u \) a linear relationship. We suppose first this reciprocal is established for Wronskians of three lines and we prove that it is true for Wronskians of four lines. Thus, define \( k,m,n,p, \) the minors of \( W\) with regard to \( {r^{\prime\prime\prime}},{s^{\prime\prime\prime}},{t^{\prime\prime\prime}},{u^{\prime\prime\prime}}. \) These minors are themselves Wronskians of three lines. If one of them is identically zero, there exists a linear relationship between the functions involved (according to the hypothesis made for Wronskians of three lines), and the theorem is demonstrated.

The first mistake in this passage is simply assuming that the theorem is true for a \( 3\times 3\) Wronskian. While we know the theorem is false for two functions, technically Peano did not give a counterexample for three. However, the theorem is indeed false for three functions as well, as Bocher showed in [B3]. Despite the problem with the base case, we will reproduce the rest of Mansion’s argument, filling in details for clarity.

If none of the minors \( k,m,n,p \) is equal to zero, we consider the identical relations \[ kr+ms+nt+pu=0,\quad \left( {{\mathcal{Z}}_1}\right) \] \[ k{r^{\prime}}+m{s^{\prime}}+n{t^{\prime}}+p{u^{\prime}}=0, \quad \left({{\mathcal{Z}}_2}\right) \] \[ k{r^{\prime\prime}}+m{s^{\prime\prime}}+n{t^{\prime\prime}}+p{u^{\prime\prime}}=0, \quad\left({{\mathcal{Z}}_3}\right) \] \[ k{r^{\prime\prime\prime}}+m{s^{\prime\prime\prime}}+n{t^{\prime\prime\prime}}+p{u^{\prime\prime\prime}}=0, \quad\left({{\mathcal{Z}}_4}\right) \] obtained in expressing the properties of the minors of the zero determinant \( W\). Because \( {D_z}W=0,\) one still has, according to formula (2), \[ k{r^{iv}}+m{s^{iv}}+n{t^{iv}}+p{u^{iv}}=0, \quad \left({{\mathcal{Z}}_5}\right) \]

These relationships can be derived by taking the determinants of the matrices: \[ \left[ {\begin{array}{*{20}c} r & s & t & u \\ r & s & t & u \\ {r'} & {s'} & {t'} & {u'} \\ {r''} & {s''} & {t''} & {u''} \\ \end{array}} \right]\quad \left[ {\begin{array}{*{20}c} {r'} & {s'} & {t'} & {u'} \\ r & s & t & u \\ {r'} & {s'} & {t'} & {u'} \\ {r''} & {s''} & {t''} & {u''} \\ \end{array}} \right]\quad \left[ {\begin{array}{*{20}c} {r''} & {s''} & {t''} & {u''} \\ r & s & t & u \\ {r'} & {s'} & {t'} & {u'} \\ {r''} & {s''} & {t''} & {u''} \\ \end{array}} \right]\quad \left[ {\begin{array}{*{20}c} {r'''} & {s'''} & {t'''} & {u'''} \\ r & s & t & u \\ {r'} & {s'} & {t'} & {u'} \\ {r''} & {s''} & {t''} & {u''} \\ \end{array}} \right] \]

Each of these matrices has a zero determinant, and expanding along the top rows gives the equations \( \left( {{\mathcal{Z}}_1}\right), \left( {{\mathcal{Z}}_2}\right), \left( {{\mathcal{Z}}_3}\right), \) and \( \left( {{\mathcal{Z}}_4}\right), \) except for having alternating signs. It appears this was an error in the original printing, because the argument will work if the signs are treated carefully. The next step is to differentiate each equation \( \left( {{\mathcal{Z}}_1}\right), \left( {{\mathcal{Z}}_2}\right), \left( {{\mathcal{Z}}_3}\right), \) and \( \left( {{\mathcal{Z}}_4}\right), \) making use of the product rule and simplifying at each step. Again, the equations should have alternating signs.

Differentiating successively \( \left( {{\mathcal{Z}}_1}\right), \left( {{\mathcal{Z}}_2}\right), \left( {{\mathcal{Z}}_3}\right), \left( {{\mathcal{Z}}_4}\right), \) we simplify the derivative of each of these equations, by means of the following, \[ {k^{\prime}}r+ {m^{\prime}}s+ {n^{\prime}}t+ {p^{\prime}}u=0, \] \[ {k^{\prime}}{r^{\prime}}+{m^{\prime}}{s^{\prime}}+{n^{\prime}}{t^{\prime}}+{p^{\prime}}{u^{\prime}}=0, \] \[ {k^{\prime}}{r^{\prime\prime}}+{m^{\prime}}{s^{\prime\prime}}+{n^{\prime}}{t^{\prime\prime}}+{p^{\prime}}{u^{\prime\prime}}=0, \] \[ {k^{\prime}}{r^{\prime\prime\prime}}+{m^{\prime}}{s^{\prime\prime\prime}}+{n^{\prime}}{t^{\prime\prime\prime}}+{p^{\prime}}{u^{\prime\prime\prime}}=0. \]

Notice that this is simply a system of linear equations in the variables \( {k^{\prime}},{m^{\prime}},{n^{\prime}}, \) and \( {p^{\prime}}. \) Mansion solved for these variables. One way of doing so would be to rewrite the corrected system as

\[ {k^{\prime}}r- {m^{\prime}}s+ {n^{\prime}}t= {p^{\prime}}u, \] \[ {k^{\prime}}{r^{\prime}}-{m^{\prime}}{s^{\prime}}+{n^{\prime}}{t^{\prime}}={p^{\prime}}{u^{\prime}}, \] \[ {k^{\prime}}{r^{\prime\prime}}-{m^{\prime}}{s^{\prime\prime}}+{n^{\prime}}{t^{\prime\prime}}={p^{\prime}}{u^{\prime\prime}}, \] \[ {k^{\prime}}{r^{\prime\prime\prime}}-{m^{\prime}}{s^{\prime\prime\prime}}+{n^{\prime}}{t^{\prime\prime\prime}}={p^{\prime}}{u^{\prime\prime\prime}}. \] and then apply Cramer’s rule to see that: \[ k' = \frac{{\left| {\begin{array}{*{20}c} {p'u} & { - s} & t \\ {p'u'} & { - s'} & {t'} \\ {p'u''} & { - s''} & {t''} \\ \end{array}} \right|}}{{\left| {\begin{array}{*{20}c} r & { - s} & t \\ {r'} & { - s'} & {t'} \\ {r''} & { - s''} & {t''} \\ \end{array}} \right|}} = \frac{{p'\left| {\begin{array}{*{20}c} s & t & u \\ {s'} & {t'} & {u'} \\ {s''} & {t''} & {u''} \\ \end{array}} \right|}}{{\left| {\begin{array}{*{20}c} r & s & t \\ {r'} & {s'} & {t'} \\ {r''} & {s''} & {t''} \\ \end{array}} \right|}} = p'\frac{k}{p} \]

This yields the formulas given by Mansion in the following passage. These relationships are all derivatives of the logarithms of the functions, and so their integrals will all differ by a constant. This step will require that not only are \( k \) and \( p \) not zero, but neither are their derivatives. Integrating and keeping track of the constants will give a relationship between \( r,s,t,\) and \( u. \)

These four relationships give immediately, according to the properties of homogeneous linear equations and the definition of \( k,m,n,p ,\)

\[ {\frac{k^\prime}{k} = \frac{m^\prime}{m} =\frac{n^\prime}{n} =\frac{p^\prime}{p} },\quad {\rm{or}}\quad {Dlk=Dlm=Dln=Dlp}. \]

As a result, according to numbers \( 221\) or \( 103\), \( \alpha, \beta, \gamma \) being the constants, \[ lm=lk+l\alpha,\quad ln=lk+l\beta,\quad lp=lk+l\gamma, \]

\[ m={\alpha}k,\quad n={\beta}k,\quad p={\gamma}k. \]

Substituting these values of \( m, n, p\) into the identity \( kr+ms+nt+pu=0,\) it becomes, after division by \( k,\) \( r + {\alpha}s + {\beta}t + {\gamma}u = 0, \) a relationship which had to be demonstrated.

IV. REMARK. One can evidently establish analogous theorems to the previous ones on the Wronskians where the derivatives are replaced by partial derivatives or total differentials of the functions in question.

The addition of such a confusing footnote (Passage 4) certainly obscured the main point of Peano’s first article. He responded with a letter, part of which was published under the name Sur les Wronskiens in the same issue of Mathesis as the original note. The letter consists of three sections: in the first part, Peano argued that Mansion’s suggested language does not lead to a true statement; next, he presented another counterexample to the contested proposition which avoids the issue that at every point of the domain, at least one of the functions will evaluate to zero; finally, he referred to the above “proof” of Mansion’s proposition, and stated the most general theorem that can actually be proved using Mansion’s reasoning.

6. On the Wronskians (Excerpt from a letter by M.G. Peano). The note added to the end of my brief article on the Wronskians (Mathesis, p. 75-76), if it contributes to the clarity, it adds nothing to the rigor of the contested proposition; and I must return to the subject. I. The proposition I contest is the following: If the determinant formed with \( n\) functions \( x_1,\dots, x_n \) of the same variable \(t,\) and their derivatives of orders \( 1,\dots, (n-1) \) is zero for all values of \(t,\) there is between these functions a homogeneous linear relationship with constant coefficients; in other words, one can determine \(n\) constants, \( C_1,\dots, C_n ,\) all of which cannot equal zero at the same time, such that \( C_1 x_1+\cdots + C_n x_n =0 \) for all values of \(t.\)

He then particularly attacked the footnote (Passage 4) appended by Mansion to his previous article. He showed that adding the words “one of the functions is identically zero” (he quoted Mansion’s footnote) does not change the validity of the proposition at all. Lest readers would be confused by the way he phrased the proposition, Peano restated the (false) theorem in multiple ways, including a geometric argument similar to what he used to show that two functions were linearly independent.

If one of the functions, for example \( x_1,\) is identically zero, these functions are connected by the linear relation \( x_1+0x_2 +\cdots +0x_n =0.\) Thus the conclusion of the proposition in question is not at all modified if one adds the words: or one of the functions is identically zero. This proposition may again be expressed in this way: If the Wronskian of the functions \( x_1,\dots, x_n \) is identically zero, the determinant formed with the values of the functions, when one gives the variable \( n\) ordinary values, is also zero. A particular case of the general theorem is as follows: If the determinant formed with the derivatives of orders \( 1, 2, 3 \) of the functions \( x, y, z \) of the same variable \( t\) is identically zero, the curve described by the coordinate point \( x, y, z \) is planar.

Peano then reiterated his previous example (Passage 1), and explained that since neither of the two functions is identically zero, Mansion’s addition does not apply to his two functions, and hence his example still shows that the proposition in question is not true.

However, Peano did note that while neither of his functions is “identically zero,” for every value of \( t,\) at least one of them is.  Realizing that this might be confusing to the reader, he gave a second example, which is merely half the sum and difference of his original.  These no longer have the odd property that one of them is zero at any \( t\) value, yet they still satisfy that the Wronskian is always zero. Recall that \( \, {\rm mod}\, t\) denotes the absolute value of \( t.\)

II. To demonstrate that these propositions are not always true, I have indicated in my previous note, an example in which I consider only two functions \( x\) and \( y\) of the variable \( t;\) the determinant \( x{y^\prime} – {x^\prime} y\) is identically zero; but between them there exists no linear relation. Neither one nor the other of the two functions is identically zero, because \( x>0\) if \( t>0,\) and \( y>0\) if \( t<0.\) But these two functions present this unusual characteristic that, for all values of \( t,\) one or the other is zero. To make this anomaly disappear, we propose \[ X={t^2},\quad Y=t{\, {\rm mod}\, t}.\] These two functions of \( t\) satisfy the condition \( X{Y^\prime}-{X^\prime}Y=0 ;\) they only cancel for \( t=0,\) and between them there is no homogeneous linear relation. The sum and the difference of these functions are the functions of my first example; the coordinate point \( (X,Y) \) now describes the two half-bisectors of the axes.

Passage 5

In the third part of this note, Peano explained that the “proofs” of all the false propositions make the assumption that the Wronskians of the functions taken \( n-1\) at a time are either all zero or never zero, referring explicitly to Mansion’s work as an example of one of these incorrect proofs.  He again suggests that readers start looking for additional hypotheses that make the proposition true.

III. The given demonstrations of the proposition, including yours, only allow us to prove that it is true if one of the minor determinants of the last line is always equal to zero, or if it is never equal to zero. For determinants of the second order, one can demonstrate the proposition, except where, for any value of the independent variable, the two given functions and their derivatives each cancel at the same time. It would be interesting to identify all the cases in which the proposition holds true.

Peano finally noted that he had never seen this fact accurately conveyed, and gave several additional citations. We think he was being very generous, because when one reads these three passages, one sees that the issue is not whether the proposition is “clearly expressed,” but rather that it is clearly incorrect!

I believed it necessary to publish this brief note on the Wronskians, because I have never seen the proposition clearly expressed. (See Hermite, Cours d’Analyse, p. 133; Jordan, Cours d’Analyse, III, p. 150; Laurent, Traité d’Analyse, I, p. 183.)

We turn our attention to these three textbooks now.

Hermite's Cours d’analyse de l’école polytechnique

Peano first referenced Charles Hermite’s 1873 textbook, Cours d’analyse de l’école polytechnique ([H]). Hermite actually was “proving” a theorem we saw stated in Sur les Wronskiens by Peano, namely:

A particular case of the general theorem is as follows: If the determinant formed with the derivatives of orders \( 1, 2, 3 \) of the functions \( x, y, z \) of the same variable \( t\) is identically zero, the curve described by the coordinate point \( x, y, z \) is planar.

Here is Hermite’s simpler version when \( z=t.\)  

We suppose in fact, for greater simplicity, \( \theta(t) = t,\) so that, \( z\) becoming the independent variable, the equations of the curve are \[ x=\phi (z),\quad y=\psi (z).\] We will find then \[ \Delta = {{\left| {\begin{array}{*{20}c} {{\phi}'} & {{\phi}''} & {{\phi}'''}   \\ {{\psi}'} & {{\psi}''} & {{\psi}'''}  \\ 1 & 0 & 0  \\ \end{array}} \right|}} = {{\phi}''}{{\psi}'''}-{{\phi}'''}{{\psi}''} \] and the condition \( \Delta =0,\)  in dividing by \( {{{\psi}''}^2},\) takes this form \[ {\left({\frac{{\phi}''}{{\psi}''}}\right)}' =0,\] where one concludes \[ {{\phi}''}=a{{\psi}''},\] \( a\) designating an arbitrary constant. It results very easily \[ {{\phi}'}=a{{\psi}'} + b,\] then finally \[ \phi = a\psi + bz + c,\] that is (to say), \[ x = ay + bz + c,\] so that the proposed curve is in fact completely contained in the same plane.

We see Hermite divided by \( {{{\psi}''}^2}\) in the proof, and so there is an implicit requirement that \( {{{\psi}''}^2}\) cannot be zero. For two functions, this is actually an important condition, as noted by Bocher in [B3, p. 140]:

Passage 6. Image used with permission from the American Mathematical Society.

Notice how this would eliminate Peano’s counterexamples (Passage 2 and Passage 5) if the interval is the real number line, since both Sur le déterminant Wronskien and Sur les Wronskiens have examples where each function has a root at the origin. In [B3], Bocher gave an example of three functions that are linearly independent and have a zero Wronskian, yet no function attains the value of zero on the interval in question.

Jordan's Cours d’analyse de l’école polytechnique

The relevant passage from Camille Jordan’s textbook occurs on page 149 of [J], and a direct translation with no analysis can be found in Appendix 2. He began by stating that the Wronskian not being identically zero is a necessary and sufficient condition for functions \( x_1,\dots, x_n \) to be linearly independent.

122. One can note that the condition \[ \left| {\begin{array}{*{20}c} {x_1 } & {} &  \cdots  & {} & {x_n }  \\ \vdots  & {} &  \ddots  & {} &  \vdots   \\ {x_1^{n - 1} } & {} &  \cdots  & {} & {x_n^{n - 1} }  \\ \end{array}} \right| \ne 0 \] expresses the necessary and sufficient condition for there to exist between the functions \( x_1,\dots, x_n \) no linear relationship with constant coefficients, such that \[ {\alpha}_1 x_1+\cdots + {\alpha}_n x_n =0.\]

He started with the standard—and valid—argument that we have given earlier, showing that if the functions are linearly dependent, then the Wronskian will be zero.

In fact, if there existed a relationship of this type, one would obtain, in differentiating it, \[ {\alpha}_1 {x_1^\prime} +\cdots + {\alpha}_n {x_n^\prime} =0,\] \[ \vdots \] \[ {\alpha}_1 {x_1^{n-1}} +\cdots + {\alpha}_n {x_n^{n-1}} =0,\] and, in eliminating the parameters \( {\alpha}_1 ,\dots , {\alpha}_n ,\) it would become \[ \left| {\begin{array}{*{20}c} {x_1 } & {} &  \cdots  & {} & {x_n }  \\ {x_1^\prime} & {} &  \cdots  & {} & {x_n^\prime}  \\ \vdots  & {} &  \cdots  & {} &  \vdots   \\ {x_1^{n - 1} } & {} &  \cdots  & {} & {x_n^{n - 1} }  \\ \end{array}} \right| = 0. \]

The other implication is the one in which we are interested. Jordan replaced the first column of the Wronskian with the differentials \( X, X^{\prime}, X^{\prime\prime},\dots , X^{n-1}.\) Then expanding the determinant of this new matrix along the first column and setting it equal to zero defines an \( (n‐1)^{\rm th} \) order linear differential equation \[ p_{n-1} (x) X^{(n-1)} + p_{n-2} (x) X^{(n-2)} + \cdots + p_{1} (x) X^{\prime} + p_{0} (x) X =0, \] where \( {p_k}(x)\) is the cofactor formed by eliminating the first column and the \( (k+1)^{\rm th} \) row.

All the functions \( x_1, x_2,\dots, x_n \) are solutions to this differential equation. If we substitute \( x_1\) into the differential equation, it vanishes by the hypothesis of the Wronskian vanishing. If we substitute any other \( x_2,\dots, x_n, \) the matrix will have two identical columns, which would have a zero determinant.

Jordan then noticed this gives \( n \) solutions to an \( (n‐1)^{\rm th} \) order linear differential equation. By the standard uniqueness theorems, a fundamental set of solutions should have only \( n‐1 \) functions, and he proceeded to argue there must be a relationship between \( x_1, x_2,\dots, x_n .\)

Similarly, if this determinant is zero, \( x_1,\dots, x_n \) will be \( n \) particular solutions of the linear equation of order \( n-1 \) \[ \left| {\begin{array}{*{20}c} {X} & {x_2} &  \cdots  & {x_n }  \\ {X^\prime} & {x_2^{\prime}} &  \cdots  & {x_n^\prime}  \\ \vdots  & {\vdots} &  \cdots  &  \vdots   \\ {X^{n - 1} } & {x_2^{n-1}} &  \cdots  & {x_n^{n - 1} }  \\ \end{array}} \right| = 0. \] One will have therefore, in designating by \( X_1,\dots, X_n \) the independent solutions of this equation, and by \( C_1^{\prime}, \dots , C_{n-1}^n \) the constants, \[ x_1 ={C_1^\prime}X_1 +\cdots + {C_{n-1}^{\prime}}X_{n-1} ,\] \[ \vdots \] \[ x_n ={C_1^{n}}X_1 +\cdots + {C_{n-1}^{n}}X_{n-1}.\] Eliminating \( X_1,\dots, X_n \) between these equations, a linear relationship between \( x_1,\dots, x_n \) will be deduced.

In order to apply the uniqueness theorem for linear differential equations, one needs to require that all the \( p_{k} (x) \) are continuous and that \( p_{n-1} (x) \) has no roots on \( I. \) This condition is exactly what fails in the case of Peano’s examples. This is most easily seen in his second example from Passage 5. Both \( u_{1} = | x| \,x \) and \( u_{2} =x^2 \) are solutions to the first order linear differential equation \[ -{x^2}{y^\prime} + 2xy =0 \] and satisfy the initial condition \( y(1)=1 \).  But we cannot conclude that \( u_{1} =c u_2 \) on the real number line because of the fact that \( p_{n-1} (x) \) (which in this case is \( u_{1} \)) has a root at zero. Notice that in fact \( u_{1} =c u_2 \) on any interval that does not contain the origin.

Jordan’s argument becomes correct if we require \( p_{n-1} (x)\,{\not=}\, 0 \) and all the \( p_{k} (x) \) to be continuous. In the case of two functions, these additional requirements are equivalent to the ones outlined by Bocher in Passage 6 above.  The case of more functions is similar to Peano’s Second Theorem (Passage 3) because \( p_{n-1} (x) \) is the Wronskian of \( u_{2} (x) ,\dots , u_n (x). \)

Laurent's Traité d’Analyse

Peano referenced the textbook Traité d’analyse written by Hermann Laurent in 1885 ([L]). The section with which Peano was concerned turns out to be a brief note of clarification added to the end of the chapter on determinants and implicit functions. Laurent wrote:

4. If one has $$ \left| {\begin{array}{*{20}c} {x_1} & {x_2} &\cdots  & {x_n }\\ {dx_1} & {dx_2} &\cdots& {dx_n}\\ \vdots& {\vdots} &\cdots  &\vdots\\ {d^{n - 1}x_1 } & {d^{n-1}x_2} &\cdots& {d^{n - 1} x_n}\\ \end{array}} \right| = 0, $$ one has necessarily, between \( x_1, x_2,\dots, x_n ,\) a relation in the form of \[ a_1 x_1 + a_2 x_2 +\cdots + a_n x_n =0, \] \( a_1, a_2,\dots, a_n \) designating the constants.

There is little to say about this statement except that it is incorrect to assume a zero Wronskian indicates dependence between functions, and that this idea is exactly what Peano disproved in his two notes.

The use of primary sources in the classroom can be a powerful teaching technique in any course. It can provide context to concepts that are being taught and also improve the retention of the material.

The connection between the Wronskian and fundamental solutions is ubiquitous in any ordinary differential equations class, but it is a struggle for students to understand the subtlety of that connection. Placing it in a historical context can help students understand the concepts. In particular, they can see that this connection was not obvious to many established mathematicians of the time, perhaps helping to alleviate concerns they may have about not easily understanding certain mathematical ideas. Additionally, students can see how before the advent of the Internet, results were sometimes submitted without knowledge of similar publications. And finally, they can see the “human” nature of the exchange between Mansion and Peano.

It is our hope that readers find this a valuable resource in their classes.

There are many excellent opportunities for additional questions or projects for students from these translations. Here are just a few that we think work particularly well. They are roughly ordered from simple exercises to more advanced projects.

1)   Consider the functions \( x \) and \( y\) described in Sur le déterminant Wronskien.

(a) Plot the functions \( x \) and \( y\) on \( (x,t) \) and \( (y,t) \) axes and give a geometric argument why the two functions are indeed linearly independent.

(b) Parametrically plot \( x \) and \( y, \) and explain why this means that \( x \) and \( y\)  are linearly independent.

(c) Calculate the Wronskian for these two functions, and show that you always get a determinant of \( 0. \) It may be easier to consider separately the cases when \( t \) is negative, positive, and \( 0. \)

2)   Consider the functions \( x \) and \( y\) described in Sur les Wronskiens.

(a) Plot the functions \( x \) and \( y\) on \( (x,t) \) and \( (y,t) \) axes and give a geometric argument why the two functions are indeed linearly independent.

(b) Parametrically plot \( x \) and \( y, \) and explain why this means that \( x \) and \( y\) are linearly independent. Show that you indeed always get a zero Wronskian.

3)    Prove Peano’s Second Theorem in the case when \( n=2. \)

4)    Building from Peano’s examples, construct an example of three functions that are not linearly dependent, but have a zero Wronskian.

5)    In [B1, p. 120], Bocher gave the following example of two functions that are linearly independent yet have a zero Wronskian. Prove this is true.

Image used with permission from the American Mathematical Society.

6)    In [B3, p. 143], Bocher gave the following example of three functions that are linearly independent yet have a zero Wronskian. Prove this is true.

Image used with permission from the American Mathematical Society.

7)    In [C, p. 292], Curtiss gave the following example of four functions that have a zero Wronskian on any interval containing the origin and are linearly dependent, but for which none of the Wronskians of three functions vanish simultaneously at a point. Prove this is true.

Image used with permission of Göttingen State and University Library.

8)    Show that the elementary function \[ \phi (t) = [1 - \psi (t)]\,{\frac{t+\psi(t)}{{\rm mod.}t+\psi(t)}},\] given by Mansion in his first footnote (see page 2; \( \psi \) is defined there), does indeed have the properties of the function \( \phi(t) \) described by Peano in Passage 2.

9)    Show that the function \[ \phi (t) = \frac{2}{\pi} {\int_0^\infty} \frac{\sin tx}{x}\,dx \] has the properties of the original function \( \phi(t) \) described by Peano in Passage 2.

10)  Provide the translation of Mansion’s proof to students.

(a) Ask students to reconstruct the argument and find the problems in Mansion's proof.

(b) In particular, assuming that the induction hypothesis is correct, correct the alternating sign typos in Mansion’s proof.

11)  Provide the translation of Jordan’s proof to students and ask them to reconstruct the argument and find the problems in the proof.

12)  Read the papers of Bocher and others and write a report on the various hypotheses that fix the proposition that zero Wronskian implies linear dependence.

Sur le déterminant Wronskien, by Giuseppe Peano

1. On the Wronskian determinant (by G. Peano, professor at the University of Turin). In nearly all papers, one finds the proposition: If the determinant formed with n functions of the same variable and their derivatives of orders \(1,\dots,(n-1)\) is identically zero, there is between these functions a homogeneous linear relationship with constant coefficients.    

This wording is too general. We offer, in fact, \[ x = t^2 [1+\phi(t)],\quad\quad y = t^2 [1-\phi(t)],\] where \( \phi(t)\) designates a function equal to zero for \( t = 0\), equal to \( 1\) for positive \( t \), to \( -1\) for negative \( t \) (*). One has for \[ t\le 0,\quad \phi(t)=-1,\quad x=0,\quad {y=2t^2};\] for \[ t= 0,\quad \phi(t)=0,\quad x=0,\quad {y=0};\] for \[ t\ge 0,\quad \phi(t)=1,\quad {x=2t^2},\quad {y=0}.\]

(*) For example:  \[ \phi (t) = \frac{2}{\pi} {\int_0^\infty} \frac{\sin tx}{x}\,dx \] says Mr. Peano. If one wants a more elementary example, we offer \[  \psi (t) = E\left({\frac{1}{1+t^2}}\right),\quad\quad \phi (t) = [1 - \psi (t)]\,{\frac{t+\psi(t)}{{\rm mod.}t+\psi(t)}},\] where \( E(u)\) designates the largest integer less than \( u,\,{\rm mod.}\, t,\) the absolute value of \( t\). (P.M.).

The functions \( x\) and \( y,\) and their derivatives \[ {x^\prime} = 2t [1+\phi(t)],\quad\quad {y^\prime} = 2t [1-\phi(t)],\] are continuous, and one has, for all values of \( t ,\) \[ \left| \begin{array}{cc} x&y\\x^\prime&y^\prime\\ \end{array} \right| =0 \] But between \( x\) and \( y,\) there is no relation of the expressed form, because the coordinate point \(  (x, y) \)  does not describe a straight line passing through the origin, but the two half-axes \( ox\) and \( oy.\)

The proposal is true if one supposes that there exists no value of \( t\) which cancels all the minors of the last line of the proposed determinant, and perhaps in other cases which would be interesting to investigate.

(*) One can correct the wording of the expressed theorem at the beginning of this note, as we have indicated in our Résume d’analyse, by adding the words: or one of the functions is identically zero. (P.M.).

Sur les Wronskiens, by Giuseppe Peano

6.  On the Wronskians (Excerpt from a letter by M.G. Peano). The note added to the end of my brief article on the Wronskians (Mathesis, p. 75-76), if it contributes to the clarity, it adds nothing to the rigor of the contested proposition; and I must return to the subject.

I. The proposition I contest is the following: If the determinant formed with \(n\) functions \( x_1,\dots, x_n \) of the same variable \(t,\) and their derivatives of orders \( 1,\dots, (n-1), \) is zero for all values of \(t,\)  there is between these functions a homogeneous linear relationship with constant coefficients; in other words, one can determine \(n\) constants, \( C_1,\dots, C_n ,\) all of which cannot equal zero at the same time, such that \( C_1 x_1+\cdots + C_n x_n =0 \) for all values of \(t.\)

If one of the functions, for example \( x_1,\) is identically zero, these functions are connected by the linear relation \( x_1+0x_2 +\cdots +0x_n =0.\) Thus the conclusion of the proposition in question is not at all modified if one adds the words: or one of the functions is identically zero. 

This proposition may again be expressed in this way: If the Wronskian of the functions \( x_1,\dots, x_n \) is identically zero, the determinant formed with the values of the functions, when one gives the variable \( n\) ordinary values, is also zero.

A particular case of the general theorem is as follows: If the determinant formed with the derivatives of orders \( 1, 2, 3 \) of the functions \( x, y, z \) of the same variable \( t\) is identically zero, the curve described by the coordinate point \( x, y, z \) is planar.

II. To demonstrate that these propositions are not always true, I have indicated in my previous note, an example in which I consider only two functions \( x\) and \( y\) of the variable \( t;\) the determinant \( x{y^\prime} – {x^\prime} y\) is identically zero; but between them there exists no linear relation. Neither one nor the other of the two functions is identically zero, because \( x>0\) if \( t>0,\) and \( y>0\) if \( t<0.\)

But these two functions present this unusual characteristic that, for all values of \( t,\) one or the other is zero. To make this anomaly disappear, we propose \[ X={t^2},\quad Y=t\,{{\rm mod}\,t}.\] These two functions of \( t\) satisfy the condition; they only cancel for \( t=0,\) and between them there is no homogeneous linear relation. The sum and the difference of these functions are the functions of my first example; the coordinate point \( (X,Y) \) now describes the two half-bisectors of the axes.

III. The given demonstrations of the proposition, including yours, only allow us to prove that it is true if one of the minor determinants of the last line is always equal to zero, or if it is never equal to zero.

For determinants of the second order, one can demonstrate the proposition, except where, for any value of the independent variable, the two given functions and their derivatives each cancel at the same time. 

It would be interesting to identify all the cases in which the proposition holds true.

I believed it necessary to publish this brief note on the Wronskians, because I have never seen the proposition clearly expressed. (See Hermite, Cours d’Analyse, p. 133; Jordan, Cours d’Analyse, III, p. 150; Laurent, Traité d’Analyse, I, p. 183.)

From Résumé du Cours d’Analyse Infinitésimale de l’Université de Gand (Survey of Infinitesimal Analysis, Ghent University), by Paul Mansion

III. A Wronskian \( W(r,s,t,u) \) is identically zero if one of the functions \( r,s,t,u \)  is identically zero, or there exists between them a homogeneous linear relationship; and VICE VERSA.

If \( r, \) for example, is identically zero, the same is true of the derivatives \( {r^{\prime}}, {r^{\prime\prime}}, {r^{\prime\prime\prime}}, \) and \( W, \) having a column of zeros, is also identically zero. If one has \( u=ar+bs, \) and as a result, \( {{u^{\prime}}=a{r^{\prime}}+b{s^{\prime}}}, {{u^{\prime\prime}}=a{r^{\prime\prime}}+b{s^{\prime\prime}}}, {{u^{\prime\prime\prime}}=a{r^{\prime\prime\prime}}+b{s^{\prime\prime\prime}}}, \) \( W\) will have a column of zeros, when one takes out the fourth column, the first multiplied by  \( a \) and the second multiplied by \( b\).  

SIMILARLY, if \( W\) is identically zero, one of the functions \( r,s,t,u \) is equal to zero, or there exists between \( r,s,t,u \) a linear relationship. We suppose first this reciprocal is established for Wronskians of three lines and we prove that it is true for Wronskians of four lines. Thus, define \( k,m,n,p, \) the minors of \( W\) with regard to \( {r^{\prime\prime\prime}},{s^{\prime\prime\prime}},{t^{\prime\prime\prime}},{u^{\prime\prime\prime}}. \) These minors are themselves Wronskians of three lines. If one of them is identically zero, there exists a linear relationship between the functions involved (according to the hypothesis made for Wronskians of three lines), and the theorem is demonstrated.

If none of the minors \( k,m,n,p \) is equal to zero, we consider the identical relations  \[ kr+ms+nt+pu=0,\quad \left({{\mathcal{Z}}_1}\right) \] \[ k{r^{\prime}}+m{s^{\prime}}+n{t^{\prime}}+p{u^{\prime}}=0, \quad \left({{\mathcal{Z}}_2}\right) \] \[ k{r^{\prime\prime}}+m{s^{\prime\prime}}+n{t^{\prime\prime}}+p{u^{\prime\prime}}=0, \quad\left({{\mathcal{Z}}_3}\right) \] \[ k{r^{\prime\prime\prime}}+m{s^{\prime\prime\prime}}+n{t^{\prime\prime\prime}}+p{u^{\prime\prime\prime}}=0, \quad\left({{\mathcal{Z}}_4}\right) \] obtained in expressing the properties of the minors of the zero determinant \( W\). Because \( {D_z}W=0,\) one still has, according to formula (2), \[ k{r^{iv}}+m{s^{iv}}+n{t^{iv}}+p{u^{iv}}=0, \quad \left({{\mathcal{Z}}_5}\right) \]

Differentiating successively \( {{ \mathcal{Z} }_1}, {{\mathcal{Z}}_2}, {{\mathcal{Z}}_3}, {{\mathcal{Z}}_4},  \) we simplify the derivative of each of these equations, by means of the following, \[ {k^{\prime}}r+ {m^{\prime}}s+ {n^{\prime}}t+ {p^{\prime}}u=0, \] \[ {k^{\prime}}{r^{\prime}}+{m^{\prime}}{s^{\prime}}+{n^{\prime}}{t^{\prime}}+{p^{\prime}}{u^{\prime}}=0, \] \[ {k^{\prime}}{r^{\prime\prime}}+{m^{\prime}}{s^{\prime\prime}}+{n^{\prime}}{t^{\prime\prime}}+{p^{\prime}}{u^{\prime\prime}}=0, \] \[ {k^{\prime}}{r^{\prime\prime\prime}}+{m^{\prime}}{s^{\prime\prime\prime}}+{n^{\prime}}{t^{\prime\prime\prime}}+{p^{\prime}}{u^{\prime\prime\prime}}=0. \]

These four relationships give immediately, according to the properties of homogeneous linear equations and the definition of \( k,m,n,p ,\)  \[ {\frac{k^\prime}{k} = \frac{m^\prime}{m} =\frac{n^\prime}{n} =\frac{p^\prime}{p} },\quad {\rm{or}}\quad {Dlk=Dlm=Dln=Dlp}. \]

As a result, according to numbers \( 221\) or \( 103\), \( \alpha, \beta, \gamma \) being the constants, \[ lm=lk+l\alpha,\quad ln=lk+l\beta,\quad lp=lk+l\gamma, \]

\[m={\alpha}k,\quad n={\beta}k,\quad p={\gamma}k. \]

Substituting these values of \( m, n, p\) into the identity \( kr+ms+nt+pu=0,\) it becomes, after division by \( k,\) \( r + {\alpha}s + {\beta}t + {\gamma}u = 0, \) a relationship which had to be demonstrated.

IV. REMARK. One can evidently establish analogous theorems to the previous ones on the Wronskians where the derivatives are replaced by partial derivatives or total differentials of the functions in question.

From Cours d’analyse de l’école polytechnique, tome troisieme, by Camille Jordan

122. One can note that the condition \[ \left| {\begin{array}{*{20}c} {x_1 } & {} &  \cdots  & {} & {x_n }  \\ \vdots  & {} &  \ddots  & {} &  \vdots   \\ {x_1^{n - 1} } & {} &  \cdots  & {} & {x_n^{n - 1} }  \\ \end{array}} \right| \ne 0 \] expresses the necessary and sufficient condition for there to exist between the functions \( x_1,\dots, x_n \) no linear relationship with constant coefficients, such that \[ {\alpha}_1 x_1+\cdots + {\alpha}_n x_n =0.\]

In fact, if there existed a relationship of this type, one would obtain, in differentiating it, \[ {\alpha}_1 {x_1^\prime} +\cdots + {\alpha}_n {x_n^\prime} =0,\] \[\ \vdots \] \[ {\alpha}_1 {x_1^{n-1}} +\cdots + {\alpha}_n {x_n^{n-1}} =0,\] and, in eliminating the parameters \( {\alpha}_1 ,\dots , {\alpha}_n ,\) it would become \[ \left| {\begin{array}{*{20}c} {x_1 } & {} &  \cdots  & {} & {x_n }  \\ {x_1^\prime} & {} &  \cdots  & {} & {x_n^\prime}  \\ \vdots  & {} &  \cdots  & {} &  \vdots   \\ {x_1^{n - 1} } & {} &  \cdots  & {} & {x_n^{n - 1} }  \\ \end{array}} \right| = 0. \]

Similarly, if this determinant is zero, \( x_1,\dots, x_n \) will be \( n \) particular solutions of the linear equation of order \( n-1 \) \[ \left| {\begin{array}{*{20}c} {X} & {x_2} &  \cdots  & {x_n }  \\ {X^\prime} & {x_2^{\prime}} &  \cdots  & {x_n^\prime}  \\ \vdots  & {\vdots} &  \cdots  &  \vdots   \\ {X^{n - 1} } & {x_2^{n-1}} &  \cdots  & {x_n^{n - 1} }  \\ \end{array}} \right| = 0. \] One will have therefore, in designating by \( X_1,\dots, X_n \) the independent solutions of this equation, and by \( C_1^{\prime}, \dots , C_{n-1}^n \) the constants, \[ x_1 ={C_1^\prime}X_1 +\cdots + {C_{n-1}^{\prime}}X_{n-1} ,\] \[\ \vdots \] \[ x_n ={C_1^{n}}X_1 +\cdots + {C_{n-1}^{n}}X_{n-1}.\] Eliminating \( X_1,\dots, X_n \) between these equations, a linear relationship between \( x_1,\dots, x_n \) will be deduced. 

[BD] A. Bostan and P. Dumas, Wronskians and linear independence, Amer. Math. Monthly 117 (2010), 722-727.

[B1] M. Bocher. On linear dependence of functions of one variable, Bull. Amer. Math. Soc. 7 (1900) 120-121. Available from American Mathematical Society:
http://www.ams.org/journals/bull/1900-07-03/S0002-9904-1900-00771-3/S0002-9904-1900-00771-3.pdf

[B2] M. Bocher. The theory of linear dependence, Ann. of Math. (2) 2 (1900/1901) 81-96. Available from JSTOR.

[B3] M. Bocher. Certain cases in which the vanishing of the Wronskian is a sufficient condition for linear dependence, Trans. Amer. Math Soc. 2 (1901) 139-149. Available from American Mathematical Society:
http://www.ams.org/journals/tran/1901-002-02/S0002-9947-1901-1500560-5/S0002-9947-1901-1500560-5.pdf

[B4] M. Bocher. On Wronskians of functions of a real variable. Bull. Amer. Math. Soc. 8 (1901) 53-63. Available from American Mathematical Society:
http://www.ams.org/journals/bull/1901-08-02/S0002-9904-1901-00852-X/S0002-9904-1901-00852-X.pdf

[C] D.R. Curtiss, The vanishing of the Wronskian and the problem of linear dependence, Math. Ann. 65 (1908) 282-298. Available from Göttingen State and University Library via Göttinger Digitalisierungszentrum (GDZ) and Deutsche Digitale Zeitschriftenarchiv (DigiZeitschriften):
http://resolver.sub.uni-goettingen.de/purl?GDZPPN002262010

[JMHW] J.M. Hoene-Wronski, Réfutation de la théorie des fonctions analytiques de Lagrange, Blankenstein, Paris, 1812.

[H] M. Ch. Hermite, Cours d’analyse de l’école polytechnique, premiere partie, Gauthier-Villars, Paris (1873) 132-134. Available from Internet Archive Open Library:
http://www.archive.org/stream/coursdanalysedel01hermuoft#page/132/mode/2up

[J] M. C. Jordan, Cours d’analyse de l’école polytechnique, tome troisieme, Gauthier-Villars, Paris (1887) 149-152. Available from Google Books.

[K] M. Krusemeyer, The teaching of mathematics: Why does the Wronskian work? Amer. Math. Monthly 95 (1988) 46-49. Available from JSTOR.

[L] H. Laurent, Traité d’analyse, tome I, Gauthier-Villars, Paris (1885), 183-184. 

[PM] P. Mansion, Résumé du course d’analyse infinitésimale de l’université de Gand, Gauthier-Villars, Paris (1887) 100-102. Available from Google Books.

[TM] T. Muir, A treatise on the theory of determinants, Macmillan, London, 1882; reprinted by Dover, New York, 1960. Also available from Google Books.  

[P1] G. Peano, Sur le déterminant Wronskien, Mathesis 9 (1889) 75-76.

[P2] G. Peano, Sur les Wronskiens, Mathesis 9 (1889) 110-112.

[P3] G. Peano, Sul determinante Wronskiano, Rend. Accad. Lincei, (5) 6 (1897) 413-415.

[PP] P. Pragacz, Notes on the life and work of Józef Maria Hoene-Wronski, Ann. Soc. Math Pol. 43 (2007). Translated by Jan Spalinski in Algebraic cycles, sheaves, shtukas, and moduli, “Trends in Mathematics,” Birkhauser, Basel, 2007, 1-20.

[V] G. Vivanti, Sul determinante wronskiano, Rend. Accad. Lincei, (5) 7 (1898), 194-197.

Acknowledgment

This paper is the result of a new collaboration between the language departments and the mathematics department at Wittenberg University called Culture and Languages Across the Curriculum (CLAC). This program allows students enrolled in any subject to obtain language credit by adding a project with a significant language component to the class. As such, the authors would like to express their great thanks to Professor Tim Wilkerson for all his help with these translations. We would also like to thank the referees for their helpful suggestions that greatly improved this paper.

About the Authors

Susannah Engdahl is a physics major at Wittenberg University in Springfield, Ohio. Upon leaving Wittenberg, she plans to attend graduate school to pursue a degree in biomedical engineering. In her spare time, she enjoys singing with the Wittenberg University choir.

Adam Parker is assistant professor of mathematics at Wittenberg University in Springfield, Ohio. He has undergraduate degrees in mathematics and psychology from the University of Michigan and earned his mathematics Ph.D. in 2005 from the University of Texas at Austin under the direction of Dr. Sean Keel. His research interests include algebraic geometry and the history of mathematics.