Triangular Numbers

In all explorations, students worked in groups of two or three. The exploration began with the reading of a passage on triangular numbers from Thomas Heath's A History of Greek Mathematics: From Thales to Euclid (1921):

The particular triangle which has 4 for its side is mentioned in a story of Pythagoras by Lucian. Pythagoras told someone to count. He said 1, 2, 3, 4, whereon Pythagoras interrupted, “Do you see? What you take for 4 is 10, a perfect triangle and our oath." (p. 77)

After reading this passage and viewing the accompanying diagram (see Figure 3a), students modeled the first four triangular numbers using the Cuisenaire rods in accordance with the passage in slightly different ways (see Figures 3b-d). They then proved the triangular number formula \[1+2+3+\cdots+n={\frac{1}{2}}n(n+1)\] via mathematical induction.

	a (1921, p. 76)
	b
	c
	d

Figure 3:  Triangular numbers \(1, 3, 6, 10\) using Cuisenaire rods

Odd Numbers (Symmetric L-Shaped Gnomons)

Prior to the construction of square numbers, class time was dedicated to the modeling of the consecutive odd numbers \(1,3,5,\dots\) as symmetric L-shaped gnomons (see Figure 4a):

All this was known to Pythagoras. The odd numbers successively added were called gnomons; this is clear from Aristotle's allusion to gnomons placed round 1 which now produce different figures every time (oblong figures, each dissimilar to the preceding one), now preserve one and the same figure (squares). (1921, p. 77)

Students noted that the only Cuisenaire rod that is already in the desired gnomon format is the white rod representing 1. Students in all groups indicated that they must combine various Cuisenaire rods to form gnomons representing odd numbers larger than 1. This activity led to a diversity of representations (see Figures 4b-e). Through a series of debates and considerations, students in all groups eventually agreed on the model in Figure 4e as the one in agreement with the passage they discussed.

	a (1921, p. 77)
	b
	c
	d
	e

Figure 4:  Odd integers \(1, 3, 5, 7, 9, 11\) with Cuisenaire rods

Gnomons and Square Numbers

Having already modeled the gnomons of square numbers (see Figure 4e), it was time to combine them to form a square number at each step while "preserv[ing] one and the same figure (squares)" (1921, p. 77).

Consider the square with \(n\) dots in its side in relation to the next smaller square \({(n-1)}^2\) and the next larger \({(n+1)}^2.\) Then \(n^2\) exceeds \({(n-1)}^2\) by the gnomon \(2n-1,\) but falls short of \({(n+1)}^2\) by the gnomon \(2n+1.\) Therefore the square \({(n+1)}^2\) exceeds the square \({(n-1)}^2\) by the sum of the two gnomons \(2n-1\) and \(2n+1,\) which is \(4n.\) (1921, p. 81)

Using the template given in the text (see Figure 5a), students developed a gnomonic representation of the first six square numbers (see Figure 5b). For \(n\ge2,\) we can see the desired representation showing that the square \({(n+1)}^2\) minus the square \({(n-1)}^2\) equals the sum of the successive gnomons \(2n-1\) and \(2n+1\) at each step (see Figure 5c).

	a (1921, p. 81)
	b
	c

Figure 5:  Square numbers with Cuisenaire rods

Gnomons and Oblong Numbers

The next task was to model oblong numbers; that is, numbers of the sequence

\[2,\,\,2+4, \,\,2+4+6,\dots, \,\,2+4+6+\cdots+2n.\]

For this purpose, students first focused on representation of the even integers with the Cuisenaire rods. As was the case for the odd numbers, students offered different visualizations of the even numbers \(2, 4, 6,\dots\) (see Figure 6).

	a
	b

Figure 6:  Even integers \(2, 4, 6, 8, 10, 12\) with Cuisenaire rods

Students then studied the text along with the accompanying template (see Figure 7a) for the purpose of generating the oblong number sequence:

While the adding of the successive odd numbers as gnomons round 1 gives only one form, the square, the addition of the successive even numbers to 2 gives a succession of 'oblong' numbers all dissimilar in form, that is to say, an infinity of forms (1921, p. 83).

When it came to the modeling of the oblong numbers, the majority of the students selected the model proposed in Figure 6b as the most suitable representation in agreement with the description in the historical resource:

It is to be noted that the word έτερομήκης (‘oblong’) is in Theon of Smyrna and Nicomachus limited to numbers which are the product of two factors differing by unity, while they apply the term προμήκης ('prolate', as it were) to numbers which are the product of factors differing by two or more (1921, p. 83).

Figure 7b depicts the oblong numbers generated based on the gnomonic structure given in Figure 6b. Other groups preferred the simplified \(n\times(n+1)\) rectangular representations in which they used the minimum number of Cuisenaire rods corresponding to each oblong number (see Figure 7c).

	a (1921, p. 82)
	b
	c

Figure 7:  Oblong numbers \(2, 6, 12, 20, 30, 42\) with Cuisenaire rods.

Students in all groups recognized the relation between the \(n\times n\) square number pattern (see Figure 5b) and the \(n\times(n+1)\) rectangular oblong number pattern (see Figure 7), which they easily verified by the distributive property as \(n\times(n+1)=n^2+n\). That is, the \(n\)th oblong number is \(n\) more than the \(n\)th square number (see Figure 8).

Figure 8: Square-oblong relation: the \(n\)th oblong number = the \(n\)th square number \(+\,n.\)

Oblong-Triangular Relationship

After students noted the relationship between square and oblong numbers depicted in Figure 8, the next task was to model any oblong number \(n(n+1)\) as the sum of two equal triangular numbers; see Figure 9a from Thomas Heath's A History of Greek Mathematics: From Thales to Euclid (1921). All students immediately noted the one-half relation between an oblong number and the corresponding triangular number (both analytically and visually) and suggested an oblong number modeling based on two congruent triangular numbers (see Figures 9b and 9c).

	a (1921, p. 83)
	b
	c

Figure 9:  Oblong-triangular relationship with Cuisenaire rods

Theorem of Theon of Smyrna

Upon establishing the oblong-triangular relationship, students focused on the theorem of Theon, which states that any square number is expressible as the sum of two consecutive triangular numbers. As Thomas Heath explained, referring to Figure 10a:

In this case, as is seen from the figure, the sides of the triangles differ by unity, and of course \[{\frac{1}{2}}n(n-1)+\frac{1}{2}n(n+1)=n^2\quad{\sf{\small{(1921, pp. 83{\mbox{-}}84).}}}\]

Figures 10b and 10c depict Cuisenaire rod representations of Theon’s theorem.

	a (1921, p. 84)
	b
	c

Figure 10:  Theon’s theorem, the sum of two consecutive triangular numbers is a square, or \(\frac{1}{2}n(n-1)+\frac{1}{2}n(n+1)=n^2,\) with Cuisenaire rods

Triangular Numbers and Squares: Another Connection

As "quoted by Plutarch and used by Diophantus" (1921, p. 84), the theorem stating

that 8 times any triangular number plus 1 makes a square … is equivalent to the formula

\[{8\cdot\frac{1}{2}}n(n+1)+1=4n(n+1)+1={(2n+1)}^2\quad{\sf\small{{(1921, p. 84).}}}\]

Students first verified the formula given in the text; they then focused on generating Cuisenaire rod patterns modeling the given template (see Figure 11a):

It may easily have been proved by means of a figure made up of dots in the usual way. Two equal triangles make up an oblong figure of the form \(n(n+1),\) as above. Therefore we have to prove that four equal figures of this form with one more dot make up \({(2n+1)}^2.\) The annexed figure representing \(7^2\) shows how it can be divided into four 'oblong' figures \(3\cdot4\) leaving \(1\) over. (1921, p. 84) 

Figures 11b and 11c depict Cuisenaire rod representations of this ancient theorem. Whereas one group of students focused on the “4 oblong plus 1” approach (see Figure 11b), other students were more explicit in their representations by embracing the “8 triangular plus 1” approach (see Figure 11c).

a  (1921, (p. 84)
b
c

Figure 11:  "Eight times any triangular number plus one makes a square" with Cuisenaire rods

Theory of Polygonal Numbers

Figurate numbers whose "figures" are regular polygons, such as triangular numbers and square numbers, are also known as polygonal numbers. Prior to the modeling of the pentagonal and hexagonal numbers using the Cuisenaire rods, students in groups first studied the excerpt on the theory of polygonal numbers, credited to Nicomachus, from Thomas Heath's A History of Greek Mathematics: From Thales to Euclid (1921):

The gnomons of triangles are therefore the successive natural numbers. Squares are obtained by adding any number of successive terms of the series of odd numbers, beginning with \(1,\) or \[1,\,\,3,\,\,5,\,\,\dots,\,2n-1,\dots.\] The gnomons of squares are the successive odd numbers. Similarly the gnomons of pentagonal numbers are the numbers forming an arithmetical progression with \(3\) as common difference, or; \[1,\,\,4,\,\,7,\,\,\dots,\,1+(n-1)\cdot3,\dots;\] and generally the gnomons of polygonal numbers of \(a\) sides  are \[1,\,\,1+(a-2),\,\,1+2(a-2),\,\,\dots,\,1+(n-1)(a-2),\dots\] and the \(a\)-gonal number with side \(n\) is \[1+1+(a-2)+1+2(a-2)+\cdots +1+(n-1)(a-2)=n+{\frac{1}{2}}n(n-1)(a-2).\] The general formula is not given by Nicomachus, who contents himself with writing down a certain number of polygonal numbers of each species up to heptagons. (p. 106)

This summative activity proved beneficial in that students appeared to excel in their understanding of the word “gnomon.” Some groups went back to the exploration of the triangular numbers and explained that every Cuisenaire rod in the formation of a triangular number could be thought of as a gnomon whose combination produces the triangular number itself: Gnomons of triangular numbers are consecutive positive integers. For instance, the gnomons of the triangular number 10 are 1, 2, 3, and 4.

Gnomons of Pentagonal Numbers

Nicomachus wrote that any square number is the sum of two consecutive triangular numbers and (without modern notation) that

an \(a\)-gonal number of side n is the sum of an \((a-1)\)-gonal number of side \(n\) plus a triangular number of side \((n-1),\) i.e. \[n+{\frac{1}{2}}n(n-1)(a-2)=n+\frac{1}{2}n(n-1)(a-3)+\frac{1}{2}n(n-1)\quad{\sf{\small{(1921, p. 106).}}}\]

Letting \(a=5\) in the first half of Nicomachus' theorem, students obtained that a pentagonal number of side \(n\) is the sum of a square number of side \(n\) plus a triangular number of side \(n-1.\) From this, together with the template given in the text (see Figure 12a), students then came up with a diversity of visualizations of the pentagonal number sequence using the Cuisenaire rods (see Figures 12b-f).

• Whereas Figures 12b and 12c model every \(n\)th pentagonal number as a combination of the \(n\)th square number and the \((n-1)\)th triangular number, Figure 12d shows every \(n\)th pentagonal number as decomposed into three structures: the \(n\)th positive integer, plus the \((n-1)\)th oblong number, plus the \((n-1)\)th triangular number.
• Using the fact that any oblong number can be decomposed into two congruent triangular numbers at the same level, the arrangement from Figure 12d was slightly modified to obtain the arrangement that contains three sets of \((n-1)\)th triangular numbers along with the Cuisenaire rod modeling the \(n\)th positive integer (see Figure 12e).
• Finally, Figure 12f shows that every \(n\)th pentagonal number is made of the \(n\)th triangular number and the \((n-1)\)th oblong number.

	a (1921, p. 79)
	b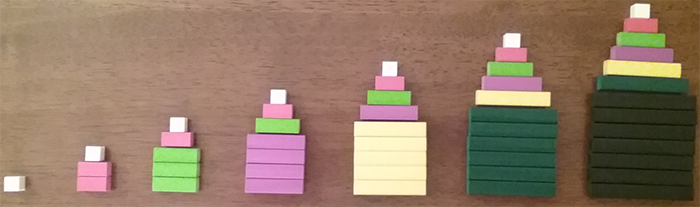
	c
	d
	e
	f

Figure 12:  Pentagonal numbers 1, 5, 12, 22, 35, 51, 70 with Cuisenaire rods

Gnomons of Hexagonal Numbers

Letting \(a=6\) in the first half of Nicomachus' theorem, students obtained that a hexagonal number of side \(n\) is the sum of a pentagonal number of side \(n\) plus a triangular number of side \(n-1.\) From this, together with the template given in the text (see Fig. 13a), students then came up with a diversity of visualizations of the pentagonal number sequence using the Cuisenaire rods (see Figures 13b-f).

• Whereas Figures 13b and 13c model every \(n\)th hexagonal number as a combination of the \(n\)th pentagonal number and the \((n-1)\)th triangular number, Figure 13d models an equivalent arrangement: every \(n\)th hexagonal number is made of the \(n\)th square number and two sets of \((n-1)\)th triangular numbers.
• Using the fact that two congruent triangular numbers are equivalent to the oblong number at the same level (see Figure 9b), an alternative arrangement is obtained as follows: every \(n\)th hexagonal number is made of the \(n\)th square number and the \((n-1)\)th oblong number (see Figure 13e).
• Figure 13f shows another equivalent form of Theon’s Theorem (see Figure 10b) as follows: Because the \(n\)th square number can be decomposed into the sum of the \(n\)th triangular number and the \((n-1)\)th triangular number, we obtain: every \(n\)th hexagonal number consists of the \(n\)th triangular number and three sets of \((n-1)\)th triangular numbers (see Figure 13f).
• Equivalently, by decomposing the \(n\)th triangular number into an \((n-1)\)th triangular number plus \(n,\) we can express every \(n\)th hexagonal number as the combination of four sets of \((n-1)\)th triangular number plus a Cuisenaire rod modeling the integer \(n\) (see Figure 13g); which is equivalent to the combination of two sets of \((n-1)\)th oblong numbers plus \(n\) (see Figure 13h).
• This arrangement also reveals the fact that every \(n\)th hexagonal number can be arranged as an \(n\times(2n-1)\) rectangle. Further decomposition yields the interesting result that every \(n\)th hexagonal number is equal to the combination of two sets of \((n-1)\)th triangular numbers plus the \((n-1)\)th oblong number plus a Cuisenaire rod modeling the integer n (see Figure 13i).
• Finally, a triangular staircase arrangement of hexagonal numbers yields the well-known fact that every hexagonal number is indeed a triangular number – the \((2n-1)\)th triangular number (see Figure 13j).

	a (1921, p. 79)
	b
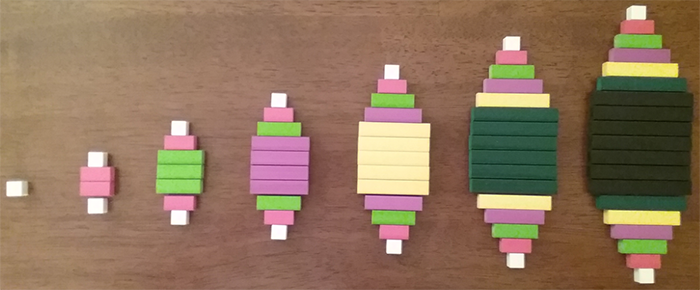	c
	d
	e
	f
	g
	h
	i
	j

Figure 13:  Hexagonal numbers 1, 6, 15, 28, 45, 66, 91 with Cuisenaire rods

Summary

We summarize the figurate numbers explored in the module along with their interrelationships modeled throughout the exploration (see Tables 1-2).

Notation	Figurate Number	Gnomonic Formula
\(T_n\)	\(n\)th triangular number	\(T_n=1+2+3+\cdots+n\)
\(O_n\)	\(n\)th oblong number	\(O_n=2+4+6+\cdots+2n\)
\(S_n\)	\(n\)th square number	\(S_n=1+3+5+\cdots+(2n-1)\)
\(P_n\)	\(n\)th pentagonal number	\(P_n=1+4+7+\cdots+(3n-2)\)
\(H_n\)	\(n\)th hexagonal number	\(H_n=1+5+9+\cdots+(4n-3)\)

Table 1: Figurate number notations and gnomonic formulas

 

Notation	Explicit Formula in \(n\)	Relations to Other Figurate Numbers
\(T_n\)	\(T_n=\frac{n(n+1)}{2}\)	\(T_n=T_{n-1} + n\)
\(O_n\)	\(O_n= {n(n+1)}\)	\(O_n=2T_n\) \(O_n=S_n+n\)
\(S_n\)	\(S_n=n^2\)	\(S_n= T_{n-1}+ T_n\)   \(S_n=O_{n-1}+n\)
\(P_n\)	\(P_n=\frac{n(3n-1)}{2}\)	\(P_n=S_n + T_{n-1}\)   \(P_n= n+ O_{n-1}+ T_{n-1}\) \(P_n=n+ 3T_{n-1}\)  \(P_n= T_n+ O_{n-1}\)
\(H_n\)	\(H_n={n(2n-1)}\)	\(H_n=P_n + T_{n-1}\)  \(H_n=S_n+ 2T_{n-1}\)   \(H_n= S_n+ O_{n-1}\)  \(H_n=T_n+ 3T_{n-1}\)   \(H_n= n+ 4T_{n-1}\)  \(H_n= n+2O_{n-1}\)   \(H_n= T_{2n-1}\)

Table 2: Figurate number formulas and relationships

References

Heath, Thomas L. (1921). A History of Greek Mathematics: From Thales to Euclid (Volume I). Oxford: Clarendon Press. (Also available as a paperback from Dover Publications since 1981 and on Google Books.)

Katz, Victor J. (2009). A History of Mathematics: An Introduction (3rd edition). Addison-Wesley.

Lawlor, R. & Lawlor, D. (1979). Mathematics Useful for Understanding Plato, by Theon of Smyrna, Platonic Philosopher. San Diego: Wizards Bookshelf.

National Council of Teachers of Mathematics (1989). Historical Topics for the Mathematics Classroom (revision of 1969 edition edited by J.K. Baumgart, D.E. Deal, B.R. Vogeli, A.E. Hallerberg). Reston, VA: NCTM.