John Napier (1550-1617)

John Napier first announced his new 'artificial numbers', for which he had coined the name logarithms (from Greek meaning 'ratio numbers'), in his Descriptio published in 1614. This work was limited in scope to the practical application of logarithms and included the first published logarithmic table or what Napier referred to as a 'Canon' (a general law, rule, or principle). This was a table not of the logarithms of numbers but of the logarithms of sines of angles for every minute of the quadrant (the first 90 degrees). It was not until 1619, in his Constructio, that Napier presented the concept of logarithms and the method he used to construct rules for their use in calculation. The preparation of the table and the two books must have taken Napier many years. That he had made significant progress at least ten years before the date of publication is evidenced by Johannes Kepler's 1594 report that Tycho Brahe had recently learned of Napier's canon and hoped it would soon be published. W. W. Rouse Ball stated that Napier “had privately communicated a summary of his results to Tycho Brahe as early as 1594” (1912/2001, p. 195).

Figure 1. Mirifici Logarithmorum Canonis Descriptio (1614). From the Erwin Tomash Library on the History of Computing.

 

Figure 2. Mirifici Logarithmorum Canonis Constructio (1620). From the collection of Sidney J. Kolpas.

The groundbreaking nature of logarithms was widely recognized by Napier's peers. Logarithms replaced clumsy methods by reducing multiplication to addition and division to subtraction. Napier's canon represented a major improvement that in principle provided practitioners of mathematics, such as astronomers, surveyors, and navigators, with a new, powerful tool with which to carry out large scale computation much more easily. In practice, however, Naperian logarithms were inconvenient because their base was not decimal. Napier quickly became aware of this difficulty for in the second issue of the first edition of the Descriptio, also published in 1614, he included a brief closing 'Admonitio' in which he mentioned that another system of logarithms more suitable for practical calculation in the decimal system would soon be available. This was a reference to the decimal logarithms that we know today, sometimes referred to as Briggsian logarithms or common logarithms.

Figure 3. Henry Briggs' proposal for base 10 logarithms from Napier's Canonis Constructio (1620). From the collection of Sidney J. Kolpas

Soon after the initial publication of the Descriptio, Henry Briggs (1561-1630), the first Gresham Professor of Geometry in London and later the first Savilian Professor of Geometry at Oxford, undertook, with the active cooperation of Napier, the calculation of a set of decimal-based logarithms. Briggs' work was taken up and completed by the Dutch mathematician, Adriaan Vlacq, so that by 1628, only 14 years after Napier's initial announcement, convenient, multi-place decimal logarithmic tables were available to simplify computation.

Figure 4. Vlacq's Tabulae Sinuum, Tangentium, et Secantium, et Logarithmorum (1670). From the Erwin Tomash Library on the History of Computing.

The calculation and application of logarithms occupied practitioners of mathematics well into the next century. Even two centuries later, one of the major influences motivating Charles Babbage to undertake the design of his Difference Engine was his desire to automatically generate error-free, easy-to-read logarithmic tables.

Figure 5. Modern execution of Babbage's plans for his Difference Engine

Figure 6. Babbage's Table of Logarithms (1827). From the Erwin Tomash Library on the History of Computing.

The invention of logarithms led in short order to the invention of mechanical calculating devices based upon them. In 1620, Edmund Gunter described his 'line of numbers', the physical expression of decimal logarithms as a sequence of lengths on a straight edge. He then added more 'lines' for the logarithms of the trigonometric functions, creating a popular device commonly referred to as Gunter's scale.  In 1630, Edmund Wingate described the sliding of two such scales against each other, thus inventing the linear slide rule. Only two years later, William Oughtred marked a Gunter's line on the edge of a circle and rotated two such circles against each other, thus inventing the circular slide rule.

Figure 7. Circular slide rule

However, Napier's interest in developing computational aids was not restricted to logarithms. In his 1617 Rabdologiae, to be discussed in the next section of this article, he presented three other inventions for this purpose.

Napier's 'Rabdologiae'

Logarithms were by no means the only computational aid invented by Napier. In 1617, the year of his death, Napier published Rabdologiae, a slender volume describing a trilogy of mechanical calculating aids. Remarkably, while none of the three are based upon logarithms, one is based on the binary number system, predating by almost a century Gottfried Wilhelm Leibniz's better known treatise on the binary number system, "Explication de l'Arithmétique Binaire" (1703). (To view this treatise in Convergence, see the article "Russian Multiplication, Microprocessors, and Leibniz".)

Figure 8. John Napier's Rabdologiae (1617). From the Erwin Tomash Library on the History of Computing. An entire copy of the Rabdologiae, with commentary by Tomash and Michael R. Williams, can be viewed here, courtesy of the Tomash Library. Another copy is available via Google Books.

Napier's 'bones'

The best known of the trilogy of topics in Rabdologiae, the so-called 'Napier's bones', was a set of rods used for multiplying, dividing, and extracting roots. As Napier noted in the introduction to Rabdologiae, his rods were in quite common use by the time the book itself was published. Napier's rods are simply portions of the multiplication table inscribed in gelosia- or lattice-format on sticks made of wood or bone. These 'bones' surely represent the first, albeit primitive, mechanical pocket calculator.

For an example of multiplication using Napier's rods, see the Convergence article, "John Napier: His Life, His Logs, and His Bones". 

Gelosia multiplication is also known as latticed multiplication. The term 'gelosia' is due to Luca Pacioli (ca. 1445-ca. 1514), who used it in his Suma de Arithmetica, Geometria, Proportioni et Proportionalita (Venice, 1494). The pattern suggests the gratings placed in Venetian windows to protect residents from the public gaze. Known as gelosia, the Italian word for jealousy, they were designed to permit women to peep out and yet remain unseen from the street. The English word 'jalousie' for a type of Venetian blind has come down to us.

Figure 9. Napier's bones (or rods)

The widespread acceptance of Napier's rods in a few short years is perhaps better understood through J. W. L. Glaisher's comment (1874):

There is abundant evidence that, till comparatively recent times (say the beginning of the eighteenth century), multiplication was regarded as a most laborious operation; this is testified not only indirectly by the very simple examples given in old arithmetics, but explicitly by Decker in his 'Erste Deel van de Nieuwe Telkonst'. The great popularity of Napier's bones, and the eagerness with which they were received all over Europe, show how great an assistance the simplest contrivance for reducing the labour of multiplications was considered to be.

Napier's 'promptuary'

In the first appendix to the Rabdologia, Napier introduced a second, more complex rod machine capable of much higher-speed multiplication. This was a two-dimensional configuration of rods which he named the Promptuary. The sole known early example of a Promptuary resides in a museum in Madrid, Spain, where the second author happily discovered it over three decades ago (Tomash, 1988).

Figure 10. Napier's Promptuary

Napier's 'chessboard calculator'

The third and final section of the Rabdologia is a 37-page addendum that describes what Napier called "Location Arithmetic as Performed on a Chessboard", and this arithmetic is the focus of the present article. Napier's enthusiasm for his Chessboard Calculator is apparent from his remarks in the preface to the addendum, which is worth quoting in its entirety (Napier, 1990):

While working in my spare time on these short methods and seeking ways in which the tedium of calculation might be removed, I developed (not only my logarithms, my Rabdology, my Promptuary for multiplication and other things, but also) a method of arithmetic on a flat surface. As this performs all the more difficult operations of common arithmetic on a chessboard, it might well be described as more of a lark than a labor, for it carries out addition, subtraction, multiplication, division, and [even] the extraction of roots purely by moving counters from place to place. There is one small difficulty in working with it, and that is that the numbers it uses differ from ordinary numbers, so that one must begin by expressing ordinary numbers in the new form and end by reducing them to common form. Either process is simple enough even during the course of the calculation, and overall my method is easier and more accurate than almost any other short method of Arithmetic. I therefore resolved not to bury it in silence nor (as it is so short) to publish it on its own but to subjoin it to my Rabdology after the aforementioned promptuary for the benefit of the studious and the scrutiny of the learned.

Despite Napier's enthusiasm, the Chessboard Calculator never seems to have found favor. "Location Arithmetic as Performed on a Chessboard" was largely ignored and in subsequent years was reported on as a mere curiosity.  It is somewhat ironic that within Napier's unheralded Chessboard Calculator lies buried one of the earliest applications of the binary number system—described as "a pioneering explanation of binary arithmetic as a computational aid" by Robin Rider in the Introduction to a translation of Napier's Rabdologiae (Napier 1990, p. xxiii). We find Napier's invention to be worthy of explication for at least two reasons: first, his early use of binary arithmetic, which he identified as the "one small difficulty in working with" his Chessboard Calculator, and, second, its potential as a source of enriching educational experiences for today's students.

Napier's Chessboard Calculator is described in the final section of his Rabdologiae. Titled "Location Arithmetic as Performed on a Chessboard" ("Arithmeticae localis, quae in Scacchiae abaco exercetur"), this 42-page section consists of 11 short chapters with diagrams. The remainder of this article amplifies and simplifies Napier's rather terse exposition of his invention, beginning with a brief discussion of his pioneering use of binary arithmetic and a physical description of the Chessboard Calculator. It then presents the axioms for its use and describes how to use it to add, subtract, multiply, divide, and take square roots. The article concludes with some potential educational applications.

From a modern perspective, the required background knowledge for understanding the operation of the Binary Chessboard Calculator is minimal, the sole prerequisite being a rudimentary understanding of the binary number system. From Napier's early 17th century perspective, the binary number system was unknown (Glaser, 1971) and neither exponential notation nor the concept of base had as yet crystallized. However, the arithmetic processes known as mediation (halving) and duplation (doubling) were well known and familiar. These are of ancient origin and their use persisted throughout the Middle Ages and well past Napier's time. (Early in the 17th century, the first form of our modern exponential notation was introduced by Rene Descartes in his La Géométrie of 1637.)

The Chessboard Calculator is based on mediation and duplation handled through an intermediate set of alphabetic "location" numbers. Napier created an alphabetic notation for the successive powers of 2 which he described as "ordinary numbers expressed in a new form." The use of alphabetic number systems was, of course, commonplace in Napier's time, the most obvious example being Roman numerals, which were used in England well into the 17th century. This article will discuss a simplified version of the Chessboard Calculator without the alphabetic "location" numbers.

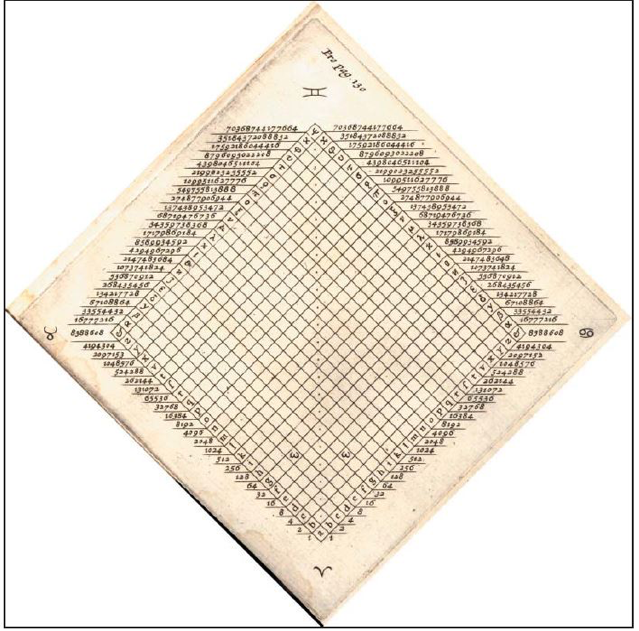
Figure 11. Napier's Chessboard Calculator from his 1617 Rabdologiae. From the Tomash Library on the History of Computing, where the authors of the commentary on the digitized copy of the book argued that Napier himself described the orientation of the chessboard calculator as it is shown here (Tomash & Williams, 2009) 

In its utilization of simple, everyday components, the Chessboard Calculator is yet another instance of Napier's faculty of uniting basic elements with great theoretical insight. Both chessboards and counters were commonplace in Napier's milieu. Boards for the games of chess and checkers, the latter known in England as "draughts", were to be found everywhere. Casting counters were likewise ubiquitous because they were used in daily life as aids to commercial calculation. We suggest using bingo chips, checkers, candy, or the like as counters. 

Napier's two-dimensional calculating board is a chessboard (or checkerboard) with the addition of a lower horizontal margin and right vertical margin, the margins being used to represent the values in a given arithmetic problem. The value of the lower right square is 1, with powers of two progressing both horizontally and vertically.  There is no limit on the size of the board, which may be increased for use with larger numbers.

Figure 12. Napier's Chessboard Calculator, updated for modern use. (Download a copy of this diagram here.)

To better understand the fundamentals of Napier's Chessboard Calculator, the board itself (see Figure 12), as well as its bottom and right edges, has been labeled using powers of 2. Equivalence lines are the Southwest-Northeast (SW-NE) diagonal lines on the board, which pass through squares of equal values. The Northwest-Southeast main diagonal passes through powers of two with even exponents; that is, powers of 2 that are perfect squares: 1, 4, 16, 64, 256, 1024, 4096, 16384.

Axioms of the Chessboard Calculator

The following axioms relate the Chessboard Calculator to the game of chess. However, the most important of the axioms below is the last, which points out that squares on the chessboard are labeled so that the square at the intersection of a horizontal margin number and a vertical margin number holds the product of those two numbers.

In the game of chess, a Bishop moves diagonally in any direction from the square it currently occupies, and a Rook moves in any direction horizontally or vertically from the square it currently occupies. The following axioms may be verified by inspection of the Binary Chessboard.

Diagonal (Bishop) Moves:

Axiom 1:  Moving diagonally up and to the left multiplies by four with each square moved.

Axiom 2:  Moving diagonally down and to the right divides by four with each square moved.

Axiom 3:  Moving diagonally along an equivalence line (SW-NE) makes no change in value with each square moved.

Horizontal/Vertical (Rook) Moves: Duplation and Mediation

Axiom 4:  Moving horizontally to the left multiplies by two with each square moved.

Axiom 5:  Moving horizontally to the right divides by two with each square moved.

Axiom 6:  Moving vertically up multiplies by two with each square moved.

Axiom 7:  Moving vertically down divides by two with each square moved.

Multiplication Axiom:

Axiom 8:  The entry in the square at the intersection of a horizontal margin number and a vertical margin number is the product of those two numbers.

In succeeding pages of this article, we will learn how to use Napier's binary chessboard calculator to add, subtract, multiply, divide, and extract square roots.

How to compute the sum of two positive integers:

Use bingo chips, checkers, candy, etc. as counters.

Step 1.  Place the first number on the bottom horizontal row.

Step 2.  Place the second number below it in the margin.

Step 3.  Combine all the counters on the bottom horizontal row.

Step 4.  Abbreviate the board from right to left. That is, remove every two counters in a square and place one counter in the square to its left. This process often causes a "chain reaction" that "carries" into squares further to the left, requiring further abbreviation.

Step 5.  The desired answer can now be obtained by converting the abbreviated sum back to a decimal number.

Example: That 116 + 84 = 200 is illustrated below.

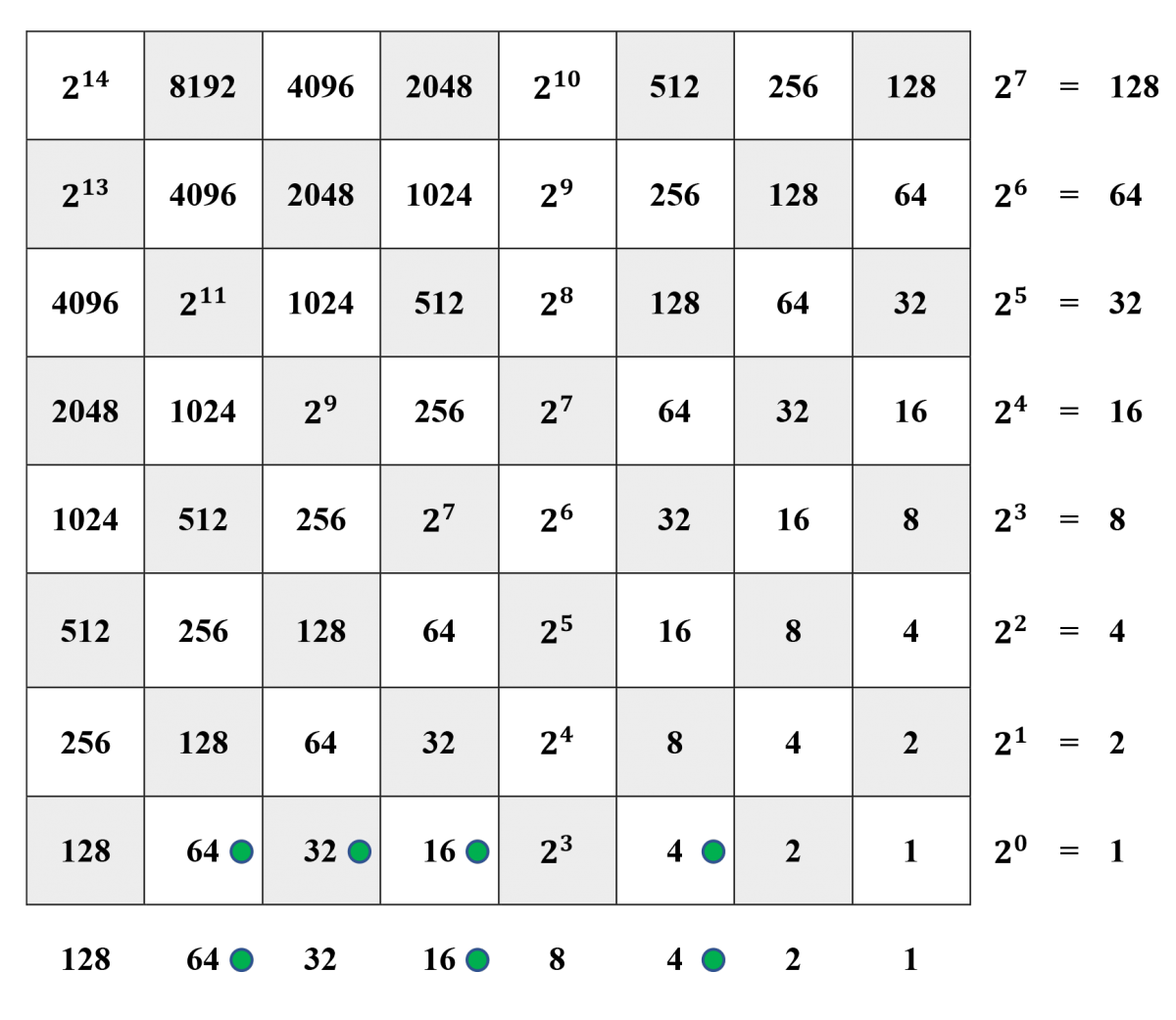
Steps 1 and 2. First 116, decomposed as \[116 = 2^6 + 2^5 + 2^4 +{\phantom{2^3}}+2^2 = 64 + 32 + 16 +{\phantom{xx}}+4,\] is represented in the bottom horizontal row and \[84 = 2^6 + {\phantom{2^5}}+ 2^4 +{\phantom{2^3}}+2^2 = 64 +{\phantom{32}}+ 16 +{\phantom{xx}}+4,\] is recorded just below it in the margin.

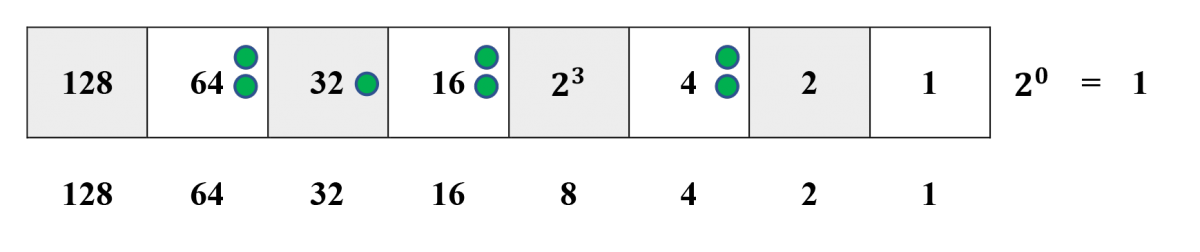
Step 3. Next, all of the counters are combined in the bottom horizontal row.

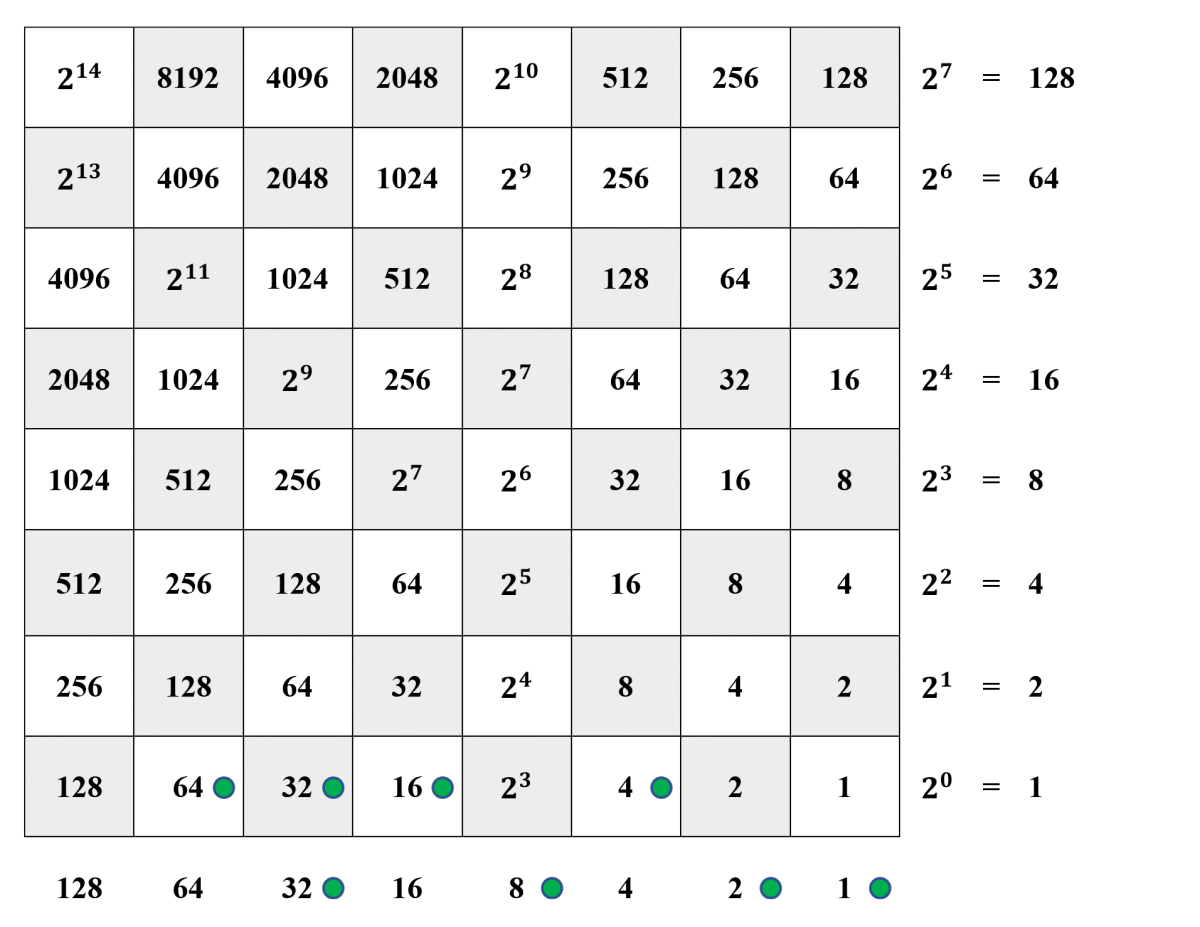
Step 4. The board is abbreviated in two steps: First, from each square that had two counters after Step 3, one counter is moved to the square to the left and the other is removed. The result is shown above. Second, the abbreviation is repeated: from the square marked "32", one counter is moved to the left and the other is removed. The result is shown below.

Step 5: Now that each square along the bottom row contains at most one counter, the sum can be read from this row as 116 + 84 = 128 + 64 + 8 = 200.

Next: subtraction on the chessboard calculator

How to subtract one positive integer (the subtrahend) from another, larger positive integer (the minuend) (Gardner 1973):

Step 1.  Place the larger number on the bottom horizontal row.

Step 2.  Place the binary complement of the number to be subtracted in the margin below the bottom horizontal row. That is, where there would be a counter in the number itself, leave the square empty; and where there would not be a counter, place one on the square. Do this for all entries from right to left through the highest power of 2 occurring in the decomposition of the larger number.

Step 3.  Combine all the counters on the bottom horizontal row.

Step 4.  Abbreviate the board from right to left; that is, remove every two counters in a square and place one counter in the square to its left. This process often causes a "chain reaction" that "carries" into other squares, requiring further abbreviation.

Step 5.  Add one more counter in the 1's place (\(2^0\)-place) square in the bottom row. This may cause a "chain reaction" that "carries" into squares to the left, requiring further abbreviation.

Step 6.  The desired answer can now be obtained by converting the abbreviated difference back to a decimal number, except that you must ignore the farthest left counter (which may already have "fallen" off the left side of the board).

Example: That 116 – 84 = 32 is illustrated below.

Steps 1 and 2. The larger integer, 116, decomposed as \[116 = 2^6 + 2^5 + 2^4 +{\phantom{2^3}}+2^2+{\phantom{2^1}}+{\phantom{2^0}} = 64 + 32 + 16 +{\phantom{8}}+4+{\phantom{2}}+{\phantom{1}},\] is represented by counters in the bottom horizontal row. Now we decompose 84 as \[\phantom{0}84 = 2^6 + {\phantom{2^5}}+ 2^4 +{\phantom{2^3}}+2^2+{\phantom{2^1}}+{\phantom{2^0}} = 64 +{\phantom{32}}+ 16 +{\phantom{8}}+4+{\phantom{2}}+{\phantom{1}},\] and write its complement \[{\phantom{84=2^6++}} {2^5} + {\phantom{2^4}} +{2^3}+{\phantom{2^2}} +{2^1}+{2^0}={\phantom{x2^6+}} 32 + {\phantom{16}} +8+{\phantom{4}} +2+1.\] The complement is represented in the margin below the bottom horizontal row.

The binary complement of a positive integer is sometimes called the "1's complement" because one can compute it by interchanging 0's and 1's in the binary-digit representation of the positive integer. For instance:

64        32        16         8         4         2         1

  1          0          1         0         1         0         0     \(\leftarrow\) Binary-digit representation for 84

  0          1          0         1         0         1         1     \(\leftarrow\) 1's complement of 84

The binary complement of 84 is therefore 32 + 8 + 2 + 1.

Step 3. Combine all of the counters in the bottom horizontal row:

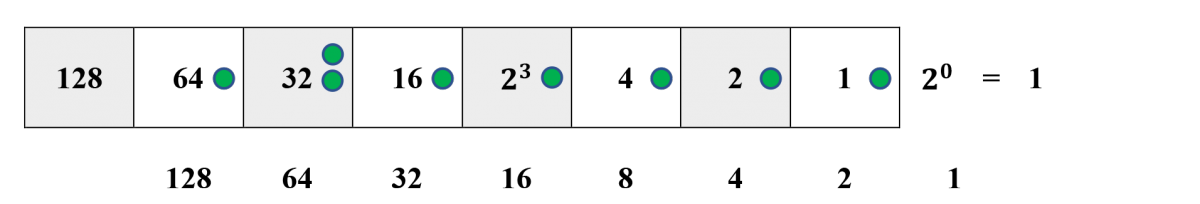
Step 4. Abbreviate the row from right to left. Note that there is now a counter in the 128 or \(2^7\) place:

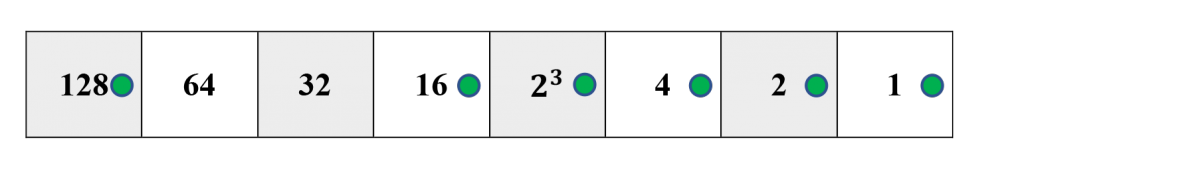
Step 5.  Add +1; that is, add one more counter to the 1's place, and ...

... abbreviate the row from right to left:

Step 6.  Now, ignoring the farthest left counter, the difference can be read from this row as 116 – 84 = 32.

Next: multiplication on the chessboard calculator

How to compute the product of two positive integers:

Step 1. Place counters representing one factor along the bottom (horizontal) margin and counters for the other along the right-hand (vertical) margin. 

Step 2. Place a counter in each square on the board representing the intersection of a column for which there is a counter in the bottom (horizontal) margin with a row for which there is a counter in the right-hand (vertical) margin. The square represents the product of those two values.

Step 3. Remove the counters representing the factors from both margins.

Step 4. Slide all counters on the board along their (SW-NE) equivalence lines down to the bottom horizontal row. (This is how a bishop moves in chess.)

Step 5. Abbreviate the bottom horizontal row from right to left, removing every two counters in a square and placing one counter in the square to its left. This process often causes a "chain reaction" that "carries" into squares to the left, requiring further abbreviation.

Step 6. The desired decimal product can now be obtained by converting the abbreviated product back to a decimal number. 

Note that products represented on our \(8\times 8\) binary chessboard can be no larger than 255.

Example: That \(18 \cdot 13 = 234\) is illustrated below.

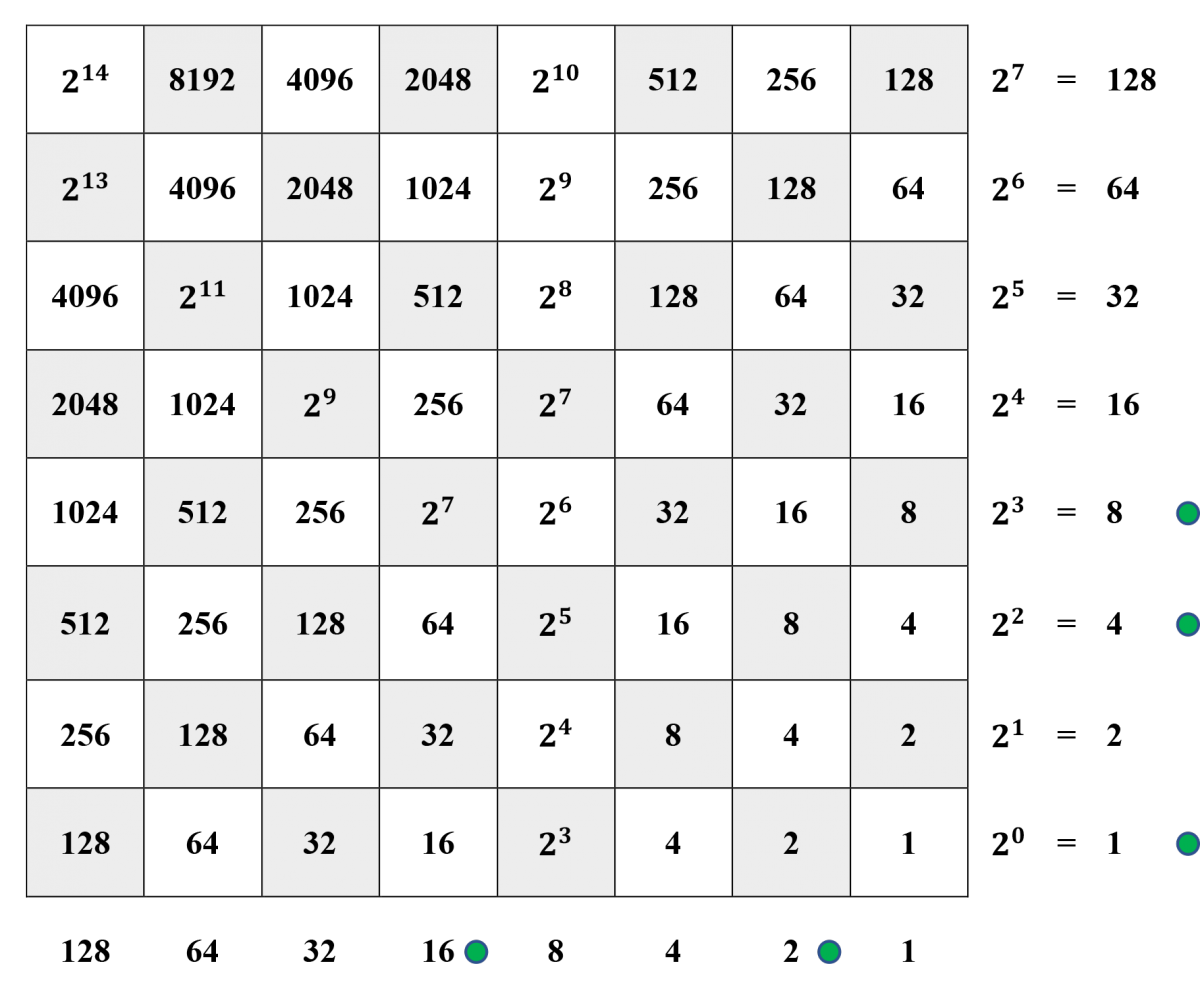
Step 1. The factor \(18=16+2\) is represented along the bottom margin and the factor \(13 = 8 + 4 + 1\) is represented along the right-hand margin.

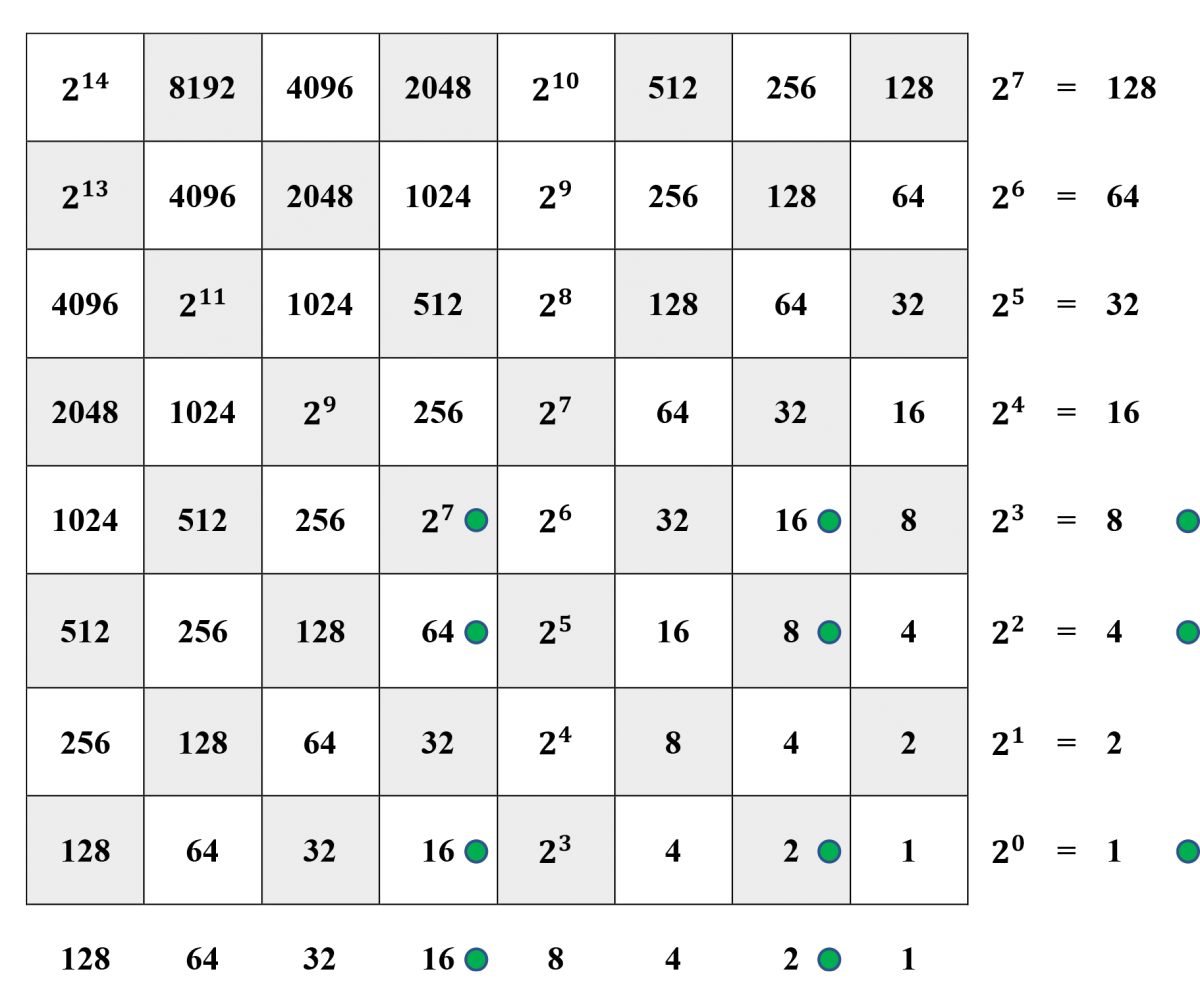
Step 2. Counters are placed at the intersections of columns and rows for which there are counters both along the bottom margin and along the right-hand margin. For instance, a counter is placed at the intersection of the column labeled 16 at the bottom and the row labeled 4 at the right. The intersection of this column and row is a square labeled 64, and you should check that \(16\cdot 4 = 64.\)

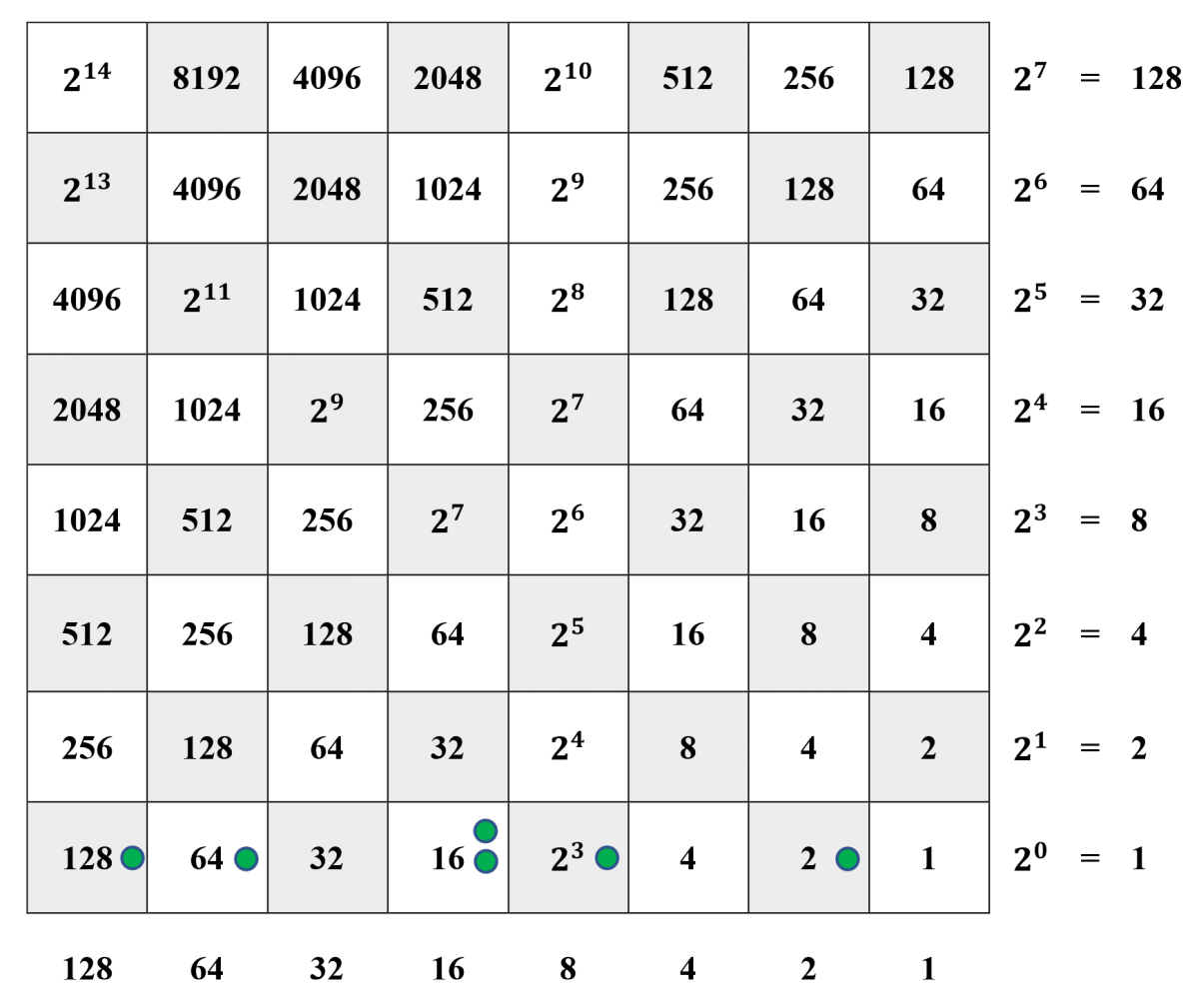
Steps 3 and 4. Counters have been cleared from the bottom (horizontal) and right-hand (vertical) margins. Counters on the board have been slid down their equivalence lines to the bottom horizontal row. For instance, the only counter at a square labeled 64 has been slid along the 64-equivalence line to the 64-square in the bottom row. Likewise, the two counters that were in squares along the 16-equivalence line now both sit in the 16-square in the bottom row.

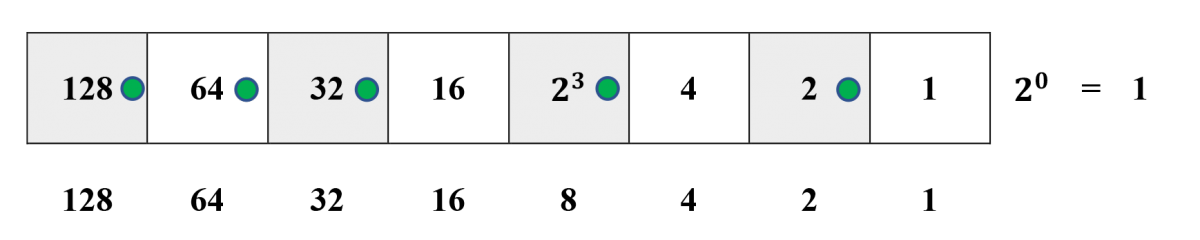
Steps 5 and 6. The bottom row has been abbreviated from right to left, leaving a row from which we can read \[18\cdot 13 = 128 + 64 + 32 + 8 + 2 = 234.\] The reader may wish to carry out the multiplication \(6\cdot 15,\) an example requiring extensive carries in the abbreviation process.

In preparation for extracting square roots on the chessboard calculator, it is worth noting here that, after obtaining \(18\cdot13=(16+2)(8+4+1)\) in Step 1 above, Step 2 amounts to an application of the distributive law to obtain:

\[(16+2)(8+4+1) = 16\cdot8 + 2\cdot8 + 16\cdot4 + 2\cdot4 + 16\cdot1 + 2\cdot1,\] with the six products on the right-hand side of this equation necessarily arranged in a 3 row by 2 column rectangle on the chessboard. More specifically, removal of one entire row and two entire columns from the second board shown above would result in a 3 row by 2 column rectangle consisting of six contiguous squares with counters in them.

Next: division on the chessboard calculator (followed by extraction of square roots)

How to divide one positive integer (the dividend) by another, smaller positive integer (the divisor), obtaining a non-negative integer quotient and a non-negative integer remainder:

Step 1. Represent the dividend in the horizontal margin and the divisor in the vertical margin.

Step 2. Place a counter on the largest number in the first column (farthest column to the right) that when multiplied by the divisor is less than or equal to the dividend.

Step 3. Multiply that number by the divisor and subtract the product from the dividend. Replace the dividend (in the horizontal margin) with this result, which will become the current dividend.

Step 4. Repeat steps 2 and 3 with the current dividend until you can no longer do so.

Step 5. The sum of the chips in the first column is the quotient. The current dividend is the remainder.

Example: That \(100\) divided by \(7\) results in a quotient of \(14\) and a remainder of \(2\) is illustrated below.

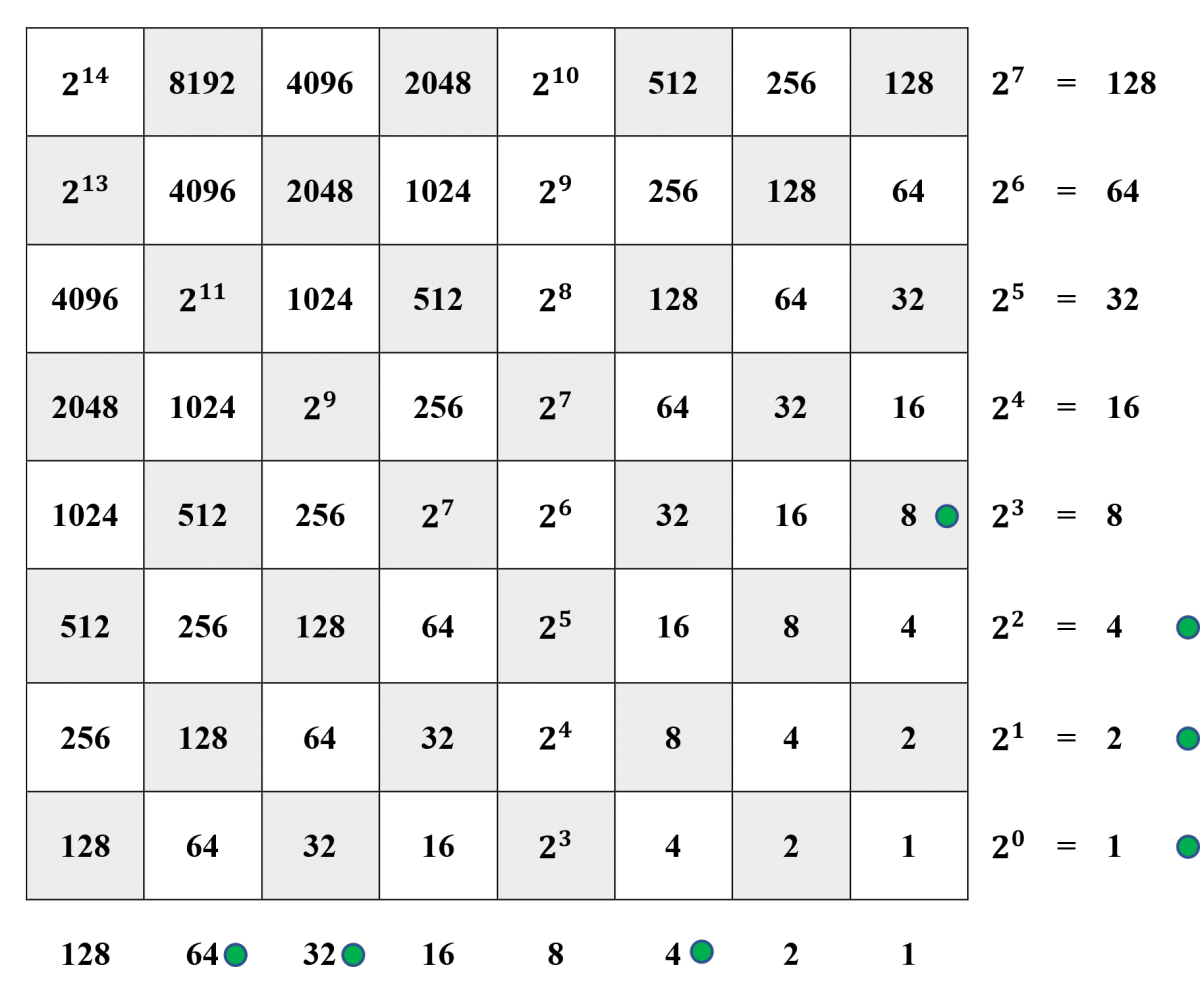
Steps 1 and 2. The dividend \(100\) is represented in the horizontal margin and the divisor \(7\) in the vertical margin. Since \(7\cdot 16 > 100,\) but \(7\cdot 8 \le 100,\) a counter has been placed on the 8-square in the right-hand column.

Steps 3 and 4. Since \(7\cdot 8 = 56,\) the current dividend is \(100-56 = 44,\) as represented in the horizontal margin. Furthermore, since \(7\cdot 8 > 44,\) but \(7\cdot 4 \le 44,\) a counter has been placed on the 4-square in the right-hand column.

Step 4. Since \(7\cdot 4 = 28,\) the current dividend is \(44-28 = 16,\) as represented in the horizontal margin. Furthermore, since \(7\cdot 4 > 16,\) but \(7\cdot 2 \le 16,\) a counter has been placed on the 2-square in the right-hand column.

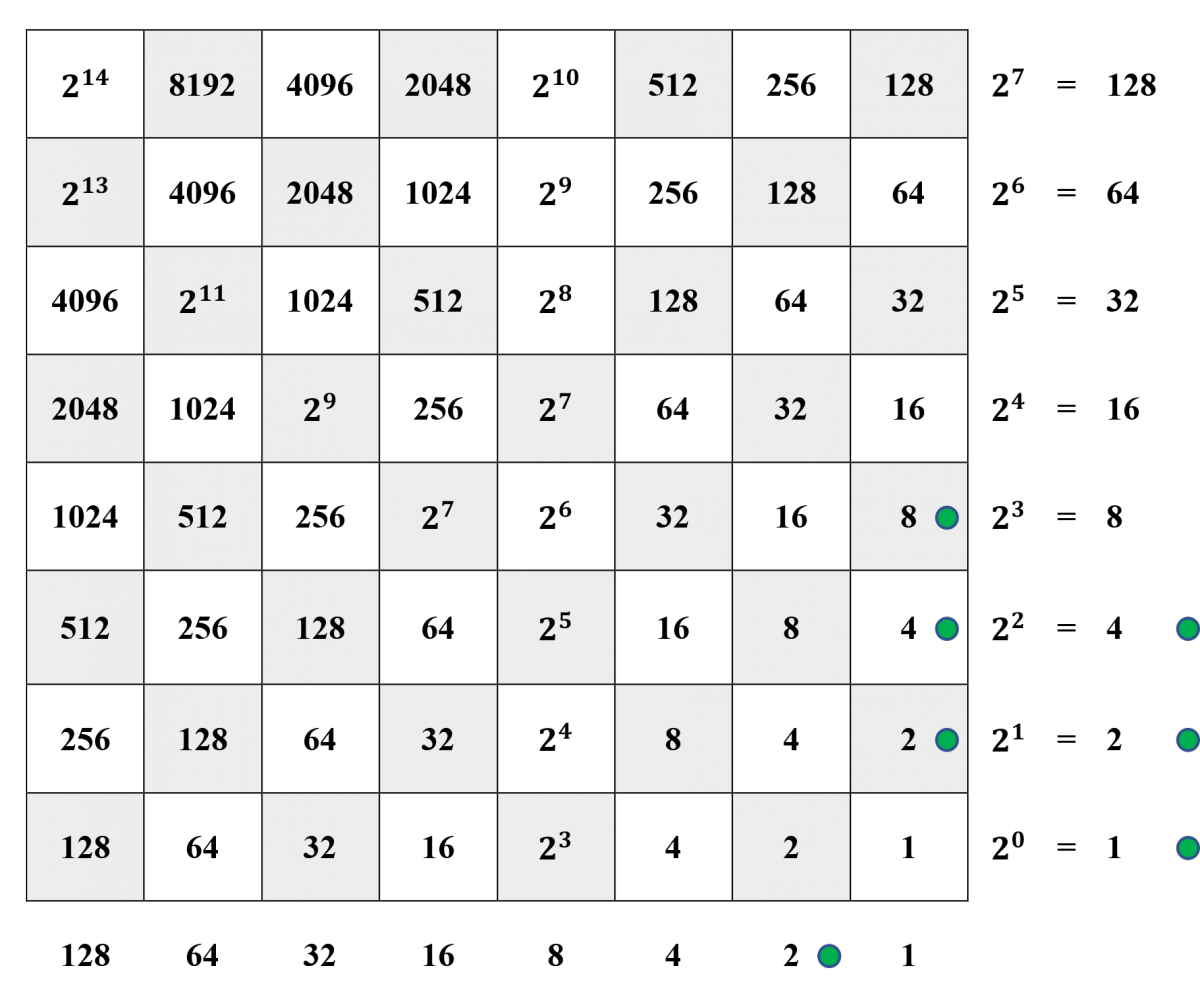
Step 4, continued. Since \(7\cdot 2 = 14,\) the current dividend is \(16-14 = 2,\) as represented in the horizontal margin. Since \(7\cdot 1 > 2,\) we cannot continue.

Step 5. We conclude that dividing \(100\) by \(7\) results in a quotient of \(8 + 4 + 2 = 14\) and a remainder of \(2.\)

Next: Extracting square roots on the chessboard calculator

How to compute the largest integer square less than or equal to a given positive integer:

Recall first that multiplication of two positive integers, such as \(18\) and \(13,\) consists of placing \(18\) along the bottom (horizontal) margin of the binary chessboard and placing \(13\) along the right-hand (vertical) margin of the binary chessboard (Step 1), which corresponds to computing their product as \(18\cdot13=(16+2)(8+4+1).\) The next step (Step 2) amounts to an application of the distributive law to obtain: \[(16+2)(8+4+1) = 16\cdot8 + 2\cdot8 + 16\cdot4 + 2\cdot4 + 16\cdot1 + 2\cdot1,\] with the six products on the right-hand side of the equation necessarily arranged in a 3 row by 2 column rectangle on the chessboard. Using the same process, if we multiply \(13\) by \(13\)––that is, if we square \(13\)––our first two steps will be to represent the product \(13\cdot13\) as \((8+4+1)(8+4+1),\) then to apply the distributive law to obtain:
\[(8+4+1)(8+4+1) = 8\cdot8 + 4\cdot8 + 1\cdot8 + 8\cdot4 + 4\cdot4 + 1\cdot4 + 8\cdot1 + 4\cdot1 + 1\cdot1,\] with the nine products on the right-hand side of the equation necessarily arranged in a 3 row by 3 column square on the chessboard, as shown below. This square will be symmetric about the main (NW-SE) diagonal and thus will have 3 entries along the main diagonal, including its upper left corner entry and its lower right corner entry. Furthermore, if we remove one row and one column from the chessboard in order to make the nine squares with counters in them contiguous to one another, the resulting 3 x 3 square still would have these properties.

For a positive integer \(n,\) Napier's goal was to find the largest integer square less than or equal to \(n.\) His strategy, essentially, was to find the geometric square of largest area less than or equal to \(n.\) He began with the largest integer square entry less than or equal to \(n\) among the even perfect square entries along the main (NW-SE) diagonal of his chessboard. He called this even perfect square the "head of the gnomons" and marked its location with a counter (\(2^6\) in our example). Napier then formed a square in the lower right corner of the chessboard with the "head of the gnomons" at its upper left. Then, among the colorful backwards L-shaped gnomons (see Note) making up the "square-of-gnomons" (our term; not his) shown in the chessboard below, Napier marked with counters entries that both formed a square and kept the total value of that square less than or equal to \(n.\) The sum of the values of the squares with markers on them was then the largest integer square less than or equal to \(n\) and the square root could be read from the margins of the chessboard. Reversing our example above, if we take \(n=169,\) then, since the sum of the entries marked with counters is \(169,\) we conclude that the square root of \(169\) is \(8+4+1=13.\)

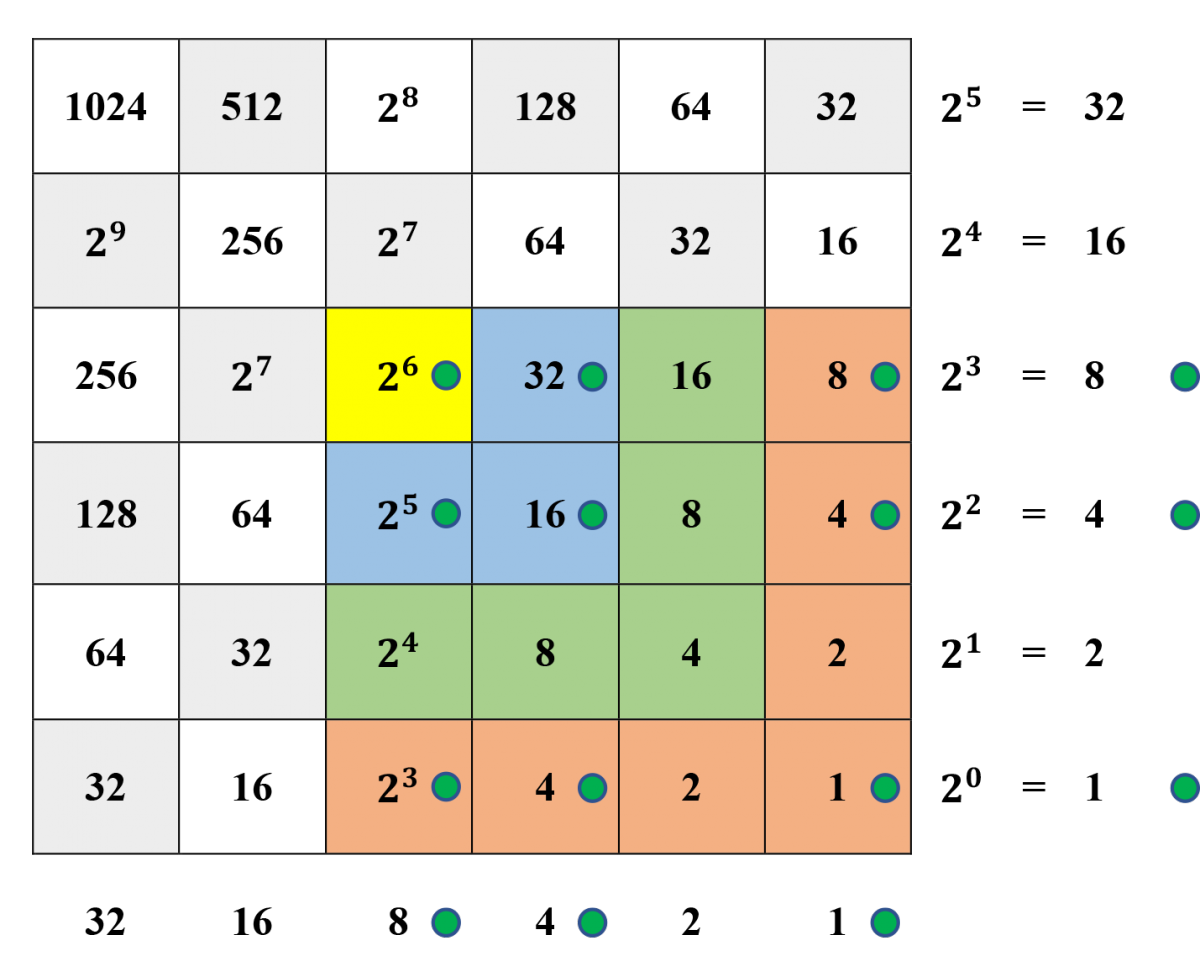
Notice that the yellow gnomon, the "head of the gnomons", consists of 1 square; the blue gnomon consists of 3 squares, each with a counter; the green gnomon has no counters; and the orange gnomon has 7 squares but only 5 of them are marked with counters. If we remove an entire row and an entire column from the chessboard, as shown below, the nine squares with counters will form a 3 x 3 square with no gaps in it.

Keeping these hints in mind, we will illustrate Napier's process using two examples.

Example:  That the square root of 180 has integer part 13 with remainder 11 is illustrated on Napier's binary chessboard calculator.

Step 1. Represent 180 in the horizontal margin.

Step 2. Identify \(2^6 = 64\) as the largest value along the main (NW-SE) diagonal that is less than or equal to 180. Designate the square along the main diagonal containing \(2^6\) the "head of the gnomons" and place a counter on it. (We have also colored it yellow.)

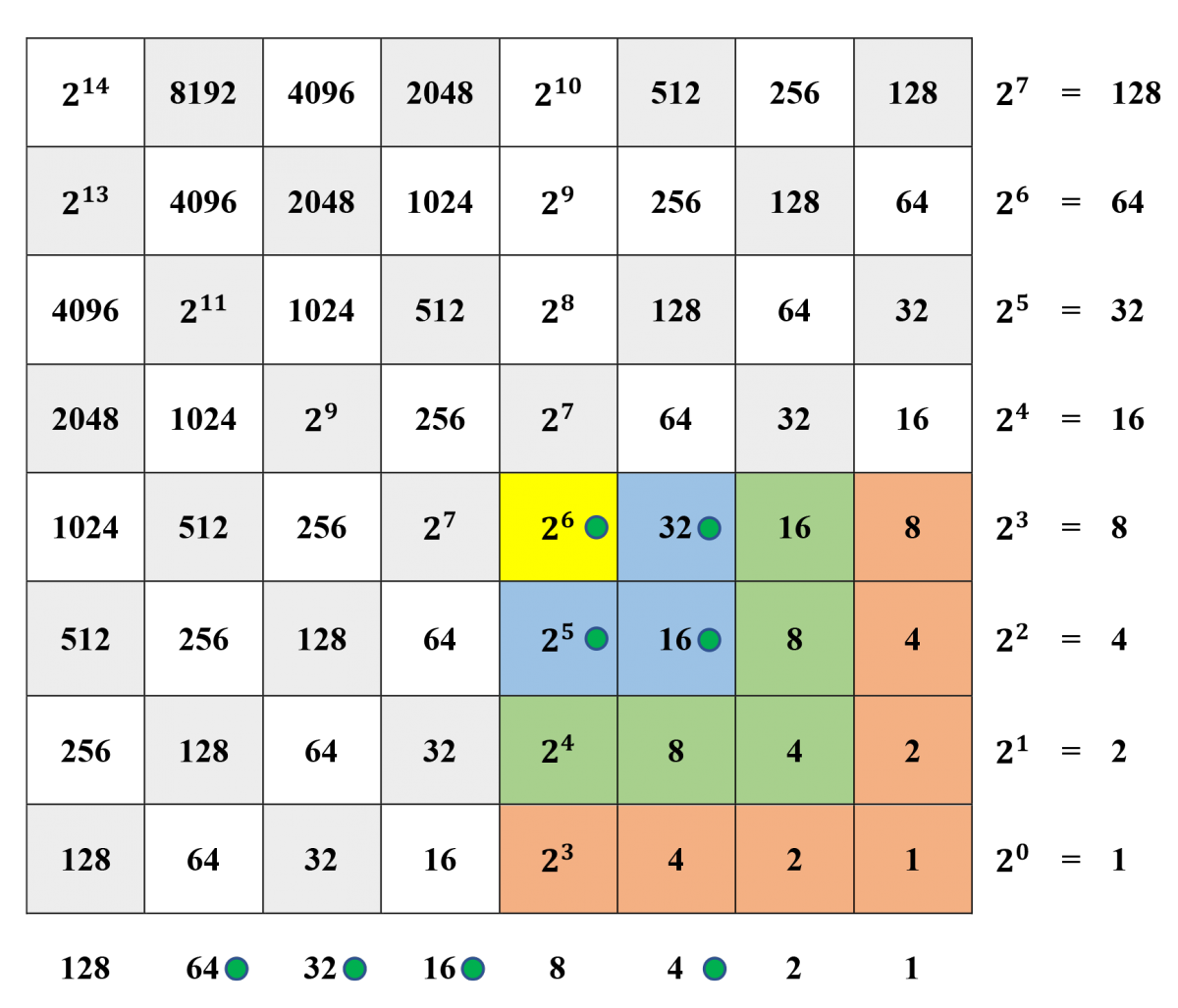
Step 3. Subtract \(2^6 = 64\) from the current value (180) along the horizontal margin, obtaining \(180 - 64 = 116\), and replace 180 with 116 along the horizontal margin.

Step 4. From the "head of the gnomons", travel down the main diagonal, searching for the first gnomon with its corner along the main diagonal in which 3 of the squares have a value less than or equal to 116. Since the blue gnomon with entries \(2^5, 2^4,\) and \(2^5\) satisfies \(2^5 + 2^4 + 2^5 = 80\le 116,\) we place counters on its three squares.

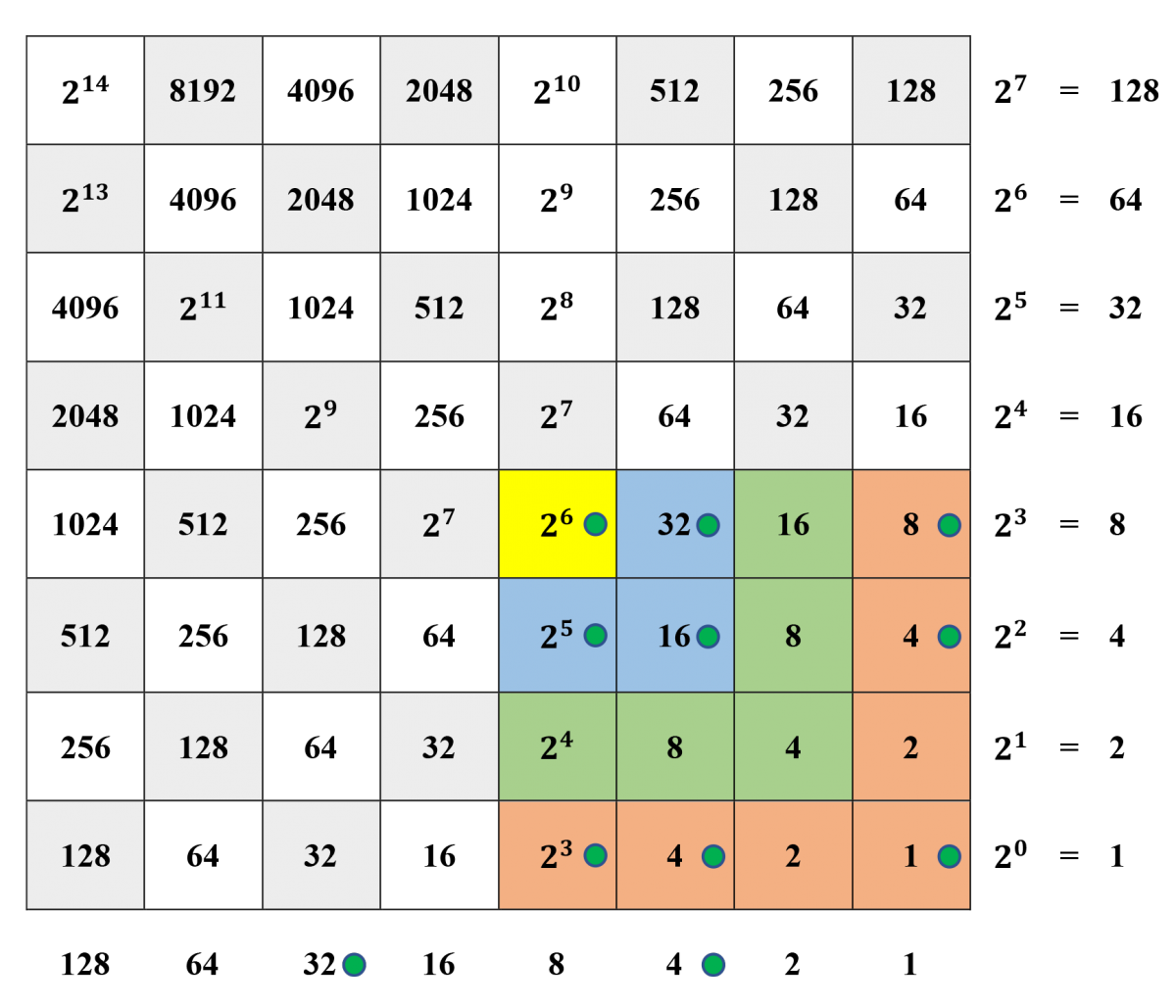
Step 5. Subtract 80 from the current value (116) along the horizontal margin, obtaining \(116 - 80 = 36,\) and replace 80 with 36 along the horizontal margin. Continue to travel down the main diagonal, searching for the first gnomon with its corner along the main diagonal in which 5 of the squares have a value less than or equal to 36. The green gnomon is next, but its 5 squares have value \(2^4 + 2^3 + 2^2 + 2^3 + 2^4 = 52,\) which is greater than 36; therefore, we cannot use it. For the orange gnomon, since \(2^3 + 2^2 + 2^0 + 2^2 + 2^3=25,\) is less than 36, we place counters on the 5 squares corresponding to this sum. (Note that \(2^3 + 2^1 + 2^0 + 2^1 + 2^3=21\) also is less than 36, but that placing counters on the corresponding squares would ruin our square, not allowing us to form a 3 x 3 square simply by removing an entire row and column. It would result in neither a geometric square nor a square value.)

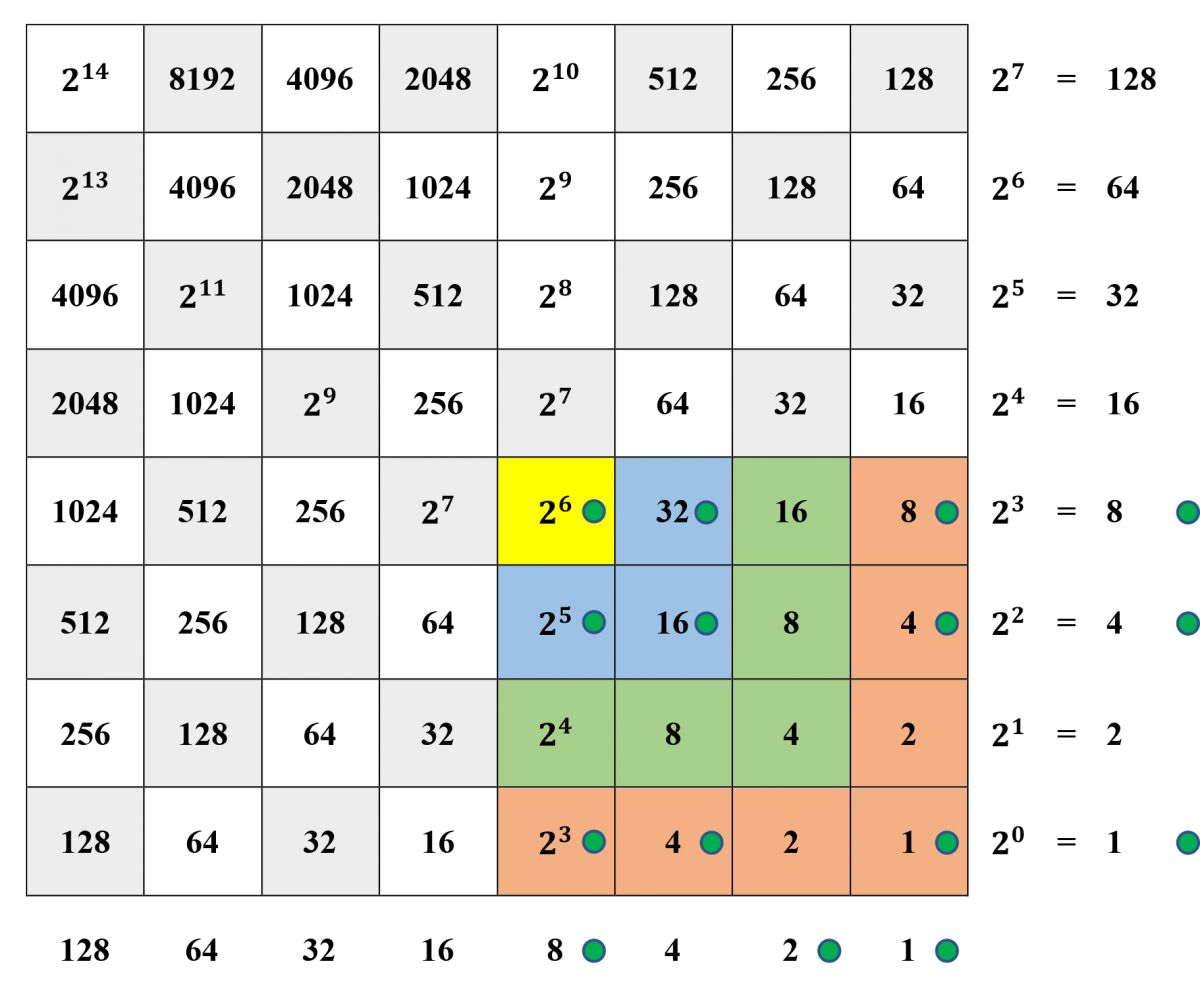
Step 6. We subtract 25 from the current value (36) along the horizontal margin, obtaining \(36 - 25 = 11,\) and replace 36 with 11 along the horizontal margin. We have reached the edge of the chessboard, and have formed a 3 x 3 square. The square root is to be found along the sides of this square. To determine this square, we place counters on the values along the right-hand (vertical) margin corresponding to the rows in which there are counters; namely, 8, 4, and 1. The highest-placed counter will always be at the end of the row containing the "head of the gnomons"; in our example, on 8 in the vertical margin. We also place counters on 4 and 1 in the vertical margin since these numbers lie at the ends of the remaining two rows in our square. Our result is that the square root of 180 has integer part 8 + 4 + 1 = 13 (vertical margin) with remainder 11 (horizontal margin).

Example:  That the square root of 121 is 11 is illustrated on Napier's binary chessboard calculator.

Step 1. Represent 121 in the horizontal margin.

Step 2. Identify \(2^6 = 64\) as the largest value along the main (NW-SE) diagonal that is less than or equal to 121. Designate the square along the main diagonal containing \(2^6 = 64\) the "head of the gnomons" and place a counter on it. (We have also colored it yellow.)

Step 3. Subtract \(2^6 = 64\) from the current value (121) along the horizontal margin, obtaining \(121 - 64 = 57\), and replace 121 with 57 along the horizontal margin.

Step 4. From the "head of the gnomons", travel down the main diagonal, searching for the first gnomon with its corner along the main diagonal in which 3 of the squares have a value less than or equal to 57. First, we try the blue gnomon with entries \(2^5, 2^4,\) and \(2^5.\) Since its value, \(2^5 + 2^4 + 2^5,\) is greater than 57, we cannot use it. For the green gnomon, since the 3 values \(2^4, 2^2,\) and \(2^4\) satisfy \(2^4 + 2^2 + 2^4\le 57,\) we place counters on the corresponding squares. (Although the squares representing \(2^3 + 2^2 + 2^3\) in this gnomon have the symmetry we require, they do not maintain the boundaries, or edges, of the square-of-gnomons and therefore would result in neither a square shape nor a square value.)

Step 5. We subtract \(2^4 + 2^2 + 2^4=36\) from the current value (57) along the horizontal margin, obtaining \(57 - 36 = 21,\) and replace 57 with 21 along the horizontal margin. We then continue to travel down the main diagonal, searching for the first gnomon in which 5 of the squares have a value less than or equal to 21. In the orange gnomon, since \(2^3 + 2^1 + 2^0 + 2^1 + 2^3\le 21,\) we place counters on the squares corresponding to this sum. (We can rule out the 5 squares that give the sum \(2^3 + 2^2 + 2^0 + 2^2 + 2^3\) for two reasons: not only is this sum greater than \(21,\) but also this option would have ruined our square – that is, it would have resulted in neither a square shape nor a square value.)

Step 6. Finally, we subtract \(2^3 + 2^1 + 2^0 + 2^1 + 2^3 = 21\) from the current value along the horizontal margin, obtaining \(21 – 21 = 0,\) and remove 21 from the horizontal margin. Since we have reached the edge of the checkerboard, we then place counters on the values along the right hand (vertical) margin corresponding to the rows in which there are counters; namely, 8, 2, and 1 For each row in our square of counters, we place a counter on the entry along the right hand (vertical) margin at the end of that row. Our result is that the integer 121 has as its square root 8 + 2 + 1 = 11 (vertical margin) with remainder 0 (horizontal margin).

The reader may wish to use the binary chessboard calculator to extract the integer part of the square root of each of 120, 170, and 225.

---

Note: For a more general history of gnomons as L-shaped parts of sundials, see Gnomon.

Conclusion

Napier's Binary Chessboard Calculator, almost completely overshadowed in Rabdologia by his Bones and Promptuary, is perhaps the earliest comprehensive, practical use of the concept of binary numbers. Here we have a calculator that can be used to add, subtract, multiply, divide, and take square roots of whole numbers.

The precedents for the use of powers of two, and the processes of doubling and halving (duplation and mediation) to accomplish multiplication can be traced to the Rhind (or Ahmes) papyrus from ancient Egypt (1650 BCE). The process was commonplace in the Middle Ages, and an interesting instance from relatively modern times is found in the so-called "Russian Peasant Multiplication" algorithm. Napier's Binary Chessboard Calculator is thus a brilliant synthesis of mathematical concepts that long preceded him, realized however with the classic simplicity inherent in commonplace objects.

The Chessboard Calculator has proven to be an excellent vehicle for teaching the binary number system and binary arithmetic, the distributive property, and place value. The first author has introduced and demonstrated Napier's Binary Chessboard Calculator as an enrichment topic to elementary and secondary teachers (at universities and at an Eisenhower Institute) and to college students in a Liberal Arts Mathematics class with great success, and to enthusiastic response. All this activity required were bingo chips and cardboard checkerboards!

In retrospect, the Chessboard Calculator seems to have been quite properly treated by Napier's peers and by history; that is, it was regarded as a curiosity and overshadowed by his other inventions. In commercial practice, where both casting counters and Napier's "bones" were readily available, it is not surprising that the Chessboard Calculator failed to find acceptance. Moreover, while more advanced practitioners of mathematics might have found the device somewhat intriguing, Napier had already given them the much more powerful tool of logarithms and ultimately the slide rule. Thus, while many examples of the Bones (and one of the Promptuary) have survived, it seems unlikely that examples of Napier's Chessboard Calculator will be found. It most likely never found common use and, if used at all, reverted back to a game board when no longer needed.

Acknowledgments

I thank my co-author, Erwin Tomash, who was a mentor and good friend to me. I spent many happy hours perusing his extensive collection of books related to the history of computer science, including many early works on computing by hand like those of Napier. I am delighted to be able to finally finish this article that we started so many years ago and I hope it honors his memory. I also thank my daughter Allison Kolpas, Associate Professor of Mathematics at West Chester University, for reviewing a late draft of the article for me.

About the Authors

By Janet Beery, Editor

Sidney J. Kolpas, a frequent contributor to Convergence, has long been interested in the history of mathematics, especially as it relates to teaching, and has been a longtime collector of historical mathematical books and objects. Kolpas holds an MS in mathematics and an EdD in mathematics curriculum and instruction. His first career, which he pursued for 19 years, was as an award-winning junior high and high school mathematics teacher in Burbank, California. His second career was as professor of mathematics at Glendale College in California from 1990 to 2011. He received many awards for his outstanding teaching during his tenure at Glendale, including the college's Distinguished Faculty Award in 2004 and the State of California's Hayward Award for Excellence in Community College Education in 2010. In 2011, he moved to Pennsylvania, and became a mathematics professor at Delaware County Community College, where in 2016 he received the college-wide Gould Award for outstanding faculty performance. He retired in 2018.

Erwin Tomash (1921-2012) was a pioneering computer scientist, helping launch the U.S. computer industry from the 1940s onward. During the 1970s he became interested in the history of computer science, and founded the Charles Babbage Society, and its research arm, the Charles Babbage Institute. The Institute, an archive and research center, is housed at the University of Minnesota. Its Erwin Tomash Library on the History of Computing began with Tomash's 2009 donation to the Institute of much of his own collection of rare books from the history of mathematics and computing. (Source: Jeffrey R. Yost, Computer Industry Pioneer: Erwin Tomash (1921-2012), IEEE Annals of the History of Computing, April-June 2013, 4-7.)

References

Ball, W. W. Rouse. A Short Account of the History of Mathematics (facsimile of 1912 5th ed.). New York: Sterling, 2001

Boyer, Carl. A History of Mathematics. New York, John Wiley, 1968.

Gardner, Martin. Negabinary Numbers Power a Chessboard Computer. Scientific American 228 (5) (May, 1973): 102-107.

Glaisher, James Whitbread Lee. Reports on the state of science. Report of the committee, consisting of Professor Cayley, F.R.S., Professor Stokes, F.R.S., Professor Sir W. Thomson, F.R.S., Professor H. J. S. Smith, F.R.S., and J. W. L. Glaisher, B.A., F.R.A.S. (Reporter), on mathematical tables. Extract from Report of the Forty-Third Meeting of the British Association for the Advancement of Science for 1873 (1874): 1-175

Glaser, Anton. History of Binary and other Nondecimal Numeration. Published by Anton Glaser, 1971.

Knott, Cargill Gilston (ed.). Napier Tercentenary Memorial Volume. London: Longmans, Green, 1925.

Kolpas, Sid. Russian Multiplication, Microprocessors, and Leibniz. MAA Convergence (March 2018).

Miller, Charles D., et al. Mathematical Ideas (13th edition). Boston: Pearson, 2016.

Napier, John (William Frank Richardson, translator). Rabdology. Cambridge, Mass.: Tomash Publishers and The MIT Press, 1990.

Napier, John. Mirifici logarithmorum canonis descriptio, 1614. (Click on the title to see a copy available via Bayerische StaatsBibliothek. Edward Wright's English translation was published in 1616.)

Napier, John. Rabdologiæ seu Numerationis per Virgulas libri duo, 1617. (Click on the title to see a copy available via Google Books. See also the entry below for Tomash, Erwin and Michael R. Williams.)

Napier, John. Mirifici logarithmorum canonis constructio, 1619 (written before the Descriptio, but published posthumously by Napier's son Robert. Click on the title to see a copy available via Google Books.)

National Council of Teacher of Mathematics. Thirty-first Yearbook, Historical Topics for the Mathematics Classroom. Washington D.C., 1969.

Smith, David Eugene. Rara Arithmetica: A Catalogue of the Arithmetics Written Before the Year MDCI with a Description of Those in the Library of George Arthur Plimpton of New York. Ginn & Co, Boston, 1908.

Tomash, Erwin. The Madrid promptuary. Annals of the History of Computing 10 (1) (January 1988): 52-67.

Tomash, Erwin and Michael R. Williams. "Napier, John (1550-1617) Rabdologiae 1617, Edinburgh", Tomash Library on the History of Computing, 2009. Available as of January 2018 from the Slide Rule Museum: www.sliderulemuseum.com/Papers/Napier_John.Rabdologiae.1617.Edinburgh.pdf

Wikipedia. Binary Number: https://en.wikipedia.org/wiki/Binary_number

For further exploration:

Erwin Tomash Library on the History of Computing descriptions of all of its works by Napier (and many other authors) are available (via the Charles Babbage Institute) here: www.cbi.umn.edu/hostedpublications/Tomash/pdf/15%20N%20chapter.pdf

A beautiful online display of the portion of the Erwin Tomash Library on the History of Computing offered for sale by Sotheby's in September of 2018 was still available as of December 2018 at http://www.sothebys.com/en/auctions/2018/the-library-of-erwin-tomash-l18409.html