Cubes, Conic Sections, and Crockett Johnson
Or: “What do the straightedge lines and compass arcs do when two parabolas and a hyperbola double a cube, just stand watching?”
In the early 1970s Crockett Johnson, author of the children’s book Harold and the Purple Crayon, sent a geometric diagram to a friend noting that the diagram “answers the question in so many minds ‘What do the straightedge lines and compass arcs do when two parabolas and a hyperbola double a cube, just stand watching?’” [Crockett Johnson to Mickey Rosenau, n.d., Rosenau Collection, Smithsonian Institution]. The diagram and the answer to this question are addressed at the end of this paper.
Cubes, Conic Sections, and Crockett Johnson - Introduction
Or, Answering “yes” to the question:
“What do the straightedge lines and compass arcs do when two parabolas and a hyperbola double a cube, just stand watching?”
In the early 1970s Crockett Johnson, author of the children’s book Harold and the Purple Crayon, sent a geometric diagram to a friend noting that the diagram “answers the question in so many minds ‘What do the straightedge lines and compass arcs do when two parabolas and a hyperbola double a cube, just stand watching?’” [Crockett Johnson to Mickey Rosenau, n.d., Rosenau Collection, Smithsonian Institution]. The diagram and the answer to this question are addressed at the end of this paper.
Introduction
Although it was written over 2,000 years ago (c. 300 BCE), Euclid’s Elements, a compilation of definitions, postulates, and propositions, serves as the basis of high school geometry courses taught today. Since constructions were utilized extensively in the Elements, we begin with a brief overview of three classical Greek construction problems that arose at least a century before Euclid. We will also explain how the author of children’s books became interested in mathematical constructions and thereby came to pose a question about cubes and conic sections.
Cubes, Conic Sections, and Crockett Johnson - Three Greek Problems
An ancient Greek problem in geometry was a very specific type of problem. Wilbur Knorr opened his book, The Ancient Tradition of Geometric Problems, with the explanation [Knorr 1986, vii]:
Within ancient geometry, a geometric ‘problem’ seeks the construction of a figure corresponding to a specific description.
Here the constructions are meant to be limited to those that can be carried out using only an unmarked ruler, usually referred to as a straightedge, and a compass. The three classical Greek problems seek
- the construction of a square with the same area as an arbitrarily given circle,
- the construction of a cube with twice the volume of an arbitrarily given cube, and
- the construction of an angle, three congruent versions of which together comprise an arbitrarily given angle.
These problems are commonly called (1) squaring a circle; (2) doubling, or duplication of, a cube; and (3) trisecting an angle. In addition to Knorr’s book, one can find a description of these three famous problems in Sir Thomas Heath’s two-volume history of Greek geometry [Heath 1981, I:218-270] and in general textbooks on the history of mathematics; for example, those by Katz [2009] or by Merzbach and Boyer [2011].
The problems of squaring a circle and doubling a cube were referenced as early as the fifth century BCE. Plutarch wrote that Anaxagoras sought to square the circle while in prison for stating the sun was not a deity. About a year before Anaxagoras’ death in 428 BCE, a severe plague erupted in Greece and it was reported that in response to the plague an oracle was offered by Apollo on the Island of Delos. The oracle advised that there would be relief from the plague if the altar at Delos was doubled, and, according to Theon of Smyrna’s Mathematics Useful for Understanding Plato, “much bewilderment fell upon the builders who sought how one was to make a solid double of a solid” [Knorr 1986, 21]. Because of this oracle, and the lack of success in doubling the Delian altar, the problem of doubling a cube is also called the “Delian problem.”
In response to a request for an explanation of the Delian oracle, Plato is reported as having [Knorr 1986, 2]
said that the god was making sport with the Greeks for their neglect of education, as it were taunting us for our ignorance and demanding that we engage in geometry, and not just as a pastime.
While Plato may have thought the Greeks did not take geometry very seriously, they took it seriously enough to classify geometric problems into solid, planar, and linear problems. Knorr quoted Pappus describing this classification of “the ancients” as [Knorr 1986, 341]
those which are capable of being solved … by means of straight line and circular arc would … be called “planar”; for the lines by means of which such problems are found … have their genesis in a plane. But those problems which are solved when there is assumed toward their discovery … one or several sections of the cone are called “solid”; for their construction … necessarily employs surfaces of solid figures, namely the conic surfaces. Yet a third kind of problem is left, the one called “linear” for there are taken for their construction lines different from those just mentioned, having a more diverse and rather contrived genesis.
Although the third classification is called linear, the term refers to a problem whose solution requires a curve that is not a straight line, a circle, or a conic. Many Greek philosophers, particularly Plato, believed that the ideal geometric construction was planar and therefore was to be drawn without the use of mechanical contrivances; i.e., the drawings were to be done with only a compass and an unmarked straightedge. Once these restrictions were more fully defined, the third problem of trisecting an angle was introduced. This problem possibly arose from attempts to construct regular polygons, which are polygons of equal sides and equal angles. In particular, if one can trisect a \(60^{\circ}\) angle then one can construct a \(20^{\circ}\) angle. Since the measure of an angle of a regular \(n\)-gon is \(\left(\frac{n-2}{n}\right)180^{\circ},\) the angle of a nonagon (a 9-sided polygon) is \(140^{\circ},\) or seven \(20^{\circ}\) angles. Therefore, copying a \(20^{\circ}\) angle makes the construction of a regular nonagon straightforward.
The three ancient problems of squaring a circle, doubling a cube, and trisecting an angle had a profound influence on mathematics. An incredibly wide range of people, from ancient Greece until modern times, have worked on these problems; among them are Archimedes, Leonardo da Vinci, René Descartes, Isaac Newton, and Carl Friedrich Gauss. As Victor Katz wrote in A History of Mathematics [Katz 2009, 40]:
The multitude of attacks on these particular problems … serve to remind us that a central goal of Greek mathematics was geometrical problem solving, and that, to a large extent, the great body of theorems found in the major extant works of Greek mathematics served as logical underpinnings for these solutions.
Cubes, Conic Sections, and Crockett Johnson - Constructions in Euclid’s Elements
Many of Euclid’s propositions in his Elements were actually not what we now think of as theorems, but were “Greek problems” as described by Knorr. In fact, such a problem appears in the very first proposition, Proposition I.1, of the Elements: “On a given finite straight line to construct an equilateral triangle” [Euclid 1956, I:241]. Euclid's justification of Proposition I.1, as translated by Heath, reads as follows [Euclid 1956, I:241-42]:
Let AB be the given finite straight line.
Thus it is required to construct an equilateral triangle on the straight line AB.
|
|
With centre A and distance AB let the circle BCD be described; [Postulate 3] |
| again, with centre B and distance BA let the circle ACE be described; [Postulate 3] | |
| and from the point C, in which the circles cut one another, to the points A, B let the straight lines CA, CB be joined. [Postulate 1] | |
| Now, since the point A is the centre of the circle CDB, AC is equal to AB. [Definition 15] | |
| Again, since the point B is the centre of the circle CAE, BC is equal to BA. [Definition 15] | |
|
But CA was also proved equal to AB; therefore each of the straight lines CA, CB is equal to AB. And things which are equal to the same thing are also equal to one another; [Common Notion 1] |
|
|
therefore CA is also equal to CB. Therefore the three straight lines CA, AB, BC are equal to one another. Therefore the triangle ABC is equilateral; and it has been constructed on the given finite straight line AB. |
|
| (Being) what it was required to do. |
It is clear that Euclid thought of propositions based on constructions somewhat differently than other propositions since they were ended with “being what it was required to do” or in the Latin translation QEF, Quod erat faciendum (that which was to be done), rather than the more well-known QED, Quod erat demonstrandum (that which was to be proven). It is also clear that the meaning of Euclid's equality of “straight lines” is what we now refer to as the equality of the lengths of line segments.
Note:
The diagram above appears in Heath's text [Euclid 1956, I:241], and was recreated using Geometer's Sketchpad.
Cubes, Conic Sections, and Crockett Johnson - Classic Greek Constructions
What tools did Euclid use in these constructions? Did he follow the tradition of the Greek philosophers, using only a compass and unmarked straightedge, or did he use a ruler with some type of markings such as centimeters, or a tool like a protractor? It is clear from the postulates and propositions in Book I that actual lengths, which would require units of measurement, were not being used, hence there would be no rulers with markings nor would there be protractors. Euclid’s Postulate 3, which hypothesizes the existence of compasses (“To describe a circle with any centre and distance” Euclid 1956, I:154), does not actually describe the instrument we now call a compass. Rather, the compass Euclid postulated lost its setting when it was picked up and is referred to as a collapsing, or collapsible, compass. If one considers the content of Propositions I.2 and I.3 (“To place at a given point (as an extremity) a straight line equal to a given straight line” and “Given two unequal straight lines, to cut off from the greater a straight line equal to the less” [Euclid 1956, I:244 and 246]), one can see that a collapsible compass can do all that a non-collapsing compass can. Thus, we can assume Euclid’s compass was non-collapsing. Heath remarked on this in his commentary on the Elements [Euclid 1956, I:246]:
Proclus alludes … to the error of those who proposed to solve [Proposition] I.2 by describing a circle with a given point as center and with a distance equal to [the given straight line], which he says is a petitio principii [begging the question]. De Morgan puts the matter very clearly… We should “insist,” he says, “here upon the restrictions imposed by the first three postulates, which do not allow a circle to be drawn with a compass-carried distance; suppose the compasses to close of themselves the moment they cease to touch the paper. These two propositions [I. 2, 3] extend the power of construction to what it would have been if all the usual power of the compasses had been assumed; they are mysterious to all who do not see that postulate iii does not ask for every use of the compasses.”
As we consider the role of constructions in the Elements, we will ask if, given a line segment of length \(1,\) is it possible to construct a segment of some other length using only a straightedge and compass? For example, given a segment of length \(1,\) can we construct segments of length \[2,\,\,{\sqrt{2}},\,\,{\rm or}\,\,\,{\sqrt[{\scriptstyle 3}]{2}}\] using only those instruments? Proposition I.2 allows us to construct a segment of length \(2\) by simply placing “at a given point [one endpoint of the given segment of length \(1\)] a straight line equal to the given straight line,” i.e., by just copying the segment of length \(1.\) To construct a segment of length \({\sqrt{2}},\) we simply construct an isosceles right triangle with legs of length \(1\) and then use the Pythagorean Theorem to show that the hypotenuse has length \({\sqrt{2}}.\) In general, we will say that a number is constructible if a segment of that length can be constructed using only a straightedge and compass.
There are other relatively simple constructions, though not necessarily the ones employed by Euclid, that illustrate arithmetical calculations. For example, given a segment of length \(1,\) it is certainly possible to construct an arbitrary positive integer by just repeated copying of segments. Also, if \(a\) and \(b\) are constructible, then \(a+b\) and \(a-b\) \((a>b)\) are constructible: we get the sum by copying a segment of length \(b\) at the end of a segment of length \(a\) and get the difference by applying Proposition I.3. The diagrams in Figure 1 (below) display methods for constructing \(ab\) and \(a/b\) for \(b\not=0.\) In each of these diagrams we find the point D by constructing a line parallel to AB through C. The calculations needed to show that \(x=ab\) and \(y=a/b,\) respectively, rely on the fact that the construction yields similar triangles so that corresponding sides are in proportion. Thus the diagram on the left gives \[{\frac{a}{1}}={\frac{a+x}{1+b}}\] and the one on the right gives \[{\frac{b}{1}}={\frac{b+a}{1+y}}.\]

Figure 1. Construction of \(ab\) and \(a/b\) from \(a\) and \(b.\) (Constructed using Geometer’s Sketchpad.)
Although it is not quite as obvious, it is not difficult to show that if \(a\) is constructible, then \({\sqrt{a}}\) is also constructible. This construction can be shown using a mean proportional, i.e., the solution to the equation \[{\frac{a}{x}}={\frac{x}{b}}\,\,\,{\rm or}\,\,\,x=\sqrt{ab}.\] It is common to see mean proportionals introduced using the diagram on the left in Figure 2 (below), where all three triangles are right triangles. This diagram can be used to prove that the three right triangles are similar and, therefore, the vertical line is the length of the mean proportional between \(a\) and \(b.\) We can form the diagram on the right by describing a circle with center the midpoint of a segment of length \(a+1.\) Since angles inscribed in semicircles are right angles, the diagram shows the mean proportional between \(a\) and \(1,\) i.e., \({\sqrt{a}}.\)

Figure 2. Construction of \(x=\sqrt{ab}\) and of \(x=\sqrt{a}.\) (Constructed using Geometer’s Sketchpad.)
Since whole numbers are constructible, any number that can be built up from a whole number using a finite number of the “operations” of \(+,\) \(-,\) \(\cdot,\) \(\div,\) and \(\sqrt{\phantom{xx}},\) is constructible. Thus, all (positive) rational numbers, and at least some positive irrational numbers, can be constructed. However, since \({\sqrt[{\scriptstyle 3}]{2}}\) is not a rational number or a finite application of square roots, it cannot be constructed in the manner we have outlined, leaving unanswered the question whether it can be constructed using only a straightedge and compass. In the seventeenth century, René Descartes, after whom the Cartesian coordinate system is named, showed that straightedge and compass constructions could be expressed algebraically in terms of the solution of iterated quadratic equations. Since François Viète had shown earlier that both trisection of an angle and duplication of a cube lead to cubic equations, Descartes concluded that neither of these problems was soluble with straightedge and compass. Although Descartes’ conclusion is correct, a rigorous proof depends on concepts that had not yet been formulated. The final proof that \({\sqrt[{\scriptstyle 3}]{2}}\) is not constructible using a compass and unmarked straightedge is generally credited to Pierre Wantzel in a paper published in 1837. Since it is necessary to construct a segment of length \({\sqrt[{\scriptstyle 3}]{2}}\) in order to duplicate a cube with edge of length \(1,\) this proof also showed that it is impossible to double an arbitrary cube with only a compass and an unmarked straightedge. In fact, none of the three Greek problems can be solved with only a compass and an unmarked straightedge.
We may now ask if there are other techniques that make the duplication of a cube possible. One answer involves conic sections but we will temporarily delay explaining how conic sections are involved and, instead, turn to the question of how an author of children’s books became interested in constructibility problems.
Cubes, Conic Sections, and Crockett Johnson - Johnson’s Introduction to Mathematics
Crockett Johnson (1906-1975), the author of the popular 1955 children’s book Harold and the Purple Crayon, was known before then for the 1940s comic strip “Barnaby” and for the illustrations in the 1945 children’s book The Carrot Seed, which was written by his wife, Ruth Krauss. Crockett Johnson was born David Johnson Leisk in New York City on October 6, 1906, and died in Norwalk, Connecticut, on July 11, 1975. Despite his death before the advent of webpages, the Crockett Johnson Homepage exists. It is maintained by Philip Nel and includes, among other things, a short biography and bibliography. Nel is also the author of a 2012 biography of Crockett Johnson and his wife Ruth Krauss and an editor of a new series of reprints of “Barnaby.”
Crockett Johnson was not a professional mathematician. In fact, he was not trained in mathematics at all. Although he drew cartoons while he was in high school, he did not become a professional cartoonist until 1934 when he published his first political cartoon for the radical news magazine New Masses. During the previous ten years, Johnson studied art at Cooper Union, a free college in New York City specializing in art, architecture, and engineering; studied typography at New York University’s School of Fine arts; worked in advertising and art editing; did free-lance designing; and “may have played semi-professional football for the Flushing Packers” (Nel 2012, 33). His first cartoon series, “The Little Man with the Eyes,” appeared weekly in Collier’s from March 1940 through January 1942. “Barnaby” first appeared in the New York newspaper PM in April 1942, shortly after the U.S. entered World War II. The comic strip has as its two main characters Barnaby, whom Harold definitely resembles, and his fairy godfather, Mr. O’Malley, who cannot be seen by the adults in the comic strip.
On May 26, 1943, mathematical symbols first appeared in the comic strip. The previous day Mr. O’Malley had introduced Barnaby to Atlas the Mental Giant, who carried a slide rule, a tool based on logarithms that was used from the late seventeenth century until the introduction of calculators for multiplication and division of large numbers. In some unexplained way, Atlas was able to use his slide rule and the mathematical symbols to produce words like O MALLEY and NO (see Figure 3). While it is possible to use mathematical expressions to produce words, it cannot be done on a slide rule, which can only produce approximate solutions to numerical problems.
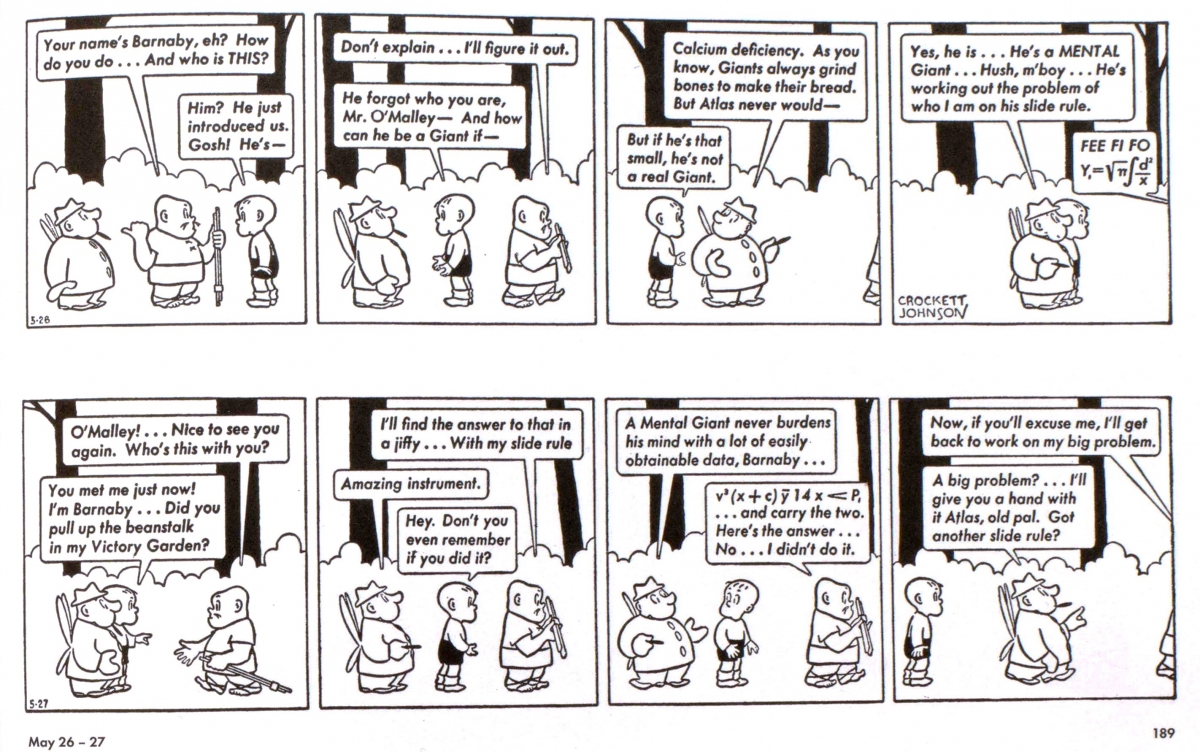
Figure 3. Barnaby, May 26 & 27, 1943 [Johnson 2013, 189]. (These comic strips are published with the permission of the Executor of the Estate of Ruth Krauss.)
The expressions that appeared in these early strips were not mathematically coherent and so they, like slide rules, could not have produced answers to the questions posed. Sometime after Atlas’ appearance in the strip, Crockett Johnson learned that strings of letters could be produced by mathematical expressions; for example, \(L^2\) can represent \(LL.\) In January 1944, Atlas reappeared using mathematical symbols in a grammatically correct way, although Johnson still misrepresented the use of slide rules.

Figure 4. Crockett Johnson's markup for Barnaby comic strip that appeared on January 27, 1944. (Reproduction courtesy of the Smithsonian Institution, image number DOR2012-8045. This comic strip is published with the permission of the Executor of the Estate of Ruth Krauss.)
While the expressions appearing in “Barnaby” were no longer nonsense, Crockett Johnson did not explain the difference to his audience, so only those who themselves knew some mathematics could evaluate the expressions in Figure 4 along with Atlas. Later in 1944 Johnson’s second collection of comic strips, Barnaby and Mr. O’Malley, appeared in book form and in that book Johnson changed the nonsense expressions he used in 1943 (Figure 5). The expressions could then be read by anyone who knew some calculus, linear algebra, and the fact that \(e^{{\pi}i}=-1.\) This experience with the language of mathematics made Crockett Johnson aware of mathematics in a way that most people are not.
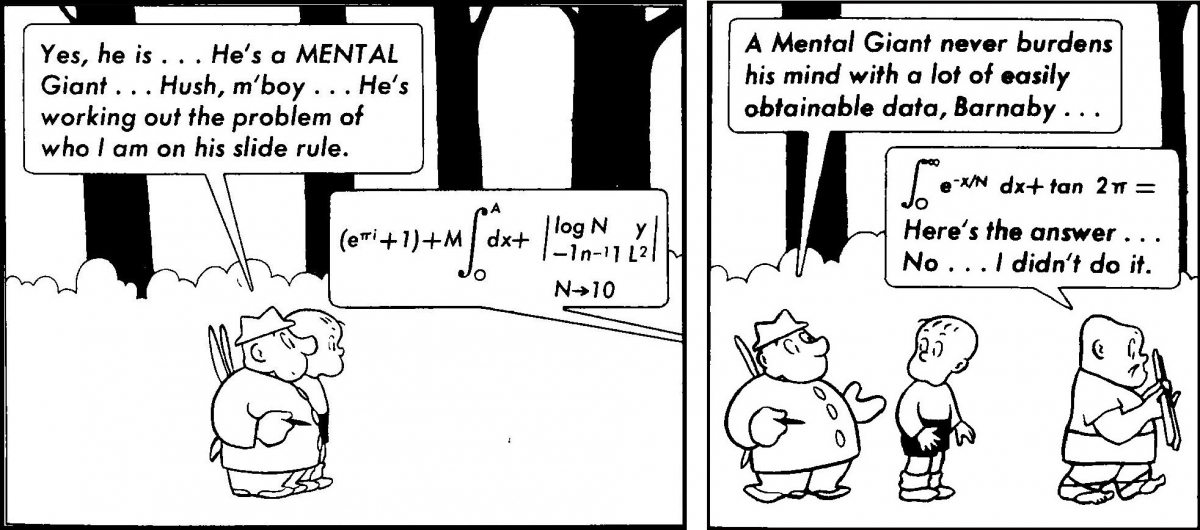
Figure 5. Rewritten panels from Barnaby strips in Figure 3 [Johnson 1944, 97 and 99]. (These panels are published with the permission of the Executor of the Estate of Ruth Krauss.)
Cubes, Conic Sections, and Crockett Johnson - Johnson’s Mathematical Paintings
In December 1965, about 20 years after he used mathematical symbols in “Barnaby,” Crockett Johnson started producing artwork based on diagrams used in the proofs of geometric theorems. On February 27, 1966, Johnson wrote to an artist friend noting that his paintings [Reinhardt Papers, Crockett Johnson Correspondence]
have an irresistible appeal to people that like coldly intellectual abstractions and warmly emotional realistic art. They comprise a series of romantic tributes to the great geometric mathematicians from Pythagoras on up.
Many of the diagrams Johnson used came from James Newman’s The World of Mathematics, a four-volume collection of essays about mathematics and mathematicians published in 1956. In addition to The World of Mathematics, Crockett Johnson acquired a collection of mathematics books, many of which were geometry books, in which he found visually interesting diagrams. He also corresponded with a number of professional and amateur mathematicians. For several years around 1970, Johnson sent diagrams accompanied by short notes about the mathematics in his paintings to a friend, Mickey Rosenau, who, unlike Johnson, had taken many mathematics courses in college. It was in such a note that Johnson posed the question that appears in the title of this paper.
In 1980, the Division of Mathematics of the National Museum of History and Technology (now the National Museum of American History) of the Smithsonian Institution obtained Johnson’s papers and 80 of his mathematical paintings, all of which appear with descriptions on the NMAH website [see Mathematical Paintings of Crockett Johnson]. Thirty-two of these paintings were included in a 1980 exhibit, “Theorems in Color,” at the museum. Some of the paintings not in the Smithsonian collection can be seen at Crockett Johnson Homepage: Paintings.
Toward the end of his life, Johnson created a number of paintings based on original constructions. These included some approximations of \(\pi\) and a construction of a regular heptagon using methods other than straightedge and compass [see Cawthorne and Green 2009]. The construction of square roots of positive integers, discussed in the "Classic Greek Constructions" section of this article, was also considered in a 1967 painting, Square Roots to Sixteen (Theodorus of Cyrene) (Figure 6), which was shown on the poster for the “Theorems in Color” exhibit. If the legs of the smallest triangle, the beginning of the “spiral” in the middle left, have length \(1,\) then the hypotenuse has length \(\sqrt{2}.\) Given that all the small legs are of length \(1,\) the hypotenuse of the adjoining triangle has length \(\sqrt{3},\) the next hypotenuse length \(\sqrt{4},\) and so on, until the hypotenuse of the last triangle is \(\sqrt{16}.\)

Figure 6. Square Roots to Sixteen (Theodorus of Cyrene) by Crockett Johnson (1967). (Courtesy of the Smithsonian Institution, image number 2008-2509.)
In 1970 and 1975 Crockett Johnson published articles about these constructions in The Mathematical Gazette, a British journal for mathematics educators. Johnson’s original constructions clearly show that he understated his mathematical abilities as he described them in his 1972 article in Leonardo, the “International Journal of the Contemporary Artist” [Johnson 1972, 99]:
I know little algebra and I avoid using it because algebra, or my ineptness with it, tends to make me lose a graphic grasp of a picture.
Johnson made this comment when referring to Problem of Delos, II (1970), a painting using one of his own constructions to solve the Delian problem. He continued as follows [Johnson 1972, 99]:
Instead, as I did in approaching the problem of the squared circle, I played with what I knew in advance to be the elements of the problem, imagining them as a construction in motion, an animated film sequence with an infinite number of frames running back and forth between plus and minus limits across the point of solution.
In the next section we return to the question posed by Crockett Johnson in the title of this paper.
Cubes, Conic Sections, and Crockett Johnson - Mean Proportionals and Doubling a Cube
As described by Eratosthenes [Knorr 1986, 23],
Hippocrates of Chios was first to come up with the idea that if one could take two mean proportionals in continued proportion between two lines, of which the greater is double the smaller, then the cube will be doubled. Thus he turned one puzzle into another one, no less of a puzzle.
It may not be clear, however, how considering the equivalent problem of “two mean proportionals” will lead to a solution of doubling a cube; i.e., given a cube with edges of length \(a\) and volume \(a^3,\) how can we construct a cube with edges of length \(\sqrt[{\scriptstyle 3}]{2a^3}=a{\sqrt[{\scriptstyle 3}]{2}}\) and volume \(2a^3\)? We saw above that a mean proportional, or geometric mean, is the solution to the equation \[{\frac{a}{x}}={\frac{x}{b}},\] i.e. \(x=\sqrt{ab}.\) Two mean proportionals are the solutions to the three equations \[{\frac{a}{x}}={\frac{x}{y}},\,\,{\frac{x}{y}}={\frac{y}{b}},\,\,{\rm{and}}\,\,{\frac{a}{x}}={\frac{y}{b}},\] which can be written more compactly as \[{\frac{a}{x}}={\frac{x}{y}}={\frac{y}{b}}.\] If \(a\) and \(b\) are taken to be the lines mentioned in Eratosthenes’ account, “of which the greater is double the smaller,” then \(b = 2a\) and the equations can be written \[{\frac{a}{x}}={\frac{x}{y}}={\frac{y}{2a}}.\]
Once again we can discuss the solutions in terms of geometric means. In general the geometric mean of \(n\) numbers is the \(n\)th root of the product of the \(n\) numbers, so the geometric mean of \(a, b,\) and \(c\) is \(\sqrt[{\scriptstyle 3}]{abc},\) which is the length of the edge of a cube with the same volume as a rectangular prism with edges of length \(a, b,\) and \(c.\) If we consider a square-based rectangular prism with edges of length \(a, a,\) and \(2a,\) then to find the length of the edge of a cube with volume \(2a^3,\) we need to find the geometric mean of \(x^3=2a^3\) which is \({\sqrt[{\scriptstyle 3}]{2a^3}}\) or \(a{\sqrt[{\scriptstyle 3}]{2}}.\) This is the smaller of the two mean proportionals of \(a\) and \(2a.\) (The larger, \({a\sqrt[{\scriptstyle 3}]{4}},\) is the length of an edge of a cube of volume \(4a^3.\)) Thus it is natural to assume that \(b=2a\) when doubling a cube.
In addition to a geometric interpretation, if we look again at \[{\frac{a}{x}}={\frac{x}{y}}={\frac{y}{2a}}\] as three separate equations, we discover that they define two parabolas, \(x^2=ay\) and \(y^2=2ax\) and a hyperbola \(xy=2a^2.\) Menaechmus, who was mathematically active in the middle of the fourth century BCE, is credited with discovering conic sections while considering mean proportionals. In a History of Greek Mathematics, Heath wrote [Heath 1981, I:251],
Two solutions by Menaechmus of the problem of finding two mean proportionals are described by Eutocius; both find a certain point as the intersection between two conics, in the one case two parabolas, in the other a parabola and a rectangular hyperbola. The solutions are referred to in Eratosthenes’ epigram: “do not”, says Eratosthenes, “cut the cone in the triads of Menaechmus.” From the solutions coupled with this remark it is inferred that Menaechmus was the discoverer of the conic sections.
More recently, Knorr conjectured that Menaechmus did not actually use conic sections but, rather, that he could have constructed curves in a pointwise fashion. Specifically Knorr proposed “that Menaechmus based his solution on curves defined with respect to second-order relations among the mean proportional lines” [Knorr 1986, 65]. The series of diagrams he showed to support his conjecture [ibid., 64-65] are much more complicated than those used by Crockett Johnson that we describe below. Gary Stoudt also discussed the question of how Menaechmus arrived at a solution in “Menaechmus’ Constructions” and “Why Do Menaechmus’ Constructions Work?,” the first two sections of his 2004 Convergence paper “Can You Really Derive Conic Formulae from a Cone?”
Euclid, who lived about fifty years after Menaechmus, discussed in his Elements mean proportionals in the context of cubic numbers such as \(1, 8, 27, 64,\) etc. The statement of Proposition 12 of Euclid’s Book VIII, begins “Between two cube numbers there are two mean proportional numbers” [Euclid 1956, II:364]. Heath’s commentary following the proof of that proposition notes that “The cube numbers \(a^3,\) \(b^3\) being given, Euclid forms the products \(a^2b,\) \(ab^2\) and then proves” that these are the two mean proportional numbers between \(a^3\) and \(b^3\) [ibid., 365]. Thus Euclid had found solutions to equations equivalent to ours; i.e., \[{\frac{a^3}{x^3}}={\frac{x^3}{y^3}}={\frac{y^3}{b^3}}\] so that \(x^3=a^2b\) and \(y^3=ab^2.\) In other words, Euclid found that \({\sqrt[{\scriptstyle 3}]{a^2b}}\) and \({\sqrt[{\scriptstyle 3}]{2}}\) are the two mean proportionals between \(a\) and \(b.\) We, too, could have found these solutions if we first solved the three equations to get \(x^2=ay,\) \(y^2=bx,\) and \(xy=ab.\)
Cubes, Conic Sections, and Crockett Johnson - Conic Sections and Doubling a Cube

Figure 7. Two parabolas meeting a hyperbola at the point \((s,t).\) (Constructed using Geometer’s Sketchpad.)
Given a cube with edge of length \(a,\) how do we now utilize conic sections to double that cube; i.e., how do we find \(x\) so that \(x^3=2a^3\)? Consider the point of intersection, \((s,t),\) in Figure 7 above. The curves in this figure represent the parabolas \(x^2=ay\) and \(y^2=2ax,\) together with the hyperbola \(xy=2a^2;\) i.e., the graphs of the functions \[y={\frac{x^2}{a}}, {y=\sqrt{2ax}},\,\,{\rm{and}}\,\,{y={\frac{2a^2}{x}}},\] for \(x>0.\) The equation of the first function tells us that \(t={{s^2}/{a}},\) so that the equation of the second parabola becomes \[{\left({\frac{s^2}{a}}\right)}^2={2as},\,\,{\rm or}\,\,\,s^3=2a^3.\] Thus the \(x\)-coordinate of the point of intersection, \(s,\) is the length of the edge of a cube which is double the volume of the original cube with edge of length \(a.\) If we look at the cube of the \(y\)-coordinate of the point we find \[t^3={\frac{s^6}{a^3}}={\frac{(s^3)^2}{a^3}}={\frac{4a^6}{ a^3}}=4a^3\] so the \(y\)-coordinate is the length of the edge of a cube which is quadruple the volume of the original cube, or the larger of the mean proportionals mentioned previously.
Cubes, Conic Sections, and Crockett Johnson - Problem of Delos (Menaechmus)

Figure 8. Problem of Delos (Menaechmus), by Crockett Johnson (1968). (Courtesy of Philip Nel.)
Answering the question: “What do the straightedge lines and compass arcs do when two parabolas and a hyperbola double a cube, just stand watching?”
As we can see in Figure 9, Crockett Johnson used the diagram in Figure 7 to produce a wonderful visualization of the two parabolas and a hyperbola doubling a cube in his painting The Problem of Delos (Menaechmus) (1968), shown in Figure 8. If the side of the small square in the lower left-hand corner of this painting, outlined in blue in Figure 9, is considered to have length \(1,\) i.e. \(a=1,\) then the larger square, sharing its lower left hand corner, has sides of length \(\sqrt[{\scriptstyle 3}]{2},\) the necessary length to construct a cube of volume \(2,\) thus doubling a unit cube! Note also that the rectangle that shares its base with the larger square has width \(\sqrt[{\scriptstyle 3}]{2}\) and height \({\sqrt[{\scriptstyle 3}]{2}}^2\) or \(2^{2/3}.\) Thus, by superimposing Crockett Johnson's painting with the unlabeled graphs of Figure 7, one can understand why the answer to the question he posed is yes!

Figure 9. Problem of Delos (Menaechmus), by Crockett Johnson (1968), with the unlabeled graphs from Figure 7 superimposed on the painting from Figure 8. The square outlined in blue has side length \(1.\)
Cubes, Conic Sections, and Crockett Johnson - Note from Johnson to Mickey Rosenau

Figure 10. Note from Crockett Johnson (David Johnson Leisk) to Mickey Rosenau. (Courtesy of the Smithsonian Institution, image number AHB2013q009103.)
The note in Figure 10 was written after Johnson created the painting, The Problem of Delos (Menaechmus), shown in Figure 8 on the preceding page. It was probably written in 1970, as the “Cambridge math mag [the Mathematical Gazette] that published [his] squared circle” did so that year. The “math mag” did not publish the item he referred to in the note, but around that time Johnson began working on an article he called “Geometric Geometric Paintings” [Note 1] that was to consist of discussions of twelve of his paintings. In 1972 Johnson published a shorter version of this article, “On the Mathematics of Geometry in My Abstract Paintings,” in the journal Leonardo. The first diagram mentioned in the note of Figure 10 and shown in Figure 11 is not related to cube roots but is one on which he based his painting, Division of a One-by-Two Rectangle by Conic Rectangles, one of the seven paintings discussed in the Leonardo article.
The second diagram, however, is related to cube roots since Johnson noted that “\(AJ/AB = 1.25992\dots = \sqrt[{\bf 3}]{2}.\)” Furthermore, it appears in early drafts of the section, “FACE OF CUBE AND A SQUARE EQUALLING ITS VOLUME (Artist’s Construction)” [Note 2]. Although we cannot be sure, it seems likely that Johnson used an approximation of his listed value of AB, \(1.28423\dots,\) to place point B since he used approximations to place similar points in other constructions found in drafts of “Geometric Geometric Paintings.” In other words, without the aid of a mark on the straightedge, the compass and straightedge of the classic Greek constructions cannot determine the cube root of two and, therefore, can only stand watching as the two parabolas and hyperbola easily double the cube.
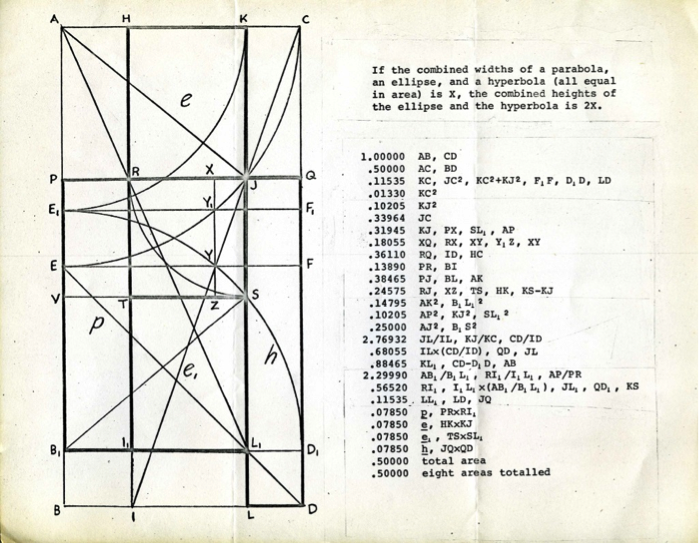
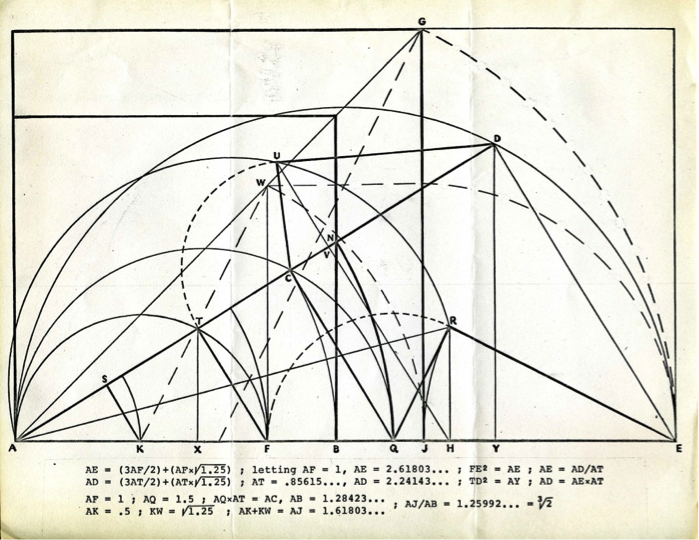
Figure 11. The two diagrams accompanying Johnson's note to Rosenau in Figure 10. (Courtesy of the Smithsonian Institution, image numbers AHB2013q009104 and AHB2013q009105.)
Notes:
1) In her 2013 article on his paintings, Peggy Kidwell made the point that his explanation of this title, specifically its redundancy, “well express[es] the distinction between his use of mathematics and that of contemporary painters” [p. 207].
2) No painting with this name appears on either a 1980 list of Johnson’s paintings or the list on the Crockett Johnson Homepage. A painting with a similar name, Cube and Square of Equal Value (Artist's construction), appears on the 1980 list, although without a photograph.
Cubes, Conic Sections, and Crockett Johnson - Conclusion and About the Authors
Conclusion
For thousands of years, construction problems have captivated the imaginations of both professional and amateur mathematicians and, because of this interest, significant contributions to mathematics have been made while attempting to solve these problems. Many of the propositions in Euclid’s Elements are actually construction problems. As we have seen, conic sections arise in attempts to solve the early Greek construction problem we know as duplication of a cube. Continued fascination with constructions, and specifically with the duplication of a cube, gave amateur mathematician and professional children's author Crockett Johnson inspiration for many of his mathematical paintings. Along the way, he framed the question “What do the straightedge lines and compass arcs do when two parabolas and a hyperbola double a cube, just stand watching?”
To see images of 80 mathematical paintings by Crockett Johnson, visit Mathematical Paintings of Crockett Johnson, an online exhibit by the Smithsonian Institution's National Museum of American History.
To learn more about Crockett Johnson visit the Crockett Johnson Homepage.
About the Authors
Stephanie Cawthorne received a 1992 B.S. from Eastern Nazarene College, and a 1998 Ph.D. from the University of Maryland under the direction of David Kueker. After spending the first part of her career at Marymount University in Arlington, VA, she is now Professor of Mathematics at Trevecca Nazarene University. Her initial research was in mathematical logic in the area of model theory and, through the influence of Judy Green, she has become interested in the history of mathematics and the works of Crockett Johnson.
Judy Green received a 1964 B.A. from Cornell University, a 1966 M.A. from Yale University, and a 1972 Ph.D. from the University of Maryland under the direction of Carol R. Karp. She is now Professor Emeritus of Mathematics having spent the first half of her career at Rutgers University in Camden, NJ, and the second half at Marymount University in Arlington, VA. Her early papers are in mathematical logic, the field of her dissertation, but she soon switched her research interests to the history of women in mathematics. She first learned of the mathematical paintings of Crockett Johnson during a 1980 sabbatical spent studying the historiography of mathematics with Uta C. Merzbach at the Smithsonian Institution.
Cubes, Conic Sections, and Crockett Johnson - References
Cawthorne, Stephanie and Judy Green. 2009. “Harold and the Purple Heptagon.” Math Horizons 17 (September): 5-9.
Euclid. 1956. The Thirteen Books of Euclid’s Elements: Translated with introduction and commentary by Sir Thomas L. Heath. 2nd ed. New York: Dover Publications. First ed. 1908; Cambridge: The University Press. Also 2002: Sante Fe, NM: Green Line Press, The Thirteen Books of Euclid’s Elements: all thirteen books in one volume.
Heath, Sir Thomas. 1981. A History of Greek Mathematics, 2 vols. New York: Dover Publications. Reprint of 1921 ed.
Johnson, Crockett. 1944. Barnaby and Mr. O’Malley. New York: Henry Holt.
———. 1970. “A Geometrical Look at \(\sqrt{\pi}.\)” Mathematical Gazette 54 no. 387: 59-60.
———. 1972. “On the Mathematics of Geometry in My Abstract Paintings.” Leonardo 5: 97-101.
———. 1975. “A Construction for a Regular Heptagon.” Mathematical Gazette 59 no. 407: 17-21.
———. 1985. Barnaby #2: Mr. O’Malley and the Haunted House. New York: Ballantine Books.
———. 1986. Barnaby #3: Jackeen J. Mr. O’Malley for Congress. New York: Ballantine Books.
———. 2013. Barnaby Volume 1: 1942-1943. Edited by Philip Nel and Eric Reynolds. Seattle: Fantagraphics Books.
Katz, Victor J. 2009. A History of Mathematics, An Introduction, 3rd ed. Pearson.
Kidwell, Peggy Aldrich. 2013. “The Mathematical Paintings of Crockett Johnson, 1965-1975: An Amateur and His Sources.” In Analyzing Art and Aesthetics, edited by Anne Collins Goodyear and Margaret A. Weitekamp, 198-211. Washington, DC: Smithsonian Institution Scholarly Press.
Knorr, Wilbur R. 1986. The Ancient Tradition of Geometric Problems. Boston: Birkhäuser. Unabridged, corrected republication 1993: New York: Dover Publications.
Mathematical Paintings of Crockett Johnson. Object Group. Smithsonian Institution, National Museum of American History.
Merzbach, Uta C. and Carl B. Boyer. 2011. A History of Mathematics, 3rd ed. Hoboken, NJ: John Wiley.
Nel, Philip. 2012. Crockett Johnson and Ruth Krauss: How an Unlikely Couple Found Love, Dodged the FBI, and Transformed Children’s Literature. Jackson: University Press of Mississippi.
Newman, James R. 1956. The World of Mathematics: A small library of the literature of mathematics from A’h-mosé the Scribe to Albert Einstein. New York: Simon and Schuster. Reprint 2000: Mineola, NY: Dover Publications.
Ad Reinhardt Papers. Smithsonian Institution, Archives of American Art.
Stoudt, Gary S. 2004. “Can You Really Derive Conic Formulae from a Cone?” Convergence, vol. 1.
Stroud, J. B. 2008. “Crockett Johnson’s Geometric Paintings.” Journal of Mathematics and the Arts 2 no. 2: 77-99.
Rosenau, Milton D., Jr., Collection. Smithsonian Institution, National Museum of American History, Division of Science and Medicine: Mathematics.
Yates, Robert C. 1971. The Trisection Problem. The National Council of Teachers of Mathematics.
