Background

Fifty years ago, when I first viewed the contents of the Chinese mathematical classic, Jiuzhang suanshu [Nine Chapters on the Mathematical Art] (ca. 100 BCE), I was impressed by the scope and depth of its mathematics and confounded that a work of such caliber remained almost completely unrecognized in the western world for so long. By incorporating the number “Nine” into the title of this work, the unknown author or authors were indicating that they thought the work to be comprehensive of the mathematical knowledge possessed at their time. In the numerology of ancient China, nine indicated completeness. The Nine Chapters show that Chinese mathematicians of this early period possessed an advanced decimal, place-value, system of numeration; recognized negative numbers; performed fractional operations with great proficiency; extracted roots of numbers; possessed correct area and volume formulae for a variety of area and volume computations; employed a “matrix” technique of computation for solving systems of linear equations; developed a high proficiency in applying “the rule of three” in solution schemes; and could perform geometric solutions involving applications of the “Pythagorean Theorem.” Further, the book’s well-organized collection of 246 mathematical problems distinguishes it, in my opinion, as the most comprehensive, extant testament to early problem solving. The Jiuzhang, within its nine chapters, had a story to tell and I sought to unravel, understand, and learn from it. (Since this initial encounter, an English language translation of the Nine Chapters has appeared [8].)

In my examination, I was particularly attracted to several mathematical features of this work: the Chinese algebraic versatility in applying the rule of three (Chapter 2, Millet and Rice); their matrix-like solution techniques for solving systems of linear equations, including those resulting in indeterminate solutions (Chapter 8, Rectangular Arrays); and their facility with right triangle applications (Chapter 9, Right Triangles). Assisted by a Chinese language translator, T. I. Kao, I ventured to obtain an English language version of the ninth chapter, Gougu [Right Triangles], a treatise in itself consisting of 24 problems involving right triangle theory and its applications to surveying and distance reckoning exercises. This effort, including my analysis and conclusions, was eventually published for a broader reading audience [12]. Whereas the Jiuzhang contains a compilation of Chinese mathematical practice actually employed centuries before the appearance of the book, it was apparent to me that the Chinese knowledge of the “Pythagorean Theorem” was developed and perfected independently, without foreign influences. Indeed the Chinese possessed an indigenous name for this relationship, the “Gougu rule,” and derived a geometric-algebraic proof, the Xian thu, for its validity. This is illustrated in Figure 1, below.

In the Chinese mathematical terminology of this time: gu was the longer leg of a right triangle; gou was the shorter leg. The Chinese characters for these terms were originally used to designate “thigh” and “leg.” The hypotenuse of the triangle was referred to as xian, which in a nonmathematical context referred to a string tightly strung between two points, such as a lute string. This analogy provides a clue as to how ancient peoples physically measured the length of an hypotenuse. The Xian thu, or hypotenuse diagram, shows a large square composed of four congruent “3-4-5” right triangles and a small center square set against a grid system. As depicted in the lefthand diagram in Figure 1, the side of the large square is an hypotenuse of measure \(5,\) therefore the area of the square is \(25\) square units, \(5^2.\) Now if the Xian thu is dissected and its regions rearranged into two smaller squares, as shown in the righthand diagram in Figure 1, it is seen that that the sum of their areas is \(3^2 + 4^2 = 25.\) This demonstration was generalized to prove that \[{\textit{gu}}^2 + {\textit{gou}}^2 = {\textit{xian}}^2,\] known as the “Gougu rule.”

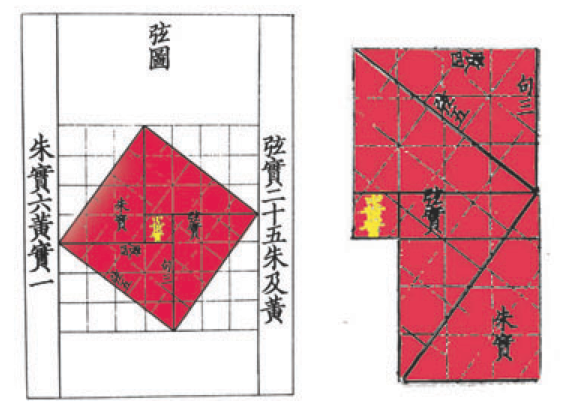
Figure 1. The Xian thu, or hypotenuse diagram, can be dissected to demonstrate the “Gougu rule,” or, as it is known in the West, the Pythagorean Theorem.

The concept of the right triangle was even embedded in Chinese myths on the origins of mathematics, as evident in a conversation in the Zhoubi suanjing [Mathematical Classic of the Zhou Gnomon], another extant second century BCE mathematical and astronomical classic [2]. In a fanciful conversation between Zhou Gong, a duke of the Zhou dynasty (ca. 1030-221 BCE), and the Grand Prefect Shang Gao, the Duke queried the old man endeavoring to learn the secrets of mathematics. Complimenting the Grand Prefect on his knowledge of mathematics, he inquired how the legendary patron of all Chinese knowledge, Fuxi (ca. 3000 BCE), could possibly obtain a mathematical understanding of the universe since “there are no steps to ascend the heavens and the earth is not measurable with a foot rule.”

The Prefect responded: “The art of numbering [mathematics] proceeds from the circle and the square.” (This statement may be purely metaphorical, as, for the ancient Chinese, the earth was symbolically represented by a square and the universe by a circle; thus perhaps Shang’s words were implying that mathematics is acquired from the physical realm.) He then continued: “The circle is derived from the square and the square from the rectangle.” [Here, “rectangle” is literally “the carpenter’s L-shaped square” or “set-square.”] His explanation proceeded, further elaborating on the importance of the right angle and included a Xian thu derivation [literally, “the hypotenuse diagram”] for the Gougu rule (see Figure 1, above).

		Alignment	Height	Depth	Distance

Figure 2. Uses of the set-square included forming right angles to align structures, and measuring heights, depths, and distances.

Zhou responded: “Great indeed is the art of numbering [mathematics]. I would like to ask the Dao [way, or method] of the use of the right-angled triangle [set-square].” To which Shang explained that the set-square placed on the ground helps to lay out structures, the set-square set on edge could be employed to observe heights, and reversed it could serve “to fathom depths.” Laid flat on the ground, the set-square was used for ascertaining distances, and rotated it could prescribe circles. See Figure 2, above. The Prefect ended his instruction by summarizing [6]:

He who understands the earth is a wise man, and he who understands the heavens is a sage. Knowledge is derived from the straight line. The straight line is derived from the right angle. And the combination of the right angle with numbers is what guides and rules the ten thousand things [meaning “everything”].

Such a prolonged discussion focused on the importance of the right triangle and its concrete template, the set-square, affirms the scientific and mathematical importance of right angled theory for the Early Chinese Empire. Reverence for the right triangle and the set square is further reinforced by a depiction on a tomb dated to the Han dynasty (ca. 200 BCE) showing Fuxi passing on his instrument of power and knowledge, the set square, to his consort, Nuwa; this divine couple are credited by the Chinese for bringing order to the universe. See Figure 3, below.

Figure 3. A tomb dated to the Han dynasty (ca. 200 BCE) shows the divine couple, Nuwa and Fuxi, sharing an instrument of power and knowledge, the set square.

In reflecting on these passages, the obvious, but often unrecognized, appreciation and utilization of the phenomena of perpendicularity by ancient peoples becomes obvious. Most likely, a primary mathematical instrument employed by humans was a simple vertical staff embedded into the ground, a gnomon. The actual placement of the staff would imitate the observed efficiency of nature such as a tree rising perpendicular to the plane of the ground. The staff would be placed perpendicular to the ground, it would “stand straight,” and the concept of vertical to horizontal would be preserved. In 1973, Jacob Bronowski, mathematician and noted philosopher of science, in writing on the place of science in human development, commented on how perpendicularity and its mathematical expression as contained in the “Pythagorean Theorem” described “the exact laws that bind the universe” and noted that its inscription had, appropriately, been considered for use in a message on a NASA Pioneer space probe as a topic of communication to other possible intelligent beings in the universe [1]. It appears, however, that it was never used.

Eventually, humans would design other tools to readily obtain this relationship: the plumb line for insuring the vertical and the surface level for the horizontal. The right angle itself would be captured in the “L” shaped design of a set-square. The use of two such sighting staffs set a distance apart allowed for the construction of straight lines. Sighting on such gnomons would eventually advance in their focus from near points to distant points, one perhaps elevated, and a slanted line of sight would be required and obtain significance. Now, a slanted sight line would allow the positional referencing of heavenly bodies, including the sun. A primitive astronomy was established and an intuitive concept of angle obtained. As a body higher in the sky was observed using a gnomon, the ray of the line of sight increased its upward slant and the angle with the horizontal increased. For the sun, this reckoning involved a shadow, a length easily observed and recorded. Such visual reckonings became important as temporal markers for the needs of agricultural societies and those that depended on the migrations of animal herds. Ancient societies kept chronological “shadow tables” [2, p. 196]. Pliny the Elder (23-79 CE), in his encyclopedic history of the ancient world, mentioned Thales employing shadow reckoning in Egypt. Plutarch, writing a few years later, also repeated this incident [7]:

Although he [the Pharaoh of Egypt] admired you [Thales] for other things, yet he particularly liked the manner by which you measured the height of the pyramid without any trouble or instrument; for, by merely placing your staff at the extremity of the shadow which the pyramid casts, you formed, by the impact of the sun’s rays, two triangles and so showed that the height of the pyramid was to the length of the staff in the same ratio as their respective shadows.

Evidence of such shadow reckoning can be found in most ancient societies. Shadow reckoning employing gnomons became a mathematical technique that embedded itself in the later designs of such instruments, such as the sun dial, astrolabe, quadrant, and eventually the nautical sextant. The ratio of gnomon length to its shadow length is now recognized as the cotangent of the intercepted angle. Indeed, a strong case can be made for shadow reckoning as the precursor of trigonometry [10].

My Misconception

Thus, more firmly impressed with the societal importance of perpendicularity as determined by a simple right triangle, I attempted to unravel the information contained in the ninth chapter, the Gougu chapter, of the Jiuzhang. My efforts in this task are best explained by taking the reader through my analysis and solution of some actual examples of problems from the text. First, one has to appreciate the form and style of ancient Chinese mathematics problems: a problem is stated, the answer given, and a brief, very concise explanation offered. The explanation assumes that its reader is familiar with the computing practices and conventions implied. Chinese mathematicians of this period would do all calculations with a set of computing rods. Calculations were performed rapidly and mechanically. As was the custom, later commentators could append their own explanations to problems. For example, the seventeenth problem of the sequence of problems given in the ninth chapter [Jiuzhang 9:17] in translation is stated as follows:

A square walled city measures 200 bu on each side. Gates are located at the center of all sides. If there is a tree 15 bu from the eastern gate, how far must a person travel out of the south gate to see the tree?

Answer: 666 2/3 bu

Method: The answer will be the quotient found by using the 15 bu as a denominator and half the width of the city squared as the numerator.

Approaching the problem situation as would a competent secondary school student, I first drew a diagram depicting the situation. Then, in a modern manner, I labeled all parts of the diagram and, as conditioned, designated the required side ED as the unknown “x”. See Figure 4.

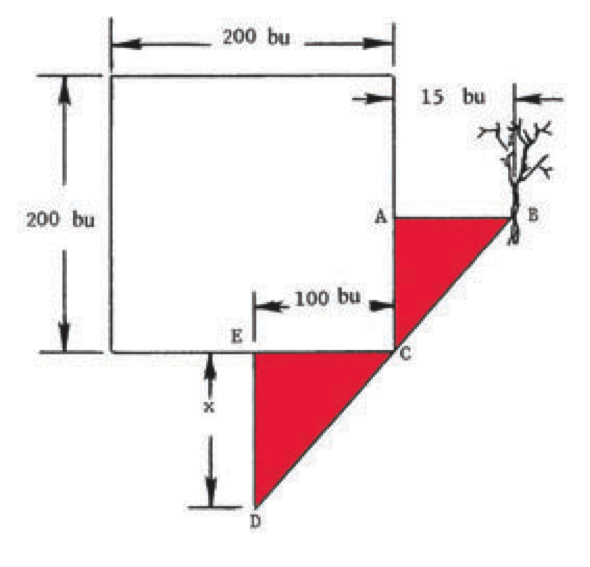
Figure 4. Diagram for Jiuzhang 9:17, assuming a solution by similar triangles

Immediately I recognized the similarity of the two relevant triangles, ABC and ECD, and this determined my solution strategy. Employing the proportionality relationship between the corresponding sides of the similar triangles, ED/EC=AC/AB, and substituting in the given information, I arrived at:

\[\frac{x}{100} = \frac{100}{15}\rightarrow x=\frac{100^2}{15}\rightarrow x=666\frac{2}{3}.\]

I obtained the correct answer and my procedure satisfied the given Jiuzhang method.

Another, more complex problem, the twenty third [Jiuzhang 9:23], is:

A hill lies west of a tree whose height is 95 chi. The distance between the tree and the hill is known to be 53 li. A man 7 chi tall stands 3 li east of the tree. If the tops of the hill and tree are aligned in the path of his vision, what is the height of the hill?

Answer: 1649 chi, 6 33/50 cun

Method: Obtain the height of the tree minus the height of the man. Multiply this difference by 53 chi and divide by 3; the result plus 95 chi gives the desired answer.

[Note: In the metrological system of this period, distance was measured as: li (mile) = 300 bu, bu (pace) = 5 chi, and chi (foot) = 10 cun.]

Once again, I drew a diagram; see Figure 5. This time, allowing for some artistic license, I embellished the diagram with a drawing of a tree and hill. Labeling all known entities as given, I allowed the height of the hill to be EF, where EF = (ED + CG).

Figure 5. Diagram for Jiuzhang 9:23, assuming a solution by similar triangles

Again, upon inspection, two similar triangles, ABC and CDE, provided a proportional relationship:

ED/DC = CB/BA → ED = (DC)(CB)/BA.

Substituting in the given values, where CB = (CG – AH), I arrived at:

ED = (53)(95–7)/3 = 1554.666 and EF = 1554.666 + 95 = 1649.666 or 1649 chi, 33/50 cun.            

Once again the desired answer followed the indicated traditional method. And so it went for all the problems in Jiuzhang, Chapter 9.

From this experience, I surmised the ancient Chinese mathematicians recognized the similarity of triangles, appreciated their proportionality of respective sides, and on this basis set up appropriate proportions to obtain their desired results. Over time, the use of these proportions resulted in their discovery of the more general “rule of three” – that is, given four quantities, a, b, c and d that are in the proportional relationship a/b = c/d, then if three of the quantities are known, the remaining quantity may be found by the proportion. They then applied “the rule of three” to a wider selection of problems. See Figure 6.

Figure 6. Similar triangles ABC and ADE yield BC/CA = DE/EA.

In Figure 6, above, similar triangles yield BC/CA = DE/EA. Therefore, if BC = a, CA = b, DE = c, and EA = d, then a/b = c/d.

This scenario – that such a numerical relationship as the “rule of three” would first be appreciated visually and physically in a geometric situation before being conceptually assimilated – appealed to my sense of logic and historical perspective. Early Chinese mathematicians were known to draw diagrams on paper, cut them up, move the regions around, experiment with them, and seek out new geometric-algebraic relationships as realized in the Xian thu demonstration. The simple but powerful algebraic technique, “the rule of three,” also known over time by many other names, such as “the Golden Rule” or “the Merchant’s Rule,” eventually became a valued computational tool used in all societies. The rule’s origin has historically been attributed to Asian sources [9]; now, to me, it appeared to be definitely Chinese. I thought that I had made a discovery: the Chinese through their recognition of the similarity of triangles and the employment of its properties had developed the “rule of three.” I had found a mathematical link in the chain of cause and effect!

The Epiphany

But yet my theory was just an hypothesis; I needed further verification. I pressed on, seeking out more Chinese problem solving situations involving right triangles. In the year 263, the scholar-official and greatest mathematician of ancient China, Liu Hui, of the Kingdom of Wei (220-280 CE), wrote a commentary on the Jiuzhang in which he supplied theoretical verifications for many of the solution procedures, corrected mistakes, and enriched the text with his own mathematical contributions.

Figure 7. Liu Hui wrote an extensive and important commentary on the Jiuzhang in 263 CE. (Source: Convergence Portrait Gallery)

Liu felt that the ninth chapter of the Jiuzhang was weak in its considerations of obtaining inaccessible distances and he extended it by adding nine new problems. (Note again that magic number nine.) All of these problems involved the use of two gnomons and were solved using a procedure called chong cha or “double differences.” Indeed, these problems were more complicated, but again, in my working of them, I recognized pairs of similar triangles from which sets of proportions could be developed and the answers obtained [11]. However, a nagging doubt began to arise: If the Chinese recognized the similarity of right triangles, why didn’t they generalize the concept of similarity to other geometric figures?

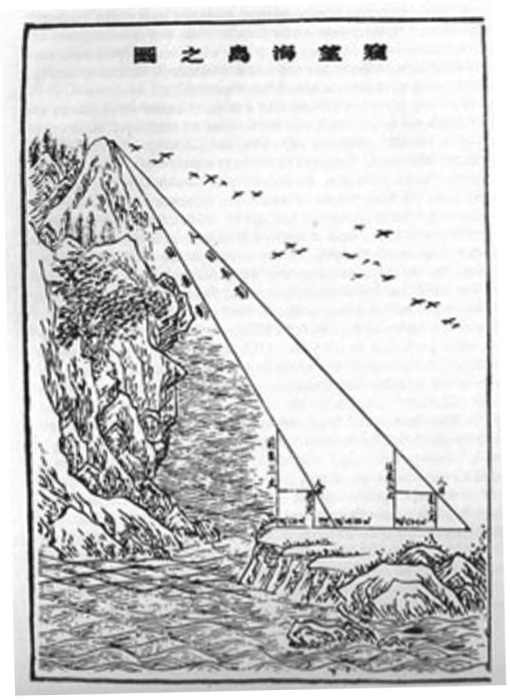
Figure 8. A technique for measuring the height of a mountainous sea island is depicted and explained in the Haidao suanjing [Sea Island Mathematical Manual].

At the beginning of the Tang Dynasty (618-906), Liu’s nine problems were separated from the Jiuzhang and published as an independent mathematical classic, the Haidao suanjing [Sea Island Mathematical Manual], so named because its first problem concerns the determination of distances involving a remote “sea island.” See Figure 8, above. Over the intervening centuries, the Haidao’s methods and techniques were followed and incorporated into other mathematical tracts with few questions asked.

The nineteenth century saw a resurgence of interest among Chinese mathematicians as to the mathematical traditions of their predecessors. The contents of the Haidao were examined and analyzed but mainly in regard to its principal solution technique, “double differences.” The first English language translation of the Haidao’s problems was published in 1912 by Yoshio Mikami, who considered only the first three problems [5]. A complete translation of the whole work into French was accomplished in 1932 by the Belgian sinologist-mathematician Louis van Hée [13]. In his commentary, van Hée concluded that the ancient Chinese mathematicians used the properties of similar triangles but only recognized similarity among right triangles. Since so many of their problems focused on surveying situations involving gnomon sightings and resulted in the development of right triangles, perhaps his conclusion was justified but it still seemed unlikely to me that an appreciation of geometric similarity would be so limited.

In my further analysis of the Sea Island problems, I became aware of a solution procedure that avoided any considerations of the concept of similarity. It involved a geometrical concept labeled “The In-and-Out Complementary Principle,” IOCP. The prevalence of this technique in traditional Chinese mathematical calculations has been traced and well documented by the contemporary Chinese academician Wu Wenjun [14]. Its basis can be best understood in reference to Figure 9.

Figure 9. Rectangles AF and FC have equal areas, according to the "In-and-Out Complementary Principle” (IOCP).

In the following discussion, I will designate rectangles by reference to their left-to-right ascending diagonals, thus the rectangle shown above in Figure 9 will be referred to as rectangle AC. Rectangle AC is bisected by diagonal BD. Let the horizontal line JG intersect the vertical line HE at point F on the diagonal. This construction partitions rectangle AC into six disjoint regions. In Figure 9, it can be seen that the triangles on opposite sides of the diagonal are congruent: \(\Delta DJF\cong\Delta FHD\) and  \(\Delta EBF\cong\Delta GFB.\) Because BD bisects rectangle AC, the areas of the remaining rectangles, AF and FC, are also equal. Since in the methods of geometric-algebra employed by the Chinese, the area of a rectangle is considered a numerical product, several numerical relationships arise from the geometric situation, for example:

AE x EF = FG x GC               AB x BG = EB x BC

JF : EB = DJ : FE                   AB : EB = DA : FE

Thus, it appears that in solving numerical problems involving right triangles, the traditional Chinese approach was to package the right triangles into rectangles where IOCP could be applied. Now, let me return to the two illustrative problems solved above and rework them using the IOCP.

Reconsidering Jiuzhang 9:17 in light of IOCP, the geometric situation depicted in Figure 4 is altered by extending the line segments ED and AB to meet at point I and constructing line segments from points D and B to intersect perpendicularly at point G. Extend line segment AC to intersect DG at point F and line segment EC to intersect BG at point H. See Figure 10.

Figure 10. Diagram for Jiuzhang 9:17, assuming the use of the In-and-Out Complementary Principle (IOCP)

Now consider rectangle DB, within which are contained rectangles EA and FH. The following relationships exist: IE x EC = CF x FG, thus CF = (IE x EC)/FG and, since CF = ED = x and FG = AB, then x = (IE x EC)/AB = (100)²/15 and x = 666 2/3.

Reworking Jiuzhang 9:23 in a similar fashion, I obtained the geometrical situation depicted in Figure 11, where the area of rectangle DC is equal to the area of rectangle CI.

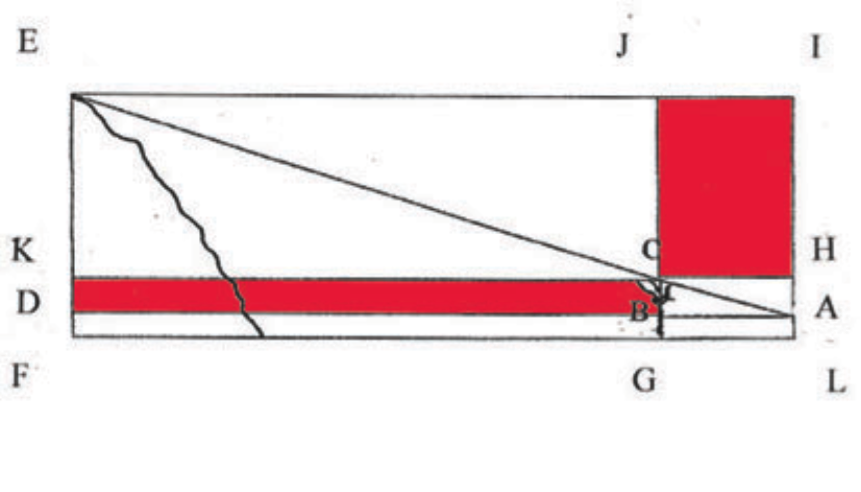
Figure 11. Diagram for Jiuzhang 9:23, assuming the use of the In-and-Out Complementary Principle (IOCP)

Now KD x DB = JC x BA. Solving for JC, we have JC = (KD x DB)/BA and, substituting in the given values, JC = 53(95-7)/3 = 1554.666. Adding to this value the height of the tree, the height of the hill is obtained: EF = 1554.666 + 95 = 1649.6662.

All the right triangle problems of the Jiuzhang and all the problems of the Haidao can be solved using IOCP. Thus, I am now convinced that early Chinese mathematicians did not utilize similar triangles, per se, but rather similar rectangles. Whether the proportions developed and used in this situation resulted in a discovery of “the rule of three” remains an open issue. As for whether or not Chapter 8 of the Jiuzhang provides evidence for the existence of matrix algebra in early China, this issue has since been carefully documented and studied and the conclusions published in [3].

Redemption

While my speculative adventure into traditional Chinese problem solving techniques and the allures of the right triangle faltered, nevertheless, I came away from the experience with several valuable insights, including:

1. An enhanced appreciation of the early importance of gnomon observations in the development of mathematical thought. Such observations provided an intuitive recognition of angle and, in particular, the importance of the right angle and the concept of perpendicularity (orthogonality). They also resulted in the eventual realization and utilization of the ratio, height of gnome to its shadow length, suggesting the strong possibility that gnomon observations and measurements provided an impetus to further investigate the properties of right triangles leading to a formulation of the Xian thu proof.
2. The facility of the ancient geometric-algebraic approach to problem solving, which reflects modern pedagogical theories for mathematics discovery and learning: physical and visual interaction, and manipulation followed by data collection and hypothesis formation and testing. Mathematical concepts were initially obtained through physical experiences. Semi-concrete modeling and visually performed solution methods were a logical step in the development of algebraic thought and, indeed, in most mathematical concept formation.
3. It is necessary to appreciate historical materials in their intellectual and societal contexts. Remain cautious that what appears to be so mathematically obvious in a modern light may have been unrecognized by our ancestors.

And finally, there is still much to learn about the evolution of mathematical concepts and the early techniques of problem solving. The journey of curiosity and inquiry still beckons.

References

[1] Bronowski, J. The Ascent of Man, Little, Brown and Company, Boston, 1973, p. 163.

[2] Cullen, C. Astronomy and Mathematics in Ancient China: the Zhou bi suan jing, Cambridge University Press, Cambridge, 2007.

[3] Hart, R. The Chinese Roots of Linear Algebra, The Johns Hopkins University Press, Baltimore, 2011.

[4] Li, Y. and Du, S. Chinese Mathematics: A Concise History, Oxford University Press, New York, 1987.

[5] Mikami, Y. The Development of Mathematics in China and Japan, 1913: Chelsea Publishing Co. reprint, 1974, pp. 34-36.

[6] Needham, J. Science and Civilization in China, vol. 3, Cambridge University Press, Cambridge, 1959, pp. 22-23.

[7] Plutarch. Septem Sapietium Convivium [Dinner of the Seven Wise Men], Loeb Classical Library, Harvard University Press, Cambridge, MA, 1928, II: pp. 345-349.

[8] Shen, K et al. The Nine Chapters of the Mathematical Art: Companion & Commentary, Oxford University Press New York, 1999.

[9] Smith, D.E. History of Mathematics (2 vols.), 1925: Dover reprint, 1958.

[10] Swetz, F. “Trigonometry Comes Out of the Shadows,” Learn from the Masters, F. Swetz et al (eds.) ,The Mathematical Association of American, Washington, DC. 1995, pp. 57-73.

[11] Swetz, F. The Sea Island Manual: Surveying and Mathematics in Ancient China, The Pennsylvania State University Press, University Park, PA, 1992.

[12] Swetz, F and Kao, T.I. Was Pythagoras Chinese? An Examination of Right Triangle Theory in Ancient China, The Pennsylvania State University Press, University Park, PA, 1977.

[13] van Hée, L. “Le Classique de l’île maritime: Ouvrage chinois de III^e siècle.” Quellen und Studien zur Geschichte der Mathematik 2, 1932, pp. 255-258.

[14] Wu, W. “Churu xiangbu yuanli [Out-in-complementary principle],” Zhongguo gudai keji chengjiu [Achievements of Ancient Chinese Science and Technology], Institute for History of Natural Sciences, Bejing, 1978.

About the Author

Frank J. Swetz is a founding editor of MAA Convergence. He currently hunts and secures images of "mathematical treasures" for Convergence from libraries and museums both near and far from his home in Harrisburg, Pennsylvania. In addition to his publications referenced above and many others over the years, he has most recently authored the book, Mathematical Expeditions: Exploring Word Problems across the Ages (Johns Hopkins University Press, 2012), and edited the collections, The European Mathematical Awakening: A Journey Through the History of Mathematics from 1000 to 1800 (Dover Publications, 2013) and The Search for Certainty: A Journey Through the History of Mathematics from 1800 to 2000 (Dover Publications, 2012).