- About MAA
- Membership
- MAA Publications
- Periodicals
- Blogs
- MAA Book Series
- MAA Press (an imprint of the AMS)
- MAA Notes
- MAA Reviews
- Mathematical Communication
- Information for Libraries
- Author Resources
- Advertise with MAA
- Meetings
- Competitions
- Programs
- Communities
- MAA Sections
- SIGMAA
- MAA Connect
- Students
- MAA Awards
- Awards Booklets
- Writing Awards
- Teaching Awards
- Service Awards
- Research Awards
- Lecture Awards
- Putnam Competition Individual and Team Winners
- D. E. Shaw Group AMC 8 Awards & Certificates
- Maryam Mirzakhani AMC 10 A Awards & Certificates
- Two Sigma AMC 10 B Awards & Certificates
- Jane Street AMC 12 A Awards & Certificates
- Akamai AMC 12 B Awards & Certificates
- High School Teachers
- News
You are here
Extending al-Karaji's Work on Sums of Odd Powers of Integers - The Sum of the Fifth Powers
Vidinli then asks, “What happens if we apply the same procedure to find the differences of third powers of \(S_n\)?” His answer consists of the following work: \begin{align} S_n^{\,3} - S_{n-1}^{\,\,3} &= {\bigg[ {\frac{n(n+1)}{2}}\bigg]}^3 - {\bigg[ {\frac{(n-1)n}{2}}\bigg]}^3 \\ &= {\frac{n^3}{8}} \Big({(n+1)}^3 - {(n-1)}^3 \Big) \\ &= {\frac{n^3}{8}}(n^3 + 3n^2 + 3n +1 - n^3 + 3n^2 - 3n + 1) \\ &= {\frac{n^3}{8}}(6n^2 + 2) \\ &= {\frac{1}{4}}(3n^5 + n^3) \\ &= {\frac{3}{4}}n^5 + {\frac{1}{4}}n^3 , \\ S_{n-1}^{\,\,3} - S_{n-2}^{\,\,3} & = {\frac{3}{4}}{(n-1)}^5 + {\frac{1}{4}}{(n-1)}^3 ,\\ S_{n-2}^{\,\,3} - S_{n-3}^{\,\,3} & = {\frac{3}{4}}{(n-2)}^5 + {\frac{1}{4}}{(n-2)}^3 , \\ \\ \\ \dots\dots\dots & \dots\dots\dots\dots\dots ,\\ \\ S_2^{\,3} - S_{1}^{\,3} & = {\frac{3}{4}}2^5 + {\frac{1}{4}}2^3 , \\ S_1^{\,3} - S_{0}^{\,3} & = {\frac{3}{4}}1^5 + {\frac{1}{4}}1^3 .\end{align}
When we add these equations, we obtain: \[ {S_n^{\,3}} = {\frac{3}{4}}\Big(1^5 + 2^5 + 3^5 + \cdots + n^5\Big) + {\frac{1}{4}}\Big(1^3 + 2^3 + 3^3 + \cdots + n^3\Big) ,\] or \[ {{(1+2+3+\cdots +n)}^3} = {\frac{3}{4}}\Big({1^5 + 2^5 + 3^5 + \cdots + n^5}\Big) \] \[ + {\frac{1}{4}}\Big({1^3 + 2^3 + 3^3 + \cdots + n^3}\Big) ,\] as can be seen in Figure 7.
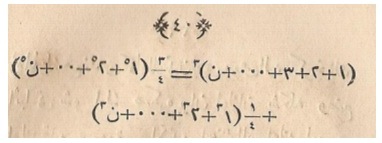
Figure 7. An equation involving the sum of the fifth powers (from Mebahis-i İlmiyye, 1867, courtesy of the authors).
We already know that \[ 1^3 + 2^3 + 3^3 + \cdots + n^3 = {{(1+2+3+\cdots +n)}^2} = {\bigg[ {\frac{n(n+1)}{2}}\bigg]}^2 \] and \[{1 + 2 + 3 + \cdots + n} = {\frac{n(n+1)}{2}}.\] When we substitute these identities into the equation in (or just above) Figure 7, we obtain: \[ {\bigg[ {\frac{n(n+1)}{2}}\bigg]}^3 = {\frac{3}{4}}\Big(1^5 + 2^5 + 3^5 + \cdots + n^5\Big) + {\frac{1}{4}}{\bigg[ {\frac{n(n+1)}{2}}\bigg]}^2 . \]
If we then re-arrange this equation, we can find a formula for the sum of the fifth powers: \[ {1^5 + 2^5 + 3^5 + \cdots + n^5} = {\frac{4}{3}}\Bigg({{\bigg[ {\frac{n(n+1)}{2}}\bigg]}^3 - {\frac{1}{4}}{\bigg[ {\frac{n(n+1)}{2}}\bigg]}^2}\Bigg) \] or \[ {1^5 + 2^5 + 3^5 + \cdots + n^5} = {\frac{1}{6}}n^6 + {\frac{1}{2}}n^5 + {\frac{5}{12}}n^4 - {\frac{1}{12}}n^2 . \]
Hakan Kursat Oral (Yildiz Technical University) and Hasan Unal (Yildiz Technical University), "Extending al-Karaji's Work on Sums of Odd Powers of Integers - The Sum of the Fifth Powers," Convergence (August 2011), DOI:10.4169/loci003725




