The Japanese Theorem for Nonconvex Polygons - The Total Inradius Function

The Total Inradius Function

Using the notation given on the previous page, elementary trigonometry, and the polygonal Carnot's theorem we are able to give an explicit formula for the function \(f: \mathcal{P}_n^c = \mathcal{P}_{R,n}^c \rightarrow {\mathbb R} .\)

Let \(P \in \mathcal{P}_n^c .\) By the polygonal Carnot's theorem,

\[f(P) = r_P = (2-n)R + \sum_{k=1}^n d_k\]

where \(d_k\) is the signed distance from the center of the circle to the \(k\)th side of the polygon. Notice that \(d_k > 0\) precisely when \(\theta_k < \pi .\) In particular, as we see in Figure 8, \(d_k = R \cos( \theta_k/2) .\) When \(\theta_k \geq \pi , \) \(d_k = -R \cos (\pi - \theta_k / 2) = R \cos( \theta_k / 2) ,\) as well.

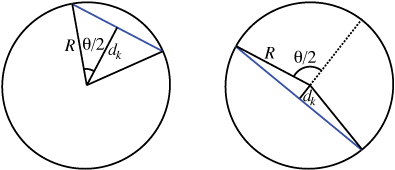
Figure 8

Thus, we obtain the following explicit expression for the total inradius function in terms of \(\theta_1 , \ldots , \theta_n :\)

\[f(P) = f(\theta_1, \ldots , \theta_n ) = R \left( 2 - n + \sum_{k=1}^n \cos \left(\frac{\theta_k}{2} \right) \right) .\]