- About MAA
- Membership
- MAA Publications
- Periodicals
- Blogs
- MAA Book Series
- MAA Press (an imprint of the AMS)
- MAA Notes
- MAA Reviews
- Mathematical Communication
- Information for Libraries
- Author Resources
- Advertise with MAA
- Meetings
- Competitions
- Programs
- Communities
- MAA Sections
- SIGMAA
- MAA Connect
- Students
- MAA Awards
- Awards Booklets
- Writing Awards
- Teaching Awards
- Service Awards
- Research Awards
- Lecture Awards
- Putnam Competition Individual and Team Winners
- D. E. Shaw Group AMC 8 Awards & Certificates
- Maryam Mirzakhani AMC 10 A Awards & Certificates
- Two Sigma AMC 10 B Awards & Certificates
- Jane Street AMC 12 A Awards & Certificates
- Akamai AMC 12 B Awards & Certificates
- High School Teachers
- News
You are here
Special Relativity and Conic Sections - A Thought Experiment
![]() Mathwright Microworld for this page
Mathwright Microworld for this page
|
|
PDF version of this page
|
I am now ready to describe the experiment that displays the similarity class properties: the focus-locus property and the focus-directrix property of the ellipses ![]() constructed in the Planes Intersecting Cones section. I give a sketch first, and then discuss the details. You might like to keep the calculation of that page in mind, where the point
constructed in the Planes Intersecting Cones section. I give a sketch first, and then discuss the details. You might like to keep the calculation of that page in mind, where the point ![]() of the hyperboloid
of the hyperboloid
|
|
represents the first tick of the moving observer's ( ![]() ) clock in standard observer's (
) clock in standard observer's ( ![]() ) coordinates. The speed that
) coordinates. The speed that ![]() attributes to
attributes to ![]() is in that case
is in that case
|
|
Sketch of the experiment:
Suppose a "standard" inertial observer: ![]() is situated at the origin
is situated at the origin ![]() of the Euclidean
of the Euclidean ![]() plane. This observer watches a "moving" inertial observer
plane. This observer watches a "moving" inertial observer ![]() pass through the origin at a time that they both measure to be 0.
pass through the origin at a time that they both measure to be 0. ![]() considers
considers ![]() to be moving at constant velocity
to be moving at constant velocity ![]() where
where ![]() is a unit vector and
is a unit vector and ![]() is the speed. I assume for this experiment that
is the speed. I assume for this experiment that ![]() .
.
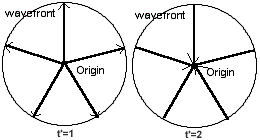
Both observers measure the speed of light to be 1. At the event where ![]() arrives at the origin it emits a circular pulse of light. From the point of view of
arrives at the origin it emits a circular pulse of light. From the point of view of ![]() , after one unit of time as measured by
, after one unit of time as measured by ![]() the wavefront forms a curve of events whose spatial coordinates
the wavefront forms a curve of events whose spatial coordinates ![]() simply form a circle centered at his origin. The events in the wavefront all have time coordinate
simply form a circle centered at his origin. The events in the wavefront all have time coordinate ![]() for
for ![]() .
.
However, from the point of view of ![]() , this curve of events is the intersection of a plane with a cone in his
, this curve of events is the intersection of a plane with a cone in his ![]() coordinatization of spacetime. I called the intersections obtained physically this way, boost intersections:
coordinatization of spacetime. I called the intersections obtained physically this way, boost intersections: ![]() . The time coordinates that he measures for points in that wavefront are not constant, but, as we saw, the curve forms, in the Euclidean structure that the plane inherits from the hyperbolic geometry, an ellipse. That ellipse is the intersection (in 2+1 spacetime) of a certain plane with a light cone. Observer
. The time coordinates that he measures for points in that wavefront are not constant, but, as we saw, the curve forms, in the Euclidean structure that the plane inherits from the hyperbolic geometry, an ellipse. That ellipse is the intersection (in 2+1 spacetime) of a certain plane with a light cone. Observer ![]() projects this ellipse into his (Euclidean)
projects this ellipse into his (Euclidean) ![]() plane t = 0, and discovers another ellipse:
plane t = 0, and discovers another ellipse: ![]() , this time with one focus at the origin
, this time with one focus at the origin ![]() . The ellipse has semi-minor axis 1, and semi-major axis
. The ellipse has semi-minor axis 1, and semi-major axis ![]() directed along
directed along ![]() .
.
If we imagine that each ray of light emitted by ![]() is reflected back to
is reflected back to ![]() at
at ![]() then they all converge again at
then they all converge again at ![]() at a time that
at a time that ![]() measures to be
measures to be ![]() .
.
For ![]() , each of those rays of light projects to a pair of intervals, the first, starting at the origin, a focus of the projected wavefront ellipse, and arriving at the ellipse, and the second reflecting to the other focus, which will be the spatial
, each of those rays of light projects to a pair of intervals, the first, starting at the origin, a focus of the projected wavefront ellipse, and arriving at the ellipse, and the second reflecting to the other focus, which will be the spatial ![]() position that
position that ![]() ascribes to
ascribes to ![]() at
at ![]() time
time ![]() . We will see in Interpretation of the Experiment that the sum of the two lengths of these intervals is, for each ray of light, the time coordinate that
. We will see in Interpretation of the Experiment that the sum of the two lengths of these intervals is, for each ray of light, the time coordinate that ![]() ascribes to the arrival back at
ascribes to the arrival back at ![]() of the reflected ray. These time coordinates are the same for all the rays, because their convergence is at a single event, clearly simultaneous both for
of the reflected ray. These time coordinates are the same for all the rays, because their convergence is at a single event, clearly simultaneous both for ![]() and
and ![]() .While
.While ![]() measures that time to be 2,
measures that time to be 2, ![]() measures it to be the time coordinate of the event: "
measures it to be the time coordinate of the event: " ![]() at
at ![]() time
time ![]() ." That number is easy to calculate. It is the length of the major axis:
." That number is easy to calculate. It is the length of the major axis:
|
|
Since 2 < ![]() , this is another example of "time dilation." Observer
, this is another example of "time dilation." Observer ![]() thinks that more time has passed than the 2 units that observer
thinks that more time has passed than the 2 units that observer ![]() measures at the event: "
measures at the event: " ![]() at
at ![]() time
time ![]() ."
."
Obviously, every ellipse in the Euclidean plane can be obtained up to similarity in this way since these ellipses have semi-minor axis 1, and semi-major axis ![]() . This will establish what I call the focus-locus property for all ellipses, and will give a physical interpretation for the constant sum of distances from the foci to the points on the ellipse.
. This will establish what I call the focus-locus property for all ellipses, and will give a physical interpretation for the constant sum of distances from the foci to the points on the ellipse.
Now to interpret the focus-directrix property, I first identify the directrix of ellipse ![]() in this experiment. This will be the intersection of the plane
in this experiment. This will be the intersection of the plane ![]() with the
with the ![]() plane t = 0. On our assumption that
plane t = 0. On our assumption that ![]() this intersection is a line in the plane of
this intersection is a line in the plane of ![]() . This will give an interpretation of the directrix, and then of the eccentricity of
. This will give an interpretation of the directrix, and then of the eccentricity of ![]() as the tangent of the dihedral angle thus formed in the Euclidean metric. We will see the details in the Interpretation of the Experiment section on the next page.
as the tangent of the dihedral angle thus formed in the Euclidean metric. We will see the details in the Interpretation of the Experiment section on the next page.
Further, it is clear that every intersection of a plane with the light cone that is the result of the ![]() for arbitrary
for arbitrary ![]() in the interior of the cone can be associated with a unique boost intersection with the physical interpretation just described. These intersections are geometrically similar, and are obtained by simple scalar expansion or contraction from the origin. Therefore, their projections are also geometrically similar. In the experiment, I work only with the
in the interior of the cone can be associated with a unique boost intersection with the physical interpretation just described. These intersections are geometrically similar, and are obtained by simple scalar expansion or contraction from the origin. Therefore, their projections are also geometrically similar. In the experiment, I work only with the ![]() that derive from the boost intersections, that is, from planes tangent to the hyperboloid:
that derive from the boost intersections, that is, from planes tangent to the hyperboloid:
![]()
That's the sketch. What makes this work, as we will see, is the fact that every ellipse of the form ![]() is a conic intersection, i.e., the intersection of two light cones. I will first establish some notation to set up the experiment.
is a conic intersection, i.e., the intersection of two light cones. I will first establish some notation to set up the experiment.
Let us suppose that our standard inertial observer establishes the basis of coordinates ![]() for this 2+1 dimensional space-time. As before, I will let the name
for this 2+1 dimensional space-time. As before, I will let the name ![]() stand for the observer and the event of his first clock tick. Suppose now that there is another inertial observer, say
stand for the observer and the event of his first clock tick. Suppose now that there is another inertial observer, say ![]() , whose world line intersects the world line of
, whose world line intersects the world line of ![]() at the origin.
at the origin.
 In particular, I assume that both
In particular, I assume that both ![]() and
and ![]() have clocks that tick time 0 for each at the origin event. As before, I will picture this by using the coordinates of
have clocks that tick time 0 for each at the origin event. As before, I will picture this by using the coordinates of ![]() to describe the event of the next tick of the clock of
to describe the event of the next tick of the clock of ![]() on the world-line of
on the world-line of ![]() . This event must occur in the future light cone of
. This event must occur in the future light cone of ![]() , as we saw earlier. Since the hyperbolic interval connecting the first and second clock tick of
, as we saw earlier. Since the hyperbolic interval connecting the first and second clock tick of ![]() along the world-line of
along the world-line of ![]() is measured by
is measured by ![]() to be -1, it must be -1 in the coordinates of
to be -1, it must be -1 in the coordinates of ![]() . This means that, in the coordinates of
. This means that, in the coordinates of ![]() , it lies on the hyperboloid
, it lies on the hyperboloid
![]() ,
,
as in the figure at the right.
For the next step in setting up the experiment, I will choose the orthonormal system of vectors ![]() (in the hyperbolic metric) in a special way. We are free to choose any orthonormal system we like because of the covariance principle, so I select one adapted to the motion of
(in the hyperbolic metric) in a special way. We are free to choose any orthonormal system we like because of the covariance principle, so I select one adapted to the motion of ![]() in the following way.
in the following way.
Note 9. Orthonormal hyperbolic coordinates
|
|
|
|
Now let's describe the experiment in these terms. For observer ![]() moving with respect to an observer
moving with respect to an observer ![]() in such a way that their origins coincide, I will construct the ellipse formed by intersecting the light cone at the common origin with the hyperbolic orthogonal bisector of the segment from the origin to the event of the second clock tick of
in such a way that their origins coincide, I will construct the ellipse formed by intersecting the light cone at the common origin with the hyperbolic orthogonal bisector of the segment from the origin to the event of the second clock tick of ![]() . All of this is easily formulated as a "thought experiment." This experiment will lead us, in this section and the next, to give the "physical" interpretation of the focus-locus and focus-directrix properties of an ellipse.
. All of this is easily formulated as a "thought experiment." This experiment will lead us, in this section and the next, to give the "physical" interpretation of the focus-locus and focus-directrix properties of an ellipse.
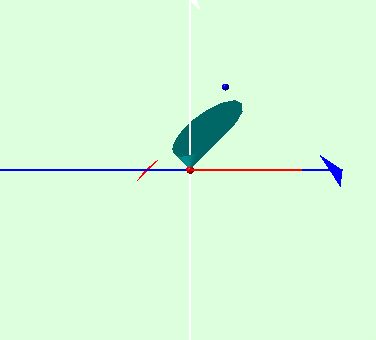
Here is the experiment performed by observer ![]() . At his time 0,
. At his time 0, ![]() emits a circular pulse of light. In his spatial coordinate system, he has a circular reflecting mirror of radius 1 with center at his origin. (Imagine a cylinder surrounding his world-line.) At
emits a circular pulse of light. In his spatial coordinate system, he has a circular reflecting mirror of radius 1 with center at his origin. (Imagine a cylinder surrounding his world-line.) At ![]() , the wavefront arrives at the mirror, and is reflected back. It arrives back at the origin at
, the wavefront arrives at the mirror, and is reflected back. It arrives back at the origin at ![]() .
.
In the coordinate system of ![]() , nothing interesting happens. The picture looks like this:
, nothing interesting happens. The picture looks like this:
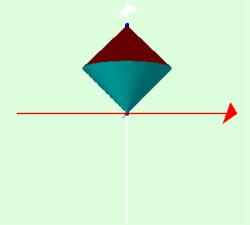
In 2+1 space-time, the process is as shown in the following figure, with emission phase the lower (green) part of the cone, and reception phase the upper (red) part of the picture. Light simply moves from the center of his circle to the boundary after 1 second, then returns to the origin after 2 seconds.
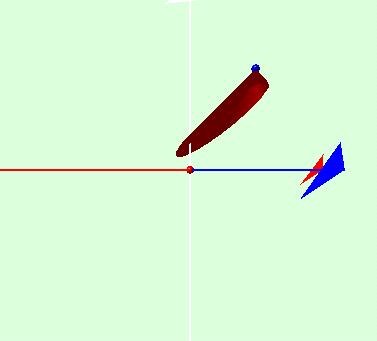
Now let's examine the same process from the viewpoint of ![]() . In his coordinates,
. In his coordinates, ![]() will be "moving" if the velocity
will be "moving" if the velocity ![]() .
.
The following figures illustrate the two halves of the experiment in his coordinates. In the first picture, the emission cone from the origin of ![]() coordinates is shaded gray. The reception cone -- the path of the reflected wavefront is shaded white.
coordinates is shaded gray. The reception cone -- the path of the reflected wavefront is shaded white.


The wavefront spreads from the origin along the gray section of the light cone. At ![]() time
time ![]() , it forms a curve obtained by intersecting the light cone at the origin with the plane that is the orthogonal bisector of the segment connecting the events
, it forms a curve obtained by intersecting the light cone at the origin with the plane that is the orthogonal bisector of the segment connecting the events ![]() and
and ![]() on the world-line of
on the world-line of ![]() . We saw in Geometry of 2+1 Spacetime that the points on the curve of arrival events must have equal hyperbolic interval (that is, 0) with the emission and reception events, hence must lie on the orthogonal bisector plane,
. We saw in Geometry of 2+1 Spacetime that the points on the curve of arrival events must have equal hyperbolic interval (that is, 0) with the emission and reception events, hence must lie on the orthogonal bisector plane, ![]() . In the second picture above, I show three light "rays" emitted in three directions.
. In the second picture above, I show three light "rays" emitted in three directions.
The following 3-dimensional picture shows this orthogonal bisector plane in grey, and it also depicts the x-y plane t = 0 in yellow. These planes intersect in the line that I have designated the directrix in the x-y plane that contains the projected ellipse (not visible in this picture).
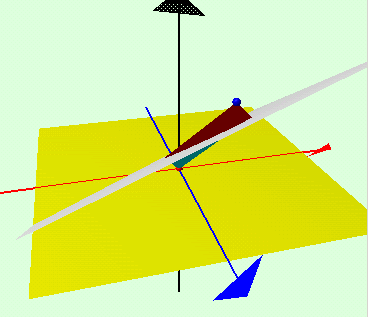
 In the figure at the right, I show the projection of the picture onto the x-y plane of observer
In the figure at the right, I show the projection of the picture onto the x-y plane of observer ![]() . I project the light rays also. We will see later that these light rays travel from one focus to the other of this projected ellipse. This indicates that the bridge from the plane-slicing-cone picture to the focus-locus picture is obtained by projecting the slice ellipse into the spatial (
. I project the light rays also. We will see later that these light rays travel from one focus to the other of this projected ellipse. This indicates that the bridge from the plane-slicing-cone picture to the focus-locus picture is obtained by projecting the slice ellipse into the spatial ( ![]() ) plane of observer
) plane of observer ![]() . We will see in Interpretation of the Experiment exactly why this is so.
. We will see in Interpretation of the Experiment exactly why this is so.
We saw in Planes Intersecting Cones that this plane is also tangent to the hyperboloid at vector ![]() ,
,
|
|
by observing that, in the Euclidean metric, the vectors ![]() are perpendicular to the gradient of the hyperboloid at that point. This Euclidean calculation gives the same result in the present hyperbolic context, since
are perpendicular to the gradient of the hyperboloid at that point. This Euclidean calculation gives the same result in the present hyperbolic context, since ![]() generate the plane
generate the plane ![]() at
at ![]() .
.
As we saw above, the wavefront then follows the inverted cone back to the reception event at ![]() . The skewed and unsymmetrical aspect of the pictures is the result of the fact that observer
. The skewed and unsymmetrical aspect of the pictures is the result of the fact that observer ![]() is actually moving with respect to observer
is actually moving with respect to observer ![]() .
.
From the plane-slicing-cone definition of a conic, we see that the intersection of the two light cones is, in this case, an ellipse. That is, the events in the light cone along the wavefront at ![]() form an ellipse (
form an ellipse ( ![]() where
where ![]() =
= ![]() ) in the frame of
) in the frame of ![]() . But since
. But since ![]() is using a hyperbolic and not a Euclidean metric, let us examine the equation for this intersection curve in
is using a hyperbolic and not a Euclidean metric, let us examine the equation for this intersection curve in ![]() coordinates.
coordinates.
The points on this curve are not "simultaneous" in the frame of ![]() (although, of course, they are simultaneous in the frame of
(although, of course, they are simultaneous in the frame of ![]() ). This curve is, however, obtained by intersecting the light cone with the plane through vector
). This curve is, however, obtained by intersecting the light cone with the plane through vector ![]() that is parallel to vectors
that is parallel to vectors ![]() , since these vectors generate the orthogonal bisector according to the relations derived above,
, since these vectors generate the orthogonal bisector according to the relations derived above,
|
|
If we let ![]() define coordinates for the plane in the obvious way, then they will give Euclidean coordinates for the plane satisfying (even in the hyperbolic metric),
define coordinates for the plane in the obvious way, then they will give Euclidean coordinates for the plane satisfying (even in the hyperbolic metric),
|
|
and the points of the form
|
|
are in the curve, since, as is easy to see, in the hyperbolic metric,
|
|
In the standard Euclidean metric for ![]() , using
, using ![]() coordinates,
coordinates, ![]() are still perpendicular, but while
are still perpendicular, but while ![]() =1, in general,
=1, in general, ![]() . So the intersection is an ellipse in the plane if we use the induced Euclidean structure from
. So the intersection is an ellipse in the plane if we use the induced Euclidean structure from ![]() .
.
|
|
|
 Both Phases |
The light cones |
Having developed these pictures, we now ask what the interpretation for the focus-locus and focus-directrix properties of the ![]() ellipse is. In the next section, Interpretation of the Experiment, we will give what may be a surprising answer to this question.
ellipse is. In the next section, Interpretation of the Experiment, we will give what may be a surprising answer to this question.
James E. White, "Special Relativity and Conic Sections - A Thought Experiment," Convergence (October 2004)