When do Cubics have Real Roots?
The Microworld…
The Story…
Every cubic function: may be transformed by a linear change of variable
to the form . In the latter form, the abscissa of the inflection point is 0, so the average of the roots of the new equation is 0. Such an equation has three real solutions if and only if the original equation did. One solves g(u) = 0 for u and then sets the roots to
This
procedure might be called completing the cube but it amounts to shifting
the function so that the graph has inflection point at u = 0. The actual shape
of the cubic graph and its position above or below the x-axis is determined by
the two parameters: d and e.
When we
develop techniques for solving cubic equations later in this Microworld we will
show that the equation with d and e real has all real solutions if
and only if the discriminant: .
The points in the plane that satisfy this condition are the
points inside the cusp:
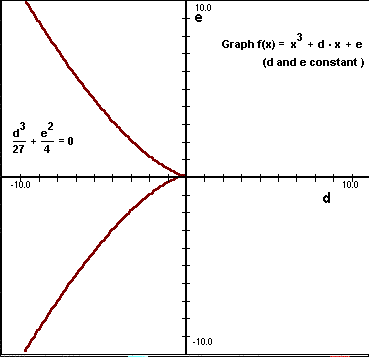
In this
exercise, you will get a little experience with how the choice of equation
(hence the choice of d and e) determine the shape of the graph and whether it
has all real roots.
To do this experiment, just click at any point on the right-hand screen to determine the values of d and e. The corresponding graph will be drawn on the left. You may clear the screens separately using the pushbuttons below.
You may notice
that all graphs drawn have abscissa of inflection point equal to 0. Now when
the roots are not all real, the average of the real roots (the single real
root) is in general not equal to 0. In order to understand this, we will have
to take a close look at complex numbers. And we shall do that in the next
section.
But for those of you who know, you may recall that we
mentioned earlier that the abscissa of the inflection point is the average
of the real parts of the roots. If one root is real, then the real parts of
each of the other two are equal (since they are conjugate). Thus the real
part of each complex (not real) root is 1/2 the negative of the real root.
What is going on here? Everything will be clear when we introduce the "roots
of unity." They are special complex numbers with some remarkable properties.
When you finish this exercise, you will be ready to move to Chapter 2: Euler's Formula.
