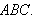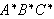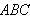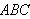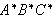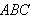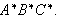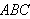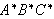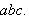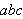Inscribed and Circumscribed Circles
December 2001
Ellen Maycock
How do we construct the inscribed and circumscribed circles of a triangle in
various geometries? Do similar constructions hold in Euclidean, spherical and
hyperbolic geometry? In this lab, we will investigate these constructions and
see some of the special features of these circles.
Before the lab
This section should be done as homework before the laboratory period.
Remember that the circumscribed circle of a triangle is the
circle that goes through the 3 vertices of the triangle. The center of this
circumscribed circle is called the circumcenter. The
inscribed circle of a triangle is the circle that touches
each side of the triangle exactly once. The center of this circle is called
the incenter.
Let's begin with a compass and straightedge construction in Euclidean
geometry. You may use pencil and paper or a dynamic software such as
Geometer's Sketchpad to do your constructions.
-
Draw an arbitrary triangle

-
Construct the angle bisectors of each angle of the triangle. If you are using
a dynamic program, convince yourself that the angle bisectors always meet in
one point by moving the vertices around. Which circle would have this point as
a center? Why?
-
Construct the perpendicular bisectors of each side of the triangle. As before,
convince yourself that they always meet in one point. Which circle would have
this point as a center? Why?
In the Lab
You will work through this section with your partner during the laboratory
period.
You will use the Lénárt Sphere to consider
these constructions in spherical (elliptic) geometry and the dynamic program
PoincaréDraw to investigate them in hyperbolic
geometry.
Spherical Geometry
You will need to answer the following questions by working on the
Lénárt Sphere.
-
Draw a triangle

 on the Lénárt Sphere. Then construct the
polar triangle
on the Lénárt Sphere. Then construct the
polar triangle

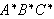 associated to triangle
associated to triangle

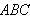 .
(See below if you are not familiar with the polar triangle).
.
(See below if you are not familiar with the polar triangle).
-
Draw the angle bisectors of the original triangle

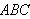 .
Do they intersect in a point? More than one point? No points? Be sure to
consider some extreme cases, such as when all the vertices lie on one great
circle. Do the angle bisectors of triangle
.
Do they intersect in a point? More than one point? No points? Be sure to
consider some extreme cases, such as when all the vertices lie on one great
circle. Do the angle bisectors of triangle

 relate to triangle
relate to triangle

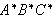 ? How?
? How?
-
For the cases when you found a common point of intersection, use this point as
the center to draw a special circle for the triangle

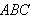 .
Is this the same construction you used in Euclidean geometry? Also use this
point to draw a special circle for triangle
.
Is this the same construction you used in Euclidean geometry? Also use this
point to draw a special circle for triangle

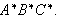
-
Now draw the perpendicular bisectors for the sides of triangle

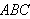 .
Do they intersect in a point? More than one point? Be sure to consider some
extreme cases. For the cases when you found a common point of intersection,
use the point to construct a special circle for triangle
.
Do they intersect in a point? More than one point? Be sure to consider some
extreme cases. For the cases when you found a common point of intersection,
use the point to construct a special circle for triangle

 .
Is this the same construction as in Euclidean geometry? Do the perpendicular
bisectors of the sides of triangle
.
Is this the same construction as in Euclidean geometry? Do the perpendicular
bisectors of the sides of triangle

 relate to the triangle
relate to the triangle

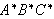 ?
?
-
Write a conjecture based on your work in this section. Explain in your own
words why you think your conjecture is true.
Hyperbolic geometry
You will need to answer the following questions by working in the computer
lab, using the program PoincaréDraw.
-
Plot 3 points on the screen, and construct the triangle

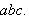 Then construct the angle bisectors of each angle. Do they intersect in one
point? Move the triangle around the screen to consider different
possibilities, making sure you include some extreme cases.
Then construct the angle bisectors of each angle. Do they intersect in one
point? Move the triangle around the screen to consider different
possibilities, making sure you include some extreme cases.
-
Try to construct an inscribed circle based on your work in the problem above.
Does the construction agree with the construction in Euclidean geometry? Does
the construction always work? Why or why not?
-
Now construct the perpendicular bisectors of each side of triangle

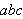 .
Do they intersect in a point? If so, draw a circumscribed circle for triangle
.
Do they intersect in a point? If so, draw a circumscribed circle for triangle

 using this point as a center. Use the dynamic property of the program to see
if the construction holds for all triangles.
using this point as a center. Use the dynamic property of the program to see
if the construction holds for all triangles.
-
What problems did you run into trying to answer question 11? Can you make a
conjecture based on the data? What possibilities can occur with the
perpendicular bisectors? When will you be able to construct a circumscribed
circle?
Further work
This section should be done as homework after the laboratory period.
-
Write a careful proof that the perpendicular bisectors of the sides of a
triangle intersect in a point that is the center of the circumscribed circle
in Euclidean geometry.
-
Write a careful proof that the angle bisectors of a triangle intersect in a
point that is the center of the inscribed circle in Euclidean geometry.
This document created by Scientific WorkPlace 4.0.