When an ellipse is presented as a "conic section" in the context of 3-dimensional Euclidean geometry, the construction is simple and visual: You're given a standard cone with vertex at the origin, and you select a plane not through the origin whose normal vector at the origin is in the interior of the cone. The intersection of the cone and plane is the metric curve we call an ellipse. This is essentially the definition given by the Greek geometer Apollonius of Perga, about 200 BCE.

The similarity classes of these curves have certain well known properties that we call the focus-locus and focus-directrix properties. Each of these properties of ellipses actually characterizes their similarity class. But the usual construction of conic sections as the curves formed by planes intersecting cones does not make it evident why the properties should be true. In this story, I give a geometric and dynamic interpretation of the fact that similarity classes of ellipses have these properties.

It is convenient to use the language of 2+1 spacetime geometry to develop the ideas. In particular, I describe a thought experiment in special relativity that gives a physical and dynamic interpretation of the fact that the sum of distances from a point to the two foci is constant. I also give a dynamic interpretation of the constant ratio of distances from a point to a focus and from the point to the directrix, as well as an interpretation of the directrix itself. In this view, the "plane-slicing-cone" description of an ellipse is the first step in the description of a second ellipse for which the similarity class properties have straightforward geometric interpretations.

---

Publication date: October, 2004

© Copyright 2004 by James E. White

My first aim in this story of ellipses, cones, and spacetime geometry is to stimulate cross-disciplinary thinking. I make connections between Euclidean and hyperbolic geometry, the latter providing the idiom of special relativity. The facts of hyperbolic geometry and linear algebra that I need are fairly elementary, but the physical intuition required to apply them depends strongly on your experience and background. The section titled Light Rays, Clocks, and Rulers: A Visual Primer (in special relativity) is my modest attempt to reinforce and develop that style of thinking.

My second purpose is to recruit 3-dimensional graphics as a heuristic method that can illustrate the hyperbolic geometry of  and support visual intuition of some elementary facts of special relativity. For that, my pages (from page 5 on) have two components:

• Static Hypertext Component: You may view the full text in any browser by continuing on with the numbered pages. Each page (after the Introduction on page 3) also contains a link to the same page in Portable Document Format (PDF), which you can download, read, and/or print at your leisure.

   

• Dynamic Component: A Mathwright Microworld, in which you can ask your own questions and experiment as you read along in your browser.

   

  • If you are on a Windows platform (Windows 98/ME/NT/2000/XP) with an ActiveX-enabled browser, such as Internet Explorer 5.0 or later, I strongly recommend that you interact with the dynamic component. You will find a link on each page (from page 5 on) to the Microworld, which will open in a new browser window. Each Microworld has instructions for the interactions within it -- click the Information button.  You will need to switch back and forth between the static and dynamic windows.

     

  • If you have already downloaded the MathwrightWeb Player , you need no further preparation. If not, please click to get the Player, and then continue on page 3.

John Kepler observed that every ellipse that is not a circle has a pair of distinct foci. If one of these foci is distinguished, so that the pair is ordered, then this ordered pair determines an oriented line, and there is another line perpendicular to it called the directrix of the ellipse. (A circle has a single focus and no directrix.) Geometric similarities between non-circular ellipses correspond both to the foci and to the directrices. Here are the similarity class properties of ellipses to which I referred in the abstract.

1. The focus-locus property: An ellipse is the locus of points the sum of whose distances from the two foci is constant, and that constant is the length of the major axis.

2. The focus-directrix property: An ellipse (when it is not a circle) is the locus of points whose distance from a certain focus has constant ratio with the distance to the line called the directrix. That ratio (strictly between 0 and 1) is called the eccentricity of the ellipse.

In the Euclidean geometry of the plane, Apollonian ellipses may be represented in certain coordinates with origin at the center as

.

Then a straightforward bit of analysis shows that ellipses do have the focus-locus and focus-directrix properties. But that analysis does not usually offer an interpretation of the similarity properties (1) and (2). In order to find an interpretation, I cast the plane-intersecting-cone construction in the light of a certain hyperbolic geometry on .

We will discover that the "slicing" plane (the one that intersects the cone) inherits in both geometries a Euclidean metric structure, and we will find coordinates that reduce it to the standard form above. Also, we will see that each slice construction determines a new ellipse by orthogonal projection to the "base plane" perpendicular to the axis of the cone and passing through the origin. This projection is the same in both geometries. The latter plane also inherits a Euclidean metric structure, and the new projected ellipse is the one for which the similarity class properties will have interpretations.

These new projected ellipses were always available in a purely Euclidean context, but special relativity provides a clue that tells us how to restrict the plane-slicing-cone construction to a certain family of slices for which the projected ellipses (as well as the slice ellipses) give representatives from every similarity class of ellipses in the plane. Further, when we use the hyperbolic metric structure on , we find the dynamic interpretation of the similarity class properties of these ellipses, the focus-locus and focus-directrix properties. In particular, for the latter, each projected ellipse has associated with it a "directrix" that has a dynamic meaning. We will see, for example, that the eccentricity of an ellipse is simply a speed (strictly between 0 and 1, where 1 is the speed of light) that determines its shape.

In the language of 2+1 spacetime geometry, we will discover a number of interesting analogies with the familiar Euclidean constructions. For example, with 1 as the speed of light, it is not a coincidence that numbers between 0 and 1 can be the eccentricities of ellipses. Since the projected ellipses range through all similarity classes, we finish with the similarity class properties (1) and (2) for all ellipses in the plane. In that context, we will also see the "conic sections" as "conic intersections," the intersections of pairs of light cones.

---

  PDF version of this page  (73 KB, 3 pages)

---

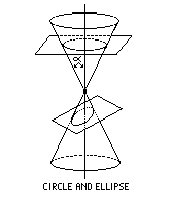The conic sections called ellipses have a number of definitions. The simplest is perhaps the one from which their name derives, the usual "plane-slicing-cone" construction.

This construction in the Euclidean geometry of is especially easy to visualize. Let's use a "standard" cone in Euclidean space given by the equation

.

Let's parameterize the construction in the following way. Suppose is an arbitrary point in the interior of the upper nappe of the cone. Then there is a unique hyperboloid, where t > 0,

that contains . Let be the plane tangent to that hyperboloid at . Finally, let be the intersection of that plane with the cone. Thus, the procedure associates an ellipse with each interior point of the cone. I will show that is the center of , and I will determine its semi-major and semi-minor axes, as well as its foci.

Now two interesting properties of these ellipses lead to new, and seemingly independent, characterizations of these metric curves. The first is the focus-locus property: Each ellipse has a pair of "foci," and the sum of distances from each point on the ellipse to the foci is constant. Richard Feynman used this latter description to construct yet another characterization of an ellipse, as well as using it to derive Kepler's first law of planetary motion from Newtonian gravitation (Goodstein and Goodstein, 1996).

The second property is the focus-directrix property: There is a line in the plane of the ellipse called its directrix. A noncircular ellipse is the locus of points whose distance from a certain focus has constant ratio (strictly between 0 and 1) with the distance to the directrix. That ratio is called the eccentricity of the ellipse.

It is natural to ask what the relation is between these constructions. Given an ellipse , what are its foci? And what are the dimensions of its major and minor axes? These questions are easy to answer, as I will show on the next page, but the straightforward calculation gives no insight into why we might expect that the sum of the distances from any point in the ellipse to the two foci to be constant. If we ask what the directrix is, we will find it easier to answer both questions not for itself but for a new ellipse that is closely related to .

Note 1. The Dandelin Spheres

I will use another characterization of conic sections to form a link between the "plane-slicing-cone" definition and the focus-locus and focus-directrix characterizations of ellipses. The latter characterizations are properties of similarity classes of ellipses, and I will establish those properties for all similarity classes. It happens that the ellipses can also be characterized as conic intersections, that is, the intersections of pairs of light cones in 2+1 spacetime. This leads to a simple physical interpretation of the focus-locus and focus-directrix properties and an interesting physical experiment that will show the way.

By 2+1 spacetime, I mean equipped with a certain nondegenerate hyperbolic inner product, not a Euclidean one. These geometries are different, so we must use some care when speaking about metric invariants. Which metric do we mean? When I speak of ellipses in 2+1 spacetime, I will always be referring to objects embedded in a plane that inherits Euclidean structure from the hyperbolic metric. In relativistic terms, all of the vectors in these planes are "space-like." The analogous constructions that yield hyperbolae must use planes that always contain a "time-like" subspace of dimension 1, hence do not inherit Euclidean structure.

Thus, I will consider simultaneously two geometric structures for : Euclidean structure and hyperbolic 2+1 space structure. Special relativity provides the lexicon that gives us a smooth transition between the points of view. This peripatetic strategy has the obvious pedagogic virtue of stimulating cross-disciplinary thinking. But it also faces the pitfalls that such nonlinear approaches usually do. While the facts of hyperbolic geometry and linear algebra that I need are fairly elementary, the physical intuition required to apply them to this problem depends strongly on the experience and the imagination of the reader.

This story has a subplot: I wish to cultivate for students of mathematics the visual and physical intuition on which special relativity is based. The section Light Rays, Clocks, and Rulers: A Visual Primer in Special Relativity is my modest attempt to do that. Throughout the story, however, I have tried to recruit 3-dimensional graphics in a variety of ways to support this visual intuition, and so I recommend that beginners experiment with the interactions (in the dynamic microworld) if that is at all practicable.

I do not wish to slight hyperbolae, for which there are analogous "focus-locus" and "focus-directrix" characterizations. There is a similar relativistic experiment that provides motivation and insight into that property. In order to keep this story within bounds, I have reluctantly decided not to include it here but to leave it to your initiative and imagination to extend these ideas to include hyperbolae.

Henri Poincaré (Poincaré, 1952) was one of the first to point out the role of groups of physical motions in determining what part of geometry is "invention" (or "convention") and what part is an expression of the biological and psychological inheritance that constrains the way we can think about the world. This is a theme that Jean Piaget (Piaget, 1971) developed throughout his life, and it has much to say about the epigenetic basis of mathematical knowledge. The Lorentz group and the Poincaré group of special relativity describe a highly nonintuitive constraint on the way that we can view the physical world, insofar as they describe experiments (about the propagation of light) that do not fall into the domain of ordinary human experience. But they also describe a geometric view of nature (Whitehead, 1919) that cannot be dismissed as "invention." This geometric and dynamic view of nature weaves "time" and "space" into a whole in which each loses its individual identity, as Minkowski observed.

Now it may seem surprising that such an esoteric view, rooted as it is in a sophisticated physical interpretation of the natural phenonema, can have something useful to say about the elementary geometry of conic sections. But when physical interpretation can build a bridge between mathematical concepts, both the mathematics and the physics are thereby enriched. In this case, physics gives a synthetic interpretation of the focus-locus definition of conic sections and attaches a straightforward (if somewhat surprising) meaning to the sum of distances to foci that appears in the construction.

In the next section, Planes Intersecting Cones, I will develop some of the details of the construction in a special case that that will later be the basis of our general strategy. In Hyperbolic Geometry of 2+1 Spacetime, I will show that conic sections are also "conic intersections," that is, intersections of light cones, in order to set the stage for the physical point of view (special relativity). Next follows a short discussion and an experiment in special relativity: Light Rays, Clocks and Rulers: A Primer in Special Relativity. With these preliminaries aside, I will describe A Thought Experiment that will give a simple way to think about the focus-locus property of ellipses while connecting it to the Apollonian plane-slicing-cone view. The final section, Interpretation of the Experiment, brings all of the facts together.

  Mathwright Microworld for this page

---

I will develop the relationship between the plane-slicing-cone picture and the focus-locus and focus-directrix properties in three stages. On this page, I sketch in a special case the strategy that I will use in 3-dimensional Euclidean Geometry.

Recall that we use a "standard" cone in Euclidean space given by the equation

,

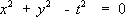as shown in the picture at the right. (Later we will interpret such a picture as a "light cone" at the origin event.) The axes are of course, orthogonal in the Euclidean metric.

Next, consider the hyperboloid (see the next figure below) defined by the equation

.

We know that each point in the interior of the cone determines a plane. Our special case will consist in choosing to belong to the upper half of the hyperboloid. (In special relativity, this hyperboloid has special significance.)

For an arbitrary choice of A and B, select the point  =  on the hyperboloid. Let , and assume that . The plane tangent to the hyperboloid at that point intersects the cone in an ellipse.

I explain in Note 2 that the center of this ellipse is  , and that it has semi-major axis of length

generated by at and semi-minor axis of length 1, generated by at .

Note 2. The Apollonian ellipse in standard form

From this, it is easy to calculate that the foci are at points:

Now this calculation tells us nothing about the focus-locus or the focus-directrix properties of . In fact, there is no directrix evident in the construction. For these, I consider a different ellipse.

Consider the plane (the plane) that is perpendicular to the axis of the cone and passes through the vertex, and let be the Euclidean orthogonal projection to this plane. Then the ellipse that I consider is the projection of to the plane. I denote that projection .

In Note 3, I show that is an ellipse. First, I represent the curve implicitly, and then I use an explicit parametric representation to cast it, after translation and rotation, in the form

,

for some .

Note 3. The projected curve is an ellipse in standard form.

Notice that there is a natural choice for the directrix for this ellipse, which I will justify below. Consider the intersection of the tangent plane, the graph of a function of x and y,

,

with the plane . Since we assume that , this intersection is the line

.

Special relativity will establish that has the focus-locus property, and that the line is the directrix for for which the focus-directrix property is true. We will see that on the Interpretation of the Experiment page.

Characterization of similarity classes of ellipses

We are now in a position to describe the similarity classes of (noncircular) ellipses in the plane. These classes are overspecified by requiring that they have

1. a focus at the origin (0, 0),
2. center at a point ,
3. semi-minor axis of length 1 (and therefore semi-major axis of length ).

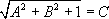It is enough in fact to choose the center and then to select the point = on the hyperboloid . The plane tangent to the hyperboloid at that point intersects the cone in the ellipse . And then the projection will satisfy the three conditions above. We will then also know the directrix and the eccentricity of , as we shall see.

If we let the unit vector , so that , we can picture the sequence of three points

as the points in the plane at times 0, 1, and 2 occupied by a uniformly moving object with (classical) velocity . When this picture is interpreted relativistically, we will also have an interpretation of the ellipse itself, as well as the focus-locus and focus-directrix properties.

In the following picture I specified only the point , the tip of the red arrow, to determine the light blue ellipse and its directrix and foci.

In Note 4 I interpret the directrix and eccentricity in this Euclidean setting. Later I will give the relativistic interpretation.

Note 4. Directrix and eccentricity of the projected ellipse

When we interpret the ellipse dynamically using special relativity, we can conclude that is the eccentricity of the ellipse characterized above. As mentioned, special relativity will establish that has the focus-locus property and that the line is the directrix for for which the focus-directrix property is true.

Here is the bridge between this Apollonian plane-slicing cone picture and the focus-locus picture:

Every ellipse that we construct via the process belongs to some hyperboloid

.

The planes that are tangent to the unit hyperboloid , as in the calculation above, form a special class. Their intersections with the cone will be called "boost intersections" -- they come from the case k = 1. Therefore, the ellipse obtained in the general case is simply a scalar multiple of a boost intersection. Scalar multiplication preserves similarity, and so every slice intersection is geometrically similar to a unique boost intersection.

Now I will interpret the focus-locus and focus-directrix properties, not for the slice intersections , but for their orthogonal projections under to in the plane perpendicular to the axis of the cone. The physical picture is simplest when we study a boost intersection, but it is easy to extend to all slice intersections.

Since the focus-locus and focus-directrix properties of ellipses are properties of their similarity classes , it is enough to establish and interpret them for the projected ellipses just studied. To do that, we must now recognize that the ellipses arising in this case (and in general, in fact) are "conic intersections." For that, I will develop the hyperbolic geometry I need on the next page, Hyperbolic Geometry of 2+1 Spacetime.

It is easy to see that for a general point , say, , the construction gives an ellipse whose eccentricity is , since scalar multiplication by transforms , and the latter gives a boost intersection with .

  Mathwright Microworld for this page

---

In order to develop the physical interpretation of the conic construction that I made on the previous page, I will now replace the 3-dimensional Euclidean geometry of with 2+1-dimensional Hyperbolic geometry. That geometry is determined by the "Hyperbolic metric" for , as opposed to the Euclidean metric.

Like the Euclidean metric, it is defined by a non-degenerate inner product, but unlike that metric, the inner product is not positive definite, as I shall explain below. In fact, there is a 2-dimensional stratified set of vectors called the "light cone" with the property that their "lengths" are zero. This lends the geometry a distinctive and interesting character. Still, many of the familiar properties of Euclidean geometry have their analog here. And in particular, as I shall show on this page, the ellipses that I constructed earlier by slicing the standard cone with a plane, may also be realized by forming the intersection of two light cones.

This is of course a preliminary for our physical interpretation of the focus-locus and focus-directrix properties. In the Thought Experiment and Interpretation of the Experiment sections, I will take the third step, and interpret that property using special relativity restricted to a 2+1-dimensional spacetime.

The geometric structure of that I will use from now on is determined by an inner product. I continue to use the Cartesian coordinates to specify that inner product, though it is understood that the inner product itself is an underlying structure that is independent of any particular choice of coordinates. That structure remains covariant under a wide class of linear transformations (Lorentz transformations) that preserve the inner product, just as the Euclidean geometry remains covariant under all rotations and inversions across planes. The inner product is defined, then, as follows:

Suppose that . I will use the words "points" and "events" interchangeably in anticipation of the discussion to come later.

Then say that the inner product of W with Z, which I shall denote is

(6.1)

Note that this implies that the light cone at the origin is defined as the set of vectors such that . If we denote by the vector , by the vector and by the vector , then in this hyperbolic metric:

(6.2)

I use the suggestive name for the third vector because in the physical interpretation, in which t represents the time, the name will do double duty as the vector which is the event of the first clock tick on the world-line of a special (stationary) observer, and as the name of the stationary observer itself. The vectors are "orthonormal" in this metric in the above sense.

This "hyperbolic" metric defines, for each pair of events in , a number: . This number may be positive, negative, or zero. I'll call that number the "hyperbolic interval" between events . Obviously this is equal to . The number is analogous to the "squared distance" between points in the Euclidean metric.

I take the liberty of coloring my prose a little (and anticipating the physics somewhat) by using terms like "light rays", "signals", "clocks" and "observers". I will say more about these ideas on the Clocks, Light Rays, and Rulers page. If the hyperbolic interval is zero, it means that a ray of light connects . If negative, it means that are causally connected, in the sense that some inertial observer may go from one of these to the other: each lies in the interior of the light cone of the other. And this means that a slower-than-light signal may pass from one to the other, so that one definitely precedes the other.

If the hyperbolic interval is positive, it means that each event lies in the exterior of the other's light cone, and the events are not causally connected. No signal may pass from one to the other, and for some inertial observers, , while for others, , and for yet others, events are simultaneous.

The following physical aside is the basic physical postulate that Einstein set down for special relativity (3+1 Hyperbolic geometry), defining, in a sense, the class of allowable geometric transformations from one inertial observer to another: The hyperbolic interval separating two events is the same (number) no matter which coordinate system of an inertial observer is used to measure the coordinates of the events. This is true as long as all inertial observers choose compatible units of measure, and use those units for all measurements. This means that it must be possible to synchronize their clocks when they are pairwise stationary with respect to one another, and that each measures the speed of light to be one unit distance per unit time. When they are in uniform motion with respect to each other, each uses his own system of coordinates to describe events, but they still measure the same hyperbolic interval between any two events. In fact, it is possible for an observer to measure this interval using clocks and light rays alone. An experiment on the next page: Clocks, Light Rays and Rulers, will allow you to see that for yourself.

In order to discuss the plane-slicing-cone construction in a physically unified way, I introduce some geometric lemmas. These lemmas generalize some obvious facts about ordinary with its Euclidean metric. They are rather trivial, but they point the way to the physical interpretation of this geometric operation.

Lemma 1 (Hyperbolic orthogonal bisector)

Suppose we are given two distinct events, , in 2+1 space with its hyperbolic metric . Let be the midpoint of the segment they determine. The set of vectors with the property that is a plane. This plane is the orthogonal bisector of the segment.

Note 5. Proof of Lemma 1

 

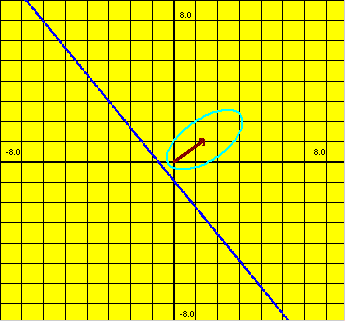
Of course, the hyperbolic orthogonal bisector of a segment does not appear perpendicular to the segment, as it would be in the Euclidean metric. For example, the "light ray" is orthogonal to itself! The orthogonal bisector of the "light segment" connecting

is the set of events

,

which contains the segment. Generally, though, the picture might look something like the figure at the right.

Lemma 2

Suppose we are given two distinct events in 2+1 space with its hyperbolic metric . Let be the midpoint of the segment they determine. The set of points with the property that the interval from to equals the interval from to , that is, such that

,

is a plane, and this plane is in fact the orthogonal bisector of the segment determined by .

Note 6. Proof of Lemma 2

 

Lemma 3 (Conic Intersections)

Suppose we are given two distinct events in 2+1 space with its hyperbolic metric . Let be the midpoint of the segment they determine, and let plane be the orthogonal bisector of the segment passing through . Let be the light cone with vertex at and be the light cone with vertex at . Let be the hyperbolic interval from :

.

If , then

,

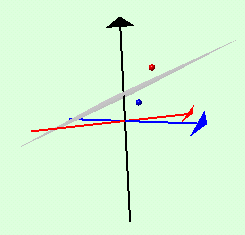
and this intersection is either an ellipse or an hyperbola.

There are two cases (see the figures below):

1. If , the hyperbolic interval is "time-like", and the common intersection is an ellipse.
2. If , the hyperbolic interval is "space-like", and the common intersection is a hyperbola.

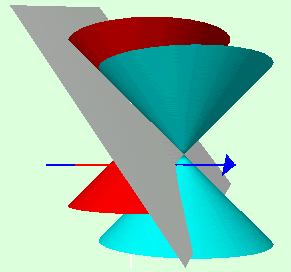An Elliptic Conic Intersection	A Hyperbolic Conic Intersection

Note 7. Proof of Lemma 3

 

I discuss the idiom of Special Relativity in the next section. And in A Thought Experiment, I will interpret the focus-locus definition of conic sections in terms of light cones.

  Mathwright Microworld for this page

---

Special relativity asserts (Einstein, 1921) that there exist frames of reference, called "inertial frames", with respect to which the laws of physics have the simplest possible expression and the equations all have the same form (principle of covariance). These inertial frames are, of course, a convenient fiction, giving only the infinitesimal approximation of the state of affairs in the presence of matter and energy. It is essentially the same as saying that a curved surface is "locally - in infinitesimal approximation" the same as a Euclidean space.

The inertial frames are what an observer uses to coordinatize the "world". And the world is space-time, or the world of "events". Inertial observers may associate numbers (coordinates) to the world of physical events, and once they do, they may derive relations among those numbers -- for example, that undisturbed bodies (such as the world lines of other inertial observers) describe straight lines, and that these straight lines have rulings determined by the ticking of the mover's clock.

Relativity asserts that all inertial coordinatizations of space-time are equivalent, in the sense that one cannot be distinguished from the other by any physical experiment. In particular, it is possible for inertial observers to come to agreement about their units of measure while they are stationary with respect to each other, and that, after this agreement is made, all will measure light to move at the same constant speed. This will be true whatever the subsequent state of relative motion of these observers.

This is astonishing, but it is what both experiment and Maxwell's Equations imply. All kinematic parameters may be expressed in terms of "light rays and clocks" from this viewpoint (Burke, 1985). The basic meaning of the principle of relativity is that there is no "privileged" viewpoint for the events in the physical world, but that there is a privileged class of inertial viewpoints, each equivalent one to the other, each indistinguishable from the others. They all describe the laws of physics in the same way.

To simplify the discussion, we suppress one of the dimensions of space-time and consider, instead of our four-dimensional space-time, a three-dimensional space-time. In this space-time there is a coordinatization by an inertial observer for which the velocity of light is 1. That is, light moves one unit distance in one unit of time. I shall call the coordinates of this space-time, as used by the inertial observer, .

To develop the physical interpretation of the focus-locus and focus-directrix properties of conic sections, I will work in this 2+1 dimensional space-time. As a preview, I mention that 2+1 space-time is a 3-dimensional vector space with a geometry very much like Euclidean geometry. That geometry is determined by the "hyperbolic metric", which I defined earlier.

Let us suppose that a "standard" observer is selected. Call that observer , and refer all spacetime events to the coordinates that attaches to them. As I mentioned earlier, the name will refer ambiguously to the observer himself and to the event of his first clock-tick. In this case the event has standard coordinates . Now all spacetime events may be referred to these standard coordinates, for convenience of description. I call the event

the Origin event.

Next, suppose that there is another inertial observer. I assume that this observer has previously synchronized clocks and rulers with those of as described above. Call this observer . The new observer describes spacetime events with coordinates, say, , and I assume that the event that describes as

is the same Origin event as that of . I will also refer to the event of the first clock tick of with coordinates as .

We notice two things according to the covariance principle. If, in standard coordinates, the light cone at the origin is the set of events given by the equation

as measured by , then it coincides with the set of events given by the equation

as measured by . That is, and see the same light cone at the origin. But for an individual event on the light cone, they might assign different coordinates.

The same is true for the unit hyperboloid represented in standard coordinates as

.

While the event on that hyperboloid has standard coordinates , the event on that hyperboloid will in general have standard ( ) coordinates different from if is "in motion" with respect to .

There is no absolute notion of simultaneity in Special Relativity, but each observer may define his own concept of simultaneity with a certain simple experiment with light and his clock that I will illustrate on this page. In particular, the events that observer considers to be "simultaneous" with his first clock-tick (the event ) will be the plane tangent to the standard hyperboloid

at the point . This is the plane for the observer . We saw this plane in the Planes Intersecting Cones page. The intersection of this plane of events with the light cone at the origin was seen to be an ellipse in standard coordinates, with the Euclidean metric. That was the ellipse of the plane-slicing cone construction.

I emphasized standard ( ) coordinates because, from the point of view of , that set of events in the plane are simultaneous, and their intersection with the light cone at the origin forms a circle of radius 1. The points on this "ellipse" will not have constant t coordinates from the point of view of , however. Now if is actually in motion with respect to then we have the following remarkable fact that will explain why an ellipse that is not a circle has a directrix. The plane of simultaneity for intersects the plane of simultaneity t = 0 for in a line!

Note 8. Digression

 

Let us illustrate with a picture. Suppose that our standard observer establishes the basis of coordinates for this 2+1 dimensional space-time. And suppose now that there is another inertial observer whose world line intersects the world line of at the origin, as above.

In particular, I assume that both and have clocks that tick time 0 for each at the origin event. What does this mean? The simplest way to picture it is to use the coordinates of to describe the event of the next tick of the clock of on the world-line of . Certainly this event must occur in the future light cone of . But it cannot be just anywhere, for the following reason. The hyperbolic interval connecting the zero-th and first clock tick of along the world-line of is obviously measured by to be -1. Therefore, it must be -1 in the coordinates of . This means that, in the coordinates of , it lies on the hyperboloid

as I observed above. The situation might be pictured as in the figure at the right.

From the picture, it is clear that the next clock tick of after the origin event must occur at a time that will measure to be greater than or equal to 1. (This is the famous "time-contraction" -- the clock of appears to be slower than that of .) It will be equal to 1 if and only if is not moving at all in the reference frame of , in which case the world-lines and the clocks of and coincide. The state of motion (with respect to ) of observer is almost determined by this picture. It is not entirely determined, because still has some freedom in the choice of his spatial coordinates (vectors ). In order to make the analysis of the conics, I will have the standard observer choose the system of vectors in a special way.

The line (the t-axis) is what I called the "world-line" of the observer . Points on the world-line are measured by the unit ticks of his clock. In this spacetime, a circular pulse of light emitted from the event describes a cone (the light cone) of events along the wave-front of that pulse. It is described by the equation:

.

A picture of the light cone (see the figure at the right) suggests that his world-line is "inside" the cone. It points "upward" in the direction, towards the "future" for the observer.

Now, while the axes are orthogonal in the Euclidean metric, the correct measure of distance in this 2+1 spacetime is given by the "hyperbolic" metric. Recall that, if

,

then the inner product of , denoted , is

.

All events in the observer's future are in the upper half of the light cone when we stipulate that the observer's "present" is the origin . In this sense, the observer can influence these future events from the "present" event by sending a signal (an inertial observer, or a ray of light) to them from the origin event, or from any event further along his world-line.

All events that are in the observer's past are in the bottom half of the light cone. An event is in the observer's past if a signal may come from that event to the origin, the present event .

In fact, it is possible for an observer to measure the interval between the origin event and any other event using clocks and light rays alone. An experiment on the dynamic version of this page will allow you to see that for yourself. It is worth our while to elaborate the last point, because it will give insight into our line of attack on conic sections. Assume that is an inertial observer whose clock has been synchronized with that of observer as above, and for whom light travels with velocity 1. Suppose also that are events.

Imagine that at time , observer passes through event . Now it is almost obvious that a unique ray of light may be emitted at some point on the world-line of that will meet event . Let be the time (as measured by ) of emission of that ray of light. And there is another unique ray if light that may be emitted from the event that will arrive back on the world-line of . We may consider this to be the reflection by a circular mirror situated in . That reception event will occur at a time on the world line of . Obviously, .

Now it takes light just as long to travel from the event at time on the world-line of to as it takes to travel from back to event at time on the world line of . Thus, in coordinates, the time coordinate of event is midway between and . It is

.

Also, since light travels with speed 1, the spatial coordinates of , , satisfy

,

because that is how long it took light to reach .

Thus, when calculates the interval from to , he gets

can, in principle, make this measurement just with light (and a mirror) and his clock. In particular, if this product is 0, then are connected by a light ray. If the product is positive, then must occur in the past of (before event on his world-line). And that would mean that have a space-like interval.

I put these ideas to use in the next section, A Thought Experiment, where I interpret the focus-locus definition of conic sections in terms of light cones.

 

  Mathwright Microworld for this page

---

I am now ready to describe the experiment that displays the similarity class properties: the focus-locus property and the focus-directrix property of the ellipses constructed in the Planes Intersecting Cones section. I give a sketch first, and then discuss the details. You might like to keep the calculation of that page in mind, where the point of the hyperboloid

represents the first tick of the moving observer's ( ) clock in standard observer's ( ) coordinates. The speed that attributes to is in that case

.

Sketch of the experiment:

Suppose a "standard" inertial observer: is situated at the origin of the Euclidean plane. This observer watches a "moving" inertial observer pass through the origin at a time that they both measure to be 0. considers to be moving at constant velocity where is a unit vector and is the speed. I assume for this experiment that .

Both observers measure the speed of light to be 1. At the event where arrives at the origin it emits a circular pulse of light. From the point of view of , after one unit of time as measured by the wavefront forms a curve of events whose spatial coordinates simply form a circle centered at his origin. The events in the wavefront all have time coordinate for .

However, from the point of view of , this curve of events is the intersection of a plane with a cone in his coordinatization of spacetime. I called the intersections obtained physically this way, boost intersections: . The time coordinates that he measures for points in that wavefront are not constant, but, as we saw, the curve forms, in the Euclidean structure that the plane inherits from the hyperbolic geometry, an ellipse. That ellipse is the intersection (in 2+1 spacetime) of a certain plane with a light cone. Observer projects this ellipse into his (Euclidean) plane t = 0, and discovers another ellipse: , this time with one focus at the origin . The ellipse has semi-minor axis 1, and semi-major axis directed along .

If we imagine that each ray of light emitted by is reflected back to at then they all converge again at at a time that measures to be .

For , each of those rays of light projects to a pair of intervals, the first, starting at the origin, a focus of the projected wavefront ellipse, and arriving at the ellipse, and the second reflecting to the other focus, which will be the spatial position that ascribes to at time . We will see in Interpretation of the Experiment that the sum of the two lengths of these intervals is, for each ray of light, the time coordinate that ascribes to the arrival back at of the reflected ray. These time coordinates are the same for all the rays, because their convergence is at a single event, clearly simultaneous both for and .While measures that time to be 2, measures it to be the time coordinate of the event: " at time ." That number is easy to calculate. It is the length of the major axis:

Since 2 < , this is another example of "time dilation." Observer thinks that more time has passed than the 2 units that observer measures at the event: " at time ."

Obviously, every ellipse in the Euclidean plane can be obtained up to similarity in this way since these ellipses have semi-minor axis 1, and semi-major axis . This will establish what I call the focus-locus property for all ellipses, and will give a physical interpretation for the constant sum of distances from the foci to the points on the ellipse.

Now to interpret the focus-directrix property, I first identify the directrix of ellipse in this experiment. This will be the intersection of the plane with the plane t = 0. On our assumption that this intersection is a line in the plane of . This will give an interpretation of the directrix, and then of the eccentricity of as the tangent of the dihedral angle thus formed in the Euclidean metric. We will see the details in the Interpretation of the Experiment section on the next page.

Further, it is clear that every intersection of a plane with the light cone that is the result of the for arbitrary in the interior of the cone can be associated with a unique boost intersection with the physical interpretation just described. These intersections are geometrically similar, and are obtained by simple scalar expansion or contraction from the origin. Therefore, their projections are also geometrically similar. In the experiment, I work only with the that derive from the boost intersections, that is, from planes tangent to the hyperboloid:

That's the sketch. What makes this work, as we will see, is the fact that every ellipse of the form is a conic intersection, i.e., the intersection of two light cones. I will first establish some notation to set up the experiment.

Let us suppose that our standard inertial observer establishes the basis of coordinates for this 2+1 dimensional space-time. As before, I will let the name stand for the observer and the event of his first clock tick. Suppose now that there is another inertial observer, say , whose world line intersects the world line of at the origin.

In particular, I assume that both and have clocks that tick time 0 for each at the origin event. As before, I will picture this by using the coordinates of to describe the event of the next tick of the clock of on the world-line of . This event must occur in the future light cone of , as we saw earlier. Since the hyperbolic interval connecting the first and second clock tick of along the world-line of is measured by to be -1, it must be -1 in the coordinates of . This means that, in the coordinates of , it lies on the hyperboloid

,

as in the figure at the right.

For the next step in setting up the experiment, I will choose the orthonormal system of vectors (in the hyperbolic metric) in a special way. We are free to choose any orthonormal system we like because of the covariance principle, so I select one adapted to the motion of in the following way.

Note 9. Orthonormal hyperbolic coordinates

Now let's describe the experiment in these terms. For observer moving with respect to an observer in such a way that their origins coincide, I will construct the ellipse formed by intersecting the light cone at the common origin with the hyperbolic orthogonal bisector of the segment from the origin to the event of the second clock tick of . All of this is easily formulated as a "thought experiment." This experiment will lead us, in this section and the next, to give the "physical" interpretation of the focus-locus and focus-directrix properties of an ellipse.

Here is the experiment performed by observer . At his time 0, emits a circular pulse of light. In his spatial coordinate system, he has a circular reflecting mirror of radius 1 with center at his origin. (Imagine a cylinder surrounding his world-line.) At , the wavefront arrives at the mirror, and is reflected back. It arrives back at the origin at .

In the coordinate system of , nothing interesting happens. The picture looks like this:

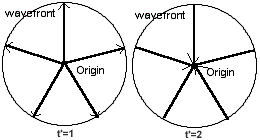
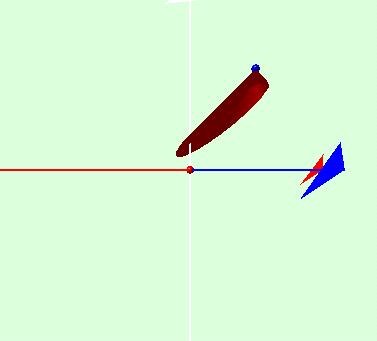
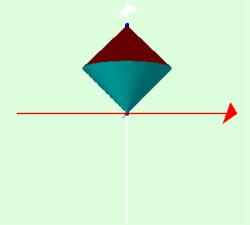
In 2+1 space-time, the process is as shown in the following figure, with emission phase the lower (green) part of the cone, and reception phase the upper (red) part of the picture. Light simply moves from the center of his circle to the boundary after 1 second, then returns to the origin after 2 seconds.

Now let's examine the same process from the viewpoint of . In his coordinates, will be "moving" if the velocity .

The following figures illustrate the two halves of the experiment in his coordinates. In the first picture, the emission cone from the origin of coordinates is shaded gray. The reception cone -- the path of the reflected wavefront is shaded white.

The wavefront spreads from the origin along the gray section of the light cone. At time , it forms a curve obtained by intersecting the light cone at the origin with the plane that is the orthogonal bisector of the segment connecting the events and  on the world-line of . We saw in Geometry of 2+1 Spacetime that the points on the curve of arrival events must have equal hyperbolic interval (that is, 0) with the emission and reception events, hence must lie on the orthogonal bisector plane, . In the second picture above, I show three light "rays" emitted in three directions.

The following 3-dimensional picture shows this orthogonal bisector plane in grey, and it also depicts the x-y plane t = 0 in yellow. These planes intersect in the line that I have designated the directrix in the x-y plane that contains the projected ellipse (not visible in this picture).

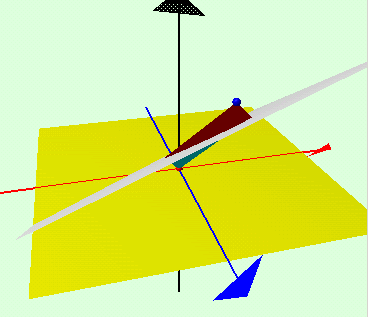
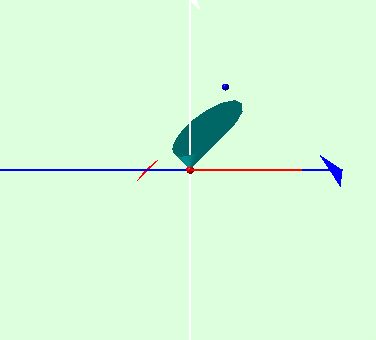
In the figure at the right, I show the projection of the picture onto the x-y plane of observer . I project the light rays also. We will see later that these light rays travel from one focus to the other of this projected ellipse. This indicates that the bridge from the plane-slicing-cone picture to the focus-locus picture is obtained by projecting the slice ellipse into the spatial ( ) plane of observer . We will see in  Interpretation of the Experiment exactly why this is so.

We saw in Planes Intersecting Cones that this plane is also tangent to the hyperboloid at vector ,

,

by observing that, in the Euclidean metric, the vectors are perpendicular to the gradient of the hyperboloid at that point. This Euclidean calculation gives the same result in the present hyperbolic context, since generate the plane at .

As we saw above, the wavefront then follows the inverted cone back to the reception event at . The skewed and unsymmetrical aspect of the pictures is the result of the fact that observer is actually moving with respect to observer .

From the plane-slicing-cone definition of a conic, we see that the intersection of the two light cones is, in this case, an ellipse. That is, the events in the light cone along the wavefront at form an ellipse ( where = ) in the frame of . But since is using a hyperbolic and not a Euclidean metric, let us examine the equation for this intersection curve in coordinates.

The points on this curve are not "simultaneous" in the frame of (although, of course, they are simultaneous in the frame of ). This curve is, however, obtained by intersecting the light cone with the plane through vector that is parallel to vectors , since these vectors generate the orthogonal bisector according to the relations derived above,

If we let define coordinates for the plane in the obvious way, then they will give Euclidean coordinates for the plane satisfying (even in the hyperbolic metric),

,

and the points of the form

,

are in the curve, since, as is easy to see, in the hyperbolic metric,

.

In the standard Euclidean metric for , using coordinates, are still perpendicular, but while =1, in general, . So the intersection is an ellipse in the plane if we use the induced Euclidean structure from _.

 

Emission Phase	Reception Phase
Both Phases	The light cones

Having developed these pictures, we now ask what the interpretation for the focus-locus and focus-directrix properties of the ellipse is. In the next section, Interpretation of the Experiment, we will give what may be a surprising answer to this question.

  Mathwright Microworld for this page

---

In order to understand what the experiment means, we must write down the equation of the curve defined by projecting the wavefront curve at to the (standard) coordinates of the plane of . I do this by introducing certain coordinates for the plane of . (We saw this in a Euclidean context in Note 3 of Planes Intersecting Cones.) The "speed" of (as measured by ) is defined to be

.

I assume for this experiment that -- otherwise, there is nothing to do.

We saw that the wavefront curve was an ellipse in the (stationary) frame of by using the basis in coordinates, given by

,

,

,

where these vectors satisfy the hyperbolic orthogonality relations,

Note 10. Coordinates adapted to the projected ellipse

We see that there are orthogonal unit vectors U and V in the plane of so that the projected ellipse is given by the parametric equation

(9.1)

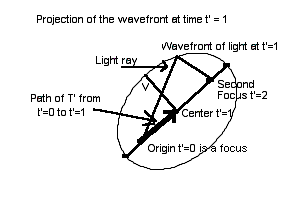From this it follows that the projected curve is an ellipse with major axis on the line generated by , as illustrated at the right.

Here is the interpretation: The projected ellipse is the projection to the plane of the observer of his measured spatial positions of the wavefront at the observer's time . It is not a circle because this wavefront does not consist of simultaneous events for (since ).

The major axis of this ellipse contains, between the focus at the origin and the second focus, the line of spatial positions of the world-line of between his time . In particular, he moves from the origin (first focus) to the center in 1 unit of his time, which is units of the observer's time. His projection must therefore move to the second focus at his time .

The rays of light, emitted at various angles from the emission event, project to segments connecting the first to the second focus via a point on the boundary of the projected ellipse. The questions, of course, are:

• Why should the sum of the lengths be constant?
• What does this constant sum represent?

And the answers will now be fairly easy to see.

Consider a ray of light that bounces from the emission to the reception event. Describe its itinerary in the reference frame of observer . It starts from the origin event

and arrives at the mirror at event

.

Next, it reflects to the reception event,

.

In the first leg of its itinerary, it lies in the light cone, so

.

Since the square roots of both sides of this equation are equal, we conclude that the spatial projection of that path has length , which is , since .

In the second leg of its itinerary, it also lies in a light cone, so

.

For the same reason, the spatial projection of that path has length .

Therefore, the sum of the spatial lengths is . This is equal to the time that observer measures from the emission event to the reception event. That is, of course, the time coordinate in the frame of of the event . But from the definition of in the previous section,

,

we see that this is

.

And this, of course, is the length of the major axis of the projected ellipse. That is a physical interpretation of why the sum of the lengths of the paths from a point on the ellipse to its foci is constant. It is the time measures between the emission and reception event, which is the same for all rays of light.

Next, I consider the focus-directrix property of the ellipse. I showed in Planes Intersecting Cones that the intersection of the plane of simultaneity  for  with the plane of simultaneity  for is a line that I call :

.

I represented the tangent plane, the plane of simultaneity  for ,  as the graph of a function of x and y:

.

In the present context, the function has the following interpretation. With each point there is a unique point on the plane of simultaneity   for that projects to it. is the time coordinate that standard observer ascribes to it. The line is the set of events with and .

Note 11. Interpretation of the eccentricity

The calculation above shows that the value of the eccentricity is the speed of as measured by ,

= ,

and it gives us the focus-directrix property of the projected ellipse.

Now, we might ask how general this construction is. It is clear that, for any particular direction of motion of with respect to , we will obtain among the projected ellipses one representative of the similarity class of ellipses for each speed . And every similarity class will be represented, simply by choosing the representative with semi-minor axis of length 1, and semi-major axis of length

.

Therefore this physical argument is completely general.

What happens if is stationary with respect to ? In that case, this construction breaks down when we attempt to build the observer's orthonormal basis. But in that case, there is no need to build such a basis. The foci collapse to the center, the ellipse is a circle, and everything happens as it does in the frame of .

Burke, W. L. (1985), Applied Differential Geometry, Cambridge University Press

Einstein, A. (1966), The Meaning of Relativity, Fifth Edition, Princeton University Press

Goodstein, D. L., and J. R. Goodstein (1996), Feynman's Lost Lecture: The Motion of Planets Around the Sun, W. W. Norton & Co.

Piaget, J. (1971), Biology and Knowledge, An Essay on the Relations between Organic Regulations and Cognitive Processes, The University of Chicago Press

Poincaré, H. (1952), Science and Hypothesis, Dover Publications

Whitehead, A. N. (1919), An Enquiry Concerning the Principles of Natural Knowledge, Cambridge University Press