An Interactive Use of the Lanchester Combat Model - Approximating the Model
When applied to a large but finite number of steps, difference equations can closely approximate the continuous behavior of differential equations. In fact, the continuous model can be seen as a limit of the discrete model. To illustrate this point, we consider the generic Euler discrete approximation method, derived by letting a difference quotient approximate the derivative:

In discrete terms, it is easy to measure the changes occurring on the battlefield by comparing the numbers of troops of each force at different time periods. If we take equal time steps  , and then use this common time step as the unit of time (so
, and then use this common time step as the unit of time (so 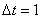 ), we have
), we have
 ,
,  .
.
So the future number of one force’s troops equals its current number of troops plus the change in the number of troops, where the change is resulting from battle and non-battle losses and reinforcements. With
 and
and  ,
,
the continuous model may be approximated by
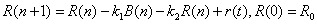 ;
;
 .
.