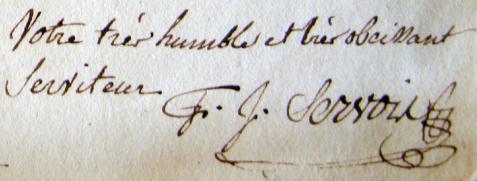Before the nineteenth century, algebra usually referred to the theory of solving equations. However, the field of algebra experienced an extensive transformation during the nineteenth century, a time period referred to by many historians as the Golden Age of mathematics. Consequently, by 1900 algebra encompassed the study of algebraic structures. One contributor to the advancement of algebra was François-Joseph Servois (1767-1847). Servois was a priest, artillery officer, professor of mathematics, and museum curator. Not only did he battle to defend Paris in 1814, but he also fought for an algebraic foundation for calculus. As we will see, Servois was an advocate of “algebraic formalism,” and the majority of his contributions to the field of mathematics fall under this category. In an “Essai” written in 1814, Servois attempted to provide a rigorous foundation for the calculus by introducing several algebraic properties, such as “commutativity” and “distributivity.” Essentially, he presented the notion of a field, an idea far ahead of his time. Although Servois was not successful in providing calculus with a proper foundation, his work did have an impact on the field of algebra, and influenced several mathematicians, including the English mathematicians Duncan Gregory and Robert Murphy. Many English mathematicians of this period used the works of French mathematicians to aid in their development of linear operator theory and abstract algebra [Koppleman 1971].

This article further illustrates that while the disciplines of algebra and analysis are studied separately today, mathematicians of the eighteenth century (and before) made little, if any, distinction between them. Finally, instructors can use the original sources found in this article to demonstrate to students the connection between classical and modern day mathematics.

According to Piccolino [1984], the nineteenth century is considered by many historians to be a Golden Age in the development of mathematics. Advancements in several branches of mathematics, such as geometry and analysis, occurred during this revolutionary time period. Another area that experienced change was algebra [Piccolino 1984]. Prior to the nineteenth century, algebra usually referred to the theory of solving equations; however, by 1900 it involved the study of mathematical structures, such as groups, rings, and fields [Katz 2009]. Mathematicians found that these structures often did not share properties found in the real and complex number systems, such as commutativity. This fundamental change in algebraic thought is characteristic of modern or abstract algebra.

Figure 1. A stamp issued in the Soviet Union in 1983 portrayed Al-Khowârizmî (public domain).

One of the earliest instances where we see a mathematician's work on the solvability of algebraic equations is in the writings of Mohammed ibn Mûsâ al-Khowârizmî (ca. 790 CE - ca. 850 CE). Al- Khowârizmî provided solutions to linear (first-degree) equations and quadratic (second-degree) equations, but his results were presented verbally, without the use of algebraic symbols [Dunham 1990]. Al-Khowârizmî did not recognize either negative coefficients or negative solutions in his general solution to the quadratic equation \(ax^2 + bx + c = 0\), which he broke up into six cases. According to David Eugene Smith [1958], the first significant treatment of negative numbers was by Girolamo Cardano (1501-1576) in his 1545 book on algebra, Ars Magna. The first consideration of imaginary solutions occurred a few years later when Rafael Bombelli (1526-1572) used imaginary numbers as a “tool” for solving cubic equations [Dunham 1990, pp. 150-151]. According to Victor Katz, Bombelli's work “provided mathematicians with the first hint that there was some sense to dealing with” imaginary numbers in their algebraic work [2009, p. 407]. 

Figure 2. Joseph-Louis Lagrange (public domain).

Initial developments in abstract algebra occurred in Continental Europe during the late eighteenth and early nineteenth centuries. These changes were driven by problems in classical algebra, such as the solvability of third, fourth, and higher degree equations [Piccolino 1984]. The works of Joseph-Louis Lagrange (1736-1813), Augustin-Louis Cauchy (1789-1857), Paolo Ruffini (1765-1822), Niels Henrik Abel (1802-1829), and Evariste Galois (1811-1832) were of central importance during this time period and contained several concepts associated with modern group theory.

Figure 3. Augustin-Louis Cauchy. Painting by Jean Roller ca. 1840 (public domain).

Lagrange explored the solvability of equations via the theory of permutations. Cauchy also made contributions to the theory of permutations by introducing concepts such as the identity permutation, a permutation that does not change a given arrangement of objects. Furthermore, Ruffini made several attempts at proving that the general equation of degree five is unsolvable in terms of radicals. Although Ruffini's efforts were not successful, his work provided a foundation for Abel's proof that such a solution cannot exist [Katz 2009]. Finally, Galois made significant contributions to the theory of solvability of algebraic equations by studying the structure of algebraic equations, particularly what he called “the group of the equation” [Katz 2009, p. 726]. We also owe to Galois the first known use of the term “group” in mathematics, which appeared in 1830 [Boyer 1989].

Although the work on algebraic solvability was carried out on the Continent, it was the British school of algebra that was primarily responsible for the shift in algebraic thinking towards abstract structural properties. As we shall see, this was not necessarily done by building on continental work on algebraic solvability, but rather by extending properties of ordinary arithmetic and of what we would call functions from analysis. Important figures in this movement included George Peacock (1791-1858), Duncan Farquharson Gregory (1813-1844), and William Rowan Hamilton (1805-1865). Peacock introduced the notions of arithmetical algebra and symbolical algebra. He defined arithmetical algebra as a universal arithmetic (using letters instead of numbers) of positive numbers [Katz 2009]. In this system, the term \(a - b\) had meaning only if \(a\) was greater than or equal to \(b\). On the other hand, symbolic algebra referred to the study of operations that were defined through arbitrary laws. In Peacock's symbolic algebra, \(a - b\) was valid regardless of the relationship between the symbols \(a\) and \(b\) [Piccolino 1984]. However, his laws in symbolic algebra were derived using principles found in his arithmetical algebra [Katz 2009]. Peacock was on the cusp of formulating an internally consistent algebra and his efforts in that direction were extended by Gregory.

Gregory, founder of the Cambridge Mathematical Journal, focused on algebraic structure. In his works, he often referred to the ideas of commutativity, distributivity, index operations (a sort of law of exponents for operators), and inverses, which he described as “circulating operations.” He also mentioned the principle of the separation of symbols of operation, crediting the French mathematician François-Joseph Servois (1767-1847) as the first to “correctly give” the procedure [Allaire and Bradley 2002, p. 410]. Gregory appears to have been one of the first mathematicians to establish a connection between differentiation in calculus and the ordinary symbols of algebra, noting that the commutative and distributive laws hold true for what he referred to as the symbols of differentiation. Despite his contributions to the development of abstract algebra, Gregory, like Peacock, maintained the stance that results in symbolic algebra had to suggest results in arithmetical algebra [Piccolino 1984].

Figure 4. Duncan Farquharson Gregory (public domain).

A new, internally consistent algebraic system was finally introduced by Hamilton with his discovery of quaternions on October 16, 1843. Hamilton extended the algebra of number pairs to ordered quadruples of numbers, \((a, b, c, d)\), and defined the quaternions as ordered quadruples of numbers that followed several rules [Katz 2009]. Most notably, Hamilton's quaternions did not satisfy the commutative postulate for multiplication [Boyer 1989]. His system was the first algebra that did not follow all of the laws established by Peacock [Katz 2009]. The freedom and structure present in Hamilton's system was unprecedented and, as a result, many historians consider his discovery of the quaternions as the beginning of abstract algebra [Piccolino 1984].

Fundamental structures in abstract algebra, such as groups and fields, were formally defined in the later part of the nineteenth century. Heinrich Weber (1842-1913) was the first mathematician to present detailed, axiomatic definitions of groups and fields [Katz 2009]. Weber's definition of a finite group was slightly different than the one that most mathematicians are familiar with today. His definition included three axioms analogous to the modern day ideas of closure, associativity, and left- and right-hand cancellation laws. The terminology of closure under an operation is first found in Saul Epsteen and J. H. Maclagan-Wedderburn's “On the Structure of Hypercomplex Number Systems,” which appeared in Transactions of the American Mathematical Society, Vol. 6, No. 2. in April of 1905 (see [Epsteen and Maclagan-Wedderburn 1905] and [Miller 2010]). Weber then showed that his three laws imply the existence of a unique identity element, and for each element, the existence of a unique inverse. He also incorporated his notion of group in the definition of a field. He defined a field as a set with two operators, addition and multiplication. In Weber's field, the entire set forms a commutative group under addition and the nonzero elements form a commutative group under multiplication as well. Weber also noted several properties of fields including the distributive law, which states that \(a\cdot (b + c) = a\cdot b + a\cdot c\) for all elements in the field [Katz 2009].

It is clear that mathematicians of the nineteenth century were concerned with foundational issues that spanned across several different areas of mathematics. Servois was no different and concentrated mainly on the foundational issues of the calculus. His attempts to settle the foundational issues of calculus were not successful [Bradley and Petrilli 2010]; however, as we will see, his work had a direct influence on the development of abstract algebra, and in particular, linear operator theory.

François-Joseph Servois was born on July 19, 1767, in the village of Mont-de-Laval, located in the Department of Doubs close to the Swiss border. Throughout his youth, Servois attended several religious schools in Mont-de-Laval and Besançon, the capital of Doubs, aspiring to become a priest. He was ordained a priest at Besançon shortly before the start of the French Revolution. He then left the priesthood in 1793 and became an officer in the Foot Artillery (sometimes referred to as the Heavy Artillery) with the outbreak of the revolutionary wars. In his leisure time Servois studied mathematics and his mathematical talents were apparent when he made improvements to one of the cannons, increasing its firing range significantly [Boyer 1895]. He suffered from poor health during his military career and, as a result, requested a non-active military position in the field of academia. He was assigned his first academic position on July 7, 1801, as a professor at the artillery school in Besançon, by virtue of a recommendation from the great mathematician Adrien-Marie Legendre (1752-1833). Throughout his academic career, Servois was on faculty at numerous artillery schools, including Besançon (1801), Châlons (March 1802 - December 1802), Metz (December 1802 - February 1808, 1815-1816), and La Fère (February 1808-1814, 1814-1815). His research spanned several areas, including mechanics, geometry, and calculus; however, he is best known for first introducing the words “distributive" and “commutative" to mathematics. On May 2, 1817, Servois was assigned to what would be his final position, as Curator of the Artillery Museum, which is currently part of the Museum of the Army in Paris. Servois retired to his hometown of Mont-de-Laval in 1827 and lived for another twenty years with his sister and his two nieces. He died on April 17, 1847. Readers interested in a more extensive biography and a review of Servois' other mathematical works can refer to Petrilli [2010].

It would be customary to include a painting or photograph of Servois in this biographical section, but there are no known images of him. However, due to Anne-Marie Aebischer and Hombeline Languereau [2010], there is now a photograph of his signature available to the public.

Figure 5. Servois' signature (April 14, 1814). Image by Anne-Marie Aebischer, courtesy of Presses universitaires de Franche-Comté, 2011.

During the years 1811 to 1817, the majority of Servois' works were published in Joseph Diaz Gergonne's (1771-1859) Annales des mathématiques pures et appliquées. Much of his work focused on what Taton [1972a and 1972b] called “algebraic formalism." In 1814, we witness Servois' first defense of algebraic formalism, when he began a heated debate with Jean Robert Argand (1768-1822) and Jacques Français (1775-1833). In 1813, Français published a paper based on the work of Argand, in which he viewed complex numbers geometrically. In modern day mathematics, the view of complex numbers in the plane is known as the Argand Plane. Servois highly criticized the work of these two mathematicians, saying: “I had long thought of calling the ideas of Messrs. Argand and Français on complex numbers by the odious qualifications of useless and erroneous ....” [Servois 1814b, p. 228]. For instance, Servois argued against Français’ geometric “demonstration,” given in the preceding issue of Gergonne’s journal [1813], that the quantity \(a\sqrt{-1}\) can be seen as the geometric mean of \(-a\) and \(a\). (In the field of complex numbers, we define the geometric mean of two real numbers \(a\) and \(b\) as \(\sqrt{ab}\).) Furthermore, he went on to state that it was in the best interest of the science to express his personal view, because in this work he saw nothing but “a geometric mask applied to analytic forms ...." [Servois 1814b, pp. 228-230]. 

Servois' fight for “algebraic formalism” continued in 1814 with the publication of his “Essai sur un nouveau mode d'exposition des principes du calcul différential” [Servois 1814a] (“Essay on a New Method of Exposition of the Principles of Differential Calculus”). This work was an extension of Lagrange's research on the foundations of the differential calculus. In his “Essai,” Servois stated his belief that the differential calculus could be unified through algebraic generality:

In the preceding article, we have sketched the set of laws that brings together and unites all the differential functions, that is, the most general theory of the differential calculus. The practice of this calculus, which is nothing other than the execution of the operations given in the definitions .... [Servois 1814a, p. 122].

The notion of algebraic generality is apparent in the opening sections of his “Essai,” where Servois essentially defined a field for his set of functions under the operations of addition and composition [Bradley and Petrilli 2010]. However, it was not Servois' intention to create formal structures within algebra, but rather he “was concerned above all else to preserve the rigor and purity of algebra” [Taton 1972a].

In this section, we make direct references to Servois' “Essai" [1814a]. Readers can find a translated version of the “Essai” in Bradley and Petrilli's paper [2010].

In his “Essai,” Servois attempted to provide a rigorous foundation for the calculus through algebra. In light of what we know today, Servois did not fully succeed in putting calculus on a mathematically correct foundation. It was Cauchy [1821] who, through his approach to calculus by means of limits and inequalities, moved the subject into the modern age. Although Servois' efforts were ultimately unsuccessful, we find several ideas associated with abstract algebra in his “Essai.”

Figure 6.  Title page of Servois' “Essai” (public domain).

In Sections 1-4 of the “Essai,” Servois presented several definitions that would be crucial to his work. He began by introducing his notation for a function as \(f\,z\),  where a modern reader would understand this as \(f(z)\). The formal definition of a function that we use today would not be introduced until 1837 by Lejeune Dirichlet (1805-1859). A reader will notice that Servois used the term function not only to describe ordinary functions of an independent variable, but also to describe operators, such as the difference and differential operators. After he presented his preliminary definitions, Servois introduced two functions or operators with special properties, namely the identity \(f^0\) and inverse \(f^{-1}\). Undergraduate students will notice that these operators and properties are analogous to the modern day notions of an identity element and inverse element. For instance, Servois explained that when the identity function or operator is applied to \(z\),  “\(z\) does not undergo any modification” [Servois 1814a, p. 96]. Additionally, Servois provided many examples of inverse functions. For instance, he considered the inverse of the sine function, noting that \[z = \mbox{sin}(\mbox{sin}^{-1} (z)) = \mbox{sin}(\mbox{arcsin}(z)).\] For the difference operator \(\Delta\), he noted that \[\Delta^n (\Delta^{-n} (z)) = \Delta^{-n} (\Delta^n (z)) = z.\]

In Section 3, Servois defined a function or operator \(\varphi\) to be distributive if it satisfied \[\varphi (x + y + ...) = \varphi(x) + \varphi(y) + \cdots.\] He presented several examples of functions and operators that are distributive and others that are not. One such distributive function was \(f(x) = ax\), because \[a(x + y + \cdots) = ax + ay + \cdots.\] Later, Cauchy [1821] showed that this is the only continuous function that satisfies this property. Conversely, Servois provided as a function that does not satisfy the distributive property \(f(x) = \mbox{ln}\,x\). He also demonstrated that the differential and integral operators are distributive.

Actually, undergraduates are exposed to specific examples of Servois' distributive property in a first-year calculus course, namely differentiation and integration under the operation of addition. Additionally, students see a generalized version of Servois' distributive property in a beginning linear algebra course. One of the properties of a linear transformation is that it preserves the addition operation.

Finally, in Section 4, Servois stated that two functions or operators \(f\) and \(\varphi\) are commutative between themselves if \[f(\varphi (z)) = \varphi (f (z)).\] For example, he stated that \(z\) commutes with any constants \(a\) and \(b\) because \[abz = baz;\] however, the sine function is not commutative with any constant \(a, a\not= -1,0,1\), because \[\mbox{sin}(az) \neq a \mbox{sin}(z).\]

If we consider the commutative operators \(f(z)\) and \(\varphi (z) = kz\), where \(k\) is any scalar, \[f(kz) = kf(z),\] then we have the familiar scalar multiplication property that undergraduates would see in a first-year calculus course for vectors or beginning linear algebra course for linear transformations.

In modern day mathematics, we speak of an algebraic structure (ring, field, etc.) as being commutative when \(a \cdot b = b \cdot a\) and distributive when \(a \cdot (b + c) = a \cdot b + a \cdot c\), for all elements \(a\), \(b\), and \(c\) in the structure. The hallmark of Servois' calculus was his examination of the set of all functions and operators that satisfy these properties. Interestingly, the words “commutative” and “distributive” were medieval legal terms [Bradley 2002] and Servois was the first to use them in a modern mathematical sense.

In Sections 5-9 of his “Essai,” Servois examined the “closure” properties of distributive and commutative functions or operators. Servois demonstrated that distributivity is closed under composition and addition, and if \(f\) and \({\rm f}\) are commutative functions or operators, then each commutes with the inverse of the other. An examination of Servois' proof of the latter theorem reveals a law from abstract algebra. By the definition of the inverse function, we have \[f( \mbox{f(f}^{-1}(z))) = \mbox{f(f}^{-1}(f(z))),\] and by virtue of the commutativity of \(f\) and \({\rm f}\), we get \[f( \mbox{f(f}^{-1}(z))) = \mbox{f}(f(\mbox{f}^{-1}(z))).\] Now, substitute \(\mbox{f}(f(\mbox{f}^{-1}(z)))\) for \(f( \mbox{f(f}^{-1}(z)))\) in equation (1), and we get \[\mbox{f}(f(\mbox{f}^{-1}(z))) = \mbox{f(f}^{-1}(f(z))).\] Finally, apply \(\mbox{f}^{-1}\) to both sides of equation (2) and we arrive at the desired result that: \[f(\mbox{f}^{-1}(z)) = \mbox{f}^{-1}(f(z)).\] Essentially, when Servois applied \(\mbox{f}^{-1}\) to both sides of equation (2), he invoked a familiar theorem from group theory, the left-hand cancellation law.

After he considered the properties of commutativity and distributivity separately, Servois examined the collection of functions or operators that satisfy both of these properties. He used this as a launching point to introduce his theory for the differential calculus.

From a modern standpoint, the first twelve sections of Servois' “Essai” constitute the creation of an algebraic structure. In them, he showed that the set of invertible, distributive, and pairwise commutative functions or operators forms a field with respect to the operations of addition and composition. Servois never discussed the associative property with respect to these two operations. However, the importance of associativity was being uncovered during the nineteenth century. For instance, Carl Friedrich Gauss (1777-1855) did prove an associative law in 1801 [Gauss 1801, Section 240] and Hamilton stated the importance of associativity in 1843 after his discovery of the quaternions [Crilly 2006, p. 102]. Hamilton’s statement was actually the first appearance of the term [Miller 2010]. Additionally, Servois assumed the existence of inverses for all of his functions. Again, a reader must keep in mind that Servois worked only with functions that were well-behaved and he did not examine the issue of domain.

Servois' influence on the development of Linear Operator theory can be traced through the works of several well-known mathematicians, including Murphy and Gregory. Although the formalization of symbolic algebra is generally credited to these two mathematicians, we see several ideas associated with Linear Operator Theory in An Essay on Algebraic Development Containing the Principle Expansion in Common Algebra, in the Differential and Integral Calculus, and in the Calculus of Finite Differences; the General Term Being in Each Case Immediately Obtained by Means of a New and Comprehensive Notation by a lesser-known academic, Thomas Jarrett (1805-1882). Jarrett was an English cleric, Professor of Arabic at the University of Cambridge, and a linguist. According to the biography by E. J. Rapson [1892], he knew at least twenty different languages and would translate Chinese characters into Roman characters using a system that he devised himself. There is no biographical information that indicates that he had any formal mathematical training.

In the Preface to his book, Jarrett stated that part of his work was taken from the following mathematicians: Servois, Louis François Antoine Arbogast (1759-1803), John Frederick William Hershel (1792-1871), Carl Friedrich Hindenburg (1741-1808), Sylvestre François Lacroix (1765-1843), Pierre-Simon Laplace (1749-1827), Ferdinand Franz Schwiens (1780-1856), and Josef-Maria Hoëné-Wronski (1776-1853). Interestingly, Wronski's calculus was based on infinitesimals and Jarrett used no such foundations. Jarrett went on to say that some of the material was partly original; however, according to his biographers [Rapson 1892], the original contributions could simply have been new notation. Their contention is supported by Jarrett himself: “In the present Work [Algebraic Notation] is applied to the demonstration of the most important series in pure Analysis” [Jarrett 1831, p. III].

Jarrett's work is similar to Servois' “Essai” in that they both used algebra as a foundation for calculus; however, Jarrett presented Servois' material in a more structured format. Interestingly, when Jarrett discussed the summation operator he distinguished between operators (which he called operations) and functions, but when he presented his theory of the calculus he made no such distinction and classified both as functions. He derived many of the same results as Servois, only using different notation. Jarrett's calculus was based on the concept of the separation of symbols, which he credited to Servois in his Preface: “The demonstration of the legitimacy of the separation of the symbols of operation and quantity, with certain limitation, belongs to Servois ...” [Jarrett 1831, p. III]. At the heart of Jarrett's theory were Servois' distributive and commutative properties:

1. If \(\varphi (u)\) is such a function of \(u\) that \(\varphi (u + v) = \varphi (u) + \varphi (v)\), then \(\varphi (u)\) is called a distributive function of \(u\)
2. If \(\varphi (u)\) and \(\psi (u)\) are such functions of \(u\) that \(\varphi \psi (u) = \psi \varphi (u)\), then the functions \(\varphi (u)\) and \(\psi (u)\) are said to be commutative with each other.

From a modern standpoint, Jarrett defined a field in a fashion similar to Servois’ by introducing the notions of an identity, inverses, and closure, in addition to these two properties. Using properties of this field he derived his theory of the differential and integral calculus.

We also see Servois' ideas in the work of another mathematician, Robert Murphy (1806-1843). Murphy's [1837] “First Memoir on the Theory of Analytic Operations” is a detailed exposition on the theory of operators. Murphy clearly distinguished between functions and operations, and called the objects on which operations are performed subjects [Allaire and Bradley 2002]. With respect to his notation, if Murphy wanted to discuss the operator \(\psi\) applied to the function \(f(x)\), then he denoted it as \([f(x)] \psi\), where the subject is contained within brackets.

Murphy [1837] began his paper by examining special types of operators. He considered the operators \(p\) and \(q\) as fixed or free, where “in the first case a change in the order in which they are to be performed would affect the result, in the second case it would not do so” [Murphy 1837, p. 181]. To relate this to Servois' work, a free operator would be one that satisfied Servois' commutative property. Now, let \(a\) and \(b\) be subjects and \(p\) be the operation of multiplying by the quantity \(p\). Then \[\left[a \pm b\right]p = \left[a\right]p \pm \left[b\right]p,\] which makes \(p\) (or multiplication by \(p\)) a linear operator according to Murphy's definition. Thus, a linear operator is one that satisfies Servois' distributive property. In modern day mathematics, in order for \(p\) to be a linear operator it would also have to be free with respect to a constant \(k\). Additionally, Murphy was the first mathematician to use the term linear to describe a special class of operators [Allaire and Bradley 2002].

Bradley and Allaire [2002] state that Murphy derived many of the same results as Servois, only with greater clarity and brevity. However, Murphy also expanded on the theory of linear operators. For example, he defined the appendage of a linear operator as “the result of its action on zero” [Murphy 1837, p. 188]. Here, “action” refers to the inverse image of the operator. In modern day mathematics, the appendage would be referred to as the kernel of a linear transformation, and this was the first time that the kernel of an operator had been considered [Allaire and Bradley 2002]. The kernel is an important concept in understanding the behavior of linear transformations.

Unlike Jarrett, Murphy did not acknowledge a debt to Servois nor is there solid evidence that he actually read his work. Murphy's opening sections are devoted to the important properties of operators – that is, Servois' commutativity and distributivity – but Murphy gave these properties different names. However, Servois began the study of linear operators and his work was read in England, as demonstrated by Jarrett's use of his research in 1831.  As we will see, Duncan Gregory gave Servois the credit he was due.

According to Piccolino [1984], Gregory played a vital role in the development of symbolic algebra and was a key contributor to the overall advancement of mathematics in England during the late 1830s and early 1840s. In addition to his mathematical demonstrations, Gregory often provided philosophical insights to reinforce his views on algebra. For example, Gregory considered symbolic algebra as “the science which treats of the combinations of operations defined not by their nature, that is, by what they are or what they do, but by the laws of combination to which they are subject” [Gregory 1865, p. 2]. Essentially, Gregory believed that the general principles of algebra must fit a certain structure, which he called a class.

Throughout his Mathematical Writings [1865], Gregory provided several examples illustrating the “laws of combination” to which operations are subject. For example, he considered two classes of operations \(F\) and \(f\), which are connected by the following laws:

1. \(FF(a) = F(a)\)
2. \(ff(a) = F(a)\)
3. \(Ff(a) = f(a)\)
4. \(fF(a) = f(a)\)

His most general interpretation of these laws was multiplication for positive and negative numbers [Allaire and Bradley 2002]. For instance, (2.) shows that a negative number multiplied by a negative number yields a positive number. In this case, \(F\) and \(f\) should not be interpreted as functions, but rather as operations.

Next, he considered a general class of operations, which satisfy the following laws:

1. \(f(a) + f(b) = f(a + b)\)
2. \(f_1 f(a) = f f_1 (a)\)  

Gregory credited Servois with the classification of these laws, writing, “Servois, in a paper which does not seem to have received the attention it deserves, has called them, in respect of the first law of combination, distributive functions, and in respect to the second law, commutative functions” [Gregory 1865, pp. 6-7]. Whereas Murphy [1837] considered the special class of functions satisfying (1.) to be linear operators, Gregory noticed that these two laws together constitute a special class of operations, which are called linear transformations or linear operators in modern mathematics.

Gregory then provided an example to demonstrate his first law, where \(f\) is taken to be the operation of multiplying by a constant \(a\): \[a(x) + a(y) = a(x + y).\] In his Mathematical Writings, Gregory stated that Cauchy sometimes utilized the “laws of combination” [p. 7], so Gregory may have been familiar with the fact that this is the only continuous function that satisfies the distributive law.

Finally, Gregory defined a class of operations by the law \[f(x) + f(y) = f(xy).\] This is the first time we see a general law for a class in which two different operations are considered. Gregory related this definition to a familiar law of logarithms, that \(\ln (x) + \ln (y) = \ln (xy)\), saying “when \(x\) and \(y\) are numbers, the operation is identical with the arithmetical logarithm” [Gregory 1865, p. 11].  

Figure 7. Augustus De Morgan (public domain).

Petrova [1978] stated that linear operator theory began with Servois and was continued by Murphy. According to Allaire and Bradley [2002], Gregory was the next key figure in the development of this theory. We wondered, however, if any other mathematicians were influenced by the work of Servois. The authors examined numerous works written by mathematicians during the Golden Age of mathematics, including Peacock, Augustus De Morgan (1806-1871), and George Boole (1815-1864). These mathematicians made great advances in algebra; however, they appear to have made no significant contributions to the theory of linear operators. After examining several of their works, we now make some observations regarding the influence Servois may have had on these mathematicians. It should be noted that this analysis is highly subjective, because even though some of these mathematicians used methods similar to Servois', none gave him direct credit. Additionally, a majority of these works began to appear in the 1840s, and the works of Murphy and Gregory were already available by this time.

According to O'Connor and Robertson [1996], Peacock was interested in making reforms to Cambridge mathematics and he aided in the creation of the Analytical Society in 1815 as a result. The society was intended to bring the continental methods of the calculus to Cambridge. The reform began when Peacock translated Lacroix's calculus text, Traité élémentaire de calcul differéntiel et du calcul intégral [1802]. Using Lacroix's ideas, Peacock published his Collection of Examples of the Applications of the Differential and Integral Calculus [1820]. Lacroix's work was based on the calculus of Lagrange. Consequently, Peacock adopted many of the methods presented by Lacroix. Peacock did not explicitly use Servois' methods in his work and made no claim about the algebraic properties of operators. However, because Peacock was interested in the continental calculus, it is possible that he was familiar with the works of Servois.  

Now, De Morgan, who was a student of Peacock's, presented the algebraic definitions for distributivity and commutativity in his work, Trigonometry and Double Algebra. These definitions are very similar to the ones that students would learn in a high school algebra course today. For instance, De Morgan stated, “A symbol is said to be distributive over terms or factors when it is the same thing whether we combine that symbol with each of the terms or factors, or whether we make it apply to the compound term or factor” [De Morgan 1849, pp. 102-103]. Being a student of Peacock's, De Morgan could have been familiar with the works of the continental mathematicians. This is further supported by the fact that he used the terms distributive and commutative in a fashion similar to Servois'.

Figure 8.  George Boole (public domain).

Finally, in his Treatise on the Calculus of Finite Differences [1860], Boole gave the laws for the symbols \(\Delta\) and \(\frac{d}{dx}\). For instance, he stated:

1. The symbol \(\Delta\) is distributive in its operation. Thus, \(\Delta \left(u_x + v_x + \&c. \right) = \Delta u_x + \Delta v_x \cdots\).
2. The symbol \(\Delta\) is commutative with respect to any constant coefficients in the terms of the subject to which it is applied. Thus \(a\) being constant, \(\Delta a u_x = a \Delta u_x\).

Interestingly, Boole defined a special case of Servois' “commutative” property, where linear operators commute with constant factors.

Since Boole was a student of Gregory's, it is reasonable to conjecture that he was introduced to the works of Servois via Gregory's teachings. Servois' influence can be seen in Boole's own statements about \(\Delta\) and \(\frac{d}{dx}\) being distributive and commutative operators.

Although Servois was not successful in providing calculus with a proper foundation, his work did have an influence on the field of algebra, where he was a pioneer ahead of his time. He knew that, when performing algebraic manipulations on quantities, he needed to have a structure consisting of a set that obeyed certain axioms. Additionally, his work on analysis spread to England and significantly influenced mathematicians such as Duncan Gregory and Robert Murphy in their efforts to establish the foundations of linear operator theory. Servois' main contribution to this development was recognizing the “distributive” and “commutative” properties of operators, terms that he coined, and the method of separating symbols and their operations. Koppleman [1971] stated that the English mathematicians found the tools for the calculus of operations in the works of French mathematicians, such as Servois'. She went on further to say that “it was the English who developed this work in the calculus of operations both in extending the scope of its applications and in relating it to the theory of abstract algebra” [Koppleman 1971, p. 175].

The material discussed in this paper can aid teachers and students of abstract algebra, linear algebra, and the history of mathematics. By presenting the history of mathematics, instructors can illustrate the idea that mathematics is a constantly evolving field. Besides providing a readable account of the history of algebraic structures and the beginnings of linear operator theory, this paper contains many explanations of and references to original sources. Additionally, this article highlights the fact that while the disciplines of algebra and analysis are studied separately today, mathematicians of the eighteenth century (and before) made little, if any, distinction between them. When analyzing the original sources, the student co-author of this article, Anthony, was initially somewhat shocked to see so many calculus-like and algebraic ideas presented together. As an undergraduate, he had rarely seen ideas from both analysis and algebra meshed so closely together.

In the spirit of Victor Katz, we would encourage instructors to incorporate original sources within their classrooms. This paper provides references to original sources that can easily be found on the internet. With a little consideration on the part of the instructor, it is easy to create historical activities that can fit in any mathematics course. For instance, pages 1-13 of Gregory's The Mathematical Writings contain many examples of symbolical algebra that can be incorporated into any course, such as a first-year calculus course. An instructor could provide a copy of Gregory's discussion of operators that satisfy the property \(f(x) + f(y) = f(xy)\) and ask students to write a list of functions that satisfy this property.

Furthermore, these sources provide students with an opportunity to conduct research on the history of mathematics. Open questions include, for instance:

• Who was Thomas Jarrett? Did he receive any formal mathematical training? If so, from whom did he receive training? Are there any other mathematical works published by him?
• Jarrett's work is taken from a few mathematicians [Jarrett 1831, pp. III-V]. Many are well-known, but who was Ferdinand Franz Schwiens? Other than the analysis textbook mentioned by Jarrett, what did Schwiens write?
• Many unanswered questions still remain regarding Servois' mathematical career. For instance, was there correspondence between Servois and any English mathematicians? If so, it would be interesting to use it to explore the extent of Servois' influence on these mathematicians.

Acknowledgments

The authors are extremely grateful to referees for their many helpful suggestions and corrections.

About the Authors

Anthony J. Del Latto is an undergraduate mathematics major at Adelphi University. He is currently a senior and serves as a tutor for the Department of Mathematics and Computer Science. After completing his bachelor's degree, he wishes to continue his studies in mathematics at the graduate level. His research interests include abstract algebra, history of mathematics, and applied statistics.

Salvatore J. Petrilli, Jr. is an assistant professor at Adelphi University. He has a B.S. in mathematics from Adelphi University and an M.A. in mathematics from Hofstra University. He received an Ed.D. in mathematics education from Teachers College, Columbia University, where his advisor was J. Philip Smith. His research interests include history of mathematics and mathematics education.

[Aebischer and Languereau 2010] Aebischer, A. and Languereau, H. (2010). Servois ou la géométrie á l'école de l'artillerie. Besançon Cedex: Presses universitaires de Franche-Comté.

[Allaire and Bradley 2002] Allaire, P. and Bradley, R. (2002). “Symbolic Algebra as a Foundation for Calculus: D.F. Gregory's Contribution,” Historia Mathematica 29, 395-426.

[Boole 1860] Boole, G. (1860). A Treatise on the Calculus of Finite Differences. London: Cambridge: Macmillian and Co.

[Boyer 1895] Boyer, J. (1895). “Le mathematicien franc-comtois François-Joseph Servois,” Mémoires de la Société d'émulation de Doubs 9, 305-313.

[Boyer 1989] Boyer, C. B. (1989). A History of Mathematics. New York: John Wiley and Sons.

[Bradley 2002] Bradley, R. E. (2002). “The Origins of Linear Operator Theory in the Work of François-Joseph Servois,” Proceedings of Canadian Society for History and Philosophy of Mathematics 14, 1 - 21.

[Bradley and Petrilli 2010] Bradley, R. E. and Petrilli, S. J. (2010). “Servois' 1814 Essay on the Principles of the Differential Calculus, with an English Translation,” Loci: Convergence 7. DOI: 10.4169/loci003487 http://mathdl.maa.org/mathDL/46/?pa=content&sa=viewDocument&nodeId=3597

[Cardano 1545] Cardano, G. (1545). Ars Magna. English translation by R. Witmer (1968): The Rules of Algebra. New York: Dover.

[Cauchy 1821] Cauchy, A.-L. (1821). Cours d'analyse. Paris: de Bure. English translation by R. E. Bradley and C. E. Sandifer (2009): Cauchy's Cours d'analyse: An Annotated Translation. New York: Springer.

[Crilly 2006] Crilly, Tony. (2006). Authur Cayley: Mathematician laureate of the Victorian age. New York: Johns Hopkins Press.

[De Morgan 1849] De Morgan, A. (1849). Trigonometry and Double Algebra. London: Cambridge.

[Dunham 1990] Dunham, W. (1990). Journey Through Genius: The Great Theorem of Mathematics. New York: Penguin Books.

[Epsteen and Maclagan-Wedderburn 1905] Epsteen, S. and Maclagan-Wedderburn, J. H. (1905). “On the Structure of Hypercomplex Number Systems,” Transactions of the American Mathematical Society 6, No. 2, 172-178.

[Français 1814] Français, J. F. (1814). “Sur la théorie des imaginaries," Annales de mathématiques pures et appliquées 4 (1814-1815), 61-71.

[Gauss 1801] Gauss, C. F. (1801). Disquisitiones Arithmeticae. English translation by Clarke et al, New York: Springer, 1986.

[Gregory 1865] Gregory, D. F. (1865). The Mathematical Writings. W. Walton (Ed.). London: Cambridge.     

[Jarrett 1831] Jarrett, T. (1831). An Essay on Algebraic Development Containing the Principle Expansion in Common Algebra, in the Differential and Integral Calculus, and in the Calculus of Finite Differences; the General Term Being in Each Case Immediately Obtained by Means of a New and Comprehensive Notation. London: Cambridge University.

[Katz 2009] Katz, V. (2009). A History of Mathematics: An Introduction. Boston, MA: Addison-Wesley.

[Koppleman 1971] Koppleman, E. (1971). “The Calculus of Operations and the Rise of Abstract Algebra,” Archive for History of Exact Sciences 8, 155-242.

[Lacroix 1802] Lacroix, S. F., 1802. Traité élémentaire de calcul différentiel et de calcul intégral. Duprat, Paris.

[Miller 2010] Miller, J. (2010). Earliest Known Uses of Some of the Words of Mathematics. Retrieved July 14, 2011, from http://jeff560.tripod.com/mathword.html.

[Murphy 1837] Murphy, R. (1837). “First Memoir on the Theory of Analytic Operations,” Philosophical Transactions of the Royal Society of London, 127, 179-210.

[O'Connor and Robertson 1996] O'Connor, J. and Robertson, E. (1996). “George Peacock.” Retrieved August 11, 2011, from MacTutor History of Mathematics Archive: http://www.gap-system.org/ history/Biographies/Peacock.html.

[Peacock 1820] Peacock, G. (1820). Collection of Examples of the Applications of the Differential and Integral Calculus. London: Cambridge.

[Petrilli 2010] Petrilli S. J. (2010). “François-Joseph Servois: Priest, Artillery Officer, and Professor of Mathematics,” Loci: Convergence 7. DOI: 10.4169/loci003498 http://mathdl.maa.org/mathDL/46/?pa=content&sa=viewDocument&nodeId=3498

[Petrova 1978] Petrova, S. S. (1978). “The Origin of Linear Operator Theory in the Works of Servois and Murphy.” History and Methodology of the Natural Sciences 20, 122-128. (Unpublished translation by Valery Krupkin.)

[Piccolino 1984] Piccolino, A. V. (1984). A Study of the Contributions of Early Nineteenth Century British Mathematicians to the Development of Abstract Algebra and Their Influence on Later Algebraists and Modern Secondary Curricula. Doctoral thesis: Columbia University Teachers College.

[Rapson 1892] Rapson, E. J. (1892). "Jarrett, Thomas," in Dictionary of National Biography, L. Stephen and S. Lee, eds. New York: Macmillian and Co., XXIX. 253-254. Also available at http://en.wikisource.org/wiki/Jarrett,_Thomas_(DNB00)

[Servois 1814a] Servois, F. J. (1814). “Essai sur un nouveau mode d'exposition des principes du calcul différentiel," Annales de mathématiques pures et appliqués 5 (1814-1815), 93-140.

[Servois 1814b] Servois, F. J. (1814). “Sur la théorie des imaginaries, Lettre de M. Servois," Annales de mathématiques pures et appliquées 5 (1814-1815), 228-235.

[Smith 1951] Smith, D. E. (1951). History of Mathematics, Volume I. London, UK: Constable and Company, Ltd.

[Smith 1958] Smith, D. E. (1958). History of Mathematics, Volume II. New York: Dover Publications.

[Taton 1972a] Taton, R. (1972). “Français," in Dictionary of Scientific Biography, 1972, C. C. Gillespie, Ed., New York: Scribner, V. 110-112.

[Taton 1972b] Taton, R. (1972). “Servois," in Dictionary of Scientific Biography, 1972, C. C. Gillespie, Ed., New York: Scribner, XII. 325-326.