Overview

Cauchy's limit-sum definition of the Riemann integral for continuous functions is regarded to be central for the understanding of the two standard versions of the Fundamental Theorem of Calculus (FTC). In this article we take exception to this viewpoint and argue that Cauchy's definition is completely extraneous to the mathematical ideas and intuitions responsible for the initial development of integration during the second half of the seventeenth century. We describe modern approaches to teaching elementary integration that do not rely on Cauchy's definition of the integral, and are directly traceable to the mathematics of the second half of the seventeenth century. It is argued that, as one would expect from the historic development of the mathematical ideas related to elementary integration, these teaching approaches have a definite didactic advantage for students of the elementary integral. Finally, it is shown how our approach to integration leads naturally to important mathematical ideas such as Riemann and Darboux integration. 

Introduction

Anyone who has ever taught introductory calculus can attest to the fact that students seldom understand Riemann sums or the fact that the integral of a continuous function is a limit of a sequence of Riemann sums (see note 1.1). In a recent article, David Bressoud [5, p. 99] remarked about the Fundamental Theorem of Calculus (FTC): 

There is a fundamental problem with this statement of this fundamental theorem: few students understand it. The common interpretation is that integration and differentiation are inverse processes. That is fine as far as it goes. The problem is that the definite integral has been defined as a limit of Riemann sums. For most students, the working definition of the definite integral is the difference of the values of “the” antiderivative. When this interpretation of the theorem is combined with the common definition of integration, this theorem ceases to have any meaning.

In this paper we argue that there is a direct cognitive evolutionary line for elementary integration that took form, mostly, in the second half of the seventeenth century, beginning with the work of Cavalieri (1635) and culminating with the work of Barrow (1635), Newton (1664), Gregory (1668), and Leibniz (1684), among others. This line of evolution has an associated teaching approach for integration, which we shall call, following Leonard Gillman [20], the axiomatic approach to the integral. There are various authors whose names have been associated with this teaching approach. Apparently, Stanislaw Saks gave the first theoretical exposition of this approach in 1933 in his monograph Theory of the Integral (published in English in 1937) [37], and Hans Hahn and Arthur Rosenthal developed it further in 1948 in their book Set Functions [24, p. 149; 38]. In 1968, Howard Levi applied the method to the elementary integral [33, p. 65] and Serge Lang gave an exposition of the integral by means of “area functions" (see note 1.2) [28, pp. 208-215]. Finally, in 1993, L. Gillman [20] presented the elementary integral following an axiomatic approach; he also presented a large collection of ingenuous heuristic principles useful in calculating the integrals that are usually found in calculus applications, such as area between curves, arc length, surface area, volumes of revolution, etc. It should be emphatically remarked that the axiomatic approach to the integral needs no mention of Riemann sums. Referring to his approach to the integral, Gillman [20, p. 17] remarked:

As a result, our intuition is relieved of the responsibility of making predictions about infinite processes. (We don't even mention Riemann sums.)

Gillman's statement markedly contrasts with that of Bressoud, and poses, in our view, an interesting problem regarding the lessons in the pedagogy of the elementary integral to be derived from the history of calculus. Of course, it goes without saying that modern analysis is practically unimaginable without Cauchy's limit-sum definition of the integral, and that his definition was instrumental in the eventual development of Darboux (see note 1.3) and Lebesgue integration. In light of the possibility of developing the elementary integral without invoking Riemann sums, the following question of a pedagogical nature gains paramount importance: How can Cauchy's limit-sum definition of the integral be considered as essential for understanding the FTC, especially when it followed the latter result by around a century and a half and, furthermore, played no role in any of the four (independent) proofs of the FTC developed by Barrow, Newton, Leibniz and Gregory during the second half of the seventeenth century?

In this work we argue that the axiomatic approach to the integral offers a definite cognitive advantage for the student of elementary integration, being totally consistent with the historical development of the calculus. Hans Freudenthal [18, 17] and others have made important remarks regarding the role that the history of mathematics can play in its teaching. The history of the evolution of mathematical ideas provides us with significant insights into the inner workings of the human mind as it tries to organize mathematical ideas into coherent bodies of knowledge. In this work we argue that the original insights and intuitions that gave rise to the notion of the integral and the discovery of the FTC are rather removed from Cauchy's conception of the area bounded by a curve as a limiting value of cumulative areas of approximating rectangles. We shall argue that the axiomatic approach to the elementary integral is the “historically correct” approach to teaching integration.

Notes for page 1:

1.1. The reference to “Riemann sums” in this connection is rather obscure and certainly a misnomer from a historical point of view. Bourbaki [3, p. 157] remarked that these sums could have more appropriately been named Archimedes or Eudoxus sums.

1.2. Actually, the phrase “area functions" is a misnomer. They should rather be called “additive functions,” since they can be used for all standard applications of the integral, not just area; for example, volume, surface area, arc length, etc.

1.3. However, as Gillman [20, Section 2, p. 17] appropriately remarked, Darboux integration is closely related to his axiomatic approach to the integral, being (as we shall see) related to the question of the existence of an integral in Gillman's sense.

As remarked by Bressoud [5], the statement of the FTC has two equivalent formulations:

Theorem 1 (FTC, Derivative version).

If \(f:[a,b]\rightarrow\mathbb{R}\) is a continuous function (\({-\infty\,{\rm{<}}\,a\,{\rm{<}}\,b\,{\rm{<}}\,\infty}\)), then \[\frac{d}{dx}\int_{a}^{x}f(u)\,du=f(x)\quad\quad\quad\quad(1)\] for each \(x\in [a,b]\) (if \(x=a\) or \(x=b,\) derivatives are taken to be unilateral).

Theorem 2 (FTC, Antiderivative version).

If \(f:[a,b]\rightarrow\mathbb{R}\) is a continuous function (\({-\infty\,{\rm{<}}\,a\,{\rm{<}}\,b\,{\rm{<}}\,\infty}\)), and \(F:[a,b]\rightarrow\mathbb{R}\) is an antiderivative of \(f\) on \([a,b]\) (that is to say, \(F\) is continuous on \([a,b]\) and \(F^{\,\prime}(x)=f(x)\) for each \(x\in (a,b)\)), then \[\int_{a}^{b}f(u)\,du=F(b)-F(a).\quad\quad\quad\quad(2)\]

The definition of the integral as a limit of Riemann sums is due to Augustin-Louis Cauchy (1789-1857). According to R. Laubenbacher and D. Pengelley [29, p. 139], his superbly elegant definition appeared in 1823, two years after the publication of his Cours d'Analyse. The definition appears in Cauchy's Resumé des Leçons sur le calcul infinitesimal in his Oevres Complètes, published in 1899 [8, Vingt et unième leçon, Intégrales Définies, p. 122]. Cauchy started with a function \(y=f(x)\), continuous “with respect to the variable \(x\) between two finite limits” (his language), and then proved (see note 2.1) that the corresponding Riemann sums converge to a value which he took as the definition of the integral of \(y=f(x)\) on the given interval (see note 2.2).

The first published statement of the FTC was authored by Isaac Barrow [9, Lecture X, Section 11, p. 117] and published around 1674. Isaac Barrow, Isaac Newton, Gottfried Leibniz, and James Gregory are all credited with having proved the FTC independently of each other (see note 2.3). It goes without saying that, in contrast to Cauchy's times, during the second half of the 17th century (the time period in which calculus was invented) there was, at best, an incipient and rudimentary notion of function, with no way to express the continuity of “curves” (roughly corresponding to the graphs of the functions of today) and no standard mathematical notation either for the integral or for the derivative. According to D. T. Whiteside [44, p. 196] the 17th century in mathematics was a period of “rapid advance using valid but tenuously defined concepts as a basis for a rich and varied technical achievement.” One of the greatest of such achievements was that of inventing notations to adequately represent the mental images of the objects of mathematical discourse and their relations [44]. This, in turn, was concomitant with the conceptual mathematical advance that culminated, eventually, in Cauchy's 19th century definition of the integral.

It is worth noticing that during the latter part of the 17th century, motion and geometric transformations were taken to be central ideas in the generation of curves, planar areas, volumes and general surfaces (see, for example, Barrow [9, Lectures I-III, pp. 35-52]). For instance, Bonaventura Cavalieri (1598-1647), a disciple of Galileo Galilei, believed that a surface consisted of an indefinite number of equidistant parallel straight lines, and a solid, in turn, of a set of equidistant parallel planes. These constituted for Cavalieri the linear and planar “indivisibles” [2, p. 124]. In the case of plane figures or solids, a regula was a line or a plane that moved parallel to itself from an initial point until it coincided with another such line or plane. This was the central idea of Cavalieri's theory of indivisibles. Thus, in the case of areas bounded by curves, these were often conceived of as being generated by the corresponding regula viewed as ordinates of variable length and infinitesimal width that moved from an initial position to a final one. This image of a moving ordinate generating an area corresponds to what we call today an “area function.”

For a non-negative continuous function defined on a closed bounded interval, the corresponding area function assigns the value of the area “swept out” by a moving ordinate as its abscissa moves from an initial to a final point in the interval. If \(X_{u}^{v}\) represents the area generated from an initial position of the ordinate with abscissa \(u\) to a final one with abscissa \(v\), then it is clear that \(X_{u}^{v}\) has the following additive property: \[X_{u}^{w}=X_{u}^{v}+X_{v}^{w}\quad{\rm{whenever}}\quad u\,{\rm{<}}\,v\,{\rm{<}}\,w.\] This discussion applies also to other quantities that can be described by one parameter that can take the form of an abscissa, an ordinate, an area, or even the abscissa or the ordinate of a changing point on a curve (e.g. the generating curves of Barrow [9, pp. 35-46, Lectures I and II]).

The property of additivity is closely related to Leibniz' introduction of the \(\int\,\) notation. According to F. Cajori [7, p. 207], in a manuscript written in 1673, Leibniz used Cavalieri's notation to establish the relation: \[ \overline{{\rm{omn.}}\overline{{\rm{omn.}} l}\,\,\frac{l}{a}}=\frac{\,\,\,\,\overline{{\rm{omn.}} l}^{\,\,\,2}}{2a}\, ,\] where \(a\) and \(l\) were, respectively, “infinitesimal elements” of the abscissa and the ordinate associated with a subnormal [7, p. 207], and \({\rm{omn.}}\) (which stood for “omnia” or “all”) was the symbol used by Cavalieri to sum infinitesimal elements such as the regula. Also, the line over \({\rm{omn.}}\) was used in place of our modern parenthesis (see note 2.4). In this manuscript Leibniz remarked: “It will be useful to write \(\int\) for \({\rm{omn.}}\), as \(\int l\) for \({\rm{omn.}} l\), that is, the sum of the \(l\)'s” (see [7, p. 207]). According to F. Cajori [7], since the symbol of summation \(\int\,\) raises dimension, Leibniz concluded that the difference symbol \(d\) would lower it. It is transparent that the operator \({\rm{omn.}}\) (and thus, the operator \(\int\,\)) has the property of additivity as defined above. According to F. Cajori [6, p. 39], the limits of integration were added to the integral sign much later, by Joseph Fourier in 1832. Before then, limits were indicated in words or in symbols within parenthesis or brackets next to the integral sign.

It is noteworthy that all four of the proofs of the FTC mentioned above use, in an essential way, the additive property of area functions. The proofs also use, in various guises, the fact that an ordinate of a curve that moves infinitesimally from a given one, can be taken to be the same as the original ordinate. This statement appears explicitly in Newton's 1669 proof of the FTC (see [23, p. 185]) and also in Section I of L'Hospital's Analyse des infiniment petits pour l'intelligence des lignes curves [34, p. 3, I, Demande ou Supposition], where it was stated as a postulate (see note 2.5), and we shall refer to it as L'Hospital's Postulate:

Grant that two quantities whose difference is an infinitely small quantity may be taken indifferently for each other: or (which is the same thing) that a quantity which is increased or decreased only by an infinitely small quantity may be considered as remaining the same.

If we make seventeenth century curves correspond to today's graphs of functions, Newton's and L'Hospital's statements say, in fact, that all curves are continuous (see page 3 for Newton's proof of the FTC). Not surprisingly, these two properties are all that is needed in order to have the theory of the integral including its usual applications, without ever mentioning a Riemann sum. The theory of the elementary integral as developed by S. Saks [37], H. Hahn and A. Rosenthal [24, 38], and H. Levi [33], and expounded by S. Lang [28, p. 213] and L. Gillman [20] is, in our view, a direct descendant of the ideas and intuitions that nurtured the mathematics of the seventeenth century. Also, it is our contention that the historical development of the integral can be used to make an argument for a teaching advantage in presenting the integral as an “area function” based on modern versions of the additive and continuity properties mentioned above in connection to Newton's proof of the FTC and L'Hospital's Postulate about infinitely close ordinates. We discuss this point in our Concluding Remarks on page 8.

Notes for page 2:

2.1. Whether this is a valid proof or not has been the subject of many discussions. Cauchy's definition of continuity [4, Section 2.2, p. 26] would seem to correspond to our definition of uniform continuity, especially if we take at face value his statement in terms of infinitesimals. In order to show that the corresponding Riemann sums converged to a real number, he used his definition of continuity, which was ready made for the proof. He did not consider (as we do today) continuity at individual points, so that our notion of uniform continuity on intervals was the only notion of continuity that he used.

It is also worth noticing that Cauchy took subdivisions of the given interval, not necessarily uniform; multiplied the lengths of the resulting subintervals by the value of the function at the left endpoint; and added all such products to get his Riemann sums.

2.2. For a commentary on Cauchy's motivation for his definition of the integral using a special case of a Riemann sum, we refer the reader to V. Katz [26, p. 177]. Katz also remarks that Joseph-Louis Lagrange used area functions in proving the FTC in the setting of functions having power series expansions [26, p. 635].

2.3. This independence is questioned in Child [9, p. 201]; see Guicciardini [23, p. 170] for different view.

2.4. With \(a=1\), the modern version of this formula, for a function \(y\) of \(x\), would be: \[\int_{a}^{b}y\bigg(\int_{a}^{x}y\,du\bigg)dx=\frac{1}{2}\bigg[\int_{a}^{b}y\,dx\bigg]^{2}.\quad\quad\quad\quad(3)\] The formula is obtained by a simple application of the method of integration by parts.

2.5. Our wording is taken from Edmund Stone's translation of Analyse des infiniment petits [35, p. 3, Postulate I].

In this section we shall examine one of Newton's proofs (see note 3.1) of the FTC, taken from Guicciardini [23, p. 185] and included in 1669 in Newton's De analysi per aequationes numero terminorum infinitas (On Analysis by Infinite Series). Modernized versions of Newton's proof, using the Mean Value Theorem for Integrals [20, p. 315], can be found in many modern calculus textbooks. The proofs of Leibniz, Barrow, and Gregory have been amply disseminated. For instance, a version of Newton's proof as well as Barrow's and Leibniz' proofs are discussed in Bressoud [5]. Barrow's proof can be found in his Geometric Lectures [9, Lecture X, Section 11, p. 117] and is also discussed in Struik [41, p. 253]. Leibniz's proof appears in Struik [41, p. 282] and also in Laubenbacher and Pengelley [29, p. 133]. Finally, James Gregory's proof is discussed in Leahy [31, 32].

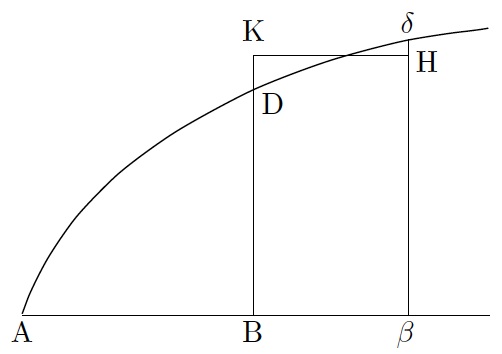
Figure 1. Figure for Newton's Proof of the FTC

Newton considered the curve \(AD\delta\) and set \(AB=x\), \(BD=y\) and \({\rm{area}}\,ABD=z\). He let \(B\beta=o\) be an infinitesimal segment and took \(BK=v\) so that \[vo=\text{area of rectangle}\,B\beta HK={\rm{area}}\,B\beta\delta D\] (see note 3.2). Hence \(A\beta=AB+B\beta=x+o\) and it follows that \[\text{area of region}\,\,A\delta\beta=\text{area of region}\,\,ADB+\text{area of region}\,\,BD\delta\beta\] \[=z+\text{area of rectangle}\,B\beta HK=z+ov.\] If the increment of the area, \(\text{area of region }B\beta\delta D=vo\), is divided by the increment of the abscissa, \(o\), one gets \(v\). Since \(o\) is infinitely small we can assume it to be zero (see note 3.3) so that \(v=y\); that is \(dz/dx=y\).

This argument can be readily expressed in modern mathematical language. If \(\Delta x\not =0\), then \[\frac{\Delta z}{\Delta x}=\frac{1}{\Delta x}\int_{x}^{x+\Delta x}f(u)\,du\] \[=\frac{\Delta x\cdot f(\theta_{x})}{\Delta x}=f(\theta_{x}),\] where \(\theta_{x}\) denotes a real number between \(x\) and \(x+\Delta x\), whose existence is guaranteed by the Mean Value Theorem for Integrals [20, p. 315]. Taking limits and using the continuity of \(y=f(x)\), we have \[\frac{dz}{dx}=\lim_{\Delta x\to 0}\frac{\Delta z}{\Delta x}=\lim_{\Delta x\to 0}f(\theta_{x})=f(x)=y.\] This is Newton's beautiful argument. Notice that besides the use of infinitesimals, he used the additive property of the integral, specifically that \[\text{area of region}\,A\delta\beta=\text{area of region}\,ADB+\text{area of region}\,BD\delta\beta,\] and the interchangeability of ordinates infinitely close to one another.

Barrow's proof [9, Lecture X, Section 11, p.117] is much more geometrical (in keeping with the style of Euclid), but the additivity property is also, as in the case of Newton's proof, crucial to the argument. Similar comments apply to Leibniz's [41, p. 282] and Gregory's [31, p. 9-10; 32, p. 2] proofs. Of Newton, Barrow, Gregory, and Leibniz, the only one who presented an argument for the antiderivative version of the FTC was Leibniz.

Notes for page 3:

3.1. There are several; see Guicciardini [23, p. 183].

3.2. This obviously corresponds to our Mean Value Theorem for Integrals. Newton took an intermediate rectangle whose area lay between the areas of the inscribed and the circumscribed rectangles.

3.3. These are Newton's own words, and they correspond precisely to the statement referred to above; that if two ordinates are infinitely close to one another, either one of them can be taken to be the same as the other. In other words, this is the same as L'Hospital’s Postulate [34, p. 3, I, Demande ou Supposition] about infinitely close ordinates.

As mentioned above, there are ways to teach the elementary integral that capitalize on its properties as an area function and that do not require Cauchy's limit-sum definition of the integral to become the center of the exposition. In fact, as pointed out by Gillman [20, p. 17], strictly speaking, Riemann sums need not be mentioned at all. It is hard to imagine, however, that one would actually want to go as far as hiding Riemann sums completely. Cauchy's limit-sum definition of the integral represented a major landmark in the development of analysis, and thus has secured a position in the curriculum of calculus. Our qualm with Riemann sums stems from the fact that there is mounting evidence in mathematics education research (see our Concluding Remarks, page 8) that the integral as a limit of Riemann sums represents knowledge of a higher order of mathematical abstraction, and thus presents special difficulties to students who are learning calculus for the first time.  In our Introduction, we described the evolution of an approach to teaching elementary integration whose origin can be traced all the way back to the seventeenth century. We now present the main features of this approach, showing how elementary integration with all of its applications might be developed and how it would lead naturally to more advanced aspects of the theory such as Cauchy's limit-sum definition of the integral and Darboux's approach to Riemann integration. Our presentation relies mainly on the work of Lang [28], Gillman and MacDowell [21], and Gillman [20] (see note 4.1).

In the following discussion \(f:[a,b]\rightarrow\mathbb{R}\) is a continuous function (see note 4.2) on \([a,b],\) where \({-\infty\,{\rm{<}}\,a\,{\rm{<}}\,b\,{\rm{<}}\,\infty}\). We begin by incorporating into the definition of an “integral” what we take to be our present day renditions of the principle of additivity and the principle stating that the area increment contains the area of the inscribed rectangle and is contained in the area of the circumscribed rectangle (see Lang [28, p. 213] and Gillman [20, p. 17]):

Definition 1. An integral for \(f\) on \([a,b]\) is a function \([u,v]\mapsto I_{u}^{v}(f)\) that maps subintervals of \([a,b]\) \((a\leq u\,{\rm{<}}\,v\leq b)\) into the reals in such a way that the following conditions are satisfied:

(A) Additivity. If \(a\leq u\,{\rm{<}}\,v\,{\rm{<}}\,w\leq b\), then \[I_{u}^{w}(f) =I_{u}^{v}(f)+I_{v}^{w}(f),\,\,{\rm and}\quad\quad\quad\quad(4)\]

(B) Boundedness. If \(a\leq u\,{\rm{<}}\,v\leq b\), then \[\min_{x\in [u,v]}f(x)\cdot(v-u)\leq \,I_{u}^{v}(f)\leq\max_{x\in[u,v]}f(x)\cdot(v-u).\quad\quad\quad\quad(5)\]

Sometimes, when \([u,v]\mapsto I_{u}^{v}(f)\) is an integral for \(f\) on \([a,b]\) as in Definition 1, we will simply say that \(I(f)\) is an integral for \(f\) on \([a,b]\). Clearly, equation (4) is a modern rendition of the additive property discussed above and used by Newton, Barrow, Gregory and Leibniz in their proofs of the FTC; on the other hand, equation (5) is a very natural condition helpful in making estimates to subsume the infinitesimal arguments of the above-mentioned proofs of the FTC. It says that the area under a curve is bounded below by the area of the inscribed rectangle and above by the area of the circumscribed rectangle. Bounding rectangles were a part of the mathematical psyche of seventeenth century calculus; they are clearly implicit in Newton's proof of the FTC and appear more explicitly in Barrow's proof of the FTC [9, Lecture X, Section 11, p. 117], where he used the estimates contained in (B) for curves that he assumed to be increasing. The other condition that came into play in the development of the integral calculus during the second half of the seventeenth century was that of the interchangeability of infinitely close ordinates mentioned above, which, as we pointed out, corresponds to our notion of continuity.

These two conditions are very natural and both are expected to hold for any integral of a continuous function. Incidentally, in equation (5) the maximum and the minimum values are attained, by virtue of the extreme value theorem for continuous functions [22, p. 164]. When there is no possibility of confusion, we shall often write \(I_{u}^{v}\) for \(I_{u}^{v}(f)\).

Suppose that we define \(I_{x}^{x}=0\) for each \(x\in[a,b]\), and, if \(a\leq u\,{\rm{<}}\,v\leq b\), we define \(I_{v}^{u}=-I_{u}^{v}\), as is conventional in calculus. With these conventions, equation (4) holds for any \(u,v,w\in[a,b]\), regardless of their relative order. Also, if \([u,v]\mapsto I_{u}^{v}(f)\) is an integral as in Definition 1, then if \(a\leq u\,{\rm{<}}\,v\leq b\), we have from (B), \[\min_{x\in  [u,v]}f(x)\leq\frac{1}{v-u}I_{u}^{v}(f)\leq\max_{x\in [u,v]}f(x).\] By the Intermediate Value Theorem [22, p. 164], for some number \(\theta_{u,v}\in[u,v]\), it is true that \[I_{u}^{v}=f(\theta_{u,v})\cdot(v-u).\] This says that our abstract integral satisfies the Mean Value Theorem for Integrals. Gillman [20, p. 18] remarked that properties (A) and (B) “constitute the two steps of the proof of the FTC,” and pointed out some of the advantages of using this definition of the integral rather than the Darboux integral itself.

The argument for the proof of the FTC is direct. If \(I(f)\) is an integral for \(y=f(x)\) on \([a,b]\), \(x\in (a,b)\) (the argument for \(x=a\) or \(x=b\) is similar), and \(\Delta x\not =0\) is chosen in \(\mathbb{R}\) so that \(x,x+\Delta x\in(a,b)\), then, by property (A) of Definition 1 and the Mean Value Theorem for Integrals, we have \[\frac{I_{a}^{x+\Delta x}(f)-I_{a}^{x}(f)}{\Delta x}=\frac{1}{\Delta x}\cdot I_{x}^{x+\Delta x}(f)=f(\theta_{x}),\] for some number \(\theta_{x}\) between \(x\) and \(x+\Delta x\). Since \(\theta_{x}\) is between \(x\) and \(x+\Delta x\), it is clear that \(\lim_{\Delta x\to 0}\theta_{x}=x\), so that, by the continuity of \(f\) and the last relation, we have \[\lim_{\Delta x\to 0}\frac{I_{a}^{x+\Delta x}(f)-I_{a}^{x}(f)}{\Delta x}=\lim_{\Delta x\to 0}f(\theta_{x})=f(x).\] Hence, \[\frac{d}{dx}I_{a}^{x}(f)=f(x).\]

Note that this is actually a modern version of Newton's argument in the case of an abstract integral. Also, once we have the FTC, it is easy to see from the Mean Value Theorem for derivatives [22, p. 167] that the integral is unique.

Interestingly, the linearity property of the integral as a function of its integrand follows from our two postulated properties without the need of Darboux or Riemann sums. In other words, if \(f:[a,b]\rightarrow\mathbb{R}\) and \(g:[a,b]\rightarrow\mathbb{R}\) are continuous functions and \(\alpha,\beta\in\mathbb{R}\), then \[I_{a}^{b}(\alpha f+\beta g)=\alpha I_{a}^{b}(f)+\beta I_{a}^{b}(g).\] In fact, since the functions \(x\mapsto I_{a}^{x}(\alpha f+\beta g)\), \(x\mapsto I_{a}^{x}(f)\), and \(x\mapsto I_{a}^{x}(g)\), where \(x\in[a,b]\), are all integrals, and \[\frac{d}{dx}I_{a}^{x}(\alpha f+\beta g)=[\alpha f+\beta g](x)\] \[=\alpha f(x)+\beta g(x)=\frac{d}{dx}(\alpha I_{a}^{x}(f)+\beta I_{a}^{x}(g)),\] it follows from the usual Mean Value Theorem [22, p. 167] that the functions \(x\mapsto I_{a}^{x}(\alpha f+\beta g)\) and \(x\mapsto\alpha I_{a}^{x}(f)+\beta I_{a}^{x}(g)\), where \(x\in[a,b]\), differ by a constant. Substituting \(x=a\), we see that the constant is zero. Hence, the functions are equal. Substitution of \(x=b\) gives the desired result.

The antiderivative version of the FTC also follows immediately. Remember that a function \(F\) is an antiderivative (or primitive function) of a second function \(f:[a,b]\rightarrow\mathbb{R}\) if and only if \(F\) is continuous on \([a,b]\) and \(F^{\,\prime}(x)=f(x)\) for all \(x\in (a,b)\).

The usual calculus argument gives the antiderivative version of the FTC: Let \(F\) be an antiderivative for \(f:[a,b]\rightarrow\mathbb{R}\) on \([a,b]\). Then \[I_{a}^{b}(f)=F(b)-F(a).\] In fact, by the derivative version of the FTC for our integral, \[\frac{d}{dx}I_{a}^{x}(f)=f(x)\] for all \(x\in [a,b]\). By hypothesis, \(F^{\,\prime}(x)=f(x)\) for all \(x\in (a,b)\) and \(F\) is continuous at \(a\) and \(b\). By the Mean Value Theorem [22, p. 167], the functions \(x\mapsto I_{a}^{x}(f)\) and \(F\) differ by some constant \(C\): \[I_{a}^{x}=F(x)+C\] for all \(x\in [a,b]\). Substituting \(x=a\), we find that \(C=-F(a)\). Hence, \(I_{a}^{x}=F(x)-F(a)\) for all \(x\in [a,b]\). Substituting \(x=b\) we obtain the desired result. 

Thus, the general integral from Definition 1 satisfies both versions of the FTC. In view of all this, if \([u,v]\mapsto I_{u}^{v}\) is the unique integral of \(f\) on \([a,b]\) we write \[I_{a}^{b}(f)=\int_{a}^{b}f(u)\,du.\]

Notes for page 4:

4.1. It is noteworthy that the difference between the editions Gillman and MacDowell [21] and Gillman and MacDowell [22] is several hundred pages of exposition; in Gillman and MacDowell [21], the integral is defined axiomatically while in Gillman and MacDowell [22], the Darboux approach plays a central role in the exposition.

4.2. The fact that only continuous functions are to be considered is a compromise accommodating L'Hospital’s axiom of interchangeability of infinitely close ordinates. This admits at the outset that all curves are continuous.

The usual applications of the integral, viewed as an additive function over the subintervals of \([a,b]\), are easy to obtain. The justification for the additive property can be accomplished through the use of heuristics in the setting of known applications of the integral, such as arc length, area, volume, work, area between two curves, etc. In all these cases the additive property is obvious. The boundedness condition is evident and easily established in many cases, such as area or work. For instance, the work completed by a one dimensional variable force applied on a straight line is bounded below by the work done if we apply the minimum value of the force during the whole trajectory, and bounded above by the work done if we apply the maximum value of the force during the whole trajectory.

In other cases the required bounds are not so clear. Gillman [20, p. 17] stated that the axiomatic presentation of the integral had been done previously with varying degrees of thoroughness, and described among his own contributions to this approach that of providing an improved version of Property (B) of Definition 1, useful in setting up integrals where the desired bounds were not so obvious. In the setting of the present approach to the integral, all applications are obtained by following the same basic pattern. First, one identifies the quantity to be represented as an integral \(I_{a}^{b}\) and verifies that properties (A) and (B) are satisfied. This is accomplished using knowledge from the field of application or, sometimes, on heuristic grounds. As Gillman himself remarked, “From then on, the rest is mathematics” [20, p. 20]. The desired quantity is the unique integral satisfying conditions (A) and (B).

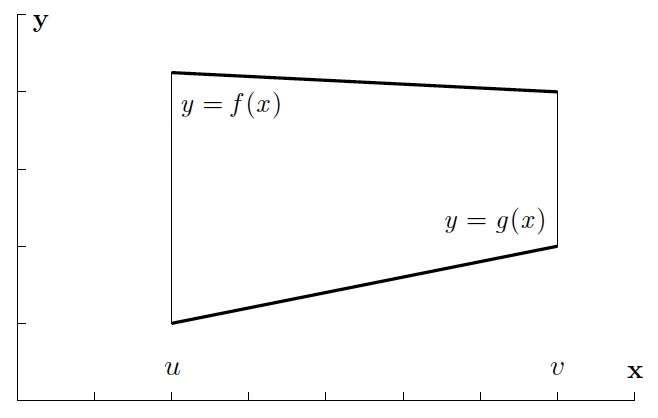
Figure 2. Estimation of Bounds I

In order to show Gillman's improvement on condition (B) of Definition 1 we look at an example where the basic pattern does not work. For instance, in Figure 2, we have two functions \(y=f(x)\) and \(y=g(x)\) defined over a portion of an interval \([a,b]\) such that \(f(x)\geq g(x)\geq 0\) over that interval. In determining the volume of the solid of revolution \(V_{u}^{v}\) around the \(x\) axis of the area bounded by both curves lying between \(x=u\) and \(x=v\), it is easy to obtain the following bounds: \[\pi\cdot\big[\min_{w\in [u,v]}f^{2}(w)-\max_{w\in [u,v]}g^{2}(w)\big](v-u)\leq V_{u}^{v}\quad\quad\quad\quad\quad\quad\notag\] \[\leq\pi\cdot\big[\max_{w\in [u,v]}f^{2}(w)-\min_{w\in[u,v]}g^{2}(w)\big](v-u).\quad\quad\quad(6)\] These bounds are not too useful to begin with, and in some cases, like the one depicted in Figure 3, expressions like \[\big[\min_{x\in [u,v]}f^{2}(x)-\max_{x\in [u,v]}g^{2}(x)\big]\] are negative.

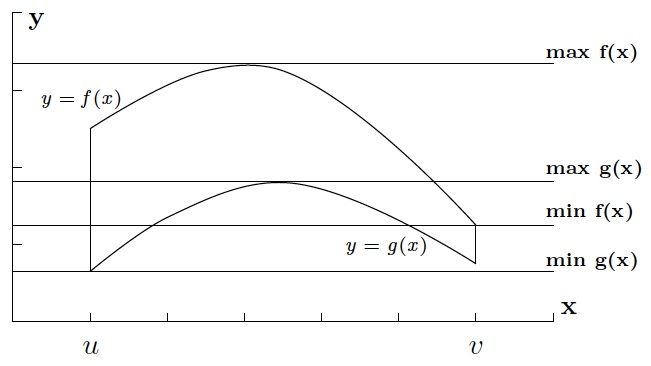
Figure 3. Estimation of Bounds II

To circumvent this difficulty Gillman [20, p. 20] invented an ingenious limit bounding condition:

(B') Gillman's limit bounding condition. Let \(f:[a,b]\rightarrow\mathbb{R}\) be continuous and suppose \([u,v]\mapsto J_{u}^{v}(f)\) (\(a\leq u\,{\rm{<}}\,v\leq b\)) is a function such that for each \(x\in [a,b]\) and \(u\leq x\leq v\) (\(u\,{\rm{<}}\,v\)) there are real parameters \(\phi_{u,v}\) and \(\varphi_{u,v}\) depending on \(x\) such that \[\phi_{u,v} \cdot (v-u)\leq \,J_{u}^{v}(f)\leq\varphi_{u,v}\cdot (v-u),\] and \[\lim_{u,v\to x}\phi_{u,v}=\lim_{u,v\to x}\varphi_{u,v}=f(x).\] If \(x=a\) or \(x=b\), we interpret the condition accordingly so as to have one-sided limits.

We can easily see that the following theorem is true.

Theorem 3. If \(f\) has an integral on \([a,b]\) and \([u,v]\mapsto J_{u}^{v}(f)\) is a function defined on the subintervals of \([a,b]\) satisfying condition (A) of Definition 1 and Gillman's limit bounding condition (B'), then \(J(f)\) is the (unique) integral for \(f\) on \([a,b]\).

Outline of the proof. By part (A) of Definition 1 and by condition (B'), it is easy  to see that \[\frac{d}{dx}J_{a}^{x}=f(x).\] If \(x\mapsto I_{a}^{x}(f)\) is an integral for \(f\) on \([a,b]\), then it must differ by a constant from \(x\mapsto J_{a}^{x}(f)\). Since \(J_{a}^{a}(f)=I_{a}^{a}(f)=0\), then \([u,v]\mapsto I_{u}^{v}(f)\) and \([u,v]\mapsto J_{u}^{v}(f)\) must be the same integrals, which establishes the theorem.

Going back to our discussion of relation (6), we see that \[\lim_{u,v\to x}\big(\min_{w\in [u,v]}f^{2}(w)-\max_{w\in [u,v]}g^{2}(w)\big)=f^{2}(x)-g^{2}(x),\] and also that \[\lim_{u,v\to x}\big(\max_{w\in [u,v]}f^{2}(w)-\min_{w\in [u,v]}g^{2}(w)\big)=f^{2}(x)-g^{2}(x).\] It follows that \[V_{a}^{b}=\pi\int_{a}^{b}[f^{2}(x)-g^{2}(x)]\, dx\] gives the volume of revolution around the \(x\) axis. This is the well known “washer method” for the calculation of volumes of revolution. When the area depicted in Figure 2 is rotated about the \(y\) axis, we get the estimates \[\pi(u+v)\big(\min_{w\in [u,v]}f(w)-\max_{w\in [u,v]}g(w)\big)(v-u)\leq V_{u}^{v}\] \[\leq\pi(u+v)\big(\max_{w\in [u,v]}f(w)-\min_{w\in [u,v]}g(w)\big) (v-u).\] Using (B') again, we get \[V_{a}^{b}=2\pi\int_{a}^{b}x[f(x)-g(x)]\,dx.\] This is the expression for the so-called “shell method” for calculating volumes of revolution.

Gillman [20] presented other examples of heuristic arguments for circumventing the difficulties of applying the estimate (B) of Definition 1.

One of the most attractive features of the didactic approach to integration presented here is that it leads naturally to the discussion of more abstract analysis, specifically to Cauchy's limit-sum definition of the integral and to Darboux sums. In fact, early in his exposition, Gillman [20, p. 17] established the relationship between his axiomatic approach to the integral and the Darboux integral. His reasoning leads also to the Cauchy limit-sum definition of the integral. Of course, both these topics are, cognitively speaking, of a higher order of mathematical abstraction, and thus harder for students to understand. One advantage of our presentation, which differs a little from the presentation found in Lang [28] and in Gillman and McDowell [21], is that the question of the existence of an integral comes up as a natural consideration, thus leading to more advanced mathematics.

Of course, the question of the existence of an integral would have been completely anachronistic in the setting of the mathematics of the seventeenth century, when the fact that all curves had areas associated with them was something that was evident and not to be questioned. This “escalation” of the level of abstraction is consistent with the fact that, historically, this discussion belongs to the 18th century, a time in the history of mathematics when such existence questions were natural (and expected). The question of which functions (not necessarily continuous) can have integrals with properties analogous to the ones we have just discussed seems impossible to formulate within the mathematical mind frame of the 17th century.

Is there an integral for a continuous \(f\) on \([a,b]\)? If so, how can we find it? In this section we point out the connection between the axiomatic formulation of the integral presented here and the integral as a limit of Riemann sums. For the discussion we shall assume the existence of at least one such integral (as in Definition 1) in order to show that it must be a limit of Riemann sums. The deliberations that ensue from pursuing the question of the existence of an integral for a continuous function on a closed bounded interval are, as expected, of a higher level of mathematical abstraction. For this reason, this discussion is also harder for the student to sort out. We present it here to show that it fits nicely into our teaching proposal.

The next result opens the door to explore Darboux integration and introduces topics and issues characteristic of mathematical analysis. The point at hand is, of course, the existence of an integral for \(f\) on \([a,b]\). The result also introduces Riemann sums.

Theorem 4. Let \([u,v]\mapsto I_{u}^{v}(f)\) \((a\leq u\leq v\leq b)\) be an integral for a continuous function \(f:[a,b]\rightarrow\mathbb{R}\) on \([a,b]\). Then \[I_{a}^{b}(f)=\lim_{n\to\infty}\sum_{k=1}^{n}f(x_{k-1})\Delta x_{k},\quad\quad\quad\quad(7)\] where for each \(n\geq 1\), \(\{ a=x_0\,{\rm{<}}\,x_1\,{\rm{<}}\,\cdots\,{\rm{<}}\,x_n = b\}\) is the uniform subdivision of \([a,b]\) into \(n\) subintervals, with \[x_{k}={a+\frac{k(b-a)}{n}}\,\,{\rm{for}}\,\,{\rm{each}}\,\,k=0,1,\dots ,n\] and \[\Delta x_{k}=x_{k}-x_{k-1}=\frac{b-a}{n}\,\,{\rm{for}}\,\,{\rm{each}}\,\,k=1,2,\dots , n.\] In fact, if for the uniform subdivision \(\{ a=x_0\,{\rm{<}}\,x_1 < \cdots\,{\rm{<}}\,x_n = b\}\) of \([a,b]\) into \(n\) subintervals, we choose any \(z_{k-1}\in [x_{k-1},x_{k}]\) for each \(k=1,\dots ,n\), then \[I_{a}^{b}(f)=\lim_{n\to\infty}\sum_{k=1}^{n}f(z_{k-1})\Delta x_{k},\] also holds.

Note that this result does not settle the issue of the existence of an integral for a continuous function on a closed bounded interval, but it gives us a glimpse into the beginnings of Darboux integration.

Proof. 

We shall assume in this proof that there is an integral for \(f\) on \([a,b]\). Let \(F\) be an antiderivative of \(f\) on \([a,b]\). Let \(\{a=x_0\,{\rm{<}}\,x_1\,{\rm{<}}\,\cdots\,{\rm{<}}\,x_n=b\}\) be the uniform partition of \(n\) subintervals \((n\geq 1)\). Then \[F(b)-F(a)=\sum_{k=1}^{n}[F(x_{k})-F(x_{k-1})].\] By the Mean Value Theorem [22, p. 167], it is possible to choose \(x_{k}^{*}\in [x_{k-1},x_{k}]\) such that \[F(b)-F(a)=\sum_{k=1}^{n}[F(x_{k})-F(x_{k-1})]\] \[=\sum_{k=1}^{n}F^{\,\prime}(x_{k}^{*})\Delta x_{k}=\sum_{k=1}^{n}f(x_{k}^{*})\Delta x_{k}.\] As every continuous function on a closed bounded interval is uniformly continuous, given \(\epsilon\,{\rm{>}}\,0\), there is a \(\delta\,{\rm{<}}\,0\) so that \(|f(u)-f(v)|\,{\rm{<}}\,\epsilon/(b-a)\) whenever \(u,v\in [a,b]\) and \(|u-v|\,{\rm{<}}\,\delta\). Choose an integer \(n_{0}\geq 1\) such that \((b a)/n_{0}\,{\rm{<}}\,\delta\). Then, for any \(n\geq n_{0}\), \[\big|F(b)-F(a)-\sum_{k=1}^{n}f(x_{k-1})\Delta x_{k}\big|\] \[=\bigg|\sum_{k=1}^{n}f(x_{k}^{*})\Delta x_{k}-\sum_{k=1}^{n}f(x_{k-1})\Delta x_{k}\bigg|\] \[\leq\sum_{k=1}^{n}|f(x_{k}^{*})-f(x_{k-1})|\Delta x_{k}\] \[\,{\rm{<}}\,\frac{\epsilon}{b-a}\cdot\sum_{k=1}^{n}\Delta x _{k}\] \[=\frac{\epsilon}{b-a}\cdot (b-a)=\epsilon.\] As \(\epsilon\) is arbitrary, \[F(b)-F(a)=\lim_{n\to\infty}\sum_{k=1}^{n}f(x_{k-1})\Delta x_{k}.\] The desired result follows. It is clear that any other value \(f(z_{k-1})\) would work the same as \(f(x_{k-1})\) if we chose \(z_{k-1}\in [x_{k-1},x_{k}]\) \((k=1,\dots ,n\)), and this completes our proof.

Note that given any subdivision \(\{a=x_0\,{\rm{<}}\,x_1\,{\rm{<}}\,\cdots\,{\rm{<}}\,x_n =b\}\) (\(n\geq 1\)) of \([a,b]\), uniform or not, we have, by virtue of condition (B) of Definition 1, \[\sum_{k=1}^{n}\min_{u\in [x_{k-1},x_{k}]}f(u)\cdot\Delta x_{k}\leq I_{a}^{b}(f)\leq\sum_{k=1}^{n}\max_{u\in [x_{k-1},x_{k}]}f(u)\cdot\Delta x_{k}.\quad\quad\quad\quad(8)\] In the case of a continuous function \(y=f(x)\), the sums in inequalities (8) are in fact Riemann sums, and the inequalities are equivalent to  \[\sum_{k=1}^{n}\inf_{u\in [x_{k-1},x_{k}]}f(u)\cdot\Delta x_{k}\leq I_{a}^{b}(f)\leq\sum_{k=1}^{n}\sup_{u\in [x_{k-1},x_{k}]}f(u)\cdot\Delta x_{k}.\quad\quad\quad\quad(9)\] Of course, inequalities (9) make sense for bounded functions \(y=f(x)\), and this is clearly the starting point of Darboux integration.

The axiomatic approach to the integral provides some clear teaching advantages when compared to the usual presentation using the Cauchy limit-sum definition of the integral. The latter presentation requires an understanding of a complicated type of limit. Whether the limit is to be understood in the sense of convergence of nets with respect to the directed order defined by refinement (inverse set inclusion), or as a limit when the “norm” of the partitions goes to zero (see note 7.1), the limit, given its complicated nature, presents obvious and sometimes insurmountable difficulties for students.  We list some of the advantages to teaching the elementary integral using Gillman's axiomatic approach:

• The properties of the integral are easily justified and readily obtained. Furthermore, both versions of the FTC are central ingredients of the presentation.
• The use of L. Gillman’s [20] heuristics and Gillman's limit bounding condition (B’) makes the setting up of integrals (usually justified through Riemann sums) an easy and attainable matter. The condition of additivity is obvious for all applications of the integral.
• The theoretical importance of Darboux integration becomes clearer in the axiomatic approach. In this approach one supposes that there is an integral for each continuous function on a given interval of the real line. In the second half of the seventeenth century, mathematicians did not contemplate the need for a justification of such a statement. But as mathematics progressed, this supposition eventually required a theoretical justification. Eventually, the Darboux approach to integration as well as Cauchy's limit-sum property led to the modern theories of integration we know today.
• If we are to believe that the history of mathematics somehow reflects “cognitively correct ways” to approach the teaching of mathematics, then the axiomatic presentation of the elementary integral is in total harmony with the historic evolution of the notion of integration.

Note for page 7:

7.1. Remember that the choice of points at which the function is evaluated must be taken into account in taking the limit. The important result that such a limit is independent of the choice of points is an idea that needs time to mature and assimilate.

It is generally believed that the history of mathematics is relevant to its teaching and learning in so far as it shows some of the workings of the human mind as it grapples in its quest to organize collections of problems and other mathematical data into coherent bodies of knowledge. While this statement is remarkable for what it says, it is perhaps more so for what it stops short of saying. Surely, history does suggest specific ways to approach the teaching of particular areas of mathematics. But in far too many instances, it is clear that we cannot limit mathematics teaching to the ideas nurtured in a particular epoch of its development, without sacrificing important ideas in the evolution of mathematical knowledge. A case in point is our reservations concerning the pedagogical wisdom of basing the theory of the elementary integral on Cauchy's idea of the limit of Riemann sums. The historical evidence available shows (and in no ambivalent terms) that during the second half of the seventeenth century, when the elementary integral was actually invented, there was no notion resembling limits of Riemann sums lurking in the air. In fact, the notion of “area" was based on the concepts of indivisibles and infinitesimals. As vague and controversial as such conceptions might have turned out to be in the perspective of history, a close analysis of the mathematical ideas of the second half of the seventeenth century convincingly shows that, apart from the notions just mentioned, the core idea of the elementary integral is based on the leitmotif of areas being generated by moving abscissas on curves (area functions). It is truly amazing the extent to which the properties of the integral are dependent on just this simple idea. An added virtue of our teaching proposal is that it leads naturally to more sophisticated ideas like those contained in Cauchy's limit-sum definition of the integral.

An ever increasing amount of evidence in the mathematics education literature documents what every mathematics professor knows: the majority of students, even high performance students, have serious difficulties with the epsilon-delta definition of limit (and also with the so-called sequential definition of the limit) [42, p. 4; 39]. In [11] evidence is presented that documents specific challenges students have with limits, and in particular with the limit involved in Cauchy's definition of the integral. Similar problems are described and data presented in [1, p. 196], [10, p. 153], and [43, p. 11]. Other interesting articles related specifically to the teaching of calculus include [12], [13], [30], [36], [40], and [45]. Mathematics teaching seems to be full of examples of the fact that mixing mathematical “notations” and “meanings” from different mathematical eras can cause difficulties for the student who is trying to understand the corresponding mathematics. This last statement should not necessarily be construed as an argument to avoid the mixing of ideas from different historical times for the sake of pedagogy, but rather as a warning that, in doing so, troubles may ensue. The case at hand, related to the use of Riemann sums in elementary integration, is one such example.

Another example is the teaching of the chain rule; see [25] and [19]. What is the chain rule? The standard answer: it is an algorithm to find the derivative of the composition of two differentiable functions. It is curious that in L'Hospital's Analyse des infiniment petits [34] or in any of Euler's famous calculus books [14, 15, 16], there is no “proof” or justifying argument for the chain rule. Of course, in L'Hospital’s time there was no notion of function, nor any idea that functions could be composed (see note 8.1). It is interesting that the notion of composition is what seems to be the main source of difficulty for student understanding of the chain rule (see note 8.2). In L'Hospital's and Euler's calculus, the use of infinitesimals turns the chain rule into a virtually trivial statement having to do with substitutions rather than compositions.

Indeed, after Abraham Robinson's vindication of Leibniz, we can successfully write most of Euler's beautiful arguments in correct mathematical language, thus giving back to calculus its dynamic character. Calculus was originally developed as a study of change and motion (see note 8.3) in a numerical setting that included infinitesimals and infinite numbers. With the construction of the standard real number system, the theory of limits was invented, and this presupposed a change of emphasis biased towards logic and quantification, and the handling of inequalities. Whether this is adequate language for the pedagogy of the calculus is an interesting topic that, unfortunately, has not received sufficient attention in the literature. Surely there must be a compromise between the extremes of teaching mathematics using the ideas of previous times as if nothing important happened afterwards, and doing so by using the cold implicit esthetics that shifts around theorems, corollaries, and definitions in order to maximize logical coherence and minimize redundancy, and without regard to the historical development of ideas. Whatever the solution to this intriguing dilemma, mathematics departments must begin to think about such issues if they are to improve on the teaching of the calculus, an area that leaves much to be desired.

Notes for page 8:

8.1. The first reference to the chain rule as a rule for differentiation of a composition of two functions occurred in [27, Section 31, p. 29] almost a century after the chain rule was first used; it was also recorded in [8, p. 25].

8.2. This information was gathered in several non-standard calculus courses given at the University of Puerto Rico by one of the authors.

8.3. This was certainly true from Barrow all the way to Leibniz (including Newton and Gregory). For instance, Barrow's Lectures II and III were devoted to the generation of quantities by “local motions” [9, p. 42]. Leibniz, in his proof of the FTC [41, p. 282], said, “Then we shall show how this line can be described by a motion that I have invented.”

Acknowledgments 

The authors are especially grateful to Robert L. Knighten, Adjunct Professor of Mathematics at Portland State University, for his help in clearing up some of the finer points related to the historical development of integration theory during the second half of the 17th century. We also want to thank the referees of this article for their careful reading of the manuscript and their useful suggestions, thanks to which this work has improved significantly.

About the Authors

Jorge López Fernández is Professor of Mathematics at the University of Puerto Rico in Río Piedras, San Juan, Puerto Rico, where his mathematical interests are harmonic analysis and mathematics education.

Omar Hernández Rodríguez is Assistant Professor in the Graduate School of Education at the University of Puerto Rico.  His interests include cognitive and meta-cognitive processes in mathematical problem-solving; incorporating new technologies into the teaching of mathematics; professional development of teachers; and theory, design, development, and evaluation of mathematics curriculum.

[1] M. Artigue, “Analysis.” In D. Tall (Ed.), Advanced Mathematical Thinking, Kluwer Academic Publishers, Dordrecht, 1992. pp. 167-198.

[2] M. E. Baron, The Origins of Infinitesimal Calculus, Dover Publications, New York, 1969.

[3] N. Bourbaki, Elements of Mathematics, Functions of a Real Variable, Elementary Theory, Springer, Heidelberg, 2003.

[4] R. E. Bradley and C. Sandifer, Cauchy’s Cours d’Analyse, An Annotated Translation, Springer Verlag, Doordrecht, 2009.

[5] D. M. Bressoud, “Historical reflections on teaching the fundamental theorem of integral calculus,” American Mathematical Monthly 118 (2011), pp. 99–115.

[6] F. Cajori, “The history of notations of the calculus,” Annals of Mathematics, Second Series 25 (1923), pp. 1–46.

[7] F. Cajori, A History of Mathematics, The Macmillan Company, New York, 1931.

[8] A. L. Cauchy, Résumé des Leçons Données a L’Ecole Royale Polytechnique, Oevres Complétes, vol. IV of 11, Gauthier-Villard, Paris, 1899.

[9] J. M. T. Child, The Geometrical Lectures of Isaac Barrow, Translated with notes and proofs, and a discussion on the advance made therein on the work of his predecessors in the infinitesimal calculus., no. 3 in Series of Classics of Science and Philosophy, The Open Court Publishing Company, Chicago, 1916.

[10] B. Cornu, “Limits.” In D. Tall (Ed.), The transition to advanced mathematical thinking: Functions, limits, infinity and proofs, Kluwer Academic Publishers, Dordrecht, 1992. pp. 153-166.

[11] J. Cottrill, D. Nichols, K. Schwingendorf, K. Thomas, and D. Vidakovic, “Understanding the limit concept: Beginning with a coordinated process schema,” Journal of Mathematical Behavior 15 (1996), pp. 167–192.

[12] R. B. Davis and S. Vinner, “The notion of limit: Some seemingly unavoidable misconception stages,” Journal of Mathematical Behavior (1986), pp. 281–303.

[13] G. Ervynck, “Conceptual difficulties for first year university students in acquisition for the notion of limit of a function.” In L. P. Mendoza and E. R. Williams (Eds.), Canadian Mathematics Education Study Group: Proceedings of the Annual Meeting, Kingston, Ontario: Memorial University of Newfoundland, 1988. pp. 330-333.

[14] L. Euler, Introductio in analysin infinitorum, vol. 1, Real Sociedad Matemática Española, Sevilla, edición facsimilar, “thales” ed., 1748.

[15] L. Euler, Introductio in analysin infinitorum, Vol. 2; Introduction to the Analysis of the Infinite, Book II (translation of vol. 2), Springer Verlag, New York, 1748.

[16] L. Euler, Institutiones calculi differentialis, 1755; Foundations of Differential Calculus (translation), Springer Verlag, New York, 2000.

[17] H. Freudenthal, Mathematics as an Educational Task, D. Reidel Publishing Co., Doordrecht, 1973.

[18] H. Freudenthal, Didactical Phenomenology of Mathematical Structures, D. Reidel Publishing Co., Doordrecht, 1999.

[19] M. N. Fried, “Some reflections on Hernández and López’s reflections on the chain rule,” The Montana Mathematics Enthusiast 7 (2010), pp. 333–338.

[20] L. Gillman, “An axiomatic approach to the integral,” American Mathematical Monthly 100 (1993), pp. 16–25. Available at http://mathdl.maa.org/mathDL/22/?pa=content&sa=viewDocument&nodeId=2913

[21] L. Gillman and R. H. MacDowell, Calculus, W. W. Norton, New York, 1973.

[22] L. Gillman and R. H. MacDowell, Calculus, W. W. Norton, New York, second ed., 1978.

[23] N. Guicciardini, Isaac Newton on Mathematical Certainty and Method, The MIT Press, Cambridge, Massachusetts, London, 2009.

[24] H. Hahn and A. Rosenthal, Set Functions, The University of New Mexico Press, 1948.

[25] O. Hernández Rodríguez and J. López Fernández, “A semiotic reflexion on the didactics of the chain rule,” The Montana Mathematics Enthusiast 7 (2010), pp. 321–332.

[26] V. J. Katz, A History of Mathematics, An Introduction, Addison Wesley, Pearson, New York, third edition, 2009.

[27] J. L. Lagrange, Theorie des fonctions analytiques, L’Imprimerie de la republique, Paris, 1797.

[28] S. Lang, A First Course in Calculus, Addison Wesley, third printing (1974), 1968.

[29] R. Laubenbacher and D. Pengelley, Mathematical Expeditions, Chronicles by the Explorers, Undergraduate Texts in Mathematics, Springer Verlag, 1999.

[30] L. Le and D. Tall, “Constructing different concept images of sequences and limits by programming,” Proceedings of the Seventeenth Conference for the Psychology of Mathematics Education, Tsukuba, Japan (1993), pp. 41–48.

[31] A. Leahy, “An Introduction to James Gregory’s Geometriae Pars Universalis,” Proceedings of the Eighth Midwest History of Mathematics Conference (2000).

[32] A. Leahy, “A Euclidean Approach to the FTC,” Loci: Convergence (2004). DOI: 10.4169/loci002156 http://mathdl.maa.org/mathDL/46/?pa=content&sa=viewDocument&nodeId=2156

[33] H. Levi, Polynomials, Power Series and Calculus, Van Nostrand Company, Inc., Princeton, 1968.

[34] G. F. A. L’Hospital, Analyse des infiniment petits pour l’ intelligence des lignes courbes, L’Imprimerie Royale, Paris, 1696.

[35] G. F. A. L’Hospital, The method of fluxions both direct and inverse (translated by Edmund Stone), printed for William Innys, London, 1730.

[36] J. Mamona-Downs, “Pupils’ interpretations of limit concept: A comparison between Greeks and English,” Proceedings of the Fourteenth Conference for the Psychology of Mathematics Education, Mexico City, Mexico (1990), pp. 69–76.

[37] J. J. O'Connor and E. F. Robertson, "Stanislaw Saks," MacTutor History of Mathematics Archive, 2000, http://www.gap-system.org/~history/Biographies/Saks.html

[38] J. J. O'Connor and E. F. Robertson, "Hans Hahn," MacTutor History of Mathematics Archive, 2006, http://www.gap-system.org/~history/Biographies/Hahn.html

[39] A. Robert, “L’acquisition de la notion de convergence des suites numeriques dans l’enseignement superieur,” Reserches en Didactique des Mathematiques 3 (1982), pp. 307–341.

[40] A. Sierpinska, “Humanities students and epistemological obstacles related to limits,” Educational Studies in Mathematics (1987), pp. 371–397.

[41] D. J. Struik, A Source Book in Mathematics, 1200-1800, Dover Publications Inc., New York, 1969.

[42] D. O. Tall, “The transition to advanced mathematical thinking: Functions, limits, infinity and proof.” In D. A. Grows (Ed.), The Handbook of research on mathematics teaching and learning, Macmillan, New York, 1992, pp. 495-511.

[43] D. O. Tall and S. Vinner, “Concept image and concept definition in mathematics, with particular reference to limits and continuity,” Educational Studies in Mathematics (1981), pp. 151–169.

[44] D. T. Whiteside, “Patterns of mathematical thought in the latter seventeenth century,” Archive for History of Exact Sciences 1 (1961), pp. 179–388.

[45] S. R. Williams, “Model of limit held by college calculus students,” Journal for Research in Mathematics Education (1991), pp. 219–236.