Kepler and the Rhombic Dodecahedron: The Cuboctahedron and Trapezo-rhombic Dodecahedron

 

Following our experiment with clay balls, we are in a position to talk about two other beautiful polyhedra.

Kepler was very interested in polyhedra in general, and in Archimedean solids in particular. In his Harmonices Mundi [The Harmony of the World, 1619], he systematically studied the Archimedean solids and made several new discoveries in that field.

Archimedean solids are named after the great Greek mathematician and physicist Archimedes, who Pappus (in Book V of his Mathematical Collection) reported was the first to give a description of them. But anything Archimedes himself wrote on this topic was lost long before Kepler’s time. This means that Kepler’s Harmonices Mundi is the oldest surviving detailed mathematical account of the Archimedean solids that is known today. It is interesting to note that Kepler referred to the Archimedean solids as “perfect in a lesser (or inferior) degree."^[4] In contrast, he considered the Platonic solids (such as the cube) as belonging to the class of what he called the “most perfect“ solids due to their symmetry and regularity: every face of a Platonic solid is the same regular polygon and all of its angles are equal.^[5] An Archimedean solid is not quite as symmetric or regular as a Platonic solid, but nearly so: the faces of an Archimedean solid always include two or more different types of regular polygons and its angles are not equal but do follow a uniform pattern.^[6]

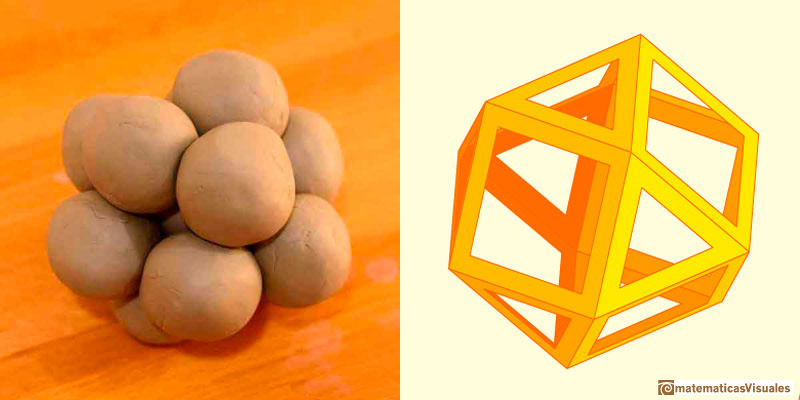
One of the 13 Archimedean solids identified by Kepler in his Harmonices Mundi is called the cuboctahedron. This beautiful polyhedron was known since prehistoric times and used in jewelry and decoration. It has a close relation with the rhombic dodecahedron and our experiment with balls.

If we put twelve balls surrounding a center ball as before, we can see that these twelve balls are in a regular disposition. They can be thought of as lying in the vertices of a cuboctahedron, as shown in Figure 18.

Figure 18. Spheres and cuboctahedron. The cuboctahedron is an Archimedean solid. Notice that any group of four adjacent faces that is formed by two squares interspersed with two triangles (such as the group we see in the top view) forms a regular hexagon.

The cuboctahedron has 8 equilateral triangles and 6 squares as its 14 faces. In comparison, recall that the rhombic dodecahedron has 14 vertices. This is not a coincidence! The twelve vertices of the cuboctahedron also correspond with the twelve faces of the rhombic dodecahedron. This kind of relationship, where the faces of one polyhedron can be matched to the vertices of another polyhedron, is called duality. The image in Figure 19 helps us see the correspondence that makes the cuboctahedron the dual of the rhombic dodecahedron (and vice versa).

Among other interesting properties, the cuboctahedron has 24 equal edges that are distributed in four “hexagonal sections,” each of which consists of three regular hexagons of the kind described in the caption of Figure 18. Do you remember the four belts of six rhombi of the rhombic dodecahedron? Each of those four belts corresponds with one of the four hexagonal sections of the cuboctahedron.

Figure 19. A cuboctahedron inside a rhombic dodecahedron, illustrating their dual relationship. Notice how each vertex of the rhombic dodecahedron corresponds to a face of the cuboctahedron and vice versa. Each of the rhombic dodecahedron’s four belts also corresponds to one of the cuboctahedron’s hexagonal sections. Cardboard and plastic. Vertices were produced using 3D printing.

To learn more about this fascinating polyhedron, building a physical model is again highly recommended. As before, one can do this by using a net such as the one provided (free for non-commercial use) on Gijs Korthals Altes’s splendid website Paper Models of Polyhedra. Another nice approach is to build a model using two different colors, one for the squares and another for the triangles, using a technique suggested by Magnus Wenninger in his classic book Polyhedron Models [1971]. Downloadable templates for printing the planar figures needed to build a two-color model of the cuboctahedron and other polyhedra are available on the author's matematicasVisuales website. Yet another interesting model of the cuboctahedron can be built using templates that first appeared in a Life Magazine article from March 1, 1943, about Buckminster Fuller’s Dymaxion World, also known as the Fuller Map Projection. Templates for constructing a model of Fuller's Dymaxion World can be downloaded from the blog site An Ocean Of Knowledge An Inch Deep.

               
Figure 20. Two models of the cuboctahedron. (Left) A two-color model using the author's templates (matematicasVisuales). (Right) Buckminster Fuller's Dymaxion World, constructed by Cory Poole (An Ocean Of Knowledge An Inch Deep).

We can also get a cuboctahedron by cutting the vertices of a cube or an octahedron. This process is called ‘truncation’. The link given in the investigation “Properties of the cuboctahedron” below illustrates how this works.

 

 

 

 

 

Applying Kepler’s compression idea with a slight twist gives us yet another polyhedron to admire. If we play with the stack of clay balls from our earlier experiment, we will easily realize that there is a second way to put twelve of those balls around the thirteenth; namely, with the bottom layer aligned exactly below the top layer, as illustrated in the right-hand image of Figure 21.

Figure 21. Notice the difference in alignment of the top layer and the bottom layer.

By squeezing this second structure, we will get a different polyhedron that is called a trapezo-rhombic dodecahedron since half of its twelve faces are rhombi and the other half are trapezoids. You can explore some of the properties of the trapezo-rhombic dodecahedron and a related polyhedron called the “twisted cuboctahedron” in the investigation "The trapezo-rhombic dodecahedron" below.

Figure 22. Trapezo-rhombic dodecahedron. Cardboard and magnets.