- About MAA
- Membership
- MAA Publications
- Periodicals
- Blogs
- MAA Book Series
- MAA Press (an imprint of the AMS)
- MAA Notes
- MAA Reviews
- Mathematical Communication
- Information for Libraries
- Author Resources
- Advertise with MAA
- Meetings
- Competitions
- Programs
- Communities
- MAA Sections
- SIGMAA
- MAA Connect
- Students
- MAA Awards
- Awards Booklets
- Writing Awards
- Teaching Awards
- Service Awards
- Research Awards
- Lecture Awards
- Putnam Competition Individual and Team Winners
- D. E. Shaw Group AMC 8 Awards & Certificates
- Maryam Mirzakhani AMC 10 A Awards & Certificates
- Two Sigma AMC 10 B Awards & Certificates
- Jane Street AMC 12 A Awards & Certificates
- Akamai AMC 12 B Awards & Certificates
- High School Teachers
- News
You are here
Led Astray by a Right Triangle: Misconception, Epiphany, and Redemption - My Misconception
My Misconception
Thus, more firmly impressed with the societal importance of perpendicularity as determined by a simple right triangle, I attempted to unravel the information contained in the ninth chapter, the Gougu chapter, of the Jiuzhang. My efforts in this task are best explained by taking the reader through my analysis and solution of some actual examples of problems from the text. First, one has to appreciate the form and style of ancient Chinese mathematics problems: a problem is stated, the answer given, and a brief, very concise explanation offered. The explanation assumes that its reader is familiar with the computing practices and conventions implied. Chinese mathematicians of this period would do all calculations with a set of computing rods. Calculations were performed rapidly and mechanically. As was the custom, later commentators could append their own explanations to problems. For example, the seventeenth problem of the sequence of problems given in the ninth chapter [Jiuzhang 9:17] in translation is stated as follows:
A square walled city measures 200 bu on each side. Gates are located at the center of all sides. If there is a tree 15 bu from the eastern gate, how far must a person travel out of the south gate to see the tree?
Answer: 666 2/3 bu
Method: The answer will be the quotient found by using the 15 bu as a denominator and half the width of the city squared as the numerator.
Approaching the problem situation as would a competent secondary school student, I first drew a diagram depicting the situation. Then, in a modern manner, I labeled all parts of the diagram and, as conditioned, designated the required side ED as the unknown “x”. See Figure 4.
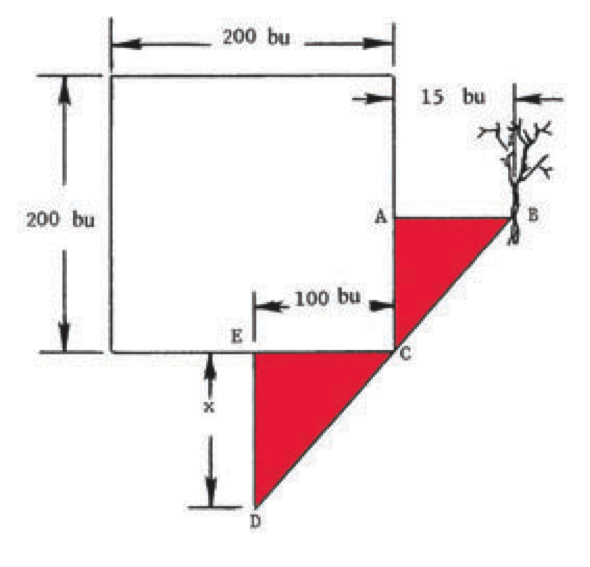
Figure 4. Diagram for Jiuzhang 9:17, assuming a solution by similar triangles
Immediately I recognized the similarity of the two relevant triangles, ABC and ECD, and this determined my solution strategy. Employing the proportionality relationship between the corresponding sides of the similar triangles, ED/EC=AC/AB, and substituting in the given information, I arrived at:
\[\frac{x}{100} = \frac{100}{15}\rightarrow x=\frac{100^2}{15}\rightarrow x=666\frac{2}{3}.\]
I obtained the correct answer and my procedure satisfied the given Jiuzhang method.
Another, more complex problem, the twenty third [Jiuzhang 9:23], is:
A hill lies west of a tree whose height is 95 chi. The distance between the tree and the hill is known to be 53 li. A man 7 chi tall stands 3 li east of the tree. If the tops of the hill and tree are aligned in the path of his vision, what is the height of the hill?
Answer: 1649 chi, 6 33/50 cun
Method: Obtain the height of the tree minus the height of the man. Multiply this difference by 53 chi and divide by 3; the result plus 95 chi gives the desired answer.
[Note: In the metrological system of this period, distance was measured as: li (mile) = 300 bu, bu (pace) = 5 chi, and chi (foot) = 10 cun.]
Once again, I drew a diagram; see Figure 5. This time, allowing for some artistic license, I embellished the diagram with a drawing of a tree and hill. Labeling all known entities as given, I allowed the height of the hill to be EF, where EF = (ED + CG).

Figure 5. Diagram for Jiuzhang 9:23, assuming a solution by similar triangles
Again, upon inspection, two similar triangles, ABC and CDE, provided a proportional relationship:
ED/DC = CB/BA → ED = (DC)(CB)/BA.
Substituting in the given values, where CB = (CG – AH), I arrived at:
ED = (53)(95–7)/3 = 1554.666 and EF = 1554.666 + 95 = 1649.666 or 1649 chi, 33/50 cun.
Once again the desired answer followed the indicated traditional method. And so it went for all the problems in Jiuzhang, Chapter 9.
From this experience, I surmised the ancient Chinese mathematicians recognized the similarity of triangles, appreciated their proportionality of respective sides, and on this basis set up appropriate proportions to obtain their desired results. Over time, the use of these proportions resulted in their discovery of the more general “rule of three” – that is, given four quantities, a, b, c and d that are in the proportional relationship a/b = c/d, then if three of the quantities are known, the remaining quantity may be found by the proportion. They then applied “the rule of three” to a wider selection of problems. See Figure 6.

Figure 6. Similar triangles ABC and ADE yield BC/CA = DE/EA.
In Figure 6, above, similar triangles yield BC/CA = DE/EA. Therefore, if BC = a, CA = b, DE = c, and EA = d, then a/b = c/d.
This scenario – that such a numerical relationship as the “rule of three” would first be appreciated visually and physically in a geometric situation before being conceptually assimilated – appealed to my sense of logic and historical perspective. Early Chinese mathematicians were known to draw diagrams on paper, cut them up, move the regions around, experiment with them, and seek out new geometric-algebraic relationships as realized in the Xian thu demonstration. The simple but powerful algebraic technique, “the rule of three,” also known over time by many other names, such as “the Golden Rule” or “the Merchant’s Rule,” eventually became a valued computational tool used in all societies. The rule’s origin has historically been attributed to Asian sources [9]; now, to me, it appeared to be definitely Chinese. I thought that I had made a discovery: the Chinese through their recognition of the similarity of triangles and the employment of its properties had developed the “rule of three.” I had found a mathematical link in the chain of cause and effect!
Frank J. Swetz (The Pennsylvania State University), "Led Astray by a Right Triangle: Misconception, Epiphany, and Redemption - My Misconception," Convergence (December 2014)




