Mathematical Treasure: Johannes Scheubel's 1551 Algebra

Dedicated to Dr. Barnabas Hughes: Professor, Mentor, Friend

Above: Title page of Scheubel’s Algebrae Compendiosa from the collection of Dr. Sid Kolpas. The full translation of the title from the Latin is Concise Algebra easily described, which brings forth the great wonders of arithmetic. The printer’s colophon (logo) is “The fat hen” (Pingui Gallina). The book was printed in Paris in 1551. “Cum Privilegio” means with privilege or permission to print from the king, in this case King Henry II of France.

Table of Contents

• A Brief Biography
• Algebrae Compendiosa
• Selected Examples for the Classroom
• Using Algebrae Compendiosa in the Classroom
• Who Was the Printer Cavellat?
• References

A Brief Biography

Johannes Scheubel was born on August 13, 1494, in Kirchheim unter Teck, Germany, and died on February 20, 1570, in Tübingen, Germany, where he is buried. According to his biographer Mary Day (1926), nothing is known about his family since the church register in Kirchheim unter Teck only went back to 1558. Scheubel attended the Latin School of Kirchheim unter Teck, continuing his studies at the University of Liberal Arts in Vienna. He began teaching in 1532 in Leipzig, and then was appointed Professor at the University of Tübingen, where he became “Magister” in 1540. According to Day, he became “Docent of Mathematics” in 1544, teaching arithmetic and geometry. At his death, his mathematical instruments, manuscripts, and library were left to the University of Tübingen. Scheubel’s publications include:

• De Numeris et Diversis Rationibus seu regulis computationum opusculum a Joannes Scheubelo compositum, Lipsiae (Leipzig), 1545. This was an arithmetic book of five chapters.
• Compendium Arithmeticae Artis per Johannem Scheubeliu adornatum et conscriptum, Basel, 1549.
• Euclidis Megarensis, Philosophi et Mathematici excellentissimi, sex libri priores, de Geometricis principiis, Graeci et Latini, una cum demonstrationibus propositionum. Algebrae porro regulae, propter numerorum … his libris praemissae sunt …, Basel, 1550. This is Scheubel’s Euclid, and is considered his masterpiece. It includes the first publication of Scheubel’s Algebrae Compendiosa as its first 76 pages. The author owns a copy of this work.
• Algebrae compendiosa facili’sque descriptio, qua depromuntur magna Arithmetices miracula, Paris, 1551. First published as a preface to Scheubel’s Euclid. The author owns a copy of this work.

According to Barnabas Hughes in his article, “The Private Library of Johann Scheubel, Sixteenth-Century Mathematician,” Scheubel was “an intellectually heavy scholar, balanced and poised, a man of dignity.” His library included works on algebra, architecture, arithmetic, the astrolabe, astrology, astronomy, biology, the compass, cosmography, geometry, Greek and Latin literature, medicine, music, natural philosophy, optics, tables, trigonometry, and weights. In particular, he owned a copy of Copernicus’s De revolutionibus. He kept abreast of publications in his area of specialty, geometry and arithmetic, which included the works of Cardano, Stifel, Peter Ramus, Christoph Rudolph, and Peter Apian; he was current in the mathematical thinking of the time.

Scheubel was considered by David Eugene Smith (1860-1944) to be an under-rated figure. In his Rara Arithmetica, Smith stated (pp. 235-236):

While Scheubel is not much appreciated to-day, he was really ahead of his time. He tried to banish the expression 'rule of three' and to substitute 'rule of proportion.' His explanation of square root is in some respects the best of the century, and he dismisses with mere mention the 'duplatio' and 'meditatio' of his contemporaries. He extracts various roots as far as the 24th, finding the binomial coefficients by means of the Pascal triangle a century before Pascal made the device famous.

Algebrae Compendiosa

Scheubel’s Algebrae Compendiosa is an algebra that does not fully use modern notation. According to Florian Cajori (1859-1930), the book contains the first appearance of the + and – symbols in France (1928, p. 151). N means x⁰ or 1, Ra., short for Radix, means x¹ or x; Pri., short for Primus, means x²; Secun., short for Secundus, means x³; etc. (refer to the table below); that is, xⁿ is indicated by an abbreviation of the Latin name for n-1, the number of multiplications starting with x to obtain xⁿ. Thus, 8N means 8x⁰ or 8 (N indicating a constant number), 8 Ra. means 8x, 8 Pri. means 8x², etc.

The table of powers above appears in Algebrae Compendiosa at page (or folio) 6 recto (or 6r).

According to Cajori, mathematical encyclopaedist and textbook author Charles Hutton (1737-1823) felt that Algebrae Compendiosa was “most beautifully printed, and is a very clear though succinct treatise; and both in the form and matter much resembles a modern printed book” (quoted in Cajori 1928, p. 151). The 2010 advertisement of Martayan Lan Rare Books of an original copy of the book, offered at $2,250, indicated that the book was of “pocket format,” a specialty of the printer, Gulielmo Cavellat. Cavellet favored the octavo size because it made his books easier to carry and less expensive for university students. (For more information, see Who Was the Printer Cavellat?)

The pages of Algebrae Compendiosa are numbered only on the front side, which was common at the time. In the following images from the book, “r” will denote recto or the front of the page and “v” will denote verso or the back of the page.

Above: Scheubel defined his addition symbol and subtraction symbol on page 1r of his Algebrae Compendiosa. As noted above, this was the first appearance of the + and – symbols in France (Cajori 1928, p. 151).

Below: Scheubel’s discussion of powers continued on page 1 verso, or 1v.

On pages 3-9 of Algebrae Compendiosa, Scheubel discussed operations on integers, done exactly as they are taught today. For example, Scheubel stated that when multiplying, if the signs are the same the product is positive, and if the signs are different the product is negative. He then used these rules for operations on integers in the examples in the book.

Algebrae Compendiosa contains many interesting algebra problems and word problems requiring algebraic solutions. There follow some selected examples that can be used in the classroom.

Selected Examples for the Classroom

Example: Subtracting binomials

From Algebrae Compendiosa (page 4v)

We would write (8x⁴ + 7x) – (5x⁴ – 4x) = 3x⁴ + 11x. Notice Scheubel’s facility with negatives: 7x – (–4x) = 11x.

Example: Multiplying binomials

From Algebrae Compendiosa (page 7r)

The goal here is to compute (8x² – 9)(8x² – 9), which is done by computing (8x² – 9)8x² = 64x⁴ – 72x² and (8x² – 9)9 = 72x² – 81 separately, followed by "subtraction of the products": (64x⁴ – 72x²) – (72x² – 81) = 64x⁴ – 144x² + 81.

Example: Dividing powers

From Algebrae Compendiosa (page 11r)

We might write: \(\frac{2}{3x}\div\frac{8x}{9x^2}=\frac{3}{4}\) or \(\frac{8x}{9x^2}\div\frac{2}{3x}=1\frac{1}{3}.\)

Examples: Solving quadratic equations

Above: Here on page 20r of Algebrae Compendiosa, Scheubel solved the equation 1x² + 12 = 8x by completing the square. We (but not Scheubel) would first rewrite the equation as 1x² – 8x = –12. Scheubel’s instructions should then sound familiar:

• Square 8/2 = 4 to get 16, then add 16 to –12 to get 4. (Literally, 16 minus 12 makes 4.)
• Now subtract \({\sqrt 4}=2\) from 4 and add 2 to 4 (that is, compute 4 ± 2) to get the roots 2 and 6 of the equation. Here, “radix quadrata” means “square root.”

Scheubel then checked his solutions 2 and 6 by substituting them into his equation, 1x² + 12 = 8x, and making sure in each case that the two sides were equal to the same number (not shown).

A few pages later, on page 24v of Algebrae Compendiosa, Scheubel solved the quartic (degree 4) equation

9x⁴ +5x² = 294

by completing the square. He first rewrote the equation as

1x⁴ + 5/9 x² = 294/9,

indicating only the new coefficients 5/9 and 249/9. From here, his method was to square one half of 5/9, or 5/18, to get 25/324, then add 25/324 to 294/9 to get 10609/324. Now \( {\sqrt{\frac{10609}{324}}}={\frac{103}{18}} \) and \[{\frac{103}{18}}-\frac{5}{18}=\frac{98}{18}=\frac{49}{9},\] so that \(x^2=\frac{49}{9}\) and \(x=\frac{7}{3}=2\frac{1}{3}.\) Note that negative square roots were twice ignored.

The equals sign

As can be seen above, Scheubel did not use our equals sign, instead using the Latin word “aequales,” meaning “equals.” Robert Recorde (1510-1558) would introduce the equals sign just six years later in his Whetstone of Witte (1557).

Barnabas Hughes, in his article, “Robert Recorde and the first published equation” (1993), concurred with Mary Day that Algebrae Compendiosa was Recorde’s main source for his Whetstone of Witte. Day, in her 1926 biography of Scheubel, stated that “Recorde must have had Scheubel’s Algebra before him when he wrote his book.” She showed that many of Recorde’s examples and word problems are similar to Scheubel’s.

Title page of Robert Recorde’s Whetstone of Witte (1557) (Courtesy of Columbia University Libraries)

First appearance of the equals sign ====== in Recorde’s Whetstone of Witte (Courtesy of Columbia University Libraries)

Modern translation of third sentence in the paragraph just above the equations: And to avoid the tedious repetition of these words: "is equal to": I will set (as I do often in work use) a pair of parallels, or Gemowe lines, of one length (thus: =), because no two things can be more equal.

See Mathematical Treasure: Robert Recorde's Whetstone of Witte for more images of and information about this text.

Examples: Square roots

Scheubel used “ra.” preceding a number to indicate radix or square root; thus, “ra. 7” indicates the square root of 7. Sometimes Scheubel used the modern square root symbol, as in \({\sqrt 7}.\) Moreover, he occasionally used “radix binomii” for the square root of a binomial. For nested square roots, he used “ra.col.,” or radix collecti, for the square root of a collection of terms, as in \[{\rm{Ra.col.}}\,32 + {\sqrt{1020}} = {\sqrt{32+\sqrt{1020}}}.\]

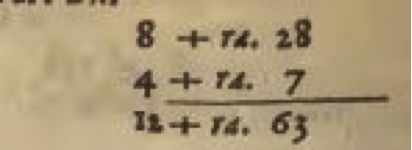
From Algebrae Compendiosa (page 33r)

Above: Note that the desired form of \[\left(8+\sqrt{28}\right) + \left(4+\sqrt{7}\right)\] was not \(12+3\sqrt{7},\) but rather \(12+\sqrt{63}.\)

Below: The product \[\left({23+\sqrt{448}}\right) \left({4-\sqrt{7}}\right)\] was simplified “only” to \[92-\sqrt{3136}-\sqrt{3703}+\sqrt{7168}.\]

From Algebrae Compendiosa (page 35r)

Examples: Higher roots

From Algebra Compendiosa (page 30r)

“Ra. cu.” indicates cube root. The problem above, then, is to compute the sum, \({\sqrt[3]{24}}+{\sqrt[3]{81}},\) simplifying the result to the single cube root, \({\sqrt[3]{375}}.\)

Scheubel’s method, in modern notation, is to rewrite \({\sqrt[3]{24}}+{\sqrt[3]{81}},\) as \[{\sqrt[3]{3}}\left({\sqrt[3]{8}}+{\sqrt[3]{27}}\right).\] The expression in parentheses simplifies to \(2+3\) or \(5,\) which is then cubed to get \(125.\) That is, \[{\sqrt[3]{3}}\left({\sqrt[3]{8}}+{\sqrt[3]{27}}\right) = {\sqrt[3]{3}}\left({2+3}\right) = {\sqrt[3]{3}}\left({5}\right)={\sqrt[3]{3}}\left({\sqrt[3]{125}}\right)={\sqrt[3]{375}}.\]

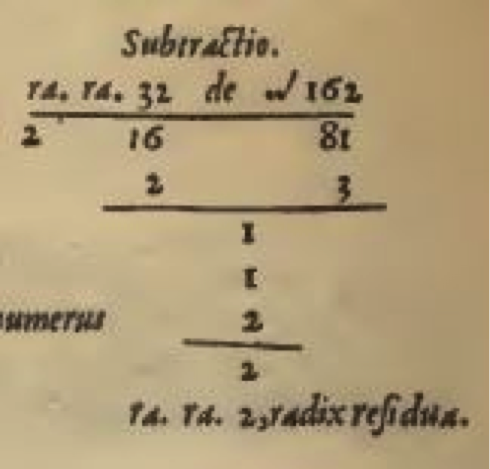From Algebrae Compendiosa: Both examples appear on page 32r.

In the examples above, “ra. ra.” indicates fourth root (square root of square root), also indicated by the modified radical sign that appears just before 162 in both examples above. Scheubel used both notations, as can be seen in the examples. In the example at left, Scheubel used the same method he used to add cubic roots in the preceding example to rewrite the sum \({\sqrt[4]{32}}+{\sqrt[4]{162}}\) as the single fourth root, \({\sqrt[4]{1250}}.\) In the example at right, he used the same method to subtract  \({\sqrt[4]{32}}\) from \({\sqrt[4]{162}}\) – that is, to compute \({\sqrt[4]{162}}-{\sqrt[4]{32}}\) – and to simplify the difference to the single fourth root, \({\sqrt[4]{2}}.\)

From Algebrae Compendiosa (page 31r)

The claim here is that \[{\sqrt[4]{\frac{36}{5}}}\cdot {\sqrt[4]{\frac{4}{5}}} = {\sqrt{\frac{12}{5}}}.\]

Examples: Continuous ratios

From Algebrae Compendiosa (page 14r)

Divide 40 into three numbers so that the parts are in a continuous ratio of 1 2/3 to 1.
Answer: 7 17/49, 12 12/49, 20 20/49.

Work: The first is 1 ra. or 1x, the second 1 2/3 x, and the third 2 7/9 x. Their sum, 5 4/9 ra., therefore equals 40 N. We would write 5 4/9 x = 40 or 49/9 x = 40, giving the first as x = 360/49 = 7 17/49, the second as 1 2/3 x = 600/49 = 12 12/49, and the third as 2 7/9 x = 1000/49 = 20 20/49.

From Algebrae Compendiosa (page 16v): King, Princes, Counts, and Soldiers

There is a king, and under him princes, counts, and soldiers.  The ratio of counts to princes is twice the number of princes, and the ratio of soldiers to counts is four times the number of princes.  One two-hundredth of the number of soldiers is equal to nine times the number of princes.  Find the number of princes, counts, and soldiers.
Answer: 15 princes, 450 counts, 27000 soldiers.

Work: By the first hypothesis, let the number of princes \(= x,\) counts \(= 2x^2,\) and soldiers \(= 8x^3.\) By the second hypothesis, \(\frac{1}{200}\cdot8x^3=9x,\) or, as in the last line shown above, \(\frac{1}{25}x^3=9x.\) Solving for \(x\) \(\left(x>0\right)\) gives \(x = 15.\)
So, the number of princes \(= x = 15,\)  counts \(= 2x^2 = 450,\) and soldiers \(= 8x^3 = 27000.\)

Example: An investment problem

From Algebrae Compendiosa (page 18r)

Three tradesmen invest 170 gold coins (aureos) in a partnership. The first wants his money returned in 3 months, the second in 6 months, and the third in 8 months. In a certain amount of time, this money bears interest amounting to 375 gold coins (aurei). The first man receives for his part, including his share and interest, 75 coins; the second 200 coins; and the third the remaining coins. How much was each man’s share, or how much money was collected from each? The result: 60 aurei from the first, 80 from the second, and 30 from the third, as announced at the top of the next page.

From Algebrae Compendiosa (page 18v)

Scheubel’s equation was 1 ra. + 1 1/3 ra. + ½ ra. = 170, or 2 5/6 ra. = 170, yielding 1 ra. = 60 and therefore 1 1/3 ra. = 80 and ½ ra. = 30 aurei as the initial investments. How did he get this equation from the given data? Perhaps he obtained it through proportional reasoning like the following:

The first person got 75 aurei for 3 months, so for 6 months the second person would have received 150 at the same rate. But he received 200, so he must have invested \(\frac{200}{150}\) or \(\frac{4}{3}\) as much as the first person. The third person invested for 8 months, and at the same rate as the first person would have received \(\frac{8}{3}(75)=200.\) But he received only 100, so he must have invested one-half as much as the first person. If the first person invested 1 ra., then the second and third invested, respectively, 4/3 ra. and ½ ra. Since the three investments total 170 aurei, Scheubel’s equation was

1 ra. + 1 1/3 ra. + ½ ra. = 170.

Example: A military problem

From Algebrae Compendiosa (page 15r)

A general has several thousand soldiers under his command. When he attempts to arrange his army in the largest possible square, there are 284 too many soldiers. If he then tries to arrange them in a square with one man more on a side than before, he lacks 25 soldiers. How many soldiers does he have?

Answer: 24 thousand soldiers.

From Algebrae Compendiosa (page 15v)

At the top of the next page, Scheubel wrote the total number of soldiers in two different ways:

• the number in a square of side length 1 ra., or \(x,\) plus 284 left over, or 1 pri. + 284 N, or \(x^2 + 284,\) and
• the number in a square of side length 1 ra. + 1 N, or \(x+1,\) minus the 25 the general lacked to make this square; that is, \((x+1)^2 – 25.\) But Scheubel wrote this as 1 pri. + 2 ra. + 1 N – 25 N, or \(x^2+2x+1-25.\) He then simplified to 1 pri. + 2 ra. – 24 N, or \(x^2+2x-24.\)

Scheubel obtained his answer by setting the two expressions equal to one another to form the equation

1 pri. + 284 N aequales 1 pri. + 2 ra. – 24 N,

or \[x^2+284=x^2+2x-24,\] where each side of the equation gives the total number of soldiers. He simplified this equation to \(308=2x,\) and then to \(x=154.\) Remembering that the total number of soldiers is given by \(x^2+284\) and also by \(x^2+2x-24,\) he could then compute the number of soldiers using one expression and then check his answer using the other expression, obtaining his answer of 24000 soldiers.

Robert Recorde’s Whetstone of Witte has the exact same problem, adding evidence that he had Algebrae Compendiosa in front of him when he wrote his book.

From Whetstone of Witte (pages 249-250)

Using Algebrae Compendiosa in the Classroom

I have used sample problems from Scheubel’s Algebra in Elementary, Intermediate, and College Algebra classes to show students how the problems they’re studying were written and solved in 1551. Students find the problems fun to work, they gain an appreciation for our modern algebraic notation, and they learn some mathematics history.

Another use in the classroom would be in a History of Mathematics course as part of a larger discussion about the evolution of mathematical notation, and/or to give students the experience of solving problems as they were written and solved in the sixteenth century. In a History of Mathematics or History of Science class, the instructor might also discuss what was happening in the world in 1551 to provide historical context to the discussion of Scheubel’s Algebra. 

A complete copy of Scheubel’s Euclid, which contains Algebrae Compendiosa, can be found on the e-rara website at http://www.e-rara.ch/bau_1/content/titleinfo/1688789. 

Algebrae Compendiosa is also available as a print-on-demand book for as low as $10.20 from ReInk (or Reink) Books. See http://www.abebooks.com/servlet/SearchResults?sts=t&tn=algebrae+compendiosa. 

A PDF copy of Algebrae Compendiosa can be downloaded from: https://archive.org/details/bub_gb_yyJhlkJwjcQC

Instructors can use the examples in this article or select their own examples from electronic copies or the print-on-demand copy.

Who Was the Printer Cavellat?

According to Joëlle Ducos of the University of Paris–Sorbonne, Guillaume Cavellat was born between 1520 and 1525, and died in 1576. His print shop/book store, established in 1547, could be found at the "sign of the fat hen” (also his colophon) near Cambrai College, University of Paris. Cavellat associated with astronomers and mathematicians, convincing them to use the books he printed. His book production and sales depended largely on the university programs. He chose an octavo format because it was easy for students to carry and was relatively inexpensive. When Cavellat died, his widow Denise Girault, who was the daughter of a bookseller, carried on the business. Back to Algebrae Compendiosa

References

Cajori, Florian. A History of Mathematical Notations. The Open Court Publishing Company, La Salle, Illinois, 1928. See especially pages 147-151.

Day, Mary S. Scheubel As An Algebraist. Teachers College, Columbia University, NYC, 1926.

Ducos, Joëlle. “Editer des livres scientifiques au XVIe siècle: Guillaume Cavellat” (“Editor of scientific books in the sixteenth century: Guillaume Cavellat”). Uranie: http://uranie.msha.fr/expositions/editer-des-livres-scientifiques-au-xvie-siecle-guillaume-cavellat/

Hughes, Barnabas B. “Johann Scheubel’s Revision of Jordanus de Nemore’s De numeris datis: An Analysis of an Unpublished Manuscript.” Isis, Vol. 63, No. 217, Balding and Mansell, London, June 1972.

Hughes, Barnabas B. “The Private Library of Johann Scheubel, Sixteenth-Century Mathematician.” Viator: Medieval and Renaissance Studies. University of California Press, Berkeley, Vol. 3, 1972.

Hughes, Barnabas B. “Robert Recorde and the first published equation.” Vestigia Mathematica. Amsterdam, 1993, pp. 163-171.

Martayan Lan Rare Books: http://www.martayanlan.com/cgi-bin/searchresults.cgi?item=371&start=30&map_or_book_id=1&cat=29&catalog=all&ke

Scheubel, Johann. Algebra Compendiosa. Gulielmum Caullet, Paris, 1551.

Smith, David Eugene. Rara Arithmetica. Ginn and Company, Boston, 1908. See especially pages 233 and 235-236.

Index to Mathematical Treasures