- About MAA
- Membership
- MAA Publications
- Periodicals
- Blogs
- MAA Book Series
- MAA Press (an imprint of the AMS)
- MAA Notes
- MAA Reviews
- Mathematical Communication
- Information for Libraries
- Author Resources
- Advertise with MAA
- Meetings
- Competitions
- Programs
- Communities
- MAA Sections
- SIGMAA
- MAA Connect
- Students
- MAA Awards
- Awards Booklets
- Writing Awards
- Teaching Awards
- Service Awards
- Research Awards
- Lecture Awards
- Putnam Competition Individual and Team Winners
- D. E. Shaw Group AMC 8 Awards & Certificates
- Maryam Mirzakhani AMC 10 A Awards & Certificates
- Two Sigma AMC 10 B Awards & Certificates
- Jane Street AMC 12 A Awards & Certificates
- Akamai AMC 12 B Awards & Certificates
- High School Teachers
- News
You are here
Mathematics Magazine - June 2012
Elliptic Curves lead off this issue, as Adrian Rice and Bud Brown trace the continuous side of their history, and warn us against confusing them with ellipses. We hear from Henry Gould and Jocelyn Quaintance about combinatorial identities involving double factorials. Or would you prefer triangles? You'll find theorems about intersecting cevians and triangle centers—as well as a Miracle by Morley, but this miracle is not about triangles. —Walter Stromquist, Editor
ARTICLES
Why Ellipses Are Not Elliptic Curves
Adrian Rice and Ezra Brown
Elliptic curves are a fascinating area of algebraic geometry with important connections to number theory, topology, and complex analysis. As their current ubiquity in mathematics suggests, elliptic curves have a long and fascinating history stretching back many centuries. This paper presents a survey of key points in their development, via elliptic integrals and functions, closing with an explanation of why no elliptically-shaped planar curved line may ever be called an elliptic curve.
Double Fun with Double Factorials
Henry Gould and Jocelyn Quaintance
The double factorial of n may be defined inductively by 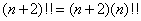 with
with 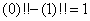 . Alternatively we may define this notion by the two relations
. Alternatively we may define this notion by the two relations 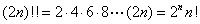 and
and  . Our object is to exhibit some properties and identities for the double factorials. Furthermore, we extend the notion of double factorial to the binomial coefficients by introducing double factorial binomial coefficients. The double factorial binomial coefficient is defined as
. Our object is to exhibit some properties and identities for the double factorials. Furthermore, we extend the notion of double factorial to the binomial coefficients by introducing double factorial binomial coefficients. The double factorial binomial coefficient is defined as  .
.
We derive identities and generating functions involving these double factorial binomial coefficients.
NOTES
The Brachistochrone Problem Solved Geometrically: A Very Elementary Approach
Raymond T. Boute
The brachistochrone is the path of swiftest descent for a particle under gravity between points not on the same vertical. The problem of finding it was posed in the 17th century, and only analytical solutions appear to be known. Here a geometrical solution is given requiring only basic properties of triangles, and the result is the cycloid. The cycloid is also shown by geometry to be Huygens's tautochrone. The presentation style is tutorial, and the geometric arguments are accessible to high school students.
My Favorite Rings
John Kiltinen and Karen Aucoin
(Tune: "My favorite things" from "The Sound of Music")
A Remarkable Combinatorial Identity
Mihail Frumosu and Alexander Teodorescu-Frumosu
We state and prove a combinatorial identity. Given an integer m, one forms all ordered partitions (or compositions) of m, then forms the product of the entries in each ordered partition. The identity combines the inverses of these products. We prove the identity by analytic methods and relate it to other identities with similar constructions.
Morley's Other Miracle
Christian Aebi and Grant Cairns
Frank Morley is famous for his theorem concerning the angle trisectors of a triangle. This note gives an elementary proof of another result of Morley's, which relates the middle binomial coefficient to a certain power of two. The striking thing about Morley's congruence is that it is valid modulo the third power of the prime being considered.
Integration by Parts without Differentiation
Vicente Muñoz
We prove the classical integration-by-parts formula without using differentiation. This provides an elementary illustration that integration by parts is valid for many non-differentiable functions. The proof is simple and accessible to undergraduates.
Two Cevians Intersecting on an Angle Bisector
Victor Oxman
We prove the next generalization of the Steiner-Lehmus theorem: if two equal cevians intersect with each other on the angle bisector of the third triangle vertex, then the triangle is isosceles.
How Rare Are Subgroups of Index 2?
Jean B. Nganou
Using elementary combinatorics and linear algebra, we compute the number of subgroups of index 2 in any finite group. This leads to necessary and sufficient conditions for groups to have no subgroups of index 2, or to have a unique subgroup of index 2. Illustrative examples are provided, along with a class of counterexamples to the converse of Lagrange's Theorem.
Inequalities Involving Six Numbers, with Applications to Triangle Geometry
Clark Kimberling and Peter Moses
The Cauchy-Schwarz inequality is generalized in a new way. The method is motivated by extremal properties of the incenter and symmedian point in the plane of a triangle with sidelengths a, b, c. Remarkably, in some of the inequalities involving six real numbers a; b; c; x; y; z, the numbers a, b, c need not be sidelengths of a triangle.
PROBLEMS
REVIEWS
Meet the Editor at MAA MathFest 2012!
The editors of the MAA journals will be offering a hands-on workshop for prospective authors of expository papers intended for submission to the MAA journals. More information.





