Mathematics Magazine - April 2006

ARTICLES

Reflecting well: Dissections of two regular polygons to one
Greg N. Frederickson
87-95
For the last half-century there have been two general methods to cut up two congruent regular polygons into pieces that we can rearrange to form a larger version of the same figure. Both methods produce elegant and symmetrical dissections and use 2n + 1 pieces for a polygon of n sides. Now the recovery of a “lost manuscript” has catalyzed the discovery of improved general methods that produce dissections with fewer pieces whenever n › 12. It’s almost enough to make you flip (the pieces, that is), but you really won’t have to.

The Evolution of the Normal Distribution
Saul Stahl
96-113
The evolution of the normal distribution is traced out. This begins with its invention as a tool for approximating cumulative binomial probabilities, goes on to its special role as an error curve and ends with a discussion of its uncanny ability to describe succinctly data sets that originate in all walks of life.

Pythagorean Triples and the Problem A = mP for Triangles
Lubomir P. Markov
114-121
A remarkable feature of the triangle (5, 12, 13) is the fact that its area equals its perimeter. Are there any other integer-sided triangles with that property? How about extending this to the case when the area is an integer multiple of the perimeter? Is it possible to find all such triangles for a fixed multiple? Our note shows that the answer is yes; the trick is to solve a Diophantine equation, resulting from a suitable rearrangement of Heron’s formula.

Euler’s ratio-sum theorem and generalizations
Branko Grünbaum and Murray S. Klamkin
122-130
The well-known theorems of Ceva and Menelaus deal with products of ratios of collinear segments associated with a triangle and certain lines; they have been generalized in many different directions. A less popular result of Euler concerns sums of ratios of segments similarly associated with a triangle. Several generalizations are presented – to simplices in all dimensions, as well as to more general polyhedra, and to other ratios of collinear segments. While Euler’s theorem and some of the generalizations yield equations, in other cases we have inequalities. In all variants we show the inequalities to be best possible. We also comment on the history of results related to the new generalizations.

NOTES

Do You Know Your Relative Driving Speed?
Mark F. Schilling
131-135
When you are driving along a busy highway, can you estimate your speed percentile among all drivers simply by comparing the number of vehicles you pass to the number that pass you? A natural estimator is the proportion of cars you pass out of the total number you see. This paper shows, under modest assumptions, that this estimator typically overestimates your extremeness in the distribution of speeds, often quite substantially.

Territorial Dynamics: Persistence in Territorial Species
Roland H. Lamberson
135-140
The widely studied and very controversial northern spotted owl, along with many other threatened and endangered species, exhibits territorial behavior. That is, adult pairs claim and defend a home range and refuse to share it with other adults of their species. Familiar population models like the logistic growth model, capture the basic concept of limited growth (carrying capacity); however they do not exhibit some fundamental characteristics of the dynamics of territorial species. In this paper we develop a model for territorial species and establish, among other things, the existence of a threshold in the density of suitable habitat below which the species is destined for extinction even if some suitable habitat is still available.

Some 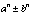Problems in Number Theory
Mihai Manea
140-145
Problems involving numbers of the form 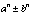appear frequently in mathematical competitions. We introduce two results that offer a unified approach to solving such problems. The first result proves that the exponent of a prime divisor, p, of a -b in aⁿ -bⁿ is the sum of the exponent of p in a – b and the exponent of p in n, while the second is concerned with the greatest common divisor of pairs of numbers that appear in the sequence in (aⁿ -bⁿ)_n-≥ . The two results provide very effective tools for solving challenging Olympiad problems involving numbers of the form .

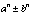Two by Two Matrices with both eigenvalues in Z/pZ
Michael P. Knapp
145-147
Suppose that p is a prime number. In an article inMathematics Magazine in 2003, Gregor Olsavsky proved a formula for the number 2 x 2 matrices with entries in the field Z/pZ which have the additional property that both eigenvalues are also in Z/pZ. This article gives a simpler proof of this result.

Irrationality of Square Roots
Peter Ungar
147-148
The irrationality of 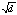is proved by producing small linear combinations of 1 and 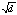by expanding 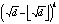. The method is then extended to solutions of monic polynomials.

PROOF WITHOUT WORDS

Putnam Proof Without Words
Robert J. MacG. Dawson
149
Problem 1A in the 2004 Wm. L. Putnam Mathematical Competition involved a basketball player whose free-throw success rate goes from less than 80% early in the season to more than 80% later in the season, asking whether it must necessarily have been exactly 80% at some point. The formal resemblance to the intermediate value theorem is, of course, completely spurious! An answer to the problem is given in the “proof-without-words” format.